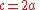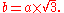Triangle
Encyclopedia
A triangle is one of the basic shape
s of geometry
: a polygon
with three corners or vertices
and three sides or edges which are line segment
s. A triangle with vertices A, B, and C is denoted .
In Euclidean geometry
any three non-collinear points determine a unique triangle and a unique plane
(i.e. a two-dimensional Euclidean space
).
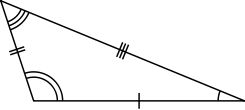
In diagrams representing triangles (and other geometric figures), "tick" marks along the sides are used to denote sides of equal lengths-- the equilateral triangle has tick marks on all 3 sides, the isosceles on 2 sides. The scalene has single, double, and triple tick marks, indicating that no sides are equal. Similarly, arcs on the inside of the vertices are used to indicate equal angles. The equilateral triangle indicates all 3 angles are equal; the isosceles shows 2 identical angles. The scalene indicates by 1, 2, and 3 arcs that no angles are equal.
s, measured here in degree
s.
A triangle that has two angles with the same measure also has two sides with the same length, and therefore it is an isosceles triangle. It follows that in a triangle where all angles have the same measure, all three sides have the same length, and such a triangle is therefore equilateral.
al plane figures, unless the context provides otherwise (see Non-planar triangles, below). In rigorous treatments, a triangle is therefore called a 2-simplex
(see also Polytope
). Elementary facts about triangles were presented by Euclid
in books 1–4 of his Elements
, around 300 BC.
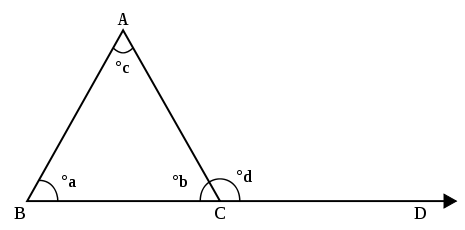The measures of the interior angles of a triangle in Euclidean space
always add up to 180 degrees. This allows determination of the measure of the third angle of any triangle given the measure of two angles. An exterior angle of a triangle is an angle that is a linear pair (and hence supplementary) to an interior angle. The measure of an exterior angle of a triangle is equal to the sum of the measures of the two interior angles that are not adjacent to it; this is the exterior angle theorem
. The sum of the measures of the three exterior angles (one for each vertex) of any triangle is 360 degrees.
The sum of the lengths of any two sides of a triangle always exceeds the length of the third side, a principle known as the triangle inequality
. Since the vertices of a triangle are assumed to be non-collinear, it is not possible for the sum of the length of two sides be equal to the length of the third side.
Two triangles are said to be similar
if every angle of one triangle has the same measure as the corresponding angle in the other triangle. The corresponding sides of similar triangles have lengths that are in the same proportion, and this property is also sufficient to establish similarity.
A few basic theorem
s about similar triangles:
Two triangles that are congruent
have exactly the same size and shape: all pairs of corresponding interior angles are equal in measure, and all pairs of corresponding sides have the same length. (This is a total of six equalities, but three are often sufficient to prove congruence.)
Some sufficient conditions for a pair of triangles to be congruent are:
An important case:
Using right triangles and the concept of similarity, the trigonometric function
s sine and cosine can be defined. These are functions of an angle
which are investigated in trigonometry
.
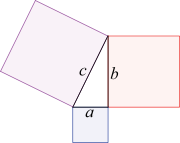A central theorem is the Pythagorean theorem
, which states in any right triangle, the square of the length of the hypotenuse
equals the sum of the squares of the lengths of the two other sides. If the hypotenuse has length c, and the legs have lengths a and b, then the theorem states that
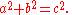
The converse is true: if the lengths of the sides of a triangle satisfy the above equation, then the triangle has a right angle opposite side c.
Some other facts about right triangles:
For all triangles, angles and sides are related by the law of cosines
and law of sines
(also called the cosine rule and sine rule).
, which gives a criterion for determining when three such lines are concurrent
. Similarly, lines associated with a triangle are often constructed by proving that three symmetrically constructed points are collinear: here Menelaus' theorem
gives a useful general criterion. In this section just a few of the most commonly encountered constructions are explained.
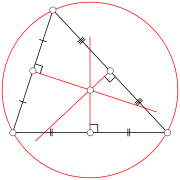A perpendicular bisector
of a side of a triangle is a straight line passing through the midpoint
of the side and being perpendicular to it, i.e. forming a right angle with it. The three perpendicular bisectors meet in a single point, the triangle's circumcenter; this point is the center of the circumcircle, the circle
passing through all three vertices. The diameter of this circle, called the circumdiameter, can be found from the law of sines stated above. The circumcircle's radius is called the circumradius.
Thales' theorem
implies that if the circumcenter is located on one side of the triangle, then the opposite angle is a right one. If the circumcenter is located inside the triangle, then the triangle is acute; if the circumcenter is located outside the triangle, then the triangle is obtuse.
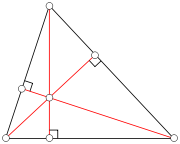An altitude
of a triangle is a straight line through a vertex and perpendicular to (i.e. forming a right angle with) the opposite side. This opposite side is called the base of the altitude, and the point where the altitude intersects the base (or its extension) is called the foot of the altitude. The length of the altitude is the distance between the base and the vertex. The three altitudes intersect in a single point, called the orthocenter of the triangle. The orthocenter lies inside the triangle if and only if the triangle is acute.
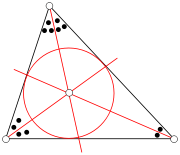An angle bisector of a triangle is a straight line through a vertex which cuts the corresponding angle in half. The three angle bisectors intersect in a single point, the incenter, the center of the triangle's incircle. The incircle is the circle which lies inside the triangle and touches all three sides. Its radius is called the inradius. There are three other important circles, the excircles; they lie outside the triangle and touch one side as well as the extensions of the other two. The centers of the in- and excircles form an orthocentric system
.
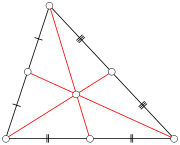A median
of a triangle is a straight line through a vertex
and the midpoint
of the opposite side, and divides the triangle into two equal areas. The three medians intersect in a single point, the triangle's centroid
or geometric barycenter. The centroid of a rigid triangular object (cut out of a thin sheet of uniform density) is also its center of mass
: the object can be balanced on its centroid in a uniform gravitational field. The centroid cuts every median in the ratio 2:1, i.e. the distance between a vertex and the centroid is twice the distance between the centroid and the midpoint of the opposite side.
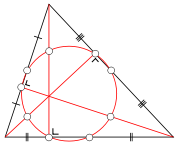The midpoints of the three sides and the feet of the three altitudes all lie on a single circle, the triangle's nine-point circle
. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the orthocenter. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the Feuerbach point) and the three excircles.
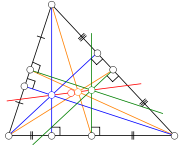The centroid (yellow), orthocenter (blue), circumcenter (green) and center of the nine-point circle (red point) all lie on a single line, known as Euler's line
(red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter.
The center of the incircle is not in general located on Euler's line.
If one reflects a median in the angle bisector that passes through the same vertex, one obtains a symmedian
. The three symmedians intersect in a single point, the symmedian point of the triangle.
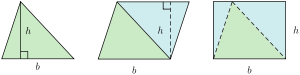Calculating the area T of a triangle is an elementary problem encountered often in many different situations. The best known and simplest formula is: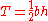
where b is the length of the base of the triangle, and h is the height or altitude of the triangle. The term 'base' denotes any side, and 'height' denotes the length of a perpendicular from the vertex opposite the side onto the line containing the side itself. In 499 CE Aryabhata
, a great mathematician
-astronomer
from the classical age of Indian mathematics
and Indian astronomy, used this method in the Aryabhatiya
(section 2.6)
.
Although simple, this formula is only useful if the height can be readily found. For example, the surveyor of a triangular field measures the length of each side, and can find the area from his results without having to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.
embedded in a three-dimensional Euclidean space
can be calculated using vectors. Let vectors AB and AC point respectively from A to B and from A to C. The area of parallelogram ABDC is then
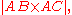
which is the magnitude of the cross product
of vectors AB and AC.
The area of triangle ABC is half of this,
.
The area of triangle ABC can also be expressed in terms of dot product
s as follows:
In two-dimensional Euclidean space, expressing vector AB as a free vector in Cartesian space equal to (x1,y1) and AC as (x2,y2), this can be rewritten as: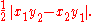
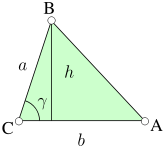The height of a triangle can be found through the application of trigonometry
.
Knowing SAS: Using the labels in the image on the left, the altitude is h = a sin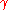
. Substituting this in the formula T = ½bh derived above, the area of the triangle can be expressed as:
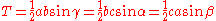
(where α is the interior angle at A, β is the interior angle at B, γ is the interior angle at C and c is the line AB).
Furthermore, since sin α = sin (π - α) = sin (β + γ), and similarly for the other two angles:
Knowing AAS:
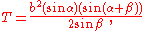
and analogously if the known side is a or c.
Knowing ASA:
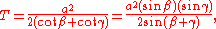
and analogously if the known side is b or c.
and the coordinates of the other two vertices are given by B = (xB, yB) and C = (xC, yC), then the area can be computed as ½ times the absolute value
of the determinant
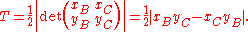
For three general vertices, the equation is:
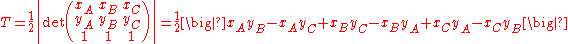
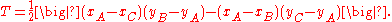
In three dimensions, the area of a general triangle {A = (xA, yA, zA), B = (xB, yB, zB) and C = (xC, yC, zC)} is the Pythagorean sum of the areas of the respective projections on the three principal planes (i.e. x = 0, y = 0 and z = 0):
If we locate the vertices in the complex plane and denote them as a=xA+yAi, b=xB+yBi, and c=xC+yCi, and denote their complex conjugates as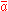
, 
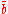, and 
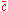, then
around the curve of the algebraic or signed distance of a point on the curve from an arbitrary oriented straight line L. Points to the right of L as oriented are taken to be at negative distance from L, while the weight for the integral is taken to be the component of arc length parallel to L rather than arc length itself.
This method is well suited to computation of the area of an arbitrary polygon
. Taking L to be the x-axis, the line integral between consecutive vertices (xi,yi) and (xi+1,yi+1) is given by the base times the mean height, namely (xi+1 − xi)(yi + yi+1)/2. The sign of the area is an overall indicator of the direction of traversal, with negative area indicating counterclockwise traversal. The area of a triangle then falls out as the case of a polygon with three sides.
While the line integral method has in common with other coordinate-based methods the arbitrary choice of a coordinate system, unlike the others it makes no arbitrary choice of vertex of the triangle as origin or of side as base. Furthermore the choice of coordinate system defined by L commits to only two degrees of freedom rather than the usual three, since the weight is a local distance (e.g. xi+1 − xi in the above) whence the method does not require choosing an axis normal to L.
When working in polar coordinates it is not necessary to convert to cartesian coordinates to use line integration, since the line integral between consecutive vertices (ri,θi) and (ri+1,θi+1) of a polygon is given directly by riri+1sin(θi+1 − θi)/2. This is valid for all values of θ, with some decrease in numerical accuracy when |θ| is many orders of magnitude greater than π. With this formulation negative area indicates clockwise traversal, which should be kept in mind when mixing polar and cartesian coordinates. Just as the choice of y-axis (x = 0) is immaterial for line integration in cartesian coordinates, so is the choice of zero heading (θ = 0) immaterial here.
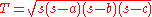
where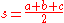
is the semiperimeter, or half of the triangle's perimeter.
Three equivalent ways of writing Heron's formula are
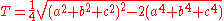
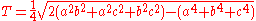
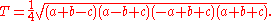
and 
and their semi-sum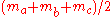 
as 
, we have
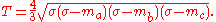
Next, denoting the altitudes from sides a, b, and c respectively as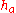
, 
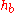, and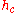 
,and denoting the semi-sum of the reciprocals of the altitudes as 
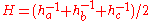we have
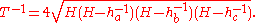
And denoting the semi-sum of the angles' sines as
, we have
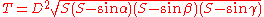
where D is the diameter of the circumcircle:
for a technique for finding the area of any arbitrary lattice polygon.
The theorem states: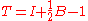
where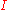
is the number of internal lattice points and 
is the number of lattice points lying on the border of the polygon.
where r is the inradius, and s is the semiperimeter
;
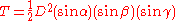
for circumdiameter D; and
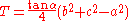
for angle
90°.
Denoting the radius of the inscribed circle as r and the radii of the excircles
as r1, r2, and r3, the area can be expressed as
In 1885, Baker gave a collection of over a hundred distinct area formulas for the triangle. These include:
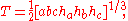
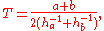
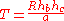
for circumradius (radius of the circumcircle) R, and
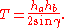
with equality holding if and only if the triangle is equilateral.
Any line through a triangle that splits both the triangle's area and its perimeter in half goes through the triangle's incenter. There can be one, two, or three of these for any given triangle.
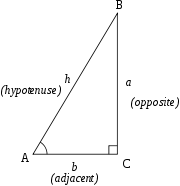In right triangle
s, the trigonometric ratios of sine, cosine and tangent can be used to find unknown angles and the lengths of unknown sides. The sides of the triangle are known as follows:
Note that this ratio does not depend on the particular right triangle chosen, as long as it contains the angle A, since all those triangles are similar.
The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. In our case
The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side. In our case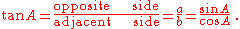
The acronym "SOH-CAH-TOA" is a useful mnemonic
for these ratios.
Arcsin can be used to calculate an angle from the length of the opposite side and the length of the hypotenuse
Arccos can be used to calculate an angle from the length of the adjacent side and the length of the hypontenuse.
Arctan can be used to calculate an angle from the length of the opposite side and the length of the adjacent side.
In introductory geometry and trigonometry courses, the notation sin−1, cos−1, etc., are often used in place of arcsin, arccos, etc. However, the arcsin, arccos, etc., notation is standard in higher mathematics where trigonometric functions are commonly raised to powers, as this avoids confusion between multiplicative inverse
and compositional inverse
.
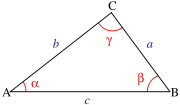The law of sines
, or sine rule, states that the ratio of the length of a side to the sine of its corresponding opposite angle is constant, that is
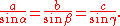
This ratio is equal to the diameter of the circumscribed circle of the given triangle. Another interpretation
of this theorem is that every triangle with angles
, 
and 
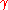
is similar to a triangle with side lengths equal to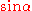
, 
and 
. This triangle can be constructed by first constructing a circle of diameter 1, and inscribing in it two of the angles of the triangle. The length of the sides of that triangle will be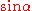 
, 
and 
. The side whose length is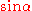 
is opposite to the angle whose measure is 
, etc.
The law of cosines
, or cosine rule, connects the length of an unknown side of a triangle to the length of the other sides and the angle opposite to the unknown side. As per the law:
For a triangle with length of sides
, 
, 
and angles of 
, 
, 
respectively, given two known lengths of a triangle 
and 
, and the angle between the two known sides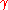 
(or the angle opposite to the unknown side 
), to calculate the third side 
, the following formula can be used: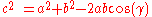
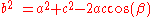
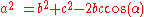
If the lengths of all three sides of any triangle are known the three angles can be calculated: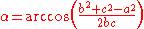
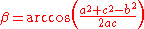
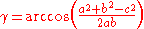
The law of tangents
or tangent rule, is less known than the other two. It states that:
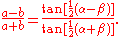
It is not used very often, but can be used to find a side or an angle when you know two sides and an angle or two angles and a side.
The medians and the sides are related by
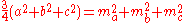
and
,
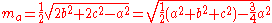and equivalently for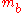
and 
.
For angle
opposite side a, the length of the internal bisector is given by
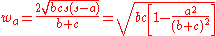
for semiperimeter s, where the bisector length is measured from the vertex to where it meets the opposite side.
The following formulas involve the circumradius R and the inradius r :
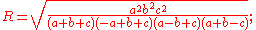
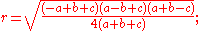
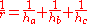
where ha etc. are the altitudes to the subscripted sides;
;
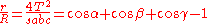and
.
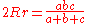Suppose two adjacent but non-overlapping triangles share the same side of length f and share the same circumcircle, so that the side of length f is a chord of the circumcircle and the triangles have side lengths (a, b, f) and (c, d, f), with the two triangles together forming a cyclic quadrilateral
with side lengths in sequence (a, b, c, d). Then
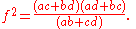
Let M be the centroid of a triangle with vertices A, B, and C, and let P be any interior point. Then the distances between the points are related by
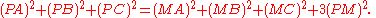
Let pa, pb, and pc be the distances from the centroid to the sides of lengths a, b, and c. Then
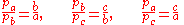
and
The product of two sides of a triangle equals the altitude to the third side times the diameter of the circumcircle.
Carnot's Theorem
states that the sum of the distances from the circumcenter to the three sides equals the sum of the circumradius and the inradius. Here a segment's length is considered to be negative if and only if the segment lies entirely outside the triangle.
Euler's theorem
states that the distance d between the circumcenter and the incenter is given by.
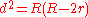
or equivalently
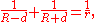
where R is the circumradius and r is the inradius. Thus for all triangles R ≥ 2r, with equality holding for equilateral triangles.
If we denote that the orthocenter divides one altitude into segments of lengths u and v, another altitude into segment lengths w and x, and the third altitude into segment lengths y and z, then uv = wx = yz.
The distance from a side to the circumcenter equals half the distance from the opposite vertex to the orthocenter.
The sum of the squares of the distances from the vertices to the orthocenter plus the sum of the squares of the sides equals twelve times the square of the circumradius.
Every triangle has a unique Steiner inellipse
which is interior to the triangle and tangent at the midpoints of the sides. Marden's theorem
shows how to find the foci of this ellipse.
Every triangle has three inscribed squares (squares in its interior such that all four of a square's vertices lie on a side of the triangle, so two of them lie on the same side and hence one side of the square coincides with part of a side of the triangle). However, in the case of a right triangle two of the squares coincide and have a vertex at the triangle's right angle, so a right triangle has only two distinct inscribed squares. Within a given triangle, a longer common side is associated with a smaller inscribed square. If an inscribed square has side of length s and the triangle has a side of length x, part of which side coincides with a side of the square, then s, x, and the triangle's area T are related according to
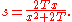
The largest possible ratio of the area of the inscribed square to the area of the triangle is 1/2, which occurs when
, s=x/2, and the altitude of the triangle from base x equals x.
and hyperbolic triangle
s in hyperbolic geometry
.
While the measures of the internal angles in planar triangles always sum to 180°, a hyperbolic triangle has measures of angles that sum to less than 180°, and a spherical triangle has measures of angles that sum to more than 180°. A hyperbolic triangle can be obtained by drawing on a negatively curved surface, such as a saddle surface
, and a spherical triangle can be obtained by drawing on a positively curved surface such as a sphere
. Thus, if one draws a giant triangle on the surface of the Earth, one will find that the sum of the measures of its angles is greater than 180°; in fact it will be between 180° and 540°. In particular it is possible to draw a triangle on a sphere such that the measure of each of its internal angles is equal to 90°, adding up to a total of 270°.
Specifically, on a sphere the sum of the angles of a triangle is
where f is the fraction of the sphere's area which is enclosed by the triangle. For example, suppose that we draw a triangle on the Earth's surface with vertices at the North Pole, at a point on the equator at 0° longitude, and a point on the equator at 90° West longitude. The great circle
line between the latter two points is the equator, and the great circle line between either of those points and the North Pole is a line of longitude; so there are right angles at the two points on the equator. Moreover, the angle at the North Pole is also 90° because the other two vertices differ by 90° of longitude. So the sum of the angles in this triangle is 90°+90°+90°=270°. The triangle encloses 1/4 of the northern hemisphere (90°/360° as viewed from the North Pole) and therefore 1/8 of the Earth's surface, so in the formula f = 1/8; thus the formula correctly gives the sum of the triangle's angles as 270°.
From the above angle sum formula we can also see that the Earth's surface is locally flat: If we draw an arbitrarily small triangle in the neighborhood of one point on the Earth's surface, the fraction f of the Earth's surface which is enclosed by the triangle will be arbitrarily close to zero. In this case the angle sum formula simplifies to 180°, which we know is what Euclidean geometry tells us for triangles on a flat surface.
s have been the most popular and common geometric form for buildings since the shape is easy to stack and organize; as a standard, it is easy to design furniture and fixtures to fit inside rectangularly-shaped buildings. But triangles, while more difficult to use conceptually, provide a great deal of strength. As computer technology helps architect
s design creative new buildings, triangular shapes are becoming increasingly prevalent as parts of buildings and as the primary shape for some types of skyscrapers as well as building materials. In Tokyo in 1989, architects had wondered whether it was possible to build a 500 story tower to provide affordable office space for this densely packed city, but with the danger to buildings from earthquake
s, architects considered that a triangular shape would have been necessary if such a building was ever to have been built (it hasn't by 2011). In New York City, as Broadway
crisscrosses major avenues, the resulting blocks are cut like triangles, and buildings have been built on these shapes; one such building is the triangularly-shaped Flatiron Building
which real estate people admit has a "warren of awkward spaces that do not easily accommodate modern office furniture" but that has not prevented the structure from becoming a landmark icon. Designers have made houses in Norway
using triangular themes. Triangle shapes have appeared in churches as well as public buildings including colleges as well as supports for innovative home designs. Triangles are sturdy; while a rectangle can collapse into a parallelogram
from pressure to one of its points, triangles have a natural strength which supports structures against lateral pressures. A triangle will not change shape unless its sides are bent or extended or broken or if its joints break; in essence, each of the three sides supports the other two. A rectangle, in contrast, is more dependent on the strength of its joints in a structural sense. Some innovative designers have proposed making bricks
not out of rectangles, but with triangular shapes which can be combined in three dimensions. It is likely that triangles will be used increasingly in new ways as architecture increases in complexity.
Shape
The shape of an object located in some space is a geometrical description of the part of that space occupied by the object, as determined by its external boundary – abstracting from location and orientation in space, size, and other properties such as colour, content, and material...
s of geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
: a polygon
Polygon
In geometry a polygon is a flat shape consisting of straight lines that are joined to form a closed chain orcircuit.A polygon is traditionally a plane figure that is bounded by a closed path, composed of a finite sequence of straight line segments...
with three corners or vertices
Vertex (geometry)
In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...
and three sides or edges which are line segment
Line segment
In geometry, a line segment is a part of a line that is bounded by two end points, and contains every point on the line between its end points. Examples of line segments include the sides of a triangle or square. More generally, when the end points are both vertices of a polygon, the line segment...
s. A triangle with vertices A, B, and C is denoted .
In Euclidean geometry
Euclidean geometry
Euclidean geometry is a mathematical system attributed to the Alexandrian Greek mathematician Euclid, which he described in his textbook on geometry: the Elements. Euclid's method consists in assuming a small set of intuitively appealing axioms, and deducing many other propositions from these...
any three non-collinear points determine a unique triangle and a unique plane
Plane (mathematics)
In mathematics, a plane is a flat, two-dimensional surface. A plane is the two dimensional analogue of a point , a line and a space...
(i.e. a two-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
).
Types of triangles
By relative lengths of sides
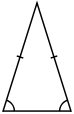
Triangles can be classified according to the relative lengths of their sides:- In an equilateral triangle all sides have the same length. An equilateral triangle is also a regular polygonRegular polygonA regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...
with all angles measuring 60°. - In an isosceles triangle, two sides are equal in length. An isosceles triangle also has two angles of the same measure; namely, the angles opposite to the two sides of the same length; this fact is the content of the Isosceles triangle theoremIsosceles triangle theoremIn Euclidean geometry, the isosceles triangle theorem, also known as the pons asinorum, states that the angles opposite the two equal sides of an isosceles triangle are equal...
. Some mathematicians define an isosceles triangle to have exactly two equal sides, whereas others define an isosceles triangle as one with at least two equal sides. The latter definition would make all equilateral triangles isosceles triangles. The 45-45-90 Right Triangle, which appears in the Tetrakis square tilingTetrakis square tilingIn geometry, the tetrakis square tiling is a tiling of the Euclidean plane. It is square tiling with each square divided into four triangles from the center point, forming an infinite arrangement of lines....
, is isosceles. - In a scalene triangle, all sides are unequal. The three angles are also all different in measure. Some (but not all) scalene triangles are also right triangles.
 |  |  |
| Equilateral | Isosceles | Scalene |
In diagrams representing triangles (and other geometric figures), "tick" marks along the sides are used to denote sides of equal lengths-- the equilateral triangle has tick marks on all 3 sides, the isosceles on 2 sides. The scalene has single, double, and triple tick marks, indicating that no sides are equal. Similarly, arcs on the inside of the vertices are used to indicate equal angles. The equilateral triangle indicates all 3 angles are equal; the isosceles shows 2 identical angles. The scalene indicates by 1, 2, and 3 arcs that no angles are equal.
By internal angles
Triangles can also be classified according to their internal angleInternal angle
In geometry, an interior angle is an angle formed by two sides of a polygon that share an endpoint. For a simple, convex or concave polygon, this angle will be an angle on the 'inner side' of the polygon...
s, measured here in degree
Degree (angle)
A degree , usually denoted by ° , is a measurement of plane angle, representing 1⁄360 of a full rotation; one degree is equivalent to π/180 radians...
s.
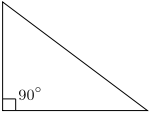
- A right triangleRight triangleA right triangle or right-angled triangle is a triangle in which one angle is a right angle . The relation between the sides and angles of a right triangle is the basis for trigonometry.-Terminology:The side opposite the right angle is called the hypotenuse...
(or right-angled triangle, formerly called a rectangled triangle) has one of its interior angles measuring 90° (a right angleAngleIn geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...
). The side opposite to the right angle is the hypotenuseHypotenuseIn geometry, a hypotenuse is the longest side of a right-angled triangle, the side opposite the right angle. The length of the hypotenuse of a right triangle can be found using the Pythagorean theorem, which states that the square of the length of the hypotenuse equals the sum of the squares of the...
; it is the longest side of the right triangle. The other two sides are called the legs or catheti (singular: cathetus) of the triangle. Right triangles obey the Pythagorean theoremPythagorean theoremIn mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a right triangle...
: the sum of the squares of the lengths of the two legs is equal to the square of the length of the hypotenuse: a2 + b2 = c2, where a and b are the lengths of the legs and c is the length of the hypotenuse. Special right trianglesSpecial right trianglesA special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist. For example, a right triangle may have angles that form simple relationships, such as 45-45-90. This is called an "angle-based" right triangle...
are right triangles with additional properties that make calculations involving them easier. One of the two most famous is the 3-4-5 right triangle, where 32 + 42 = 52. In this situation, 3, 4, and 5 are a Pythagorean TriplePythagorean tripleA Pythagorean triple consists of three positive integers a, b, and c, such that . Such a triple is commonly written , and a well-known example is . If is a Pythagorean triple, then so is for any positive integer k. A primitive Pythagorean triple is one in which a, b and c are pairwise coprime...
. The other one is an isosceles triangle that has 2 angles that each measure 45 degrees. - Triangles that do not have an angle that measures 90° are called oblique triangles.
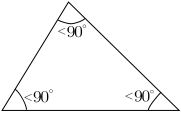
- A triangle that has all interior angles measuring less than 90° is an acute triangle or acute-angled triangle.
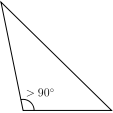
- A triangle that has one angle that measures more than 90° is an obtuse triangle or obtuse-angled triangle.
- A "triangle" with an interior angle of 180° (and collinear vertices) is degenerateDegeneracy (mathematics)In mathematics, a degenerate case is a limiting case in which a class of object changes its nature so as to belong to another, usually simpler, class....
.
A triangle that has two angles with the same measure also has two sides with the same length, and therefore it is an isosceles triangle. It follows that in a triangle where all angles have the same measure, all three sides have the same length, and such a triangle is therefore equilateral.
 |  |  |
| Right | Obtuse | Acute |
 | ||
Basic facts
Triangles are assumed to be two-dimensionDimension
In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...
al plane figures, unless the context provides otherwise (see Non-planar triangles, below). In rigorous treatments, a triangle is therefore called a 2-simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
(see also Polytope
Polytope
In elementary geometry, a polytope is a geometric object with flat sides, which exists in any general number of dimensions. A polygon is a polytope in two dimensions, a polyhedron in three dimensions, and so on in higher dimensions...
). Elementary facts about triangles were presented by Euclid
Euclid
Euclid , fl. 300 BC, also known as Euclid of Alexandria, was a Greek mathematician, often referred to as the "Father of Geometry". He was active in Alexandria during the reign of Ptolemy I...
in books 1–4 of his Elements
Euclid's Elements
Euclid's Elements is a mathematical and geometric treatise consisting of 13 books written by the Greek mathematician Euclid in Alexandria c. 300 BC. It is a collection of definitions, postulates , propositions , and mathematical proofs of the propositions...
, around 300 BC.
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
always add up to 180 degrees. This allows determination of the measure of the third angle of any triangle given the measure of two angles. An exterior angle of a triangle is an angle that is a linear pair (and hence supplementary) to an interior angle. The measure of an exterior angle of a triangle is equal to the sum of the measures of the two interior angles that are not adjacent to it; this is the exterior angle theorem
Exterior angle theorem
The exterior angle theorem can mean one of two things: Postulate 1.16 in Euclid's Elements which states that the exterior angle of a triangle is bigger than either of the remote interior angles, or a theorem in elementary geometry which states that the exterior angle of a triangle is equal to the...
. The sum of the measures of the three exterior angles (one for each vertex) of any triangle is 360 degrees.
The sum of the lengths of any two sides of a triangle always exceeds the length of the third side, a principle known as the triangle inequality
Triangle inequality
In mathematics, the triangle inequality states that for any triangle, the sum of the lengths of any two sides must be greater than or equal to the length of the remaining side ....
. Since the vertices of a triangle are assumed to be non-collinear, it is not possible for the sum of the length of two sides be equal to the length of the third side.
Two triangles are said to be similar
Similarity (geometry)
Two geometrical objects are called similar if they both have the same shape. More precisely, either one is congruent to the result of a uniform scaling of the other...
if every angle of one triangle has the same measure as the corresponding angle in the other triangle. The corresponding sides of similar triangles have lengths that are in the same proportion, and this property is also sufficient to establish similarity.
A few basic theorem
Theorem
In mathematics, a theorem is a statement that has been proven on the basis of previously established statements, such as other theorems, and previously accepted statements, such as axioms...
s about similar triangles:
- If two corresponding internal angles of two triangles have the same measure, the triangles are similar.
- If two corresponding sides of two triangles are in proportion, and their included angles have the same measure, then the triangles are similar. (The included angle for any two sides of a polygon is the internal angle between those two sides.)
- If three corresponding sides of two triangles are in proportion, then the triangles are similar.
Two triangles that are congruent
Congruence (geometry)
In geometry, two figures are congruent if they have the same shape and size. This means that either object can be repositioned so as to coincide precisely with the other object...
have exactly the same size and shape: all pairs of corresponding interior angles are equal in measure, and all pairs of corresponding sides have the same length. (This is a total of six equalities, but three are often sufficient to prove congruence.)
Some sufficient conditions for a pair of triangles to be congruent are:
- SAS Postulate: Two sides in a triangle have the same length as two sides in the other triangle, and the included angles have the same measure.
- ASA: Two interior angles and the included side in a triangle have the same measure and length, respectively, as those in the other triangle. (The included side for a pair of angles is the side that is common to them.)
- SSS: Each side of a triangle has the same length as a corresponding side of the other triangle.
- AAS: Two angles and a corresponding (non-included) side in a triangle have the same measure and length, respectively, as those in the other triangle. (This is sometimes referred to as AAcorrS and then includes ASA above.)
- Hypotenuse-Leg (HL) Theorem: The hypotenuse and a leg in a right triangle have the same length as those in another right triangle. This is also called RHS (right-angle, hypotenuse, side).
- Hypotenuse-Angle Theorem: The hypotenuse and an acute angle in one right triangle have the same length and measure, respectively, as those in the other right triangle. This is just a particular case of the AAS theorem.
An important case:
- Side-Side-Angle (or Angle-Side-Side) condition: If two sides and a corresponding non-included angle of a triangle have the same length and measure, respectively, as those in another triangle, then this is not sufficient to prove congruence; but if the angle given is opposite to the longer side of the two sides, then the triangles are congruent. The Hypotenuse-Leg Theorem is a particular case of this criterion. The Side-Side-Angle condition does not by itself guarantee that the triangles are congruent because one triangle could be obtuse-angled and the other acute-angled.
Using right triangles and the concept of similarity, the trigonometric function
Trigonometric function
In mathematics, the trigonometric functions are functions of an angle. They are used to relate the angles of a triangle to the lengths of the sides of a triangle...
s sine and cosine can be defined. These are functions of an angle
Angle
In geometry, an angle is the figure formed by two rays sharing a common endpoint, called the vertex of the angle.Angles are usually presumed to be in a Euclidean plane with the circle taken for standard with regard to direction. In fact, an angle is frequently viewed as a measure of an circular arc...
which are investigated in trigonometry
Trigonometry
Trigonometry is a branch of mathematics that studies triangles and the relationships between their sides and the angles between these sides. Trigonometry defines the trigonometric functions, which describe those relationships and have applicability to cyclical phenomena, such as waves...
.
Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a right triangle...
, which states in any right triangle, the square of the length of the hypotenuse
Hypotenuse
In geometry, a hypotenuse is the longest side of a right-angled triangle, the side opposite the right angle. The length of the hypotenuse of a right triangle can be found using the Pythagorean theorem, which states that the square of the length of the hypotenuse equals the sum of the squares of the...
equals the sum of the squares of the lengths of the two other sides. If the hypotenuse has length c, and the legs have lengths a and b, then the theorem states that
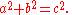
The converse is true: if the lengths of the sides of a triangle satisfy the above equation, then the triangle has a right angle opposite side c.
Some other facts about right triangles:
- The acute angles of a right triangle are complementaryComplementary anglesIn geometry, complementary angles are angles whose measures sum to 90°. If the two complementary angles are adjacent their non-shared sides form a right angle....
.
- If the legs of a right triangle have the same length, then the angles opposite those legs have the same measure. Since these angles are complementary, it follows that each measures 45 degrees. By the Pythagorean theorem, the length of the hypotenuse is the length of a leg times √2.
- In a right triangle with acute angles measuring 30 and 60 degrees, the hypotenuse is twice the length of the shorter side, and the longer side is equal to the length of the shorter side times √3 :
For all triangles, angles and sides are related by the law of cosines
Law of cosines
In trigonometry, the law of cosines relates the lengths of the sides of a plane triangle to the cosine of one of its angles. Using notation as in Fig...
and law of sines
Law of sines
In trigonometry, the law of sines is an equation relating the lengths of the sides of an arbitrary triangle to the sines of its angles...
(also called the cosine rule and sine rule).
Points, lines, and circles associated with a triangle
There are hundreds of different constructions that find a special point associated with (and often inside) a triangle, satisfying some unique property: see the references section for a catalogue of them. Often they are constructed by finding three lines associated in a symmetrical way with the three sides (or vertices) and then proving that the three lines meet in a single point: an important tool for proving the existence of these is Ceva's theoremCeva's theorem
Ceva's theorem is a theorem about triangles in plane geometry. Given a triangle ABC, let the lines AO, BO and CO be drawn from the vertices to a common point O to meet opposite sides at D, E and F respectively...
, which gives a criterion for determining when three such lines are concurrent
Concurrent lines
In geometry, two or more lines are said to be concurrent if they intersect at a single point.In a triangle, four basic types of sets of concurrent lines are altitudes, angle bisectors, medians, and perpendicular bisectors:...
. Similarly, lines associated with a triangle are often constructed by proving that three symmetrically constructed points are collinear: here Menelaus' theorem
Menelaus' theorem
Menelaus' theorem, named for Menelaus of Alexandria, is a theorem about triangles in plane geometry. Given a triangle ABC, and a transversal line that crosses BC, AC and AB at points D, E and F respectively, with D, E, and F distinct from A, B and C, thenThis equation uses signed lengths of...
gives a useful general criterion. In this section just a few of the most commonly encountered constructions are explained.
Bisection
In geometry, bisection is the division of something into two equal or congruent parts, usually by a line, which is then called a bisector. The most often considered types of bisectors are the segment bisector and the angle bisector In geometry, bisection is the division of something into two equal...
of a side of a triangle is a straight line passing through the midpoint
Midpoint
The midpoint is the middle point of a line segment. It is equidistant from both endpoints.-Formulas:...
of the side and being perpendicular to it, i.e. forming a right angle with it. The three perpendicular bisectors meet in a single point, the triangle's circumcenter; this point is the center of the circumcircle, the circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
passing through all three vertices. The diameter of this circle, called the circumdiameter, can be found from the law of sines stated above. The circumcircle's radius is called the circumradius.
Thales' theorem
Thales' theorem
In geometry, Thales' theorem states that if A, B and C are points on a circle where the line AC is a diameter of the circle, then the angle ABC is a right angle. Thales' theorem is a special case of the inscribed angle theorem...
implies that if the circumcenter is located on one side of the triangle, then the opposite angle is a right one. If the circumcenter is located inside the triangle, then the triangle is acute; if the circumcenter is located outside the triangle, then the triangle is obtuse.
Altitude (triangle)
In geometry, an altitude of a triangle is a straight line through a vertex and perpendicular to a line containing the base . This line containing the opposite side is called the extended base of the altitude. The intersection between the extended base and the altitude is called the foot of the...
of a triangle is a straight line through a vertex and perpendicular to (i.e. forming a right angle with) the opposite side. This opposite side is called the base of the altitude, and the point where the altitude intersects the base (or its extension) is called the foot of the altitude. The length of the altitude is the distance between the base and the vertex. The three altitudes intersect in a single point, called the orthocenter of the triangle. The orthocenter lies inside the triangle if and only if the triangle is acute.
Orthocentric system
In geometry, an orthocentric system is a set of four points in the plane one of which is the orthocenter of the triangle formed by the other three....
.
Median (geometry)
In geometry, a median of a triangle is a line segment joining a vertex to the midpoint of the opposing side. Every triangle has exactly three medians; one running from each vertex to the opposite side...
of a triangle is a straight line through a vertex
Vertex (geometry)
In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...
and the midpoint
Midpoint
The midpoint is the middle point of a line segment. It is equidistant from both endpoints.-Formulas:...
of the opposite side, and divides the triangle into two equal areas. The three medians intersect in a single point, the triangle's centroid
Centroid
In geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...
or geometric barycenter. The centroid of a rigid triangular object (cut out of a thin sheet of uniform density) is also its center of mass
Center of mass
In physics, the center of mass or barycenter of a system is the average location of all of its mass. In the case of a rigid body, the position of the center of mass is fixed in relation to the body...
: the object can be balanced on its centroid in a uniform gravitational field. The centroid cuts every median in the ratio 2:1, i.e. the distance between a vertex and the centroid is twice the distance between the centroid and the midpoint of the opposite side.
Nine-point circle
In geometry, the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant points defined from the triangle...
. The remaining three points for which it is named are the midpoints of the portion of altitude between the vertices and the orthocenter. The radius of the nine-point circle is half that of the circumcircle. It touches the incircle (at the Feuerbach point) and the three excircles.
Euler's line
In geometry, the Euler line, named after Leonhard Euler, is a line determined from any triangle that is not equilateral; it passes through several important points determined from the triangle...
(red line). The center of the nine-point circle lies at the midpoint between the orthocenter and the circumcenter, and the distance between the centroid and the circumcenter is half that between the centroid and the orthocenter.
The center of the incircle is not in general located on Euler's line.
If one reflects a median in the angle bisector that passes through the same vertex, one obtains a symmedian
Symmedian
Symmedians are three particular geometrical lines associated with every triangle. They are constructed by taking a median of the triangle , and reflecting the line over the corresponding angle bisector...
. The three symmedians intersect in a single point, the symmedian point of the triangle.
Computing the area of a triangle
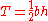
where b is the length of the base of the triangle, and h is the height or altitude of the triangle. The term 'base' denotes any side, and 'height' denotes the length of a perpendicular from the vertex opposite the side onto the line containing the side itself. In 499 CE Aryabhata
Aryabhata
Aryabhata was the first in the line of great mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy...
, a great mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
-astronomer
Astronomer
An astronomer is a scientist who studies celestial bodies such as planets, stars and galaxies.Historically, astronomy was more concerned with the classification and description of phenomena in the sky, while astrophysics attempted to explain these phenomena and the differences between them using...
from the classical age of Indian mathematics
Indian mathematics
Indian mathematics emerged in the Indian subcontinent from 1200 BCE until the end of the 18th century. In the classical period of Indian mathematics , important contributions were made by scholars like Aryabhata, Brahmagupta, and Bhaskara II. The decimal number system in use today was first...
and Indian astronomy, used this method in the Aryabhatiya
Aryabhatiya
Āryabhaṭīya or Āryabhaṭīyaṃ, a Sanskrit astronomical treatise, is the magnum opus and only extant work of the 5th century Indian mathematician, Āryabhaṭa.- Structure and style:...
(section 2.6)
.
Although simple, this formula is only useful if the height can be readily found. For example, the surveyor of a triangular field measures the length of each side, and can find the area from his results without having to construct a 'height'. Various methods may be used in practice, depending on what is known about the triangle. The following is a selection of frequently used formulae for the area of a triangle.
Using vectors
The area of a parallelogramParallelogram
In Euclidean geometry, a parallelogram is a convex quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure...
embedded in a three-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
can be calculated using vectors. Let vectors AB and AC point respectively from A to B and from A to C. The area of parallelogram ABDC is then
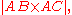
which is the magnitude of the cross product
Cross product
In mathematics, the cross product, vector product, or Gibbs vector product is a binary operation on two vectors in three-dimensional space. It results in a vector which is perpendicular to both of the vectors being multiplied and normal to the plane containing them...
of vectors AB and AC.
The area of triangle ABC is half of this,
.The area of triangle ABC can also be expressed in terms of dot product
Dot product
In mathematics, the dot product or scalar product is an algebraic operation that takes two equal-length sequences of numbers and returns a single number obtained by multiplying corresponding entries and then summing those products...
s as follows:
In two-dimensional Euclidean space, expressing vector AB as a free vector in Cartesian space equal to (x1,y1) and AC as (x2,y2), this can be rewritten as:
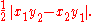
Using trigonometry
Trigonometry
Trigonometry is a branch of mathematics that studies triangles and the relationships between their sides and the angles between these sides. Trigonometry defines the trigonometric functions, which describe those relationships and have applicability to cyclical phenomena, such as waves...
.
Knowing SAS: Using the labels in the image on the left, the altitude is h = a sin
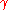. Substituting this in the formula T = ½bh derived above, the area of the triangle can be expressed as: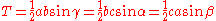(where α is the interior angle at A, β is the interior angle at B, γ is the interior angle at C and c is the line AB).
Furthermore, since sin α = sin (π - α) = sin (β + γ), and similarly for the other two angles:
Knowing AAS:
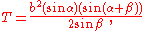
and analogously if the known side is a or c.
Knowing ASA:
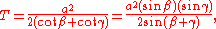
and analogously if the known side is b or c.
Using coordinates
If vertex A is located at the origin (0, 0) of a Cartesian coordinate systemCartesian coordinate system
A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
and the coordinates of the other two vertices are given by B = (xB, yB) and C = (xC, yC), then the area can be computed as ½ times the absolute value
Absolute value
In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...
of the determinant
Determinant
In linear algebra, the determinant is a value associated with a square matrix. It can be computed from the entries of the matrix by a specific arithmetic expression, while other ways to determine its value exist as well...
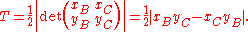
For three general vertices, the equation is:
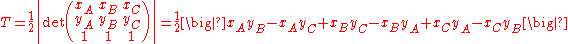
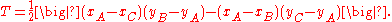
In three dimensions, the area of a general triangle {A = (xA, yA, zA), B = (xB, yB, zB) and C = (xC, yC, zC)} is the Pythagorean sum of the areas of the respective projections on the three principal planes (i.e. x = 0, y = 0 and z = 0):
If we locate the vertices in the complex plane and denote them as a=xA+yAi, b=xB+yBi, and c=xC+yCi, and denote their complex conjugates as
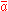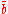, , and 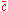, thenUsing line integrals
The area within any closed curve, such as a triangle, is given by the line integralLine integral
In mathematics, a line integral is an integral where the function to be integrated is evaluated along a curve.The function to be integrated may be a scalar field or a vector field...
around the curve of the algebraic or signed distance of a point on the curve from an arbitrary oriented straight line L. Points to the right of L as oriented are taken to be at negative distance from L, while the weight for the integral is taken to be the component of arc length parallel to L rather than arc length itself.
This method is well suited to computation of the area of an arbitrary polygon
Polygon
In geometry a polygon is a flat shape consisting of straight lines that are joined to form a closed chain orcircuit.A polygon is traditionally a plane figure that is bounded by a closed path, composed of a finite sequence of straight line segments...
. Taking L to be the x-axis, the line integral between consecutive vertices (xi,yi) and (xi+1,yi+1) is given by the base times the mean height, namely (xi+1 − xi)(yi + yi+1)/2. The sign of the area is an overall indicator of the direction of traversal, with negative area indicating counterclockwise traversal. The area of a triangle then falls out as the case of a polygon with three sides.
While the line integral method has in common with other coordinate-based methods the arbitrary choice of a coordinate system, unlike the others it makes no arbitrary choice of vertex of the triangle as origin or of side as base. Furthermore the choice of coordinate system defined by L commits to only two degrees of freedom rather than the usual three, since the weight is a local distance (e.g. xi+1 − xi in the above) whence the method does not require choosing an axis normal to L.
When working in polar coordinates it is not necessary to convert to cartesian coordinates to use line integration, since the line integral between consecutive vertices (ri,θi) and (ri+1,θi+1) of a polygon is given directly by riri+1sin(θi+1 − θi)/2. This is valid for all values of θ, with some decrease in numerical accuracy when |θ| is many orders of magnitude greater than π. With this formulation negative area indicates clockwise traversal, which should be kept in mind when mixing polar and cartesian coordinates. Just as the choice of y-axis (x = 0) is immaterial for line integration in cartesian coordinates, so is the choice of zero heading (θ = 0) immaterial here.
Using Heron's formula
The shape of the triangle is determined by the lengths of the sides alone. Therefore the area can also be derived from the lengths of the sides. By Heron's formula: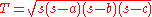where
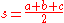is the semiperimeter, or half of the triangle's perimeter.Three equivalent ways of writing Heron's formula are
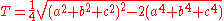
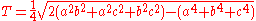
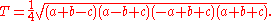
Formulas mimicking Heron's formula
Three formulas have the same structure as Heron's formula but are expressed in terms of different variables. First, denoting the medians from sides a, b, and c respectively as and and their semi-sum 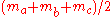as , we have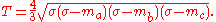Next, denoting the altitudes from sides a, b, and c respectively as
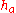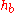, , and 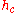,and denoting the semi-sum of the reciprocals of the altitudes as 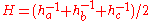we have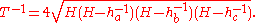And denoting the semi-sum of the angles' sines as
, we have 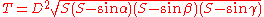where D is the diameter of the circumcircle:
Using Pick's Theorem
See Pick's theoremPick's theorem
Given a simple polygon constructed on a grid of equal-distanced points such that all the polygon's vertices are grid points, Pick's theorem provides a simple formula for calculating the area A of this polygon in terms of the number i of lattice points in the interior located in the polygon and the...
for a technique for finding the area of any arbitrary lattice polygon.
The theorem states:
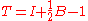
where
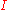is the number of internal lattice points and is the number of lattice points lying on the border of the polygon.Other area formulas
Numerous other area formulas exist, such aswhere r is the inradius, and s is the semiperimeter
Semiperimeter
In geometry, the semiperimeter of a polygon is half its perimeter. Although it has such a simple derivation from the perimeter, the semiperimeter appears frequently enough in formulas for triangles and other figures that it is given a separate name...
;
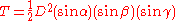
for circumdiameter D; and
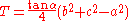
for angle
90°.Denoting the radius of the inscribed circle as r and the radii of the excircles
Incircle and excircles of a triangle
In geometry, the incircle or inscribed circle of a triangle is the largest circle contained in the triangle; it touches the three sides...
as r1, r2, and r3, the area can be expressed as
In 1885, Baker gave a collection of over a hundred distinct area formulas for the triangle. These include:
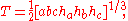
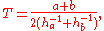
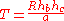
for circumradius (radius of the circumcircle) R, and
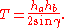
Upper bound on the area
The area of any triangle with perimeter p is less than or equal to with equality holding if and only if the triangle is equilateral.Bisecting the area
There are infinitely many lines that bisect the area of a triangle. Three of them are the medians, which are the only area bisectors that go through the centroid. Three other area bisectors are parallel to the triangle's sides.Any line through a triangle that splits both the triangle's area and its perimeter in half goes through the triangle's incenter. There can be one, two, or three of these for any given triangle.
Computing the sides and angles
There are various standard methods for calculating the length of a side or the size of an angle. Certain methods are suited to calculating values in a right-angled triangle; more complex methods may be required in other situations.Trigonometric ratios in right triangles
Right triangle
A right triangle or right-angled triangle is a triangle in which one angle is a right angle . The relation between the sides and angles of a right triangle is the basis for trigonometry.-Terminology:The side opposite the right angle is called the hypotenuse...
s, the trigonometric ratios of sine, cosine and tangent can be used to find unknown angles and the lengths of unknown sides. The sides of the triangle are known as follows:
- The hypotenuseHypotenuseIn geometry, a hypotenuse is the longest side of a right-angled triangle, the side opposite the right angle. The length of the hypotenuse of a right triangle can be found using the Pythagorean theorem, which states that the square of the length of the hypotenuse equals the sum of the squares of the...
is the side opposite the right angle, or defined as the longest side of a right-angled triangle, in this case h. - The opposite side is the side opposite to the angle we are interested in, in this case a.
- The adjacent side is the side that is in contact with the angle we are interested in and the right angle, hence its name. In this case the adjacent side is b.
Sine, cosine and tangent
The sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse. In our caseNote that this ratio does not depend on the particular right triangle chosen, as long as it contains the angle A, since all those triangles are similar.
The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse. In our case
The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side. In our case
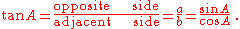
The acronym "SOH-CAH-TOA" is a useful mnemonic
Mnemonic
A mnemonic , or mnemonic device, is any learning technique that aids memory. To improve long term memory, mnemonic systems are used to make memorization easier. Commonly encountered mnemonics are often verbal, such as a very short poem or a special word used to help a person remember something,...
for these ratios.
Inverse functions
The inverse trigonometric functions can be used to calculate the internal angles for a right angled triangle with the length of any two sides.Arcsin can be used to calculate an angle from the length of the opposite side and the length of the hypotenuse
Arccos can be used to calculate an angle from the length of the adjacent side and the length of the hypontenuse.
Arctan can be used to calculate an angle from the length of the opposite side and the length of the adjacent side.
In introductory geometry and trigonometry courses, the notation sin−1, cos−1, etc., are often used in place of arcsin, arccos, etc. However, the arcsin, arccos, etc., notation is standard in higher mathematics where trigonometric functions are commonly raised to powers, as this avoids confusion between multiplicative inverse
Multiplicative inverse
In mathematics, a multiplicative inverse or reciprocal for a number x, denoted by 1/x or x−1, is a number which when multiplied by x yields the multiplicative identity, 1. The multiplicative inverse of a fraction a/b is b/a. For the multiplicative inverse of a real number, divide 1 by the...
and compositional inverse
Inverse function
In mathematics, an inverse function is a function that undoes another function: If an input x into the function ƒ produces an output y, then putting y into the inverse function g produces the output x, and vice versa. i.e., ƒ=y, and g=x...
.
The sine, cosine and tangent rules
Law of sines
In trigonometry, the law of sines is an equation relating the lengths of the sides of an arbitrary triangle to the sines of its angles...
, or sine rule, states that the ratio of the length of a side to the sine of its corresponding opposite angle is constant, that is
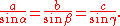
This ratio is equal to the diameter of the circumscribed circle of the given triangle. Another interpretation
of this theorem is that every triangle with angles
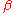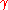
, and is similar to a triangle with side lengths equal to
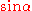, and . This triangle can be constructed by first constructing a circle of diameter 1, and inscribing in it two of the angles of the triangle. The length of the sides of that triangle will be 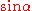, and . The side whose length is 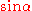is opposite to the angle whose measure is , etc.The law of cosines
Law of cosines
In trigonometry, the law of cosines relates the lengths of the sides of a plane triangle to the cosine of one of its angles. Using notation as in Fig...
, or cosine rule, connects the length of an unknown side of a triangle to the length of the other sides and the angle opposite to the unknown side. As per the law:
For a triangle with length of sides
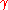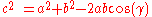
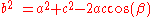
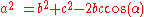
, , and angles of , , respectively, given two known lengths of a triangle and , and the angle between the two known sides (or the angle opposite to the unknown side ), to calculate the third side , the following formula can be used:If the lengths of all three sides of any triangle are known the three angles can be calculated:
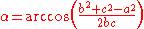
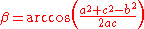
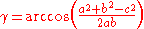
The law of tangents
Law of tangents
In trigonometry, the law of tangents is a statement about the relationship between the tangents of two angles of a triangle and the lengths of the opposite sides....
or tangent rule, is less known than the other two. It states that:
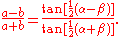
It is not used very often, but can be used to find a side or an angle when you know two sides and an angle or two angles and a side.
Further formulas for general Euclidean triangles
The formulas in this section are true for all Euclidean triangles.The medians and the sides are related by
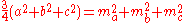
and
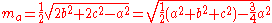,and equivalently for
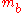and .For angle
opposite side a, the length of the internal bisector is given by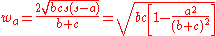for semiperimeter s, where the bisector length is measured from the vertex to where it meets the opposite side.
The following formulas involve the circumradius R and the inradius r :
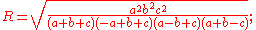
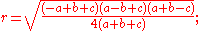
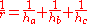
where ha etc. are the altitudes to the subscripted sides;
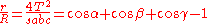;and
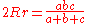.Suppose two adjacent but non-overlapping triangles share the same side of length f and share the same circumcircle, so that the side of length f is a chord of the circumcircle and the triangles have side lengths (a, b, f) and (c, d, f), with the two triangles together forming a cyclic quadrilateral
Cyclic quadrilateral
In Euclidean geometry, a cyclic quadrilateral is a quadrilateral whose vertices all lie on a single circle. This circle is called the circumcircle or circumscribed circle, and the vertices are said to be concyclic. Other names for these quadrilaterals are chordal quadrilateral and inscribed...
with side lengths in sequence (a, b, c, d). Then
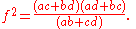
Let M be the centroid of a triangle with vertices A, B, and C, and let P be any interior point. Then the distances between the points are related by
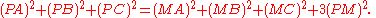
Let pa, pb, and pc be the distances from the centroid to the sides of lengths a, b, and c. Then
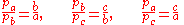
and
The product of two sides of a triangle equals the altitude to the third side times the diameter of the circumcircle.
Carnot's Theorem
Carnot's theorem
In Euclidean geometry, Carnot's theorem, named after Lazare Carnot , is as follows. Let ABC be an arbitrary triangle. Then the sum of the signed distances from the circumcenter D to the sides of triangle ABC is...
states that the sum of the distances from the circumcenter to the three sides equals the sum of the circumradius and the inradius. Here a segment's length is considered to be negative if and only if the segment lies entirely outside the triangle.
Euler's theorem
Euler's theorem in geometry
In geometry, Euler's theorem, named after Leonhard Euler, states that the distance d between the circumcentre and incentre of a triangle can be expressed as d^2=R \,...
states that the distance d between the circumcenter and the incenter is given by.
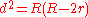
or equivalently
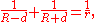
where R is the circumradius and r is the inradius. Thus for all triangles R ≥ 2r, with equality holding for equilateral triangles.
If we denote that the orthocenter divides one altitude into segments of lengths u and v, another altitude into segment lengths w and x, and the third altitude into segment lengths y and z, then uv = wx = yz.
The distance from a side to the circumcenter equals half the distance from the opposite vertex to the orthocenter.
The sum of the squares of the distances from the vertices to the orthocenter plus the sum of the squares of the sides equals twelve times the square of the circumradius.
Morley's trisector theorem
Morley's trisector theorem states that in any triangle, the three points of intersection of the adjacent angle trisectors form an equilateral triangle, called the Morley triangle.Figures inscribed in a triangle
As discussed above, every triangle has a unique inscribed circle (incircle) that is interior to the triangle and tangent to all three sides.Every triangle has a unique Steiner inellipse
Steiner inellipse
In geometry, the Steiner inellipse of a triangle is the unique ellipse inscribed in the triangle and tangent to the sides at their midpoints. It is an example of an inconic. By comparison the inscribed circle of a triangle is another inconic that is tangent to the sides, but not necessarily at the...
which is interior to the triangle and tangent at the midpoints of the sides. Marden's theorem
Marden's theorem
In mathematics, Marden's theorem, named after Morris Marden, gives a geometric relationship between the zeroes of a third-degree polynomial with complex coefficients and the zeroes of its derivative....
shows how to find the foci of this ellipse.
Every triangle has three inscribed squares (squares in its interior such that all four of a square's vertices lie on a side of the triangle, so two of them lie on the same side and hence one side of the square coincides with part of a side of the triangle). However, in the case of a right triangle two of the squares coincide and have a vertex at the triangle's right angle, so a right triangle has only two distinct inscribed squares. Within a given triangle, a longer common side is associated with a smaller inscribed square. If an inscribed square has side of length s and the triangle has a side of length x, part of which side coincides with a side of the square, then s, x, and the triangle's area T are related according to
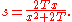
The largest possible ratio of the area of the inscribed square to the area of the triangle is 1/2, which occurs when
, s=x/2, and the altitude of the triangle from base x equals x.Non-planar triangles
A non-planar triangle is a triangle which is not contained in a (flat) plane. Some examples of non-planar triangles in non-Euclidean geometries are spherical triangles in spherical geometrySpherical geometry
Spherical geometry is the geometry of the two-dimensional surface of a sphere. It is an example of a geometry which is not Euclidean. Two practical applications of the principles of spherical geometry are to navigation and astronomy....
and hyperbolic triangle
Hyperbolic triangle
In mathematics, the term hyperbolic triangle has more than one meaning.-Hyperbolic geometry:In hyperbolic geometry, a hyperbolic triangle is a figure in the hyperbolic plane, analogous to a triangle in Euclidean geometry, consisting of three sides and three angles...
s in hyperbolic geometry
Hyperbolic geometry
In mathematics, hyperbolic geometry is a non-Euclidean geometry, meaning that the parallel postulate of Euclidean geometry is replaced...
.
While the measures of the internal angles in planar triangles always sum to 180°, a hyperbolic triangle has measures of angles that sum to less than 180°, and a spherical triangle has measures of angles that sum to more than 180°. A hyperbolic triangle can be obtained by drawing on a negatively curved surface, such as a saddle surface
Saddle surface
A saddle surface is a smooth surface containing one or more saddle points.The term derives from the peculiar shape of historical horse saddles, which curve both up and down....
, and a spherical triangle can be obtained by drawing on a positively curved surface such as a sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
. Thus, if one draws a giant triangle on the surface of the Earth, one will find that the sum of the measures of its angles is greater than 180°; in fact it will be between 180° and 540°. In particular it is possible to draw a triangle on a sphere such that the measure of each of its internal angles is equal to 90°, adding up to a total of 270°.
Specifically, on a sphere the sum of the angles of a triangle is
- 180°×(1+4f ),
where f is the fraction of the sphere's area which is enclosed by the triangle. For example, suppose that we draw a triangle on the Earth's surface with vertices at the North Pole, at a point on the equator at 0° longitude, and a point on the equator at 90° West longitude. The great circle
Great circle
A great circle, also known as a Riemannian circle, of a sphere is the intersection of the sphere and a plane which passes through the center point of the sphere, as opposed to a general circle of a sphere where the plane is not required to pass through the center...
line between the latter two points is the equator, and the great circle line between either of those points and the North Pole is a line of longitude; so there are right angles at the two points on the equator. Moreover, the angle at the North Pole is also 90° because the other two vertices differ by 90° of longitude. So the sum of the angles in this triangle is 90°+90°+90°=270°. The triangle encloses 1/4 of the northern hemisphere (90°/360° as viewed from the North Pole) and therefore 1/8 of the Earth's surface, so in the formula f = 1/8; thus the formula correctly gives the sum of the triangle's angles as 270°.
From the above angle sum formula we can also see that the Earth's surface is locally flat: If we draw an arbitrarily small triangle in the neighborhood of one point on the Earth's surface, the fraction f of the Earth's surface which is enclosed by the triangle will be arbitrarily close to zero. In this case the angle sum formula simplifies to 180°, which we know is what Euclidean geometry tells us for triangles on a flat surface.
Triangles in construction
RectangleRectangle
In Euclidean plane geometry, a rectangle is any quadrilateral with four right angles. The term "oblong" is occasionally used to refer to a non-square rectangle...
s have been the most popular and common geometric form for buildings since the shape is easy to stack and organize; as a standard, it is easy to design furniture and fixtures to fit inside rectangularly-shaped buildings. But triangles, while more difficult to use conceptually, provide a great deal of strength. As computer technology helps architect
Architect
An architect is a person trained in the planning, design and oversight of the construction of buildings. To practice architecture means to offer or render services in connection with the design and construction of a building, or group of buildings and the space within the site surrounding the...
s design creative new buildings, triangular shapes are becoming increasingly prevalent as parts of buildings and as the primary shape for some types of skyscrapers as well as building materials. In Tokyo in 1989, architects had wondered whether it was possible to build a 500 story tower to provide affordable office space for this densely packed city, but with the danger to buildings from earthquake
Earthquake
An earthquake is the result of a sudden release of energy in the Earth's crust that creates seismic waves. The seismicity, seismism or seismic activity of an area refers to the frequency, type and size of earthquakes experienced over a period of time...
s, architects considered that a triangular shape would have been necessary if such a building was ever to have been built (it hasn't by 2011). In New York City, as Broadway
Broadway (New York City)
Broadway is a prominent avenue in New York City, United States, which runs through the full length of the borough of Manhattan and continues northward through the Bronx borough before terminating in Westchester County, New York. It is the oldest north–south main thoroughfare in the city, dating to...
crisscrosses major avenues, the resulting blocks are cut like triangles, and buildings have been built on these shapes; one such building is the triangularly-shaped Flatiron Building
Flatiron Building
The Flatiron Building, or Fuller Building, as it was originally called, is located at 175 Fifth Avenue in the borough of Manhattan, New York City and is considered to be a groundbreaking skyscraper. Upon completion in 1902 it was one of the tallest buildings in the city and the only skyscraper...
which real estate people admit has a "warren of awkward spaces that do not easily accommodate modern office furniture" but that has not prevented the structure from becoming a landmark icon. Designers have made houses in Norway
Norway
Norway , officially the Kingdom of Norway, is a Nordic unitary constitutional monarchy whose territory comprises the western portion of the Scandinavian Peninsula, Jan Mayen, and the Arctic archipelago of Svalbard and Bouvet Island. Norway has a total area of and a population of about 4.9 million...
using triangular themes. Triangle shapes have appeared in churches as well as public buildings including colleges as well as supports for innovative home designs. Triangles are sturdy; while a rectangle can collapse into a parallelogram
Parallelogram
In Euclidean geometry, a parallelogram is a convex quadrilateral with two pairs of parallel sides. The opposite or facing sides of a parallelogram are of equal length and the opposite angles of a parallelogram are of equal measure...
from pressure to one of its points, triangles have a natural strength which supports structures against lateral pressures. A triangle will not change shape unless its sides are bent or extended or broken or if its joints break; in essence, each of the three sides supports the other two. A rectangle, in contrast, is more dependent on the strength of its joints in a structural sense. Some innovative designers have proposed making bricks
Bricks
Bricks may refer to:* Brick, an artificial stone made by forming clay into rectangular blocks* Brick , a slang term for a device that cannot function due to internal failure...
not out of rectangles, but with triangular shapes which can be combined in three dimensions. It is likely that triangles will be used increasingly in new ways as architecture increases in complexity.
External links
- Clark Kimberling: Encyclopedia of triangle centers. Lists some 3200 interesting points associated with any triangle.