Tetrakis square tiling
Encyclopedia
In geometry
, the tetrakis square tiling is a tiling of the Euclidean plane. It is square tiling with each square divided into four triangles from the center point, forming an infinite arrangement of lines
.
Conway
calls it a kisquadrille, represented by a kis operation that adds a center point and triangles to replace the faces of a square tiling (quadrille). It is also called the Union Jack lattice because of the similitude of its unit cell to the UK flag.
It is labeled V4.8.8 because each isosceles triangle face has two types of vertices: one with 4 triangles, and two with 8 triangles.
which has one square and two octagons at each vertex.
, V4.6.6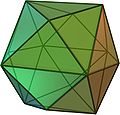
The symmetry type is:
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the tetrakis square tiling is a tiling of the Euclidean plane. It is square tiling with each square divided into four triangles from the center point, forming an infinite arrangement of lines
Arrangement of lines
In geometry an arrangement of lines is the partition of the plane formed by a collection of lines. Bounds on the complexity of arrangements have been studied in discrete geometry, and computational geometers have found algorithms for the efficient construction of arrangements.-Definition:For any...
.
Conway
John Horton Conway
John Horton Conway is a prolific mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory...
calls it a kisquadrille, represented by a kis operation that adds a center point and triangles to replace the faces of a square tiling (quadrille). It is also called the Union Jack lattice because of the similitude of its unit cell to the UK flag.
It is labeled V4.8.8 because each isosceles triangle face has two types of vertices: one with 4 triangles, and two with 8 triangles.
Dual tiling
It is the dual tessellation of the truncated square tilingTruncated square tiling
In geometry, the truncated square tiling is a semiregular tiling of the Euclidean plane. There is one square and two octagons on each vertex. This is the only edge-to-edge tiling by regular convex polygons which contains an octagon...
which has one square and two octagons at each vertex.
Related polyhedra and tilings
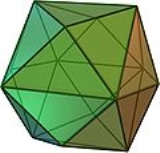
It is topologically related to the polyhedron tetrakis hexahedronTetrakis hexahedron
In geometry, a tetrakis hexahedron is a Catalan solid. Its dual is the truncated octahedron, an Archimedean solid. It can be seen as a cube with square pyramids covering each square face; that is, it is the Kleetope of the cube....
, V4.6.6

The symmetry type is:
- with the coloring: cmm; a primitive cellPrimitive cellUsed predominantly in geometry, solid state physics, and mineralogy, particularly in describing crystal structure, a primitive cell is a minimum cell corresponding to a single lattice point of a structure with translational symmetry in 2 dimensions, 3 dimensions, or other dimensions...
is 8 triangles, a fundamental domainFundamental domainIn geometry, the fundamental domain of a symmetry group of an object is a part or pattern, as small or irredundant as possible, which determines the whole object based on the symmetry. More rigorously, given a topological space and a group acting on it, the images of a single point under the group...
2 triangles (1/2 for each color) - with the dark triangles in black and the light ones in white: p4g; a primitive cell is 8 triangles, a fundamental domain 1 triangle (1/2 each for black and white)
- with the edges in black and the interiors in white: p4m; a primitive cell is 2 triangles, a fundamental domain 1/2

