24-cell
Encyclopedia
In geometry
, the 24-cell (or icositetrachoron) is the convex regular 4-polytope
, or polychoron
, with Schläfli symbol {3,4,3}. It is also called an octaplex (short for "octahedral complex"), octacube
, or polyoctahedron, being constructed of octahedral
cells
.
The boundary of the 24-cell is composed of 24 octahedral
cells with six meeting at each vertex, and three at each edge. Together they have 96 triangular faces, 96 edges, and 24 vertices. The vertex figure
is a cube
. The 24-cell is self-dual. In fact, the 24-cell is the unique self-dual regular Euclidean polytope which is neither a polygon
nor a simplex
. Due to this singular property, it does not have a good analogue in 3 dimensions, but in 2 dimensions the hexagon, along with all regular polygons, are self-dual.
of its vertices. The vertices of a 24-cell centered at the origin of 4-space, with edges of length 1, can be given as follows: 8 vertices obtained by permuting
and 16 vertices of the form.
The first 8 vertices are the vertices of a regular 16-cell
and the other 16 are the vertices of the dual tesseract
. This gives a construction equivalent to cutting a tesseract into 8 cubical pyramids, and then attaching them to the facets of a second tesseract. The analogous construction in 3-space gives the rhombic dodecahedron
which, however, is not regular.
We can further divide the last 16 vertices into two groups: those with an even number of minus (−) signs and those with an odd number. Each of groups of 8 vertices also define a regular 16-cell. The vertices of the 24-cell can then be grouped into three sets of eight with each set defining a regular 16-cell, and with the complement defining the dual tesseract.
The vertices of the dual 24-cell are given by all permutations of.
The dual 24-cell has edges of length √2 and is inscribed in a 3-sphere
of radius √2.
Another method of constructing the 24-cell is by the rectification
of the 16-cell. The vertex figure of the 16-cell is the octahedron; thus, cutting the vertices of the 16-cell at the midpoint of its incident edges produce 8 octahedral cells. This process also rectifies the tetrahedral cells of the 16-cell which also become octahedra, thus forming the 24 octahedral cells of the 24-cell.
exists with 24-cells, called an icositetrachoric honeycomb, with Schläfli symbol {3,4,3,3}. The regular dual tessellation, {3,3,4,3} has 16-cell
s. (See also List of regular polytopes which includes a third regular tessellation, the tesseractic honeycomb {4,3,3,4}.)
D4. When combined with the 8 vertices of the 16-cell
these vertices represent the 32 root vectors of the simple Lie group B4.
The 48 vertices (or strictly speaking their radius vectors) of the 24-cell and its dual form the root system
of type F4. The 24 vertices of the original 24-cell as well as the 24 vertices of the dual form root systems of type D4; their sizes have the ratio sqrt(2):1. The full symmetry group
of the 24-cell is the Weyl group
of F4 which is generated by reflections
through the hyperplanes orthogonal to the F4 roots. This is a solvable group
of order 1152. The rotational symmetry group of the 24-cell is of order 576.
When interpreted as the quaternion
s, the F4 root lattice (which is integral span of the vertices of the 24-cell) is closed under multiplication and is therefore forms a ring
. This is the ring of Hurwitz integral quaternions. The vertices of the 24-cell form the group of units (i.e. the group of invertible elements) in the Hurwitz quaternion ring (this group is also known as the binary tetrahedral group). The vertices of the 24-cell are precisely the 24 Hurwitz quaternions with norm squared 1, and the vertices of the dual 24-cell are those with norm squared 2. The D4 root lattice is the dual of the F4 and is given by the subring of Hurwitz quaternions with even norm squared.
The Voronoi cells of the D4 root lattice are regular 24-cells. The corresponding Voronoi tessellation gives a tessellation
of 4-dimensional Euclidean space
by regular 24-cells. The 24-cells are centered at the D4 lattice points (Hurwitz quaternions with even norm squared) while the vertices are at the F4 lattice points with odd norm squared. Each 24-cell has 24 neighbors with which it shares an octahedron and 32 neighbors with which it shares only a single point. Eight 24-cells meet at any given vertex in this tessellation. The Schläfli symbol for this tessellation is {3,4,3,3}. The dual tessellation, {3,3,4,3}, is one by regular 16-cell
s. Together with the regular tesseract
tessellation, {4,3,3,4}, these are the only regular tessellations of R4.
It is interesting to note that the unit balls
inscribed in the 24-cells of the above tessellation give rise to the densest lattice packing of hypersphere
s in 4 dimensions. The vertex configuration of the 24-cell has also been shown to give the highest possible kissing number in 4 dimensions.
s, the points of an appropriately scaled and orientated 24-cell form a multiplicative group
. This property applies to two other regular polychora, namely the 16-cell
and 600-cell, with 8 and 120 points, respectively. The group of 120 points is known as the icosian group.
 The vertex-first parallel projection of the 24-cell into 3-dimensional space has a rhombic dodecahedral
The vertex-first parallel projection of the 24-cell into 3-dimensional space has a rhombic dodecahedral
envelope. Twelve of the 24 octahedral cells project in pairs onto six square dipyramids that meet at the center of the rhombic dodecahedron. The remaining 12 octahedral cells project onto the 12 rhombic faces of the rhombic dodecahedron.
The cell-first parallel projection of the 24-cell into 3-dimensional space has a cuboctahedral
envelope. Two of the octahedral cells, the nearest and farther from the viewer along the W-axis, project onto an octahedron whose vertices lie at the center of the cuboctahedron's square faces. Surrounding this central octahedron lie the projections of 16 other cells, having 8 pairs that each project to one of the 8 volumes lying between a triangular face of the central octahedron and the closest triangular face of the cuboctahedron. The remaining 6 cells project onto the square faces of the cuboctahedron. This corresponds with the decomposition of the cuboctahedron into a regular octahedron and 8 irregular but equal octahedra, each of which is in the shape of the convex hull of a cube with two opposite vertices removed.
The edge-first parallel projection has an elongated hexagonal dipyramid
al envelope, and the face-first parallel projection has a nonuniform hexagonal bi-antiprismic
envelope.
The vertex-first perspective projection of the 24-cell into 3-dimensional space has a tetrakis hexahedral
envelope. The layout of cells in this image is similar to the image under parallel projection.
The following sequence of images shows the structure of the cell-first perspective projection of the 24-cell into 3 dimensions. The 4D viewpoint is placed at a distance of five times the vertex-center radius of the 24-cell.
16-cell, with B4 or [3,3,4] symmetry drawn bicolored with 8 and 16 octahedral
cells. Lastly it can be constructed from D4 or [31,1,1] symmetry, and drawn tricolored with 8 octahedra each.
cells
. For visualization purposes, it is convenient that the octahedron has opposing parallel faces
(a trait it shares with the cells of the tesseract
and the 120-cell). One can stack octahedrons face to face in a straight line bent in the 4th direction into a great circle
with a circumference
of 6 cells. The cell locations lend themselves to a hyperspherical
description. Pick an arbitrary cell and label it the "North Pole
". Eight great circle meridians (two cells long) radiate out in 3 dimensions, converging at the 3rd "South Pole
" cell. This skeleton accounts for 18 of the 24 cells (2 + 8*2). See the table below.
There is another related great circle in the 24-cell, the dual of the one above. A path that traverses 6 vertices solely along edges, resides in the dual of this polytope, which is itself since it is self dual. One can easily follow this path in a rendering of the equatorial cuboctahedron
cross-section. One can also follow a great circle route, through the octahedrons' opposing vertices, that is four cells long. This corresponds to traversing diagonally through the squares in the cuboctahedron cross-section. The 24-cell is the only regular polytope in more than two dimensions where you can traverse a great circle purely through opposing vertices (and the interior) of each cell. This great circle is self dual.
Starting at the North Pole, we can build up the 24-cell in 5 latitudinal layers. With the exception of the poles, each layer represents a separate 2-sphere, with the equator being a great 2-sphere. The cells labeled equatorial in the following table are interstitial to the meridian great circle cells. The interstitial "equatorial" cells touch the meridian cells at their faces. They touch each other, and the pole cells at their vertices. This latter subset of eight non-meridian and pole cells has the same relative position to each other as the cells in a tesseract (8-cell), although they touch at their vertices instead of their faces.
The 24-cell can be partitioned into disjoint sets of four of these 6-cell great circle rings, forming a discrete Hopf fibration of four interlocking rings. One ring is "vertical", encompassing the pole cells and four meridian cells. The other three rings each encompass two equatorial cells and four meridian cells, two from the northern hemisphere and two from the southern.
can be derived from the 24-cell via truncation
:
The 96 edges of the 24-cell can be partitioned into the golden ratio
to produce the 96 vertices of the snub 24-cell
. This is done by first placing vectors along the 24-cell's edges such that each two-dimensional face is bounded by a cycle, then similarly partitioning each edge into the golden ratio along the direction of its vector. An analogous modification to an octahedron
produces an icosahedron
, or "snub octahedron."
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the 24-cell (or icositetrachoron) is the convex regular 4-polytope
Convex regular 4-polytope
In mathematics, a convex regular 4-polytope is a 4-dimensional polytope that is both regular and convex. These are the four-dimensional analogs of the Platonic solids and the regular polygons ....
, or polychoron
Polychoron
In geometry, a polychoron or 4-polytope is a four-dimensional polytope. It is a connected and closed figure, composed of lower dimensional polytopal elements: vertices, edges, faces , and cells...
, with Schläfli symbol {3,4,3}. It is also called an octaplex (short for "octahedral complex"), octacube
Octacube (mathematics)
The octacube is a large 3-dimensional mathematical sculpture illustrating a 4-dimensional structure in the Galois theory of operator algebras....
, or polyoctahedron, being constructed of octahedral
Octahedron
In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
cells
Cell (geometry)
In geometry, a cell is a three-dimensional element that is part of a higher-dimensional object.- In polytopes :A cell is a three-dimensional polyhedron element that is part of the boundary of a higher-dimensional polytope, such as a polychoron or honeycomb For example, a cubic honeycomb is made...
.
The boundary of the 24-cell is composed of 24 octahedral
Octahedron
In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
cells with six meeting at each vertex, and three at each edge. Together they have 96 triangular faces, 96 edges, and 24 vertices. The vertex figure
Vertex figure
In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:...
is a cube
Cube
In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. The cube can also be called a regular hexahedron and is one of the five Platonic solids. It is a special kind of square prism, of rectangular parallelepiped and...
. The 24-cell is self-dual. In fact, the 24-cell is the unique self-dual regular Euclidean polytope which is neither a polygon
Polygon
In geometry a polygon is a flat shape consisting of straight lines that are joined to form a closed chain orcircuit.A polygon is traditionally a plane figure that is bounded by a closed path, composed of a finite sequence of straight line segments...
nor a simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
. Due to this singular property, it does not have a good analogue in 3 dimensions, but in 2 dimensions the hexagon, along with all regular polygons, are self-dual.
Constructions
A 24-cell is given as the convex hullConvex hull
In mathematics, the convex hull or convex envelope for a set of points X in a real vector space V is the minimal convex set containing X....
of its vertices. The vertices of a 24-cell centered at the origin of 4-space, with edges of length 1, can be given as follows: 8 vertices obtained by permuting
and 16 vertices of the form.
The first 8 vertices are the vertices of a regular 16-cell
16-cell
In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century....
and the other 16 are the vertices of the dual tesseract
Tesseract
In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...
. This gives a construction equivalent to cutting a tesseract into 8 cubical pyramids, and then attaching them to the facets of a second tesseract. The analogous construction in 3-space gives the rhombic dodecahedron
Rhombic dodecahedron
In geometry, the rhombic dodecahedron is a convex polyhedron with 12 rhombic faces. It is an Archimedean dual solid, or a Catalan solid. Its dual is the cuboctahedron.-Properties:...
which, however, is not regular.
We can further divide the last 16 vertices into two groups: those with an even number of minus (−) signs and those with an odd number. Each of groups of 8 vertices also define a regular 16-cell. The vertices of the 24-cell can then be grouped into three sets of eight with each set defining a regular 16-cell, and with the complement defining the dual tesseract.
The vertices of the dual 24-cell are given by all permutations of.
The dual 24-cell has edges of length √2 and is inscribed in a 3-sphere
3-sphere
In mathematics, a 3-sphere is a higher-dimensional analogue of a sphere. It consists of the set of points equidistant from a fixed central point in 4-dimensional Euclidean space...
of radius √2.
Another method of constructing the 24-cell is by the rectification
Rectification (geometry)
In Euclidean geometry, rectification is the process of truncating a polytope by marking the midpoints of all its edges, and cutting off its vertices at those points...
of the 16-cell. The vertex figure of the 16-cell is the octahedron; thus, cutting the vertices of the 16-cell at the midpoint of its incident edges produce 8 octahedral cells. This process also rectifies the tetrahedral cells of the 16-cell which also become octahedra, thus forming the 24 octahedral cells of the 24-cell.
Tessellations
A regular tessellation of 4-dimensional Euclidean spaceEuclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
exists with 24-cells, called an icositetrachoric honeycomb, with Schläfli symbol {3,4,3,3}. The regular dual tessellation, {3,3,4,3} has 16-cell
16-cell
In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century....
s. (See also List of regular polytopes which includes a third regular tessellation, the tesseractic honeycomb {4,3,3,4}.)
Symmetries, root systems, and tessellations
The 24 vertices of the 24-cell represent the root vectors of the simple Lie groupSimple Lie group
In group theory, a simple Lie group is a connected non-abelian Lie group G which does not have nontrivial connected normal subgroups.A simple Lie algebra is a non-abelian Lie algebra whose only ideals are 0 and itself...
D4. When combined with the 8 vertices of the 16-cell
16-cell
In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century....
these vertices represent the 32 root vectors of the simple Lie group B4.
The 48 vertices (or strictly speaking their radius vectors) of the 24-cell and its dual form the root system
Root system
In mathematics, a root system is a configuration of vectors in a Euclidean space satisfying certain geometrical properties. The concept is fundamental in the theory of Lie groups and Lie algebras...
of type F4. The 24 vertices of the original 24-cell as well as the 24 vertices of the dual form root systems of type D4; their sizes have the ratio sqrt(2):1. The full symmetry group
Symmetry group
The symmetry group of an object is the group of all isometries under which it is invariant with composition as the operation...
of the 24-cell is the Weyl group
Weyl group
In mathematics, in particular the theory of Lie algebras, the Weyl group of a root system Φ is a subgroup of the isometry group of the root system. Specifically, it is the subgroup which is generated by reflections through the hyperplanes orthogonal to the roots, and as such is a finite reflection...
of F4 which is generated by reflections
Reflection (mathematics)
In mathematics, a reflection is a mapping from a Euclidean space to itself that is an isometry with a hyperplane as set of fixed points; this set is called the axis or plane of reflection. The image of a figure by a reflection is its mirror image in the axis or plane of reflection...
through the hyperplanes orthogonal to the F4 roots. This is a solvable group
Solvable group
In mathematics, more specifically in the field of group theory, a solvable group is a group that can be constructed from abelian groups using extensions...
of order 1152. The rotational symmetry group of the 24-cell is of order 576.
When interpreted as the quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s, the F4 root lattice (which is integral span of the vertices of the 24-cell) is closed under multiplication and is therefore forms a ring
Ring (mathematics)
In mathematics, a ring is an algebraic structure consisting of a set together with two binary operations usually called addition and multiplication, where the set is an abelian group under addition and a semigroup under multiplication such that multiplication distributes over addition...
. This is the ring of Hurwitz integral quaternions. The vertices of the 24-cell form the group of units (i.e. the group of invertible elements) in the Hurwitz quaternion ring (this group is also known as the binary tetrahedral group). The vertices of the 24-cell are precisely the 24 Hurwitz quaternions with norm squared 1, and the vertices of the dual 24-cell are those with norm squared 2. The D4 root lattice is the dual of the F4 and is given by the subring of Hurwitz quaternions with even norm squared.
The Voronoi cells of the D4 root lattice are regular 24-cells. The corresponding Voronoi tessellation gives a tessellation
Tessellation
A tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
of 4-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
by regular 24-cells. The 24-cells are centered at the D4 lattice points (Hurwitz quaternions with even norm squared) while the vertices are at the F4 lattice points with odd norm squared. Each 24-cell has 24 neighbors with which it shares an octahedron and 32 neighbors with which it shares only a single point. Eight 24-cells meet at any given vertex in this tessellation. The Schläfli symbol for this tessellation is {3,4,3,3}. The dual tessellation, {3,3,4,3}, is one by regular 16-cell
16-cell
In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century....
s. Together with the regular tesseract
Tesseract
In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...
tessellation, {4,3,3,4}, these are the only regular tessellations of R4.
It is interesting to note that the unit balls
Ball (mathematics)
In mathematics, a ball is the space inside a sphere. It may be a closed ball or an open ball ....
inscribed in the 24-cells of the above tessellation give rise to the densest lattice packing of hypersphere
Hypersphere
In mathematics, an n-sphere is a generalization of the surface of an ordinary sphere to arbitrary dimension. For any natural number n, an n-sphere of radius r is defined as the set of points in -dimensional Euclidean space which are at distance r from a central point, where the radius r may be any...
s in 4 dimensions. The vertex configuration of the 24-cell has also been shown to give the highest possible kissing number in 4 dimensions.
Quaternions
Translating the four-dimensional coordinates into quaternionQuaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s, the points of an appropriately scaled and orientated 24-cell form a multiplicative group
Multiplicative group
In mathematics and group theory the term multiplicative group refers to one of the following concepts, depending on the context*any group \scriptstyle\mathfrak \,\! whose binary operation is written in multiplicative notation ,*the underlying group under multiplication of the invertible elements of...
. This property applies to two other regular polychora, namely the 16-cell
16-cell
In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century....
and 600-cell, with 8 and 120 points, respectively. The group of 120 points is known as the icosian group.
Projections

Rhombic dodecahedron
In geometry, the rhombic dodecahedron is a convex polyhedron with 12 rhombic faces. It is an Archimedean dual solid, or a Catalan solid. Its dual is the cuboctahedron.-Properties:...
envelope. Twelve of the 24 octahedral cells project in pairs onto six square dipyramids that meet at the center of the rhombic dodecahedron. The remaining 12 octahedral cells project onto the 12 rhombic faces of the rhombic dodecahedron.
The cell-first parallel projection of the 24-cell into 3-dimensional space has a cuboctahedral
Cuboctahedron
In geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron,...
envelope. Two of the octahedral cells, the nearest and farther from the viewer along the W-axis, project onto an octahedron whose vertices lie at the center of the cuboctahedron's square faces. Surrounding this central octahedron lie the projections of 16 other cells, having 8 pairs that each project to one of the 8 volumes lying between a triangular face of the central octahedron and the closest triangular face of the cuboctahedron. The remaining 6 cells project onto the square faces of the cuboctahedron. This corresponds with the decomposition of the cuboctahedron into a regular octahedron and 8 irregular but equal octahedra, each of which is in the shape of the convex hull of a cube with two opposite vertices removed.
The edge-first parallel projection has an elongated hexagonal dipyramid
Elongated hexagonal dipyramid
In geometry, the elongated hexagonal bipyramid is constructed by elongating a hexagonal bipyramid .- Related polyhedra:...
al envelope, and the face-first parallel projection has a nonuniform hexagonal bi-antiprismic
Hexagonal antiprism
In geometry, the hexagonal antiprism is the 4th in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps.If faces are all regular, it is a semiregular polyhedron.- See also :* Set of antiprisms...
envelope.
The vertex-first perspective projection of the 24-cell into 3-dimensional space has a tetrakis hexahedral
Tetrakis hexahedron
In geometry, a tetrakis hexahedron is a Catalan solid. Its dual is the truncated octahedron, an Archimedean solid. It can be seen as a cube with square pyramids covering each square face; that is, it is the Kleetope of the cube....
envelope. The layout of cells in this image is similar to the image under parallel projection.
The following sequence of images shows the structure of the cell-first perspective projection of the 24-cell into 3 dimensions. The 4D viewpoint is placed at a distance of five times the vertex-center radius of the 24-cell.
| Cell-first perspective projection | ||
|---|---|---|
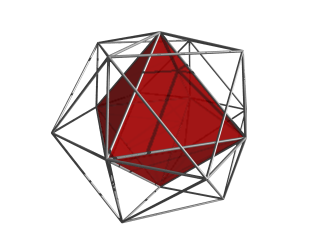 In this image, the nearest cell is rendered in red, and the remaining cells are in edge-outline. For clarity, cells facing away from the 4D viewpoint have been culled. |
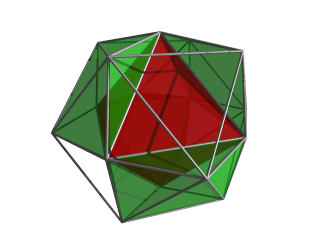 In this image, four of the 8 cells surrounding the nearest cell are shown in green. The fourth cell is behind the central cell in this viewpoint (slightly discernible since the red cell is semi-transparent). |
 Finally, all 8 cells surrounding the nearest cell are shown, with the last four rendered in magenta. Note that these images do not include cells which are facing away from the 4D viewpoint. Hence, only 9 cells are shown here. On the far side of the 24-cell are another 9 cells in an identical arrangement. The remaining 6 cells lie on the "equator" of the 24-cell, and bridge the two sets of cells. |
 Stereographic projection Stereographic projection The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it... |
||
 Animated cross-section of 24-cell |
 A 3D projection of a 24-cell performing a simple rotation. |
|
 A stereoscopic Stereogram A stereogram is pair of two-dimensional panels depicting the view of a scene or an object from the vantage points of the right and left eyes. Observing the panels superimposed in a stereoscope results in the experience of three-dimensionality by virtue of the fact that object depth is encoded as... 3D projection of an icositetrachoron (24-cell). |
||
| Isometric Orthogonal Projection of: 8 Cell(Tesseract) + 16 Cell = 24 Cell |
||
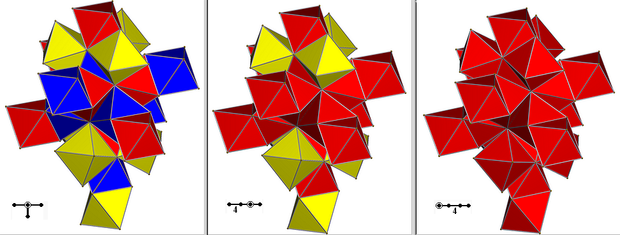
Three Coxeter group constructions
There are two lower symmetry forms of the 24-cell, derived as a rectifiedRectification (geometry)
In Euclidean geometry, rectification is the process of truncating a polytope by marking the midpoints of all its edges, and cutting off its vertices at those points...
16-cell, with B4 or [3,3,4] symmetry drawn bicolored with 8 and 16 octahedral
Octahedron
In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
cells. Lastly it can be constructed from D4 or [31,1,1] symmetry, and drawn tricolored with 8 octahedra each.
| Rectified demitesseract | Rectified 16-cell | Regular 24-cell |
|---|---|---|
 |
||
| D4: Three sets of 8 rectified tetrahedral cells | B4:One set of 16 rectified tetrahedral cells and one set of 8 octahedral Octahedron In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.... cells. |
F4: One set of 24 octahedral Octahedron In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.... cells |
| Vertex figure Vertex figure In geometry a vertex figure is, broadly speaking, the figure exposed when a corner of a polyhedron or polytope is sliced off.-Definitions - theme and variations:... (Each edge corresponds to one triangular face, colored by symmetry arrangement) |
||
 |
 |
 |
Visualization
The 24-cell consists of 24 octahedralOctahedron
In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
cells
Cell (geometry)
In geometry, a cell is a three-dimensional element that is part of a higher-dimensional object.- In polytopes :A cell is a three-dimensional polyhedron element that is part of the boundary of a higher-dimensional polytope, such as a polychoron or honeycomb For example, a cubic honeycomb is made...
. For visualization purposes, it is convenient that the octahedron has opposing parallel faces
Face (geometry)
In geometry, a face of a polyhedron is any of the polygons that make up its boundaries. For example, any of the squares that bound a cube is a face of the cube...
(a trait it shares with the cells of the tesseract
Tesseract
In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8...
and the 120-cell). One can stack octahedrons face to face in a straight line bent in the 4th direction into a great circle
Great circle
A great circle, also known as a Riemannian circle, of a sphere is the intersection of the sphere and a plane which passes through the center point of the sphere, as opposed to a general circle of a sphere where the plane is not required to pass through the center...
with a circumference
Circumference
The circumference is the distance around a closed curve. Circumference is a special perimeter.-Circumference of a circle:The circumference of a circle is the length around it....
of 6 cells. The cell locations lend themselves to a hyperspherical
3-sphere
In mathematics, a 3-sphere is a higher-dimensional analogue of a sphere. It consists of the set of points equidistant from a fixed central point in 4-dimensional Euclidean space...
description. Pick an arbitrary cell and label it the "North Pole
North Pole
The North Pole, also known as the Geographic North Pole or Terrestrial North Pole, is, subject to the caveats explained below, defined as the point in the northern hemisphere where the Earth's axis of rotation meets its surface...
". Eight great circle meridians (two cells long) radiate out in 3 dimensions, converging at the 3rd "South Pole
South Pole
The South Pole, also known as the Geographic South Pole or Terrestrial South Pole, is one of the two points where the Earth's axis of rotation intersects its surface. It is the southernmost point on the surface of the Earth and lies on the opposite side of the Earth from the North Pole...
" cell. This skeleton accounts for 18 of the 24 cells (2 + 8*2). See the table below.
There is another related great circle in the 24-cell, the dual of the one above. A path that traverses 6 vertices solely along edges, resides in the dual of this polytope, which is itself since it is self dual. One can easily follow this path in a rendering of the equatorial cuboctahedron
Cuboctahedron
In geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron,...
cross-section. One can also follow a great circle route, through the octahedrons' opposing vertices, that is four cells long. This corresponds to traversing diagonally through the squares in the cuboctahedron cross-section. The 24-cell is the only regular polytope in more than two dimensions where you can traverse a great circle purely through opposing vertices (and the interior) of each cell. This great circle is self dual.
Starting at the North Pole, we can build up the 24-cell in 5 latitudinal layers. With the exception of the poles, each layer represents a separate 2-sphere, with the equator being a great 2-sphere. The cells labeled equatorial in the following table are interstitial to the meridian great circle cells. The interstitial "equatorial" cells touch the meridian cells at their faces. They touch each other, and the pole cells at their vertices. This latter subset of eight non-meridian and pole cells has the same relative position to each other as the cells in a tesseract (8-cell), although they touch at their vertices instead of their faces.
| Layer # | Number of Cells | Description | Colatitude | Region |
|---|---|---|---|---|
| 1 | 1 cell | North Pole | 0° | Northern Hemisphere |
| 2 | 8 cells | First layer of meridian cells | 60° | |
| 3 | 6 cells | Non-meridian / interstitial | 90° | Equator |
| 4 | 8 cells | Second layer of meridian cells | 120° | Southern Hemisphere |
| 5 | 1 cell | South Pole | 180° | |
| Total | 24 cells | |||
The 24-cell can be partitioned into disjoint sets of four of these 6-cell great circle rings, forming a discrete Hopf fibration of four interlocking rings. One ring is "vertical", encompassing the pole cells and four meridian cells. The other three rings each encompass two equatorial cells and four meridian cells, two from the northern hemisphere and two from the southern.
Related 4-polytopes
Several uniform polychoraUniform polychoron
In geometry, a uniform polychoron is a polychoron or 4-polytope which is vertex-transitive and whose cells are uniform polyhedra....
can be derived from the 24-cell via truncation
Truncation (geometry)
In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex.- Uniform truncation :...
:
- truncating at 1/3 of the edge length yields the truncated 24-cellTruncated 24-cellIn geometry, a truncated 5-cell is a uniform polychoron formed as the truncation of the regular 5-cell.There are two degrees of trunctions, including a bitruncation.- Truncated 5-cell:...
; - truncating at 1/2 of the edge length yields the rectified 24-cellRectified 24-cellIn geometry, the rectified 24-cell is a uniform 4-dimensional polytope , which is bounded by 48 cells: 24 cubes, and 24 cuboctahedra. It can be obtained by reducing the icositetrachoron's cells to cubes or cuboctahedra....
; - and truncating at half the depth to the dual 24-cell yields the bitruncated 24-cell, which is cell-transitive.
The 96 edges of the 24-cell can be partitioned into the golden ratio
Golden ratio
In mathematics and the arts, two quantities are in the golden ratio if the ratio of the sum of the quantities to the larger quantity is equal to the ratio of the larger quantity to the smaller one. The golden ratio is an irrational mathematical constant, approximately 1.61803398874989...
to produce the 96 vertices of the snub 24-cell
Snub 24-cell
In geometry, the snub 24-cell is a convex uniform polychoron composed of 120 regular tetrahedral and 24 icosahedral cells. Five tetrahedra and three icosahedra meet at each vertex. In total it has 480 triangular faces, 432 edges, and 96 vertices....
. This is done by first placing vectors along the 24-cell's edges such that each two-dimensional face is bounded by a cycle, then similarly partitioning each edge into the golden ratio along the direction of its vector. An analogous modification to an octahedron
Octahedron
In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
produces an icosahedron
Icosahedron
In geometry, an icosahedron is a regular polyhedron with 20 identical equilateral triangular faces, 30 edges and 12 vertices. It is one of the five Platonic solids....
, or "snub octahedron."
Related uniform polytopes
The 24-cell can also be derived as a rectified 16-cell:See also
- The 24-cell family of uniform polychora

