
3-sphere
Encyclopedia

Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a 3-sphere is a higher-dimensional analogue of a sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
. It consists of the set of points equidistant from a fixed central point in 4-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
. Just as an ordinary sphere (or 2-sphere) is a two-dimensional surface
Surface
In mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
that forms the boundary of a ball
Ball (mathematics)
In mathematics, a ball is the space inside a sphere. It may be a closed ball or an open ball ....
in three dimensions, a 3-sphere is an object with three dimension
Dimension
In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...
s that forms the boundary of a ball in four dimensions. A 3-sphere is an example of a 3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
.
Definition
In coordinates, a 3-sphere with center (C0, C1, C2, C3) and radius r is the set of all points (x0, x1, x2, x3) in real, 4-dimensional space (R4) such that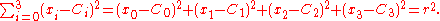
The 3-sphere centered at the origin with radius 1 is called the unit 3-sphere and is usually denoted S3:
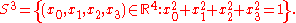
It is often convenient to regard R4 as the space with 2 complex dimensions (C2) or the quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s (H). The unit 3-sphere is then given by
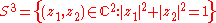
or

This description as the quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s of norm one, identifies the 3-sphere with the versors in the quaternion division ring
Division ring
In abstract algebra, a division ring, also called a skew field, is a ring in which division is possible. Specifically, it is a non-trivial ring in which every non-zero element a has a multiplicative inverse, i.e., an element x with...
. Just as the unit circle
Unit circle
In mathematics, a unit circle is a circle with a radius of one. Frequently, especially in trigonometry, "the" unit circle is the circle of radius one centered at the origin in the Cartesian coordinate system in the Euclidean plane...
is important for planar polar coordinates, so the 3-sphere is important in the polar view of 4-space involved in quaternion multiplication. See polar decomposition of a quaternion for details of this development of the three-sphere.
This view of the 3-sphere is the basis for the study of elliptic space as developed by Georges Lemaître
Georges Lemaître
Monsignor Georges Henri Joseph Édouard Lemaître was a Belgian priest, astronomer and professor of physics at the Catholic University of Louvain. He was the first person to propose the theory of the expansion of the Universe, widely misattributed to Edwin Hubble...
Elementary properties
The 3-dimensional volume (or hyperarea) of a 3-sphere of radius r is
while the 4-dimensional hypervolume (the volume of the 4-dimensional region bounded by the 3-sphere) is
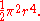
Every non-empty intersection of a 3-sphere with a three-dimensional hyperplane
Hyperplane
A hyperplane is a concept in geometry. It is a generalization of the plane into a different number of dimensions.A hyperplane of an n-dimensional space is a flat subset with dimension n − 1...
is a 2-sphere (unless the hyperplane is tangent to the 3-sphere, in which case the intersection is a single point). As a 3-sphere moves through a given three-dimensional hyperplane, the intersection starts out as a point, then becomes a growing 2-sphere that reaches its maximal size when the hyperplane cuts right through the "equator" of the 3-sphere. Then the 2-sphere shrinks again down to a single point as the 3-sphere leaves the hyperplane.
Topological properties
A 3-sphere is a compactCompact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, connected
Connected space
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint nonempty open subsets. Connectedness is one of the principal topological properties that is used to distinguish topological spaces...
, 3-dimensional manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
without boundary. It is also simply-connected. What this means, in the broad sense, is that any loop, or circular path, on the 3-sphere can be continuously shrunk to a point without leaving the 3-sphere. The Poincaré conjecture
Poincaré conjecture
In mathematics, the Poincaré conjecture is a theorem about the characterization of the three-dimensional sphere , which is the hypersphere that bounds the unit ball in four-dimensional space...
, proved in 2003 by Grigori Perelman
Grigori Perelman
Grigori Yakovlevich Perelman is a Russian mathematician who has made landmark contributions to Riemannian geometry and geometric topology.In 1992, Perelman proved the soul conjecture. In 2002, he proved Thurston's geometrization conjecture...
, provides that the 3-sphere is the only three-dimensional manifold (up to homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
) with these properties.
The 3-sphere is homeomorphic to the one-point compactification of
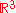 . In general, any topological space
. In general, any topological spaceTopological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
that is homeomorphic to the 3-sphere is called a topological 3-sphere.
The homology groups of the 3-sphere are as follows: H0(S3,Z) and H3(S3,Z) are both infinite cyclic, while Hi(S3,Z) = {0} for all other indices i. Any topological space with these homology groups is known as a homology 3-sphere
Homology sphere
In algebraic topology, a homology sphere is an n-manifold X having the homology groups of an n-sphere, for some integer n ≥ 1. That is,andTherefore X is a connected space, with one non-zero higher Betti number: bn...
. Initially Poincaré
Henri Poincaré
Jules Henri Poincaré was a French mathematician, theoretical physicist, engineer, and a philosopher of science...
conjectured that all homology 3-spheres are homeomorphic to S3, but then he himself constructed a non-homeomorphic one, now known as the Poincaré homology sphere. Infinitely-many homology spheres are now known to exist. For example, a Dehn filling with slope 1/n on any knot
Knot theory
In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...
in the three-sphere gives a homology sphere; typically these are not homeomorphic to the three-sphere.
As to the homotopy groups, we have π1(S3) = π2(S3) = {0} and π3(S3) is infinite cyclic. The higher-homotopy groups (k ≥ 4) are all finite abelian but otherwise follow no discernible pattern. For more discussion see homotopy groups of spheres
Homotopy groups of spheres
In the mathematical field of algebraic topology, the homotopy groups of spheres describe how spheres of various dimensions can wrap around each other. They are examples of topological invariants, which reflect, in algebraic terms, the structure of spheres viewed as topological spaces, forgetting...
.
| k | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 |
| πk(S3) | 0 | 0 | 0 | Z | Z2 | Z2 | Z12 | Z2 | Z2 | Z3 | Z15 | Z2 | Z2⊕Z2 | Z12⊕Z2 | Z84⊕Z2⊕Z2 | Z2⊕Z2 | Z6 |
Geometric properties
The 3-sphere is naturally a smooth manifold, in fact, a closed embedded submanifold of R4. The Euclidean metric on R4 induces a metricMetric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
on the 3-sphere giving it the structure of a Riemannian manifold
Riemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
. As with all spheres, the 3-sphere has constant positive sectional curvature
Sectional curvature
In Riemannian geometry, the sectional curvature is one of the ways to describe the curvature of Riemannian manifolds. The sectional curvature K depends on a two-dimensional plane σp in the tangent space at p...
equal to 1/r2 where r is the radius.
Much of the interesting geometry of the 3-sphere stems from the fact that the 3-sphere has a natural Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
structure given by quaternion multiplication (see the section below on group structure). The only other spheres with such a structure are the 0-sphere and the 1-sphere (see circle group).
Unlike the 2-sphere, the 3-sphere admits nonvanishing vector field
Vector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
s (sections
Section (fiber bundle)
In the mathematical field of topology, a section of a fiber bundle π is a continuous right inverse of the function π...
of its tangent bundle
Tangent bundle
In differential geometry, the tangent bundle of a differentiable manifold M is the disjoint unionThe disjoint union assures that for any two points x1 and x2 of manifold M the tangent spaces T1 and T2 have no common vector...
). One can even find three linearly-independent and nonvanishing vector fields. These may be taken to be any left-invariant vector fields forming a basis for the Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
of the 3-sphere. This implies that the 3-sphere is parallelizable. It follows that the tangent bundle of the 3-sphere is trivial. For a general discussion of the number of linear independent vector fields on a n-sphere, see the article vector fields on spheres
Vector fields on spheres
In mathematics, the discussion of vector fields on spheres was a classical problem of differential topology, beginning with the hairy ball theorem, and early work on the classification of division algebras....
.
There is an interesting action
Group action
In algebra and geometry, a group action is a way of describing symmetries of objects using groups. The essential elements of the object are described by a set, and the symmetries of the object are described by the symmetry group of this set, which consists of bijective transformations of the set...
of the circle group T on S3 giving the 3-sphere the structure of a principal circle bundle known as the Hopf bundle
Hopf bundle
In the mathematical field of topology, the Hopf fibration describes a 3-sphere in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle...
. If one thinks of S3 as a subset of C2, the action is given by
 .
.The orbit space of this action is homeomorphic to the two-sphere S2. Since S3 is not homeomorphic to S2×S1, the Hopf bundle is nontrivial.
Topological construction
There are several well-known constructions of the three-sphere. Here we describe gluing a pair of three-balls and then the one-point compactification.Gluing
A 3-sphere can be constructed topologicallyTopology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
by "gluing" together
Quotient space
In topology and related areas of mathematics, a quotient space is, intuitively speaking, the result of identifying or "gluing together" certain points of a given space. The points to be identified are specified by an equivalence relation...
the boundaries of a pair of 3-balls. The boundary of a 3-ball is a 2-sphere, and these two 2-spheres are to be identified. That is, imagine a pair of 3-balls of the same size, then superpose them so that their 2-spherical boundaries match, and let matching pairs of points on the pair of 2-spheres be identically equivalent to each other. In analogy with the case of the 2-sphere (see below), the gluing surface is called an equatorial sphere.
Note that the interiors of the 3-balls are not glued to each other. One way to think of the fourth dimension is as a continuous real-valued function of the 3-dimensional coordinates of the 3-ball, perhaps considered to be "temperature". We take the "temperature" to be zero along the gluing 2-sphere and let one of the 3-balls be "hot" and let the other 3-ball be "cold". The "hot" 3-ball could be thought of as the "upper hemisphere" and the "cold" 3-ball could be thought of as the "lower hemisphere". The temperature is highest/lowest at the centers of the two 3-balls.
This construction is analogous to a construction of a 2-sphere, performed by gluing the boundaries of a pair of disks. A disk is a 2-ball, and the boundary of a disk is a circle (a 1-sphere). Let a pair of disks be of the same diameter. Superpose them and glue corresponding points on their boundaries. Again one may think of the third dimension as temperature. Likewise, we may inflate the 2-sphere, moving the pair of disks to become the northern and southern hemispheres.
One-point compactification
After removing a single point from the 2-sphere, what remains is homeomorphic to the Euclidean plane. In the same way, removing a single point from the 3-sphere yields three-dimensional space.An extremely useful way to see this is via stereographic projection
Stereographic projection
The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it...
. We first describe the lower-dimensional version.
Rest the south pole of a unit 2-sphere on the xy-plane in three-space. We map a point
 of the sphere (minus the north pole
of the sphere (minus the north pole  ) to the plane by sending
) to the plane by sending 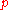 to the intersection of the line
to the intersection of the line 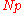 with the plane. Stereographic projection of a 3-sphere (again removing the north pole) maps to three-space in the same manner. (Notice that, since sterographic projection is conformal, round spheres are sent to round spheres or to planes.)
with the plane. Stereographic projection of a 3-sphere (again removing the north pole) maps to three-space in the same manner. (Notice that, since sterographic projection is conformal, round spheres are sent to round spheres or to planes.)A somewhat different way to think of the one-point compactification is via the exponential map
Exponential map
In differential geometry, the exponential map is a generalization of the ordinary exponential function of mathematical analysis to all differentiable manifolds with an affine connection....
. Returning to our picture of the unit two-sphere sitting on the Euclidean plane: Consider a geodesic in the plane, based at the origin, and map this to a geodesic in the two-sphere of the same length, based at the south pole. Under this map all points of the circle of radius
 are sent to the north pole. Since the open unit disk is homeomorphic to the Euclidean plane, this is again a one-point compactification.
are sent to the north pole. Since the open unit disk is homeomorphic to the Euclidean plane, this is again a one-point compactification.The exponential map for 3-sphere is similarly constructed; it may also be discussed using the fact that the 3-sphere is the Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
of unit quaternions.
Coordinate systems on the 3-sphere
The four Euclidean coordinates for S3 are redundant since they are subject to the condition that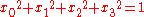 . As a 3-dimensional manifold one should be able to parameterize S3 by three coordinates, just as one can parameterize the 2-sphere using two coordinates (such as latitude
. As a 3-dimensional manifold one should be able to parameterize S3 by three coordinates, just as one can parameterize the 2-sphere using two coordinates (such as latitudeLatitude
In geography, the latitude of a location on the Earth is the angular distance of that location south or north of the Equator. The latitude is an angle, and is usually measured in degrees . The equator has a latitude of 0°, the North pole has a latitude of 90° north , and the South pole has a...
and longitude
Longitude
Longitude is a geographic coordinate that specifies the east-west position of a point on the Earth's surface. It is an angular measurement, usually expressed in degrees, minutes and seconds, and denoted by the Greek letter lambda ....
). Due to the nontrivial topology of S3 it is impossible to find a single set of coordinates that cover the entire space. Just as on the 2-sphere, one must use at least two coordinate charts. Some different choices of coordinates are given below.
Hyperspherical coordinates
It is convenient to have some sort of hyperspherical coordinates on S3 in analogy to the usual spherical coordinates on S2. One such choice — by no means unique — is to use (ψ, θ, φ), where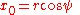

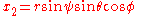
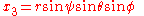
where ψ and θ run over the range 0 to π, and φ runs over 0 to 2π. Note that, for any fixed value of ψ, θ, and φ, parameterize a 2-sphere of radius sin(ψ), except for the degenerate cases, when ψ equals 0 or π, in which case they describe a point.
The round metric
Metric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
on the 3-sphere in these coordinates is given by
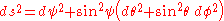
and the volume form
Volume form
In mathematics, a volume form on a differentiable manifold is a nowhere-vanishing differential form of top degree. Thus on a manifold M of dimension n, a volume form is an n-form, a section of the line bundle Ωn = Λn, that is nowhere equal to zero. A manifold has a volume...
by

These coordinates have an elegant description in terms of quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s. Any unit quaternion q can be written as a versor:
- q = eτψ = cos ψ + τ sin ψ
where τ is a unit imaginary quaternion; that is, any quaternion that satisfies τ2 = −1. This is the quaternionic analogue of Euler's formula
Euler's formula
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the deep relationship between the trigonometric functions and the complex exponential function...
. Now the unit imaginary quaternions all lie on the unit 2-sphere in Im H so any such τ can be written:
- τ = cos θ i + sin θ cos φ j + sin θ sin φ k
With τ in this form, the unit quaternion q is given by
- q = eτψ = x0 + x1 i + x2 j + x3 k
where the x’s are as above.
When q is used to describe spatial rotations (cf. quaternions and spatial rotation
Quaternions and spatial rotation
Unit quaternions provide a convenient mathematical notation for representing orientations and rotations of objects in three dimensions. Compared to Euler angles they are simpler to compose and avoid the problem of gimbal lock. Compared to rotation matrices they are more numerically stable and may...
s), it describes a rotation about τ through an angle of 2ψ.
Hopf coordinates
Another choice of hyperspherical coordinates, (η, ξ1, ξ2), makes use of the embedding of S3 in C2. In complex coordinates (z1, z2) ∈ C2 we write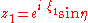

Here η runs over the range 0 to π/2, and ξ1 and ξ2 can take any values between 0 and 2π. These coordinates are useful in the description of the 3-sphere as the Hopf bundle
Hopf bundle
In the mathematical field of topology, the Hopf fibration describes a 3-sphere in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle...

For any fixed value of η between 0 and π/2, the coordinates (ξ1, ξ2) parameterize a 2-dimensional torus
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
. In the degenerate cases, when η equals 0 or π/2, these coordinates describe a circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
.
The round metric on the 3-sphere in these coordinates is given by
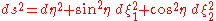
and the volume form by

Stereographic coordinates
Another convenient set of coordinates can be obtained via stereographic projectionStereographic projection
The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it...
of S3 from a pole onto the corresponding equatorial R3 hyperplane
Hyperplane
A hyperplane is a concept in geometry. It is a generalization of the plane into a different number of dimensions.A hyperplane of an n-dimensional space is a flat subset with dimension n − 1...
. For example, if we project from the point (−1, 0, 0, 0) we can write a point p in S3 as

where u = (u1, u2, u3) is a vector in R3 and ||u||2 = u12 + u22 + u32. In the second equality above, we have identified p with a unit quaternion and u = u1 i + u2 j + u3 k with a pure quaternion. (Note that the numerator and denominator commute here even though quaternionic multiplication is generally noncommutative). The inverse of this map takes p = (x0, x1, x2, x3) in S3 to

We could just as well have projected from the point (1, 0, 0, 0), in which case the point p is given by
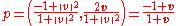
where v = (v1, v2, v3) is another vector in R3. The inverse of this map takes p to

Note that the u coordinates are defined everywhere but (−1, 0, 0, 0) and the v coordinates everywhere but (1, 0, 0, 0). This defines an atlas
Atlas (topology)
In mathematics, particularly topology, one describesa manifold using an atlas. An atlas consists of individualcharts that, roughly speaking, describe individual regionsof the manifold. If the manifold is the surface of the Earth,...
on S3 consisting of two coordinate charts or "patches", which together cover all of S3. Note that the transition function between these two charts on their overlap is given by

and vice-versa.
Group structure
When considered as the set of unit quaternionQuaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s, S3 inherits an important structure, namely that of quaternionic multiplication. Because the set of unit quaternions is closed under multiplication, S3 takes on the structure of a group
Group (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
. Moreover, since quaternionic multiplication is smooth
Smooth function
In mathematical analysis, a differentiability class is a classification of functions according to the properties of their derivatives. Higher order differentiability classes correspond to the existence of more derivatives. Functions that have derivatives of all orders are called smooth.Most of...
, S3 can be regarded as a real Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
. It is a nonabelian
Nonabelian group
In mathematics, a non-abelian group, also sometimes called a non-commutative group, is a group in which there are at least two elements a and b of G such that a * b ≠ b * a...
, compact
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
Lie group of dimension 3. When thought of as a Lie group S3 is often denoted Sp(1)
Symplectic group
In mathematics, the name symplectic group can refer to two different, but closely related, types of mathematical groups, denoted Sp and Sp. The latter is sometimes called the compact symplectic group to distinguish it from the former. Many authors prefer slightly different notations, usually...
or U(1, H).
It turns out that the only spheres
Hypersphere
In mathematics, an n-sphere is a generalization of the surface of an ordinary sphere to arbitrary dimension. For any natural number n, an n-sphere of radius r is defined as the set of points in -dimensional Euclidean space which are at distance r from a central point, where the radius r may be any...
that admit a Lie group structure are S1
Unit circle
In mathematics, a unit circle is a circle with a radius of one. Frequently, especially in trigonometry, "the" unit circle is the circle of radius one centered at the origin in the Cartesian coordinate system in the Euclidean plane...
, thought of as the set of unit complex number
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
s, and S3, the set of unit quaternions. One might think that S7, the set of unit octonion
Octonion
In mathematics, the octonions are a normed division algebra over the real numbers, usually represented by the capital letter O, using boldface O or blackboard bold \mathbb O. There are only four such algebras, the other three being the real numbers R, the complex numbers C, and the quaternions H...
s, would form a Lie group, but this fails since octonion multiplication is nonassociative. The octonionic structure does give S7 one important property: parallelizability. It turns out that the only spheres that are parallelizable are S1, S3, and S7.
By using a matrix representation of the quaternions, H, one obtains a matrix representation of S3. One convenient choice is given by the Pauli matrices
Pauli matrices
The Pauli matrices are a set of three 2 × 2 complex matrices which are Hermitian and unitary. Usually indicated by the Greek letter "sigma" , they are occasionally denoted with a "tau" when used in connection with isospin symmetries...
:
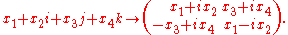
This map gives an injective algebra homomorphism
Algebra homomorphism
A homomorphism between two algebras, A and B, over a field K, is a map F:A\rightarrow B such that for all k in K and x,y in A,* F = kF* F = F + F...
from H to the set of 2×2 complex matrices. It has the property that the absolute value
Absolute value
In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...
of a quaternion q is equal to the square root
Square root
In mathematics, a square root of a number x is a number r such that r2 = x, or, in other words, a number r whose square is x...
of the determinant
Determinant
In linear algebra, the determinant is a value associated with a square matrix. It can be computed from the entries of the matrix by a specific arithmetic expression, while other ways to determine its value exist as well...
of the matrix image of q.
The set of unit quaternions is then given by matrices of the above form with unit determinant. This matrix subgroup is precisely the special unitary group
Special unitary group
The special unitary group of degree n, denoted SU, is the group of n×n unitary matrices with determinant 1. The group operation is that of matrix multiplication...
SU(2). Thus, S3 as a Lie group is isomorphic to SU(2).
Using our hyperspherical coordinates (η, ξ1, ξ2) we can then write any element of SU(2) in the form

Another way to state this result is if we express the matrix representation of an element of SU(2) as a linear combination of the Pauli matrices. It is seen that an arbitrary element
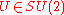 can be written as
can be written as  . The condition that the determinant of U is +1 implies that the coefficients
. The condition that the determinant of U is +1 implies that the coefficients 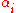 are constrained to lie on a 3-sphere.
are constrained to lie on a 3-sphere.In literature
In Edwin Abbott AbbottEdwin Abbott Abbott
Edwin Abbott Abbott , English schoolmaster and theologian, is best known as the author of the satirical novella Flatland .-Biography:...
's Flatland
Flatland
Flatland: A Romance of Many Dimensions is an 1884 satirical novella by the English schoolmaster Edwin Abbott Abbott. Writing pseudonymously as "A Square", Abbott used the fictional two-dimensional world of Flatland to offer pointed observations on the social hierarchy of Victorian culture...
, published in 1884, and in Sphereland
Sphereland
Sphereland: A Fantasy About Curved Spaces and an Expanding Universe is a 1965 novel by Dionys Burger, and is a sequel to Flatland, a novel by "A Square" . The novel expands upon the social and mathematical foundations on which Flatland is based...
, a 1965 sequel to Flatland by Dionys Burger
Dionys Burger
Dionys Burger was a Dutch mathematician and author of the novel Sphereland....
, the 3-sphere is referred to as an oversphere, and a 4-sphere is referred to as a hypersphere.
Writing in the American Journal of Physics
American Journal of Physics
The American Journal of Physics is a monthly, peer-reviewed scientific journal published by the American Association of Physics Teachers and the American Institute of Physics. The editor is Jan Tobochnik of Kalamazoo College.-Aims and scope:...
, Mark A. Peterson describes three different ways of visualizing 3-spheres and points out language in The Divine Comedy
The Divine Comedy
The Divine Comedy is an epic poem written by Dante Alighieri between 1308 and his death in 1321. It is widely considered the preeminent work of Italian literature, and is seen as one of the greatest works of world literature...
that suggests Dante
Dante Alighieri
Durante degli Alighieri, mononymously referred to as Dante , was an Italian poet, prose writer, literary theorist, moral philosopher, and political thinker. He is best known for the monumental epic poem La commedia, later named La divina commedia ...
viewed the Universe in the same way.

