Magnetic moment
Encyclopedia
The magnetic moment of a magnet
is a quantity that determines the force
that the magnet can exert on electric currents and the torque
that a magnetic field
will exert on it. A loop of electric current
, a bar magnet
, an electron
, a molecule
, and a planet
all have magnetic moments.
Both the magnetic moment and magnetic field may be considered to be vectors
having a magnitude and direction. The direction of the magnetic moment points from the south to north pole of a magnet. The magnetic field produced by a magnet is proportional to its magnetic moment as well. More precisely, the term magnetic moment normally refers to a system's magnetic dipole moment, which produces the first term in the multipole expansion
of a general magnetic field. The dipole
component of an object's magnetic field is symmetric about the direction of its magnetic dipole moment, and decreases as the inverse cube of the distance from the object.
. Consider a bar magnet which has magnetic poles of equal magnitude but opposite polarity. Each pole is the source of magnetic force which weakens with distance. Since magnetic poles always come in pairs, their forces partially cancel each other because while one pole pulls, the other repels. This cancellation is greatest when the poles are close to each other i.e. when the bar magnet is short. The magnetic force produced by a bar magnet, at a given point in space, therefore depends on two factors: on both the strength
of its poles, and on the vector 
separating them. The moment is defined as
It points in the direction from South to North pole. The analogy with electric dipoles should not be taken too far because magnetic dipoles are associated with angular momentum
(see Magnetic moment and angular momentum). Nevertheless, magnetic poles are very useful for magnetostatic
calculations, particularly in applications to ferromagnets. Practitioners using the magnetic pole approach generally represent the magnetic field
by the irrotational field
, in analogy to the electric field
.
and has vector area 
(
, 
, and 
coordinates of this vector are the areas of projections of the loop onto the 
, 
, and 
planes). Its magnetic moment 
, vector, is defined as:
By convention, the direction of the vector area is given by the right hand grip rule (curling the fingers of one's right hand in the direction of the current around the loop, when the palm of the hand is "touching" the loop's outer edge, and the straight thumb indicates the direction of the vector area and thus of the magnetic moment).
If the loop is not planar, the moment is given as
In the most general case of an arbitrary current distribution in space, the magnetic moment of such a distribution can be found from the following equation:
where
is the position vector pointing from the origin to the location of the volume element, and 
is the current density
vector at that location.
The above equation can be used for calculating a magnetic moment of any assembly of moving charges, such as a spinning charged solid, by substituting
where
is the electric charge density at a given point and 
is the instantaneous linear velocity of that point.
For example, the magnetic moment produced by an electric charge moving along a circular path is
where
is the position of the charge 
relative to the center of the circle and 
is the instantaneous velocity of the charge.
Practitioners using the current loop model generally represent the magnetic field by the solenoidal field
, analogous to the electrostatic field 
.
. Its moment is the vector sum of the moments of individual turns. If the solenoid has
identical turns (single-layer winding),
in the International System of Units
(SI) and it can be represented in more than one way. For example, in the current loop definition, the area is measured in square meters and
is measured in amperes, so the magnetic moment is measured in ampere–square meters (
). In the equation for torque on a moment, the torque is measured in joule
s and the magnetic field in tesla
, so the moment is measured in Joules per Tesla (
). These two representations are equivalent:
In the CGS
system, there are several different sets of electromagnetism units, of which the main ones are ESU, Gaussian
, and EMU. Among these, there are two alternative (non-equivalent) units of magnetic dipole moment in CGS:
and (more frequently used)
The ratio of these two non-equivalent CGS units (EMU/ESU) is equal exactly to the speed of light in free space
, expressed in cm
/s
.
All formulas in this article are correct in SI
units, but in other unit systems, the formulas may need to be changed. For example, in SI units, a loop of current with current I and area A has magnetic moment I×A (see below), but in Gaussian units
the magnetic moment is I×A/c
.
In a case when the external magnetic field is non-uniform, there will be a
force, proportional to the magnetic field gradient
, acting on the magnetic moment itself. There has been some discussion on how to calculate the force acting on a magnetic dipole. There are two expressions for the force acting on a magnetic dipole, depending on whether the model used for the dipole is a current loop or two monopoles (analogous to the electric dipole). The force obtained in the case of a current loop model is
\mathbf{F}_l=\nabla \left(\mathbf{m}\cdot\mathbf{B}\right)
In the case of a pair of monopoles are used (i.e. electric dipole model)
Magnet
A magnet is a material or object that produces a magnetic field. This magnetic field is invisible but is responsible for the most notable property of a magnet: a force that pulls on other ferromagnetic materials, such as iron, and attracts or repels other magnets.A permanent magnet is an object...
is a quantity that determines the force
Force
In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...
that the magnet can exert on electric currents and the torque
Torque
Torque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....
that a magnetic field
Magnetic field
A magnetic field is a mathematical description of the magnetic influence of electric currents and magnetic materials. The magnetic field at any given point is specified by both a direction and a magnitude ; as such it is a vector field.Technically, a magnetic field is a pseudo vector;...
will exert on it. A loop of electric current
Electric current
Electric current is a flow of electric charge through a medium.This charge is typically carried by moving electrons in a conductor such as wire...
, a bar magnet
Magnet
A magnet is a material or object that produces a magnetic field. This magnetic field is invisible but is responsible for the most notable property of a magnet: a force that pulls on other ferromagnetic materials, such as iron, and attracts or repels other magnets.A permanent magnet is an object...
, an electron
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
, a molecule
Molecule
A molecule is an electrically neutral group of at least two atoms held together by covalent chemical bonds. Molecules are distinguished from ions by their electrical charge...
, and a planet
Planet
A planet is a celestial body orbiting a star or stellar remnant that is massive enough to be rounded by its own gravity, is not massive enough to cause thermonuclear fusion, and has cleared its neighbouring region of planetesimals.The term planet is ancient, with ties to history, science,...
all have magnetic moments.
Both the magnetic moment and magnetic field may be considered to be vectors
Vector (mathematics and physics)
In mathematics and physics, a vector is an element of a vector space. If n is a non negative integer and K is either the field of the real numbers or the field of the complex number, then K^n is naturally endowed with a structure of vector space, where K^n is the set of the ordered sequences of n...
having a magnitude and direction. The direction of the magnetic moment points from the south to north pole of a magnet. The magnetic field produced by a magnet is proportional to its magnetic moment as well. More precisely, the term magnetic moment normally refers to a system's magnetic dipole moment, which produces the first term in the multipole expansion
Multipole expansion
A multipole expansion is a mathematical series representing a function that depends on angles — usually the two angles on a sphere. These series are useful because they can often be truncated, meaning that only the first few terms need to be retained for a good approximation to the original...
of a general magnetic field. The dipole
Dipole
In physics, there are several kinds of dipoles:*An electric dipole is a separation of positive and negative charges. The simplest example of this is a pair of electric charges of equal magnitude but opposite sign, separated by some distance. A permanent electric dipole is called an electret.*A...
component of an object's magnetic field is symmetric about the direction of its magnetic dipole moment, and decreases as the inverse cube of the distance from the object.
Two definitions of moment
In text books, two complementary approaches have been used to define magnetic moments. In pre-1930's textbooks, they were defined using magnetic poles. Most recent textbooks define it in terms of Ampèrian currents.Magnetic pole definition
The sources of magnetic moments in materials can be represented by poles in analogy to electrostaticsElectrostatics
Electrostatics is the branch of physics that deals with the phenomena and properties of stationary or slow-moving electric charges....
. Consider a bar magnet which has magnetic poles of equal magnitude but opposite polarity. Each pole is the source of magnetic force which weakens with distance. Since magnetic poles always come in pairs, their forces partially cancel each other because while one pole pulls, the other repels. This cancellation is greatest when the poles are close to each other i.e. when the bar magnet is short. The magnetic force produced by a bar magnet, at a given point in space, therefore depends on two factors: on both the strength
Magnetic pole strength
Magnetic pole strength is a physical quantity used to measure the strength of the pole of a bar magnet...
of its poles, and on the vector separating them. The moment is defined as
It points in the direction from South to North pole. The analogy with electric dipoles should not be taken too far because magnetic dipoles are associated with angular momentum
Angular momentum
In physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
(see Magnetic moment and angular momentum). Nevertheless, magnetic poles are very useful for magnetostatic
Magnetostatics
Magnetostatics is the study of magnetic fields in systems where the currents are steady . It is the magnetic analogue of electrostatics, where the charges are stationary. The magnetization need not be static; the equations of magnetostatics can be used to predict fast magnetic switching events that...
calculations, particularly in applications to ferromagnets. Practitioners using the magnetic pole approach generally represent the magnetic field
Magnetic field
A magnetic field is a mathematical description of the magnetic influence of electric currents and magnetic materials. The magnetic field at any given point is specified by both a direction and a magnitude ; as such it is a vector field.Technically, a magnetic field is a pseudo vector;...
by the irrotational field
, in analogy to the electric fieldElectric field
In physics, an electric field surrounds electrically charged particles and time-varying magnetic fields. The electric field depicts the force exerted on other electrically charged objects by the electrically charged particle the field is surrounding...
.Current loop definition
Suppose a planar closed loop carries an electric currentElectric current
Electric current is a flow of electric charge through a medium.This charge is typically carried by moving electrons in a conductor such as wire...
and has vector area (, , and coordinates of this vector are the areas of projections of the loop onto the , , and planes). Its magnetic moment , vector, is defined as:
By convention, the direction of the vector area is given by the right hand grip rule (curling the fingers of one's right hand in the direction of the current around the loop, when the palm of the hand is "touching" the loop's outer edge, and the straight thumb indicates the direction of the vector area and thus of the magnetic moment).
If the loop is not planar, the moment is given as
In the most general case of an arbitrary current distribution in space, the magnetic moment of such a distribution can be found from the following equation:
where
is the position vector pointing from the origin to the location of the volume element, and is the current densityCurrent density
Current density is a measure of the density of flow of a conserved charge. Usually the charge is the electric charge, in which case the associated current density is the electric current per unit area of cross section, but the term current density can also be applied to other conserved...
vector at that location.
The above equation can be used for calculating a magnetic moment of any assembly of moving charges, such as a spinning charged solid, by substituting
where
is the electric charge density at a given point and is the instantaneous linear velocity of that point.For example, the magnetic moment produced by an electric charge moving along a circular path is
-
,
where
is the position of the charge relative to the center of the circle and is the instantaneous velocity of the charge.Practitioners using the current loop model generally represent the magnetic field by the solenoidal field
, analogous to the electrostatic field .Magnetic moment of a solenoid
A generalization of the above current loop is a multi-turn coil, or solenoidSolenoid
A solenoid is a coil wound into a tightly packed helix. In physics, the term solenoid refers to a long, thin loop of wire, often wrapped around a metallic core, which produces a magnetic field when an electric current is passed through it. Solenoids are important because they can create...
. Its moment is the vector sum of the moments of individual turns. If the solenoid has
identical turns (single-layer winding),
Units
The unit for magnetic moment is not a base unitBase unit
Base unit may refer to:* Fundamental unit, in physics, used to form other compound units for other quantities* Computer case, the main body of a computer* An administrative unit of the United States Army Air Forces ms:Unit asas...
in the International System of Units
International System of Units
The International System of Units is the modern form of the metric system and is generally a system of units of measurement devised around seven base units and the convenience of the number ten. The older metric system included several groups of units...
(SI) and it can be represented in more than one way. For example, in the current loop definition, the area is measured in square meters and
is measured in amperes, so the magnetic moment is measured in ampere–square meters (). In the equation for torque on a moment, the torque is measured in jouleJoule
The joule ; symbol J) is a derived unit of energy or work in the International System of Units. It is equal to the energy expended in applying a force of one newton through a distance of one metre , or in passing an electric current of one ampere through a resistance of one ohm for one second...
s and the magnetic field in tesla
Tesla (unit)
The tesla is the SI derived unit of magnetic field B . One tesla is equal to one weber per square meter, and it was defined in 1960 in honour of the inventor, physicist, and electrical engineer Nikola Tesla...
, so the moment is measured in Joules per Tesla (
). These two representations are equivalent:
In the CGS
Centimetre gram second system of units
The centimetre–gram–second system is a metric system of physical units based on centimetre as the unit of length, gram as a unit of mass, and second as a unit of time...
system, there are several different sets of electromagnetism units, of which the main ones are ESU, Gaussian
Gaussian units
Gaussian units comprise a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units...
, and EMU. Among these, there are two alternative (non-equivalent) units of magnetic dipole moment in CGS:
- (ESU CGS) 1 statA·cm² = 3.33564095×10-14 (mMetreThe metre , symbol m, is the base unit of length in the International System of Units . Originally intended to be one ten-millionth of the distance from the Earth's equator to the North Pole , its definition has been periodically refined to reflect growing knowledge of metrology...
2·AAmpereThe ampere , often shortened to amp, is the SI unit of electric current and is one of the seven SI base units. It is named after André-Marie Ampère , French mathematician and physicist, considered the father of electrodynamics...
or JJouleThe joule ; symbol J) is a derived unit of energy or work in the International System of Units. It is equal to the energy expended in applying a force of one newton through a distance of one metre , or in passing an electric current of one ampere through a resistance of one ohm for one second...
/TTesla (unit)The tesla is the SI derived unit of magnetic field B . One tesla is equal to one weber per square meter, and it was defined in 1960 in honour of the inventor, physicist, and electrical engineer Nikola Tesla...
)
and (more frequently used)
- (EMU CGS and Gaussian-CGSGaussian unitsGaussian units comprise a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units...
) 1 ergErgAn erg is the unit of energy and mechanical work in the centimetre-gram-second system of units, symbol "erg". Its name is derived from the Greek ergon, meaning "work"....
/GGauss (unit)The gauss, abbreviated as G, is the cgs unit of measurement of a magnetic field B , named after the German mathematician and physicist Carl Friedrich Gauss. One gauss is defined as one maxwell per square centimeter; it equals 1 tesla...
= 1 abAAbampereThe abampere , also called the biot after Jean-Baptiste Biot, is the basic electromagnetic unit of electric current in the emu-cgs system of units . One abampere is equal to ten amperes in the SI system of units...
·cm² = 10-3 (mMetreThe metre , symbol m, is the base unit of length in the International System of Units . Originally intended to be one ten-millionth of the distance from the Earth's equator to the North Pole , its definition has been periodically refined to reflect growing knowledge of metrology...
2·AAmpereThe ampere , often shortened to amp, is the SI unit of electric current and is one of the seven SI base units. It is named after André-Marie Ampère , French mathematician and physicist, considered the father of electrodynamics...
or JJouleThe joule ; symbol J) is a derived unit of energy or work in the International System of Units. It is equal to the energy expended in applying a force of one newton through a distance of one metre , or in passing an electric current of one ampere through a resistance of one ohm for one second...
/TTesla (unit)The tesla is the SI derived unit of magnetic field B . One tesla is equal to one weber per square meter, and it was defined in 1960 in honour of the inventor, physicist, and electrical engineer Nikola Tesla...
).
The ratio of these two non-equivalent CGS units (EMU/ESU) is equal exactly to the speed of light in free space
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
, expressed in cm
Centimetre
A centimetre is a unit of length in the metric system, equal to one hundredth of a metre, which is the SI base unit of length. Centi is the SI prefix for a factor of . Hence a centimetre can be written as or — meaning or respectively...
/s
Second
The second is a unit of measurement of time, and is the International System of Units base unit of time. It may be measured using a clock....
.
All formulas in this article are correct in SI
Si
Si, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
units, but in other unit systems, the formulas may need to be changed. For example, in SI units, a loop of current with current I and area A has magnetic moment I×A (see below), but in Gaussian units
Gaussian units
Gaussian units comprise a metric system of physical units. This system is the most common of the several electromagnetic unit systems based on cgs units. It is also called the Gaussian unit system, Gaussian-cgs units, or often just cgs units...
the magnetic moment is I×A/c
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
.
Force on a moment
A magnetic moment in an externally-produced magnetic field has a potential energy U:
In a case when the external magnetic field is non-uniform, there will be a
force, proportional to the magnetic field gradient
Gradient
In vector calculus, the gradient of a scalar field is a vector field that points in the direction of the greatest rate of increase of the scalar field, and whose magnitude is the greatest rate of change....
, acting on the magnetic moment itself. There has been some discussion on how to calculate the force acting on a magnetic dipole. There are two expressions for the force acting on a magnetic dipole, depending on whether the model used for the dipole is a current loop or two monopoles (analogous to the electric dipole). The force obtained in the case of a current loop model is
\mathbf{F}_l=\nabla \left(\mathbf{m}\cdot\mathbf{B}\right)
In the case of a pair of monopoles are used (i.e. electric dipole model)
-
and one can be put in terms of the other via the relation
\mathbf{F}_l=\mathbf{F}_d + \mathbf{m}\times \left(\nabla \times \mathbf{B} \right)
In all these expressions
is the dipole and 
is the magnetic field at its position. Note that if there are no currents or time-varying electrical fields 
and the two expressions agree.
An electron, nucleus, or atom placed in a uniform magnetic field will precess with a frequency known as the Larmor frequency. See ResonanceResonanceIn physics, resonance is the tendency of a system to oscillate at a greater amplitude at some frequencies than at others. These are known as the system's resonant frequencies...
.
Torque on a moment
The magnetic moment can also be defined as a vector relating the aligning torqueTorqueTorque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....
on the object from an externally applied magnetic fieldMagnetic fieldA magnetic field is a mathematical description of the magnetic influence of electric currents and magnetic materials. The magnetic field at any given point is specified by both a direction and a magnitude ; as such it is a vector field.Technically, a magnetic field is a pseudo vector;...
to the field vector itself. The relationship is given by
where
is the torque and 
is the magnetic field.
Magnetic moment and angular momentum
The magnetic moment has a close connection with angular momentumAngular momentumIn physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
called the gyromagnetic effect. This effect is expressed on a macroscopic scale in the Einstein-de Haas effectEinstein-de Haas effectThe Einstein–de Haas effect, or the Richardson effect , is a physical phenomenon delineated by Albert Einstein and Wander Johannes de Haas in the mid 1910's, that exposes a relationship between magnetism, angular momentum, and the spin of elementary particles...
, or "rotation by magnetization," and its inverse, the Barnett effectBarnett EffectThe Barnett effect is the magnetization of an uncharged body when spun on its axis. It was discovered by American physicist Samuel Barnett in 1915....
, or "magnetization by rotation." In particular, when a magnetic moment is subject to a torqueTorqueTorque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....
in a magnetic field that tends to align it with the applied magnetic field, the moment precessesPrecessionPrecession is a change in the orientation of the rotation axis of a rotating body. It can be defined as a change in direction of the rotation axis in which the second Euler angle is constant...
(rotates about the axis of the applied field). This is a consequence of the angular momentum associated with the moment.
Viewing a magnetic dipole as a rotating charged sphere brings out the close connection between magnetic moment and angular momentum. Both the magnetic moment and the angular momentum increase with the rate of rotation of the sphere. The ratio of the two is called the gyromagnetic ratio, usually denoted by the symbol γ.
For a spinning charged solid with a uniform charge density to mass density ratio, the gyromagnetic ratio is equal to half the charge-to-mass ratio. This implies that a more massive assembly of charges spinning with the same angular momentumAngular momentumIn physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
will have a proportionateProportionality (mathematics)In mathematics, two variable quantities are proportional if one of them is always the product of the other and a constant quantity, called the coefficient of proportionality or proportionality constant. In other words, are proportional if the ratio \tfrac yx is constant. We also say that one...
ly weaker magnetic moment, compared to its lighter counterpart. Even though atomic particles cannot be accurately described as spinning charge distributions of uniform charge-to-mass ratio, this general trend can be observed in the atomic world, where the intrinsic angular momentum (spinSpin (physics)In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
) of each type of particle is a constant: a small half-integerHalf-integerIn mathematics, a half-integer is a number of the formn + 1/2,where n is an integer. For example,are all half-integers. Note that a half of an integer is not always a half-integer: half of an even integer is an integer but not a half-integer...
times the reduced Planck constantPlanck constantThe Planck constant , also called Planck's constant, is a physical constant reflecting the sizes of energy quanta in quantum mechanics. It is named after Max Planck, one of the founders of quantum theory, who discovered it in 1899...
. This is the basis for defining the magnetic moment units of Bohr magneton (assuming charge-to-mass ratio of the electronElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
) and nuclear magneton (assuming charge-to-mass ratio of the protonProtonThe proton is a subatomic particle with the symbol or and a positive electric charge of 1 elementary charge. One or more protons are present in the nucleus of each atom, along with neutrons. The number of protons in each atom is its atomic number....
).
Magnetic dipoles
A magnetic dipole is the limit of either a current loop or a pair of poles as the dimensions of the source are reduced to zero while keeping the moment constant. As long as these limits only applies to fields far from the sources, they are equivalent. However, the two models give different predictions for the internal field (see below).
External magnetic field produced by a magnetic dipole moment
Any system possessing a net magnetic dipole moment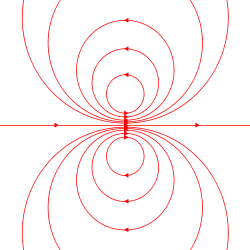
will produce a dipolarDipoleIn physics, there are several kinds of dipoles:*An electric dipole is a separation of positive and negative charges. The simplest example of this is a pair of electric charges of equal magnitude but opposite sign, separated by some distance. A permanent electric dipole is called an electret.*A...
magnetic field (described below) in the space surrounding the system. While the net magnetic field produced by the system can also have higher-order multipole components, those will drop off with distance more rapidly, so that only the dipolar component will dominate the magnetic field of the system at distances far away from it.
The vector potential of magnetic field produced by magnetic moment m is
and magnetic flux density is
Alternatively one can obtain the scalar potential first from the magnetic pole perspective,
and hence magnetic field strength is
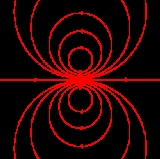
The magnetic field of an ideal magnetic dipoleDipoleIn physics, there are several kinds of dipoles:*An electric dipole is a separation of positive and negative charges. The simplest example of this is a pair of electric charges of equal magnitude but opposite sign, separated by some distance. A permanent electric dipole is called an electret.*A...
is depicted on the left.
Internal magnetic field of a dipole
The two models for a dipole (current loop and magnetic poles) give the same predictions for the magnetic field far from the source. However, inside the source region they give different predictions. The magnetic field between poles (see figure for Magnetic pole definition) is in the opposite direction to the magnetic moment (which points from the negative charge to the positive charge), while inside a current loop it is in the same direction (see the figure to the right). Clearly, the limits of these fields must also be different as the sources shrink to zero size. This distinction only matters if the dipole limit is used to calculate fields inside a magnetic material.
If a magnetic dipole is formed by making a current loop smaller and smaller, but keeping the product of current and area constant, the limiting field is
Unlike the expressions in the previous section, this limit is correct for the internal field of the dipole.
If a magnetic dipole is formed by taking a "north pole" and a "south pole", bringing them closer and closer together but keeping the product of magnetic pole-charge and distance constant, the limiting field is
These fields are related by
, where
is the magnetizationMagnetizationIn classical electromagnetism, magnetization or magnetic polarization is the vector field that expresses the density of permanent or induced magnetic dipole moments in a magnetic material...
.
Forces between two magnetic dipoles
If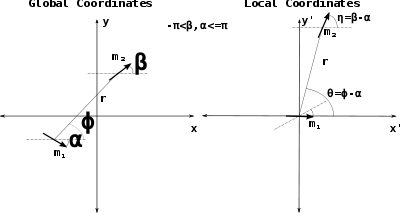
in the previous equations is replaced with the expression of the field of a magnetic dipole under the approximation for distances bigger than the characteristic length of the dipole. Namely,
B_{x'}(\mathbf{r}) = \frac{\mu_0}{4 \pi} m_1 \left(\frac{3\cos^2\theta-1}{r^3}\right)
B_{y'}(\mathbf{r}) = \frac{\mu_0}{4 \pi} m_1 \left(\frac{3\cos\theta\sin\theta}{r^3}\right)
where the variables
and 
are measured in a frame of reference with origin in 
and oriented in such a way that 
lies in the x-axis. This frame is called Local coordinates and is shown in the Figure on the right.
The final formulas are shown next. They are expressed in the global coordinate system,
-
-
Using vector notationVector notationThis page is an overview of the common notations used when working with vectors, which may be spatial or more abstract members of vector spaces....
, the above equations can be written as,
-
where
is the distance-vector from dipole moment 
to dipole moment 
, with 
, and where 
is the force acting on 
. The force acting on 
is in opposite direction.
The torque is straightforward to obtain from the formula
which gives
Two kinds of magnetic sources
Fundamentally, contributions to any system's magnetic moment may come from sources of two kinds: (1) motion of electric chargeElectric chargeElectric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two...
s, such as electric currentElectric currentElectric current is a flow of electric charge through a medium.This charge is typically carried by moving electrons in a conductor such as wire...
s, and (2) the intrinsic magnetism of elementary particleElementary particleIn particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
s, such as the electronElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
.
Contributions due to the sources of the first kind can be calculated from knowing the distribution of all the electric currents (or, alternatively, of all the electric charges and their velocities) inside the system, by using the formulas below. On the other hand, the magnitude of each elementary particle's intrinsic magnetic moment is a fixed number, often measured experimentally to a great precision. For example, any electron's magnetic moment is measured to be −9.284764×10−24 J/T. The direction of the magnetic moment of any elementary particle is entirely determined by the direction of its spinSpin (physics)In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
(the minusMínusMínus is an Icelandic alternative rock/hardcore band from Reykjavík. They are signed to the record label Smekkleysa. Mínus have shared the stage with, among others, Metallica, Foo Fighters, and Queens of the Stone Age...
in front of the value above indicates that any electron's magnetic moment is antiparallel to its spin).
The net magnetic moment of any system is a vector sum of contributions from one or both types of sources.
For example, the magnetic moment of an atom of hydrogen-1Hydrogen atomA hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively-charged proton and a single negatively-charged electron bound to the nucleus by the Coulomb force...
(the lightest hydrogen isotope, consisting of a proton and an electron) is a vector sum of the following contributions: (1) the intrinsic moment of the electron, (2) the orbital motion of the electron around the proton, (3) the intrinsic moment of the proton. Similarly, the magnetic moment of a bar magnet is the sum of the intrinsic and orbital magnetic moments of the unpaired electronElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
s of the magnet's material.
Magnetic moment of an atom
For an atom, individual electron spins are added to get a total spin, and individual orbital angular momenta are added to get a total orbital angular momentumAngular momentumIn physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
. These two then are added using angular momentum couplingAngular momentum couplingIn quantum mechanics, the procedure of constructing eigenstates of total angular momentum out of eigenstates of separate angular momenta is called angular momentum coupling. For instance, the orbit and spin of a single particle can interact through spin-orbit interaction, in which case the...
to get a total angular momentum. The magnitude of the atomic dipole moment is then:
where
is the total angular momentum quantum number, 
is the Landé g-factorLandé g-factorIn physics, the Landé g-factor is a particular example of a g-factor, namely for an electron with both spin and orbital angular momenta. It is named after Alfred Landé, who first described it in 1921....
, and
is the Bohr magneton. The component of this magnetic moment along the direction of the magnetic field is then:
where
is called the magnetic quantum numberMagnetic quantum numberIn atomic physics, the magnetic quantum number is the third of a set of quantum numbers which describe the unique quantum state of an electron and is designated by the letter m...
or the equatorial quantum number, which can take on any of
values: 
, 
, 
, 
, 
. The negative sign occurs because electrons have negative charge.
Because of the angular momentum, the dynamics of a magnetic dipole in a magnetic field differs from that of an electric dipole in an electric field. The field does exert a torque on the magnetic dipole tending to align it with the field. However, torque is proportional to rate of change of angular momentum, so precessionPrecessionPrecession is a change in the orientation of the rotation axis of a rotating body. It can be defined as a change in direction of the rotation axis in which the second Euler angle is constant...
occurs: the direction of spin changes. This behavior is described by the Landau-Lifshitz-Gilbert equation:
where
is gyromagnetic ratio, 
is magnetic moment, 
is damping coefficient and 
is effective magnetic field (the external field plus any self-field), and 
is the vector cross product. The first term describes precession of the moment about the effective field, while the second is a damping term related to dissipation of energy caused by interaction with surroundings.
Magnetic moment of an electron
ElectronElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
s and many elementary particles also have intrinsic magnetic momentsElectron magnetic dipole momentIn atomic physics, the electron magnetic dipole moment is the magnetic moment of an electron caused by its intrinsic property of spin.-Magnetic moment of an electron:...
, an explanation of which requires a quantum mechanical treatment and relates to the intrinsic angular momentumAngular momentumIn physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...
of the particles as discussed in the article electron magnetic dipole momentElectron magnetic dipole momentIn atomic physics, the electron magnetic dipole moment is the magnetic moment of an electron caused by its intrinsic property of spin.-Magnetic moment of an electron:...
. It is these intrinsic magnetic moments that give rise to the macroscopic effects of magnetismMagnetismMagnetism is a property of materials that respond at an atomic or subatomic level to an applied magnetic field. Ferromagnetism is the strongest and most familiar type of magnetism. It is responsible for the behavior of permanent magnets, which produce their own persistent magnetic fields, as well...
, and other phenomena, such as electron paramagnetic resonanceElectron paramagnetic resonanceElectron paramagnetic resonance or electron spin resonance spectroscopyis a technique for studying chemical species that have one or more unpaired electrons, such as organic and inorganic free radicals or inorganic complexes possessing a transition metal ion...
.
The magnetic moment of the electron is
where
-
is the Bohr magneton, 
is electron spinSpin (physics)In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
,
and the electron g-factor is
-
in DiracDirac equationThe Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
mechanics, but is slightly larger, -
in reality due to quantum electrodynamicsQuantum electrodynamicsQuantum electrodynamics is the relativistic quantum field theory of electrodynamics. In essence, it describes how light and matter interact and is the first theory where full agreement between quantum mechanics and special relativity is achieved...
effects.
Again it is important to notice that
is a negative constant multiplied by the spinSpin (physics)In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
, so the magnetic moment of the electron is antiparallel to the spin. This can be understood with the following classical picture: if we imagine that the spin angular momentum is created by the electron mass spinning around some axis, the electric current that this rotation creates circulates in the opposite direction, because of the negative charge of the electron; such current loops produce a magnetic moment which is antiparallel to the spin. Hence, for a positron (the anti-particle of the electron) the magnetic moment is parallel to its spin.
Magnetic moment of a nucleus
The nuclear system is a complex physical system consisting of nucleons, i.e., protonProtonThe proton is a subatomic particle with the symbol or and a positive electric charge of 1 elementary charge. One or more protons are present in the nucleus of each atom, along with neutrons. The number of protons in each atom is its atomic number....
s and neutronNeutronThe neutron is a subatomic hadron particle which has the symbol or , no net electric charge and a mass slightly larger than that of a proton. With the exception of hydrogen, nuclei of atoms consist of protons and neutrons, which are therefore collectively referred to as nucleons. The number of...
s. The quantum mechanical properties of the nucleons include the spin among others. Since the electromagnetic moments of the nucleus depend on the spin of the individual nucleons, one can look at these properties with measurements of nuclear moments, and more specifically the nuclear magnetic dipole moment.
Most common nuclei exist in their ground stateGround stateThe ground state of a quantum mechanical system is its lowest-energy state; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state...
, although nuclei of some isotopeIsotopeIsotopes are variants of atoms of a particular chemical element, which have differing numbers of neutrons. Atoms of a particular element by definition must contain the same number of protons but may have a distinct number of neutrons which differs from atom to atom, without changing the designation...
s have long-lived excited stateExcited stateExcitation is an elevation in energy level above an arbitrary baseline energy state. In physics there is a specific technical definition for energy level which is often associated with an atom being excited to an excited state....
s. Each energy state of a nucleus of a given isotope is characterized by a well-defined magnetic dipole moment, the magnitude of which is a fixed number, often measured experimentally to a great precision. This number is very sensitive to the individual contributions from nucleons, and a measurement or prediction of its value can reveal important information about the content of the nuclear wave function. There are several theoretical models that predict the value of the magnetic dipole moment and a number of experimental techniques aiming to carry out measurements in nuclei along the nuclear chart.
Magnetic moment of a molecule
Any molecule has a well-defined magnitude of magnetic moment, which may depend on the molecule's energy state. Typically, the overall magnetic moment of a molecule is a combination of the following contributions, in the order of their typical strength:
- magnetic moments due to its unpaired electron spins (paramagnetic contribution), if any
- orbital motion of its electrons, which in the ground state is often proportional to the external magnetic field (diamagnetic contribution)
- the combined magnetic moment of its nuclear spins, which depends on the nuclear spin configuration.
Examples of molecular magnetism
- OxygenOxygenOxygen is the element with atomic number 8 and represented by the symbol O. Its name derives from the Greek roots ὀξύς and -γενής , because at the time of naming, it was mistakenly thought that all acids required oxygen in their composition...
molecule, O2, exhibits strong paramagnetismParamagnetismParamagnetism is a form of magnetism whereby the paramagnetic material is only attracted when in the presence of an externally applied magnetic field. In contrast with this, diamagnetic materials are repulsive when placed in a magnetic field...
, due to unpaired spins of its outermost two electrons. - Carbon dioxideCarbon dioxideCarbon dioxide is a naturally occurring chemical compound composed of two oxygen atoms covalently bonded to a single carbon atom...
molecule, CO2, mostly exhibits diamagnetismDiamagnetismDiamagnetism is the property of an object which causes it to create a magnetic field in opposition to an externally applied magnetic field, thus causing a repulsive effect. Specifically, an external magnetic field alters the orbital velocity of electrons around their nuclei, thus changing the...
, a much weaker magnetic moment of the electron orbitalMolecular orbitalIn chemistry, a molecular orbital is a mathematical function describing the wave-like behavior of an electron in a molecule. This function can be used to calculate chemical and physical properties such as the probability of finding an electron in any specific region. The term "orbital" was first...
s that is proportional to the external magnetic field. In the rare instance when a magnetic isotopeIsotopeIsotopes are variants of atoms of a particular chemical element, which have differing numbers of neutrons. Atoms of a particular element by definition must contain the same number of protons but may have a distinct number of neutrons which differs from atom to atom, without changing the designation...
, such as 13C or 17O, is present, it will contribute its nuclear magnetism to the molecule's magnetic moment. - HydrogenHydrogenHydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly...
molecule, H2, in a weak (or zero) magnetic field exhibits nuclear magnetism, and can be in a para- or an ortho- nuclear spin configuration.
Elementary particles
In atomic and nuclear physics, the symbol
represents the magnitude of the magnetic moment, often measured in Bohr magnetons or nuclear magnetons, associated with the intrinsic spin of the particle and/or with the orbital motion of the particle in a system. Values of the intrinsic magnetic moments of some particles are given in the table below:
Intrinsic magnetic moments and spins of some elementary particles Particle Magnetic dipole moment in SI SiSi, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
units (10−27 J/TTesla (unit)The tesla is the SI derived unit of magnetic field B . One tesla is equal to one weber per square meter, and it was defined in 1960 in honour of the inventor, physicist, and electrical engineer Nikola Tesla...
)Spin quantum number Spin (physics)In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
(dimensionless)electron ElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
-9284.764 1/2 proton ProtonThe proton is a subatomic particle with the symbol or and a positive electric charge of 1 elementary charge. One or more protons are present in the nucleus of each atom, along with neutrons. The number of protons in each atom is its atomic number....
14.106067 1/2 neutron NeutronThe neutron is a subatomic hadron particle which has the symbol or , no net electric charge and a mass slightly larger than that of a proton. With the exception of hydrogen, nuclei of atoms consist of protons and neutrons, which are therefore collectively referred to as nucleons. The number of...
-9.66236 1/2 muon MuonThe muon |mu]] used to represent it) is an elementary particle similar to the electron, with a unitary negative electric charge and a spin of ½. Together with the electron, the tau, and the three neutrinos, it is classified as a lepton...
-44.904478 1/2 deuteron 4.3307346 1 triton TritiumTritium is a radioactive isotope of hydrogen. The nucleus of tritium contains one proton and two neutrons, whereas the nucleus of protium contains one proton and no neutrons...
15.046094 1/2
For relation between the notions of magnetic moment and magnetization see magnetizationMagnetizationIn classical electromagnetism, magnetization or magnetic polarization is the vector field that expresses the density of permanent or induced magnetic dipole moments in a magnetic material...
.
See also
- MagnetismMagnetismMagnetism is a property of materials that respond at an atomic or subatomic level to an applied magnetic field. Ferromagnetism is the strongest and most familiar type of magnetism. It is responsible for the behavior of permanent magnets, which produce their own persistent magnetic fields, as well...
- Magnetic dipole models
- DipoleDipoleIn physics, there are several kinds of dipoles:*An electric dipole is a separation of positive and negative charges. The simplest example of this is a pair of electric charges of equal magnitude but opposite sign, separated by some distance. A permanent electric dipole is called an electret.*A...
- Electric dipole momentElectric dipole momentIn physics, the electric dipole moment is a measure of the separation of positive and negative electrical charges in a system of charges, that is, a measure of the charge system's overall polarity with SI units of Coulomb-meter...
- MagnetizationMagnetizationIn classical electromagnetism, magnetization or magnetic polarization is the vector field that expresses the density of permanent or induced magnetic dipole moments in a magnetic material...
- Magnetic fieldMagnetic fieldA magnetic field is a mathematical description of the magnetic influence of electric currents and magnetic materials. The magnetic field at any given point is specified by both a direction and a magnitude ; as such it is a vector field.Technically, a magnetic field is a pseudo vector;...
- Magnetic susceptibilityMagnetic susceptibilityIn electromagnetism, the magnetic susceptibility \chi_m is a dimensionless proportionality constant that indicates the degree of magnetization of a material in response to an applied magnetic field...
- Magnetic dipole-dipole interactionMagnetic dipole-dipole interactionMagnetic dipole–dipole interaction, also called dipolar coupling, refers to the direct interaction between two magnetic dipoles. The potential energy of the interaction is as follows:...
- Landau-Lifshitz-Gilbert equation
-
-
-
