
Half-integer
Encyclopedia
In mathematics
, a half-integer is a number
of the form
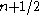 ,
,
where is an integer
is an integer
. For example,
are all half-integers. Note that a half of an integer is not always a half-integer: half of an even integer is an integer but not a half-integer. The half-integers are precisely those numbers that are half of an odd integer.
The set of all half-integers is often denoted
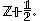
s in four dimensions places a sphere at every point whose coordinates are either all integers or all half-integers; this packing is closely related to the Hurwitz integers, which are quaternion
s whose real coefficients are either all integers or all half-integers.
Moreover, the Pauli exclusion principle
results from definition of fermion
s as particles which have spin
s that are half-integers. The energy level
s of the quantum harmonic oscillator
occur at half-integers and thus its lowest energy is not zero.
Also, the factorial
and gamma function
s, while not defined for negative and non-positive integers respectively, are defined for all half-integers as rational multiples of the square root
of pi
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a half-integer is a number
Number
A number is a mathematical object used to count and measure. In mathematics, the definition of number has been extended over the years to include such numbers as zero, negative numbers, rational numbers, irrational numbers, and complex numbers....
of the form
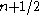 ,
,where
 is an integer
is an integerInteger
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
. For example,
- 4½, 7/2, −13/2, 8.5
are all half-integers. Note that a half of an integer is not always a half-integer: half of an even integer is an integer but not a half-integer. The half-integers are precisely those numbers that are half of an odd integer.
The set of all half-integers is often denoted
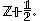
Uses
Half-integers occur frequently enough in mathematical contexts that a special term for them is convenient. For example, the densest lattice packing of unit sphereSphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
s in four dimensions places a sphere at every point whose coordinates are either all integers or all half-integers; this packing is closely related to the Hurwitz integers, which are quaternion
Quaternion
In mathematics, the quaternions are a number system that extends the complex numbers. They were first described by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space...
s whose real coefficients are either all integers or all half-integers.
Moreover, the Pauli exclusion principle
Pauli exclusion principle
The Pauli exclusion principle is the quantum mechanical principle that no two identical fermions may occupy the same quantum state simultaneously. A more rigorous statement is that the total wave function for two identical fermions is anti-symmetric with respect to exchange of the particles...
results from definition of fermion
Fermion
In particle physics, a fermion is any particle which obeys the Fermi–Dirac statistics . Fermions contrast with bosons which obey Bose–Einstein statistics....
s as particles which have spin
Spin (physics)
In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
s that are half-integers. The energy level
Energy level
A quantum mechanical system or particle that is bound -- that is, confined spatially—can only take on certain discrete values of energy. This contrasts with classical particles, which can have any energy. These discrete values are called energy levels...
s of the quantum harmonic oscillator
Quantum harmonic oscillator
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...
occur at half-integers and thus its lowest energy is not zero.
Also, the factorial
Factorial
In mathematics, the factorial of a non-negative integer n, denoted by n!, is the product of all positive integers less than or equal to n...
and gamma function
Gamma function
In mathematics, the gamma function is an extension of the factorial function, with its argument shifted down by 1, to real and complex numbers...
s, while not defined for negative and non-positive integers respectively, are defined for all half-integers as rational multiples of the square root
Square root
In mathematics, a square root of a number x is a number r such that r2 = x, or, in other words, a number r whose square is x...
of pi
Pi
' is a mathematical constant that is the ratio of any circle's circumference to its diameter. is approximately equal to 3.14. Many formulae in mathematics, science, and engineering involve , which makes it one of the most important mathematical constants...
.

