Casimir effect - AbsoluteAstronomy.com

Quantum field theory

Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...

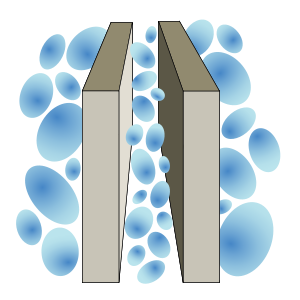
, the Casimir effect and the Casimir–Polder force are physical forces arising from a quantized field

Quantum field theory

. The typical example is of two uncharged

Electric charge

Electric charge is a physical property of matter that causes it to experience a force when near other electrically charged matter. Electric charge comes in two types, called positive and negative. Two positively charged substances, or objects, experience a mutual repulsive force, as do two...

metallic plates in a vacuum

Vacuum

In everyday usage, vacuum is a volume of space that is essentially empty of matter, such that its gaseous pressure is much less than atmospheric pressure. The word comes from the Latin term for "empty". A perfect vacuum would be one with no particles in it at all, which is impossible to achieve in...

, like capacitor

Capacitor

A capacitor is a passive two-terminal electrical component used to store energy in an electric field. The forms of practical capacitors vary widely, but all contain at least two electrical conductors separated by a dielectric ; for example, one common construction consists of metal foils separated...

s placed a few micrometers apart, without any external electromagnetic field

Electromagnetic field

An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...

. In a classical

Classical electromagnetism

Classical electromagnetism is a branch of theoretical physics that studies consequences of the electromagnetic forces between electric charges and currents...

description, the lack of an external field also means that there is no field between the plates, and no force would be measured between them. When this field is instead studied using quantum electrodynamics

Quantum electrodynamics

Quantum electrodynamics is the relativistic quantum field theory of electrodynamics. In essence, it describes how light and matter interact and is the first theory where full agreement between quantum mechanics and special relativity is achieved...

, it is seen that the plates do affect the virtual photons

Virtual particle

In physics, a virtual particle is a particle that exists for a limited time and space. The energy and momentum of a virtual particle are uncertain according to the uncertainty principle...

which constitute the field, and generate a net force—either an attraction or a repulsion depending on the specific arrangement of the two plates. Although the Casimir effect can be expressed in terms of virtual particle

Virtual particle

In physics, a virtual particle is a particle that exists for a limited time and space. The energy and momentum of a virtual particle are uncertain according to the uncertainty principle...

s interacting with the objects, it is best described and more easily calculated in terms of the zero-point energy

Zero-point energy

Zero-point energy is the lowest possible energy that a quantum mechanical physical system may have; it is the energy of its ground state. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave-like nature...

of a quantized field

Quantum field theory

in the intervening space between the objects. This force has been measured, and is a striking example of an effect purely due to second quantization. However, the treatment of boundary conditions in these calculations has led to some controversy.
In fact "Casimir's original goal was to compute the van der Waals force

Van der Waals force

In physical chemistry, the van der Waals force , named after Dutch scientist Johannes Diderik van der Waals, is the sum of the attractive or repulsive forces between molecules other than those due to covalent bonds or to the electrostatic interaction of ions with one another or with neutral...

between polarizable molecules" of the metallic plates. Thus it can be interpreted without any reference to the zero-point energy

Zero-point energy

(vacuum energy) or virtual particle

Virtual particle

In physics, a virtual particle is a particle that exists for a limited time and space. The energy and momentum of a virtual particle are uncertain according to the uncertainty principle...

s of quantum fields.

Dutch

Netherlands

The Netherlands is a constituent country of the Kingdom of the Netherlands, located mainly in North-West Europe and with several islands in the Caribbean. Mainland Netherlands borders the North Sea to the north and west, Belgium to the south, and Germany to the east, and shares maritime borders...

physicist

Physicist

A physicist is a scientist who studies or practices physics. Physicists study a wide range of physical phenomena in many branches of physics spanning all length scales: from sub-atomic particles of which all ordinary matter is made to the behavior of the material Universe as a whole...

s Hendrik B. G. Casimir

Hendrik Casimir

Hendrik Brugt Gerhard Casimir FRS was a Dutch physicist best known for his research on the two-fluid model of superconductors in 1934 and the Casimir effect Hendrik Brugt Gerhard Casimir FRS (July 15, 1909 in The Hague, Netherlands – May 4, 2000 in Heeze) was a Dutch physicist best known...

and Dirk Polder

Dirk Polder

Dirk Polder was a Dutch physicist who, together with Hendrik Casimir, first predicted the existence of what today is known as the Casimir-Polder force, sometimes also referred to as the Casimir effect or Casimir force. He also worked on the similar topic of radiative heat transfer at nanoscale.-...

proposed the existence of the force and formulated an experiment to detect it in 1948 while participating in research at Philips

Philips

Koninklijke Philips Electronics N.V. , more commonly known as Philips, is a multinational Dutch electronics company....

Research Labs. The classic form of the experiment, described above, successfully demonstrated the force to within 15% of the value predicted by the theory.

Because the strength of the force falls off rapidly with distance, it is only measurable when the distance between the objects is extremely small. On a submicron scale, this force becomes so strong that it becomes the dominant force between uncharged conductors. In fact, at separations of 10 nm—about 100 times the typical size of an atom—the Casimir effect produces the equivalent of 1 atmosphere

Atmosphere (unit)

The standard atmosphere is an international reference pressure defined as 101325 Pa and formerly used as unit of pressure. For practical purposes it has been replaced by the bar which is 105 Pa...

of pressure (101.325 kPa), the precise value depending on surface geometry and other factors.

In modern theoretical physics

Theoretical physics

Theoretical physics is a branch of physics which employs mathematical models and abstractions of physics to rationalize, explain and predict natural phenomena...

, the Casimir effect plays an important role in the chiral bag model of the nucleon

Nucleon

In physics, a nucleon is a collective name for two particles: the neutron and the proton. These are the two constituents of the atomic nucleus. Until the 1960s, the nucleons were thought to be elementary particles...

; and in applied physics

Applied physics

Applied physics is a general term for physics which is intended for a particular technological or practical use.It is usually considered as a bridge or a connection between "pure" physics and engineering....

, it is significant in some aspects of emerging microtechnologies and nanotechnologies.

Overview

The Casimir effect can be understood by the idea that the presence of conducting metals and dielectric

Dielectric

A dielectric is an electrical insulator that can be polarized by an applied electric field. When a dielectric is placed in an electric field, electric charges do not flow through the material, as in a conductor, but only slightly shift from their average equilibrium positions causing dielectric...

s alters the vacuum expectation value

Vacuum expectation value

In quantum field theory the vacuum expectation value of an operator is its average, expected value in the vacuum. The vacuum expectation value of an operator O is usually denoted by \langle O\rangle...

of the energy of the second quantized electromagnetic field

Electromagnetic field

. Since the value of this energy depends on the shapes and positions of the conductors and dielectrics, the Casimir effect makes itself manifest as a force between such objects.

Vacuum energy

The causes of the Casimir effect are described by quantum field theory

Quantum field theory

, which states that all of the various fundamental fields

Field (physics)

In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...

, such as the electromagnetic field

Electromagnetic field

, must be quantized at each and every point in space. In a simplified view, a "field" in physics may be envisioned as if space were filled with interconnected vibrating balls and springs, and the strength of the field can be visualized as the displacement of a ball from its rest position. Vibrations in this field propagate and are governed by the appropriate wave equation

Wave equation

The wave equation is an important second-order linear partial differential equation for the description of waves – as they occur in physics – such as sound waves, light waves and water waves. It arises in fields like acoustics, electromagnetics, and fluid dynamics...

for the particular field in question. The second quantization of quantum field theory requires that each such ball-spring combination be quantized, that is, that the strength of the field be quantized at each point in space. At the most basic level, the field at each point in space is a simple harmonic oscillator

Harmonic oscillator

In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force, F, proportional to the displacement, x: \vec F = -k \vec x \, where k is a positive constant....

, and its quantization places a quantum harmonic oscillator

Quantum harmonic oscillator

The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...

at each point. Excitations of the field correspond to the elementary particle

Elementary particle

In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...

s of particle physics

Particle physics

Particle physics is a branch of physics that studies the existence and interactions of particles that are the constituents of what is usually referred to as matter or radiation. In current understanding, particles are excitations of quantum fields and interact following their dynamics...

. However, even the vacuum

Vacuum

has a vastly complex structure, so all calculations of quantum field theory must be made in relation to this model of the vacuum.

The vacuum has, implicitly, all of the properties that a particle may have: spin

Spin (physics)

In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...

, or polarization in the case of light

Light

Light or visible light is electromagnetic radiation that is visible to the human eye, and is responsible for the sense of sight. Visible light has wavelength in a range from about 380 nanometres to about 740 nm, with a frequency range of about 405 THz to 790 THz...

, energy

Energy

In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...

, and so on. On average, most of these properties cancel out: the vacuum is, after all, "empty" in this sense. One important exception is the vacuum energy

Vacuum energy

Vacuum energy is an underlying background energy that exists in space even when the space is devoid of matter . The concept of vacuum energy has been deduced from the concept of virtual particles, which is itself derived from the energy-time uncertainty principle...

or the vacuum expectation value

Vacuum expectation value

of the energy. The quantization of a simple harmonic oscillator states that the lowest possible energy or zero-point energy

Zero-point energy

that such an oscillator may have is

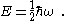
Summing over all possible oscillators at all points in space gives an infinite quantity. To remove this infinity, one may argue that only differences in energy are physically measurable; this argument is the underpinning of the theory of renormalization

Renormalization

In quantum field theory, the statistical mechanics of fields, and the theory of self-similar geometric structures, renormalization is any of a collection of techniques used to treat infinities arising in calculated quantities....

. In all practical calculations, this is how the infinity is always handled. In a deeper sense, however, renormalization is unsatisfying, and the removal of this infinity presents a challenge in the search for a Theory of Everything

Theory of everything

A theory of everything is a putative theory of theoretical physics that fully explains and links together all known physical phenomena, and predicts the outcome of any experiment that could be carried out in principle....

. Currently there is no compelling explanation for how this infinity should be treated as essentially zero; a non-zero value is essentially the cosmological constant

Cosmological constant

In physical cosmology, the cosmological constant was proposed by Albert Einstein as a modification of his original theory of general relativity to achieve a stationary universe...

and any large value causes trouble in cosmology

Physical cosmology

Physical cosmology, as a branch of astronomy, is the study of the largest-scale structures and dynamics of the universe and is concerned with fundamental questions about its formation and evolution. For most of human history, it was a branch of metaphysics and religion...

Effects

Casimir's observation was that the second-quantized

Canonical quantization

In physics, canonical quantization is a procedure for quantizing a classical theory while attempting to preserve the formal structure of the classical theory, to the extent possible. Historically, this was Werner Heisenberg's route to obtaining quantum mechanics...

quantum electromagnetic field, in the presence of bulk bodies such as metals or dielectric

Dielectric

s, must obey the same boundary condition

Boundary value problem

In mathematics, in the field of differential equations, a boundary value problem is a differential equation together with a set of additional restraints, called the boundary conditions...

s that the classical electromagnetic field must obey. In particular, this affects the calculation of the vacuum energy in the presence of a conductor

Electrical conductor

In physics and electrical engineering, a conductor is a material which contains movable electric charges. In metallic conductors such as copper or aluminum, the movable charged particles are electrons...

or dielectric.

Consider, for example, the calculation of the vacuum expectation value of the electromagnetic field inside a metal cavity, such as, for example, a radar cavity

Cavity magnetron

The cavity magnetron is a high-powered vacuum tube that generates microwaves using the interaction of a stream of electrons with a magnetic field. The 'resonant' cavity magnetron variant of the earlier magnetron tube was invented by John Randall and Harry Boot in 1940 at the University of...

or a microwave

Microwave

Microwaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...

waveguide

Waveguide

A waveguide is a structure which guides waves, such as electromagnetic waves or sound waves. There are different types of waveguides for each type of wave...

. In this case, the correct way to find the zero point energy of the field is to sum the energies of the standing wave

Standing wave

In physics, a standing wave – also known as a stationary wave – is a wave that remains in a constant position.This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling...

s of the cavity. To each and every possible standing wave corresponds an energy; say the energy of the nth standing wave is

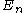. The vacuum expectation value of the energy of the electromagnetic field in the cavity is then

with the sum running over all possible values of n enumerating the standing waves. The factor of 1/2 corresponds to the fact that the zero-point energies are being summed (it is the same 1/2 as appears in the equation

). Written in this way, this sum is clearly divergent; however, it can be used to create finite expressions.

In particular, one may ask how the zero point energy depends on the shape s of the cavity. Each energy level

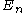depends on the shape, and so one should write

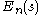for the energy level, and

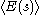for the vacuum expectation value. At this point comes an important observation: the force at point p on the wall of the cavity is equal to the change in the vacuum energy if the shape s of the wall is perturbed a little bit, say by

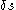, at point p. That is, one has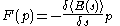

This value is finite in many practical calculations.

Casimir's calculation

In the original calculation done by Casimir, he considered the space between a pair of conducting metal plates at distance

apart. In this case, the standing waves are particularly easy to calculate, since the transverse component of the electric field and the normal component of the magnetic field must vanish on the surface of a conductor. Assuming the parallel plates lie in the xy-plane, the standing waves are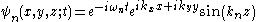

where

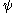stands for the electric component of the electromagnetic field, and, for brevity, the polarization and the magnetic components are ignored here. Here,

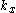and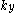

are the wave vector

Wave vector

In physics, a wave vector is a vector which helps describe a wave. Like any vector, it has a magnitude and direction, both of which are important: Its magnitude is either the wavenumber or angular wavenumber of the wave , and its direction is ordinarily the direction of wave propagation In...

s in directions parallel to the plates, and

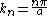
is the wave-vector perpendicular to the plates. Here, n is an integer, resulting from the requirement that ψ vanish on the metal plates. The energy of this wave is

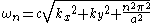
where c is the speed of light

Speed of light

The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...

. The vacuum energy is then the sum over all possible excitation modes

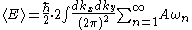
where A is the area of the metal plates, and a factor of 2 is introduced for the two possible polarizations of the wave. This expression is clearly infinite, and to proceed with the calculation, it is convenient to introduce a regulator

Regularization (physics)

-Introduction:In physics, especially quantum field theory, regularization is a method of dealing with infinite, divergent, and non-sensical expressions by introducing an auxiliary concept of a regulator...

(discussed in greater detail below). The regulator will serve to make the expression finite, and in the end will be removed. The zeta-regulated

Zeta function regularization

In mathematics and theoretical physics, zeta function regularization is a type of regularization or summability method that assigns finite values to divergent sums or products, and in particular can be used to define determinants and traces of some self-adjoint operators...

version of the energy per unit-area of the plate is

In the end, the limit

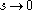is to be taken. Here s is just a complex number

Complex number

A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...

, not to be confused with the shape discussed previously. This integral/sum is finite for s real

Real number

In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...

and larger than 3. The sum has a pole at s = 3, but may be analytically continued

Analytic continuation

In complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which...

to s = 0, where the expression is finite. Expanding this, one gets

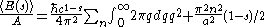
where polar coordinates

Polar coordinate system

In mathematics, the polar coordinate system is a two-dimensional coordinate system in which each point on a plane is determined by a distance from a fixed point and an angle from a fixed direction....

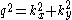were introduced to turn the double integral

Multiple integral

The multiple integral is a type of definite integral extended to functions of more than one real variable, for example, ƒ or ƒ...

into a single integral. The

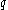in front is the Jacobian, and the

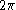comes from the angular integration. The integral is easily performed, resulting in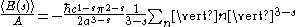

The sum may be understood to be the Riemann zeta function, and so one has

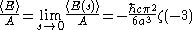
But

and so one obtains

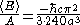
The Casimir force per unit area

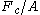for idealized, perfectly conducting plates with vacuum between them is

where

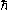(hbar, ħ) is the reduced Planck constant,

is the speed of light

Speed of light

is the distance

Distance

Distance is a numerical description of how far apart objects are. In physics or everyday discussion, distance may refer to a physical length, or an estimation based on other criteria . In mathematics, a distance function or metric is a generalization of the concept of physical distance...

between the two plates.

The force is negative, indicating that the force is attractive: by moving the two plates closer together, the energy is lowered. The presence of

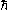shows that the Casimir force per unit area

is very small, and that furthermore, the force is inherently of quantum-mechanical origin.

More recent theory

Casimir's analysis of idealized metal plates was generalized to arbitrary dielectric and realistic metal plates by Lifshitz

Evgeny Lifshitz

Evgeny Mikhailovich Lifshitz was a leading Soviet physicist of Jewish origin and the brother of physicist Ilya Mikhailovich Lifshitz. Lifshitz is well known in general relativity for coauthoring the BKL conjecture concerning the nature of a generic curvature...

and his students. Using this approach, complications of the bounding surfaces, such as the modifications to the Casimir force due to finite conductivity, can be calculated numerically using the tabulated complex dielectric functions of the bounding materials. Lifshitz' theory for two metal plates reduces to Casimir's idealized 1/a⁴ force law for large separations a much greater than the skin depth of the metal, and conversely reduces to the 1/a³ force law of the London dispersion force

London dispersion force

London dispersion forces is a type of force acting between atoms and molecules. They are part of the van der Waals forces...

(with a coefficient called a Hamaker constant

Hamaker Constant

The Hamaker constant A can be defined for a Van der Waals body-body interaction:A=\pi^2\times C \times \rho_1 \times \rho_2where \rho_1 and \rho_2 are the number of atoms per unit volume in two interacting bodies and C is the coefficient in the particle-particle pair interaction.The Hamaker...

) for small a, with a more complicated dependence on a for intermediate separations determined by the dispersion

Dispersion (optics)

In optics, dispersion is the phenomenon in which the phase velocity of a wave depends on its frequency, or alternatively when the group velocity depends on the frequency.Media having such a property are termed dispersive media...

of the materials.

Lifshitz' result was subsequently generalized to arbitrary multilayer planar geometries as well as to anisotropic and magnetic materials, but for several decades the calculation of Casimir forces for non-planar geometries remained limited to a few idealized cases admitting analytical solutions. For example, the force in the experimental sphere–plate geometry was computed with an approximation (due to Derjaguin) that the sphere radius R is much larger than the separation a, in which case the nearby surfaces are nearly parallel and the parallel-plate result can be adapted to obtain an approximate R/a³ force (neglecting both skin-depth and higher-order

Orders of approximation

In science, engineering, and other quantitative disciplines, orders of approximation refer to formal or informal terms for how precise an approximation is, and to indicate progressively more refined approximations: in increasing order of precision, a zeroth order approximation, a first order...

curvature effects). However, in the 2000s a number of authors developed and demonstrated a variety of numerical techniques, in many cases adapted from classical computational electromagnetics

Computational electromagnetics

Computational electromagnetics, computational electrodynamics or electromagnetic modeling is the process of modeling the interaction of electromagnetic fields with physical objects and the environment....

, that are capable of accurately calculating Casimir forces for arbitrary geometries and materials, from simple finite-size effects of finite plates to more complicated phenomena arising for patterned surfaces or objects of various shapes.

Measurement

One of the first experimental tests was conducted by Marcus Sparnaay at Philips in Eindhoven, in 1958, in a delicate and difficult experiment with parallel plates, obtaining results not in contradiction with the Casimir theory, but with large experimental errors. Some of the experimental details as well as some background information on how Casimir, Polder and Sparnaay arrived at this point are highlighted in a 2007 interview with Marcus Sparnaay.

The Casimir effect was measured more accurately in 1997 by Steve K. Lamoreaux of Los Alamos National Laboratory

Los Alamos National Laboratory

Los Alamos National Laboratory is a United States Department of Energy national laboratory, managed and operated by Los Alamos National Security , located in Los Alamos, New Mexico...

, and by Umar Mohideen and Anushree Roy of the University of California at Riverside. In practice, rather than using two parallel plates, which would require phenomenally accurate alignment to ensure they were parallel, the experiments use one plate that is flat and another plate that is a part of a sphere

Sphere

A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...

with a large radius

Radius

In classical geometry, a radius of a circle or sphere is any line segment from its center to its perimeter. By extension, the radius of a circle or sphere is the length of any such segment, which is half the diameter. If the object does not have an obvious center, the term may refer to its...

.

In 2001, a group (Giacomo Bressi, Gianni Carugno, Roberto Onofrio and Giuseppe Ruoso) at the University of Padua (Italy)

University of Padua

The University of Padua is a premier Italian university located in the city of Padua, Italy. The University of Padua was founded in 1222 as a school of law and was one of the most prominent universities in early modern Europe. It is among the earliest universities of the world and the second...

finally succeeded in measuring the Casimir force between parallel plates using microresonators.

Regularisation

In order to be able to perform calculations in the general case, it is convenient to introduce a regulator

Regularization (physics)

in the summations. This is an artificial device, used to make the sums finite so that they can be more easily manipulated, followed by the taking of a limit so as to remove the regulator.

The heat kernel or exponentially

Exponential function

In mathematics, the exponential function is the function ex, where e is the number such that the function ex is its own derivative. The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change In mathematics,...

regulated sum is

where the limit

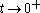is taken in the end. The divergence of the sum is typically manifested as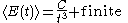

for three-dimensional cavities. The infinite part of the sum is associated with the bulk constant C which does not depend on the shape of the cavity. The interesting part of the sum is the finite part, which is shape-dependent. The Gaussian regulator

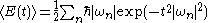
is better suited to numerical calculations because of its superior convergence properties, but is more difficult to use in theoretical calculations. Other, suitably smooth, regulators may be used as well. The zeta function regulator

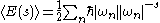
is completely unsuited for numerical calculations, but is quite useful in theoretical calculations. In particular, divergences show up as poles in the complex s plane

Complex plane

In mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...

, with the bulk divergence at s = 4. This sum may be analytically continued

Analytic continuation

past this pole, to obtain a finite part at s = 0.

Not every cavity configuration necessarily leads to a finite part (the lack of a pole at s = 0) or shape-independent infinite parts. In this case, it should be understood that additional physics has to be taken into account. In particular, at extremely large frequencies (above the plasma frequency), metals become transparent to photon

Photon

In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...

s (such as X-ray

X-ray

X-radiation is a form of electromagnetic radiation. X-rays have a wavelength in the range of 0.01 to 10 nanometers, corresponding to frequencies in the range 30 petahertz to 30 exahertz and energies in the range 120 eV to 120 keV. They are shorter in wavelength than UV rays and longer than gamma...

s), and dielectrics show a frequency-dependent cutoff as well. This frequency dependence acts as a natural regulator. There are a variety of bulk effects in solid state physics, mathematically very similar to the Casimir effect, where the cutoff frequency

Cutoff frequency

In physics and electrical engineering, a cutoff frequency, corner frequency, or break frequency is a boundary in a system's frequency response at which energy flowing through the system begins to be reduced rather than passing through.Typically in electronic systems such as filters and...

comes into explicit play to keep expressions finite. (These are discussed in greater detail in Landau and Lifshitz, "Theory of Continuous Media".)

Generalities

The Casimir effect can also be computed using the mathematical mechanisms of functional integrals of quantum field theory, although such calculations are considerably more abstract, and thus difficult to comprehend. In addition, they can be carried out only for the simplest of geometries. However, the formalism of quantum field theory makes it clear that the vacuum expectation value summations are in a certain sense summations over so-called "virtual particle

Virtual particle

In physics, a virtual particle is a particle that exists for a limited time and space. The energy and momentum of a virtual particle are uncertain according to the uncertainty principle...

s".

More interesting is the understanding that the sums over the energies of standing waves should be formally understood as sums over the eigenvalues of a Hamiltonian

Hamiltonian (quantum mechanics)

In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...

. This allows atomic and molecular effects, such as the van der Waals force

Van der Waals force

, to be understood as a variation on the theme of the Casimir effect. Thus one considers the Hamiltonian of a system as a function of the arrangement of objects, such as atoms, in configuration space

Configuration space

- Configuration space in physics :In classical mechanics, the configuration space is the space of possible positions that a physical system may attain, possibly subject to external constraints...

. The change in the zero-point energy as a function of changes of the configuration can be understood to result in forces acting between the objects.

In the chiral bag model of the nucleon, the Casimir energy plays an important role in showing the mass of the nucleon is independent of the bag radius. In addition, the spectral asymmetry

Spectral asymmetry

In mathematics and physics, the spectral asymmetry is the asymmetry in the distribution of the spectrum of eigenvalues of an operator. In mathematics, the spectral asymmetry arises in the study of elliptic operators on compact manifolds, and is given a deep meaning by the Atiyah-Singer index theorem...

is interpreted as a non-zero vacuum expectation value of the baryon number, cancelling the topological winding number of the pion

Pion

In particle physics, a pion is any of three subatomic particles: , , and . Pions are the lightest mesons and they play an important role in explaining the low-energy properties of the strong nuclear force....

field surrounding the nucleon.

Wormholes

Exotic matter

In physics, exotic matter is a term which refers to matter which would somehow deviate from the norm and have "exotic" properties. There are several uses of the term....

with negative energy density may be required to stabilize a wormhole

Wormhole

In physics, a wormhole is a hypothetical topological feature of spacetime that would be, fundamentally, a "shortcut" through spacetime. For a simple visual explanation of a wormhole, consider spacetime visualized as a two-dimensional surface. If this surface is folded along a third dimension, it...

. Morris, Thorne

Kip Thorne

Kip Stephen Thorne is an American theoretical physicist, known for his prolific contributions in gravitation physics and astrophysics and for having trained a generation of scientists...

and Yurtsever pointed out that the quantum mechanics of the Casimir effect can be used to produce a locally mass-negative region of space-time, and suggested that negative effect could be used to stabilize a wormhole to allow faster than light travel.

In physics

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

, a wormhole is a hypothetical topological

Topology

Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...

feature of spacetime

Spacetime

In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...

that would be, fundamentally, a "shortcut" through spacetime. For a simple visual explanation of a wormhole, consider spacetime visualized as a two-dimensional (2D) surface. If this surface is folded along a third dimension, it allows one to picture a wormhole "bridge". (Please note, though, that this is merely a visualization displayed to convey an essentially unvisualisable structure existing in 4 or more dimensions. The parts of the wormhole could be higher-dimensional analogues for the parts of the curved 2D surface; for example, instead of mouths which are circular holes in a 2D plane, a real wormhole's mouths could be spheres in 3D space.) A wormhole is, in theory, much like a tunnel with two ends each in separate points in spacetime, or it can be also known as two connecting black holes.

See also the Scharnhorst effect

Scharnhorst effect

The Scharnhorst effect is a hypothetical phenomenon in which light signals travel faster than c between two closely spaced conducting plates. It was predicted by Klaus Scharnhorst of the Humboldt University of Berlin, Germany, and Gabriel Barton of the University of Sussex in Brighton, England...

. This concept has been used extensively in science fiction

Science fiction

Science fiction is a genre of fiction dealing with imaginary but more or less plausible content such as future settings, futuristic science and technology, space travel, aliens, and paranormal abilities...

Analogies and the dynamic Casimir effect

A similar analysis can be used to explain Hawking radiation

Hawking radiation

Hawking radiation is a thermal radiation with a black body spectrum predicted to be emitted by black holes due to quantum effects. It is named after the physicist Stephen Hawking, who provided a theoretical argument for its existence in 1974, and sometimes also after the physicist Jacob Bekenstein...

that causes the slow "evaporation" of black hole

Black hole

A black hole is a region of spacetime from which nothing, not even light, can escape. The theory of general relativity predicts that a sufficiently compact mass will deform spacetime to form a black hole. Around a black hole there is a mathematically defined surface called an event horizon that...

s (although this is generally visualised as the escape of one particle from a virtual particle

Virtual particle

In physics, a virtual particle is a particle that exists for a limited time and space. The energy and momentum of a virtual particle are uncertain according to the uncertainty principle...

-antiparticle

Antiparticle

Corresponding to most kinds of particles, there is an associated antiparticle with the same mass and opposite electric charge. For example, the antiparticle of the electron is the positively charged antielectron, or positron, which is produced naturally in certain types of radioactive decay.The...

pair, the other particle having been captured by the black hole).

The dynamical Casimir effect is the production of particles and energy from an accelerated boundary, often referred to as a moving mirror or motion-induced radiation.

Constructed within the framework of quantum field theory in curved spacetime

Quantum field theory in curved spacetime

Quantum field theory in curved spacetime is an extension of standard, Minkowski-space quantum field theory to curved spacetime. A general prediction of this theory is that particles can be created by time dependent gravitational fields , or by time independent gravitational fields that contain...

, the dynamical Casimir effect has been used to better understand acceleration radiation; i.e. the Unruh effect

Unruh effect

The Unruh effect , was first described by Stephen Fulling in 1973, Paul Davies in 1975 and Bill Unruh in 1976. It is the prediction that an accelerating observer will observe black-body radiation where an inertial observer would observe none...

.

Moving mirrors create entropy, particles, energy and gravitational-like effects. In analogy to the event horizon of a black hole, an accelerated mirror amplifies quantum field vacuum fluctuations.

An experimental verification of the dynamical Casimir effect was first achieved in May 2011 at Chalmers University of Technology

Chalmers University of Technology

Chalmers University of Technology , is a Swedish university located in Gothenburg that focuses on research and education in technology, natural science and architecture.-History:...

, in Gothenburg , Sweden.

Repulsive forces

There are few instances wherein the Casimir effect can give rise to repulsive forces between uncharged objects. In a seminal paper, Evgeny Lifshitz

Evgeny Lifshitz

showed (theoretically) that in certain circumstances (most commonly involving liquids), repulsive forces can arise. This has sparked interest in applications of the Casimir effect toward the development of levitating devices. An experimental demonstration of the Casimir-based repulsion predicted by Lifshitz was recently carried out by Munday et al. Other scientists have also suggested the use of gain media to achieve a similar levitation effect, though this is controversial because these materials seem to violate fundamental causality constraints and the requirement of thermodynamic equilibrium.

Applications

It has been suggested that the Casimir forces have application in nanotechnology, in particular silicon integrated circuit technology based micro- and nanoelectromechanical systems, silicon array propulsion for space drives, and so-called Casimir oscillators.

Introductory readings

Casimir effect description from University of California, Riverside
University of California, Riverside
The University of California, Riverside, commonly known as UCR or UC Riverside, is a public research university and one of the ten general campuses of the University of California system. UCR is consistently ranked as one of the most ethnically and economically diverse universities in the United...

's version of the Usenet physics FAQ.
A. Lambrecht, The Casimir effect: a force from nothing, Physics World, September 2002.
Casimir effect on Astronomy Picture of the Day
Physicists have 'solved' mystery of levitation Telegraph interviews Prof. Ulf Leonhardt and Dr Thomas Philbin

Papers, books and lectures

H. B. G. Casimir
Hendrik Casimir
Hendrik Brugt Gerhard Casimir FRS was a Dutch physicist best known for his research on the two-fluid model of superconductors in 1934 and the Casimir effect Hendrik Brugt Gerhard Casimir FRS (July 15, 1909 in The Hague, Netherlands – May 4, 2000 in Heeze) was a Dutch physicist best known...

, and D. Polder
Dirk Polder
Dirk Polder was a Dutch physicist who, together with Hendrik Casimir, first predicted the existence of what today is known as the Casimir-Polder force, sometimes also referred to as the Casimir effect or Casimir force. He also worked on the similar topic of radiative heat transfer at nanoscale.-...

, "The Influence of Retardation on the London-van der Waals Forces", Phys. Rev. 73, 360–372 (1948).
S. K. Lamoreaux, "Demonstration of the Casimir Force in the 0.6 to 6 µm Range", Phys. Rev. Lett. 78, 5–8 (1997)
M. Bordag, U. Mohideen, V.M. Mostepanenko, "New Developments in the Casimir Effect", Phys. Rep. 353, 1–205 (2001), arXiv. (200+ page review paper.)
Kimball A.Milton: "The Casimir effect", World Scientific, Singapore 2001,ISBN 981-02-4397-9
J. D. Barrow, "Much ado about nothing", (2005) Lecture at Gresham College
Gresham College
Gresham College is an institution of higher learning located at Barnard's Inn Hall off Holborn in central London, England. It was founded in 1597 under the will of Sir Thomas Gresham and today it hosts over 140 free public lectures every year within the City of London.-History:Sir Thomas Gresham,...

. (Includes discussion of French naval analogy.) (Also includes discussion of French naval analogy.)
Jonathan P. Dowling, "The Mathematics of the Casimir Effect", Math. Mag.
Mathematics Magazine
Mathematics Magazine is a refereed bimonthly publication of the Mathematical Association of America. Its intended audience is teachers of collegiate mathematics, especially at the junior/senior level, and their students. It is explicitly a journal of mathematics rather than pedagogy...

62, 324–331 (1989).

Temperature dependence

Measurements Recast Usual View of Elusive Force from NIST
V.V. Nesterenko, G. Lambiase, G. Scarpetta, Calculation of the Casimir energy at zero and finite temperature: some recent results, arXiv:hep-th/0503100 v2 13 May 2005

External links

Casimir effect article search on arxiv.org
G. Lang, The Casimir Force web site, 2002
J. Babb, bibliography on the Casimir Effect web site, 2009

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.