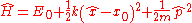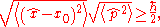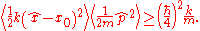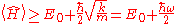Zero-point energy
Encyclopedia
Zero-point energy is the lowest possible energy
that a quantum mechanical physical system
may have; it is the energy of its ground state
. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave
-like nature. The uncertainty principle
requires every physical system to have a zero-point energy greater than the minimum of its classical potential well
, even at absolute zero
. For example, liquid helium
does not freeze under atmospheric pressure at any temperature because of its zero-point energy.
The concept of zero-point energy was developed in Germany by Albert Einstein
and Otto Stern
in 1913, using a formula developed by Max Planck
in 1900. The term zero-point energy originates
from the German Nullpunktsenergie. The German name is also spelled Nullpunktenergie (without the "s").
Vacuum energy
is the zero-point energy of all the field
s in space, which in the Standard Model
includes the electromagnetic field
, other gauge fields, fermionic field
s, and the Higgs field. It is the energy of the vacuum
, which in quantum field theory
is defined not as empty space but as the ground state of the fields. In cosmology
, the vacuum energy is one possible explanation for the cosmological constant
. A related term is zero-point field, which is the lowest energy state of a particular field.
derived the formula for the energy of a single energy radiator, e.g. a vibrating atomic unit:
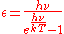
where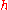
is Planck's constant, 
is the frequency
, k is Boltzmann's constant, and T is the absolute temperature
.
Then in 1913, using this formula as a basis, Albert Einstein
and Otto Stern
published a paper of great significance in which they suggested for the first time the existence of a residual energy that all oscillators have at absolute zero. They called this residual energy Nullpunktsenergie (German), later translated
as zero-point energy. They carried out an analysis of the specific heat of hydrogen gas at low temperature, and concluded that the data are best represented if the vibrational energy is
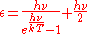
According to this expression, an atomic system at absolute zero
retains an energy of ½hν.
In 1916 Walther Nernst
postulated that the vacuum of space is filled with zero-point electromagnetic radiation.
Near the bottom of a potential well
, the Hamiltonian
of a system (the quantum-mechanical operator
giving its energy) can be approximated as
where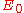
is the minimum of the classical potential well. The uncertainty principle tells us that
making the expectation value
s of the kinetic
and potential
terms above satisfy
The expectation value of the energy must therefore be at least
where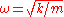
is the angular frequency
at which the system oscillates.
A more thorough treatment, showing that the energy of the ground state actually is
requires solving for the ground state of the system. See quantum harmonic oscillator
for details.
In ordinary quantum mechanics, the zero-point energy is the energy associated with the ground state
of the system. The professional physics literature tends to measure frequency, as denoted by
above, using angular frequency
, denoted with
and defined by 
= 
. This leads to a convention of writing Planck's constant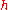 
with a bar through its top to denote the quantity 
/
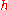. In those terms, the most famous such example of zero-point energy is 
associated with the ground state of the quantum harmonic oscillator
. In quantum mechanical terms, the zero-point energy is the expectation value of the Hamiltonian
of the system in the ground state.
In quantum field theory
, the fabric of space is visualized as consisting of fields
, with the field at every point in space and time being a quantum harmonic oscillator
, with neighboring oscillators interacting. In this case, one has a contribution of
from every point in space, resulting in a calculation of infinite zero-point energy in any finite volume; this is one reason renormalization
is needed to make sense of quantum field theories. The zero-point energy is again the expectation value of the Hamiltonian; here, however, the phrase vacuum expectation value
is more commonly used, and the energy is called the vacuum energy
.
In quantum perturbation theory
, it is sometimes said that the contribution of one-loop and multi-loop Feynman diagram
s to elementary particle
propagator
s are the contribution of vacuum fluctuations or the zero-point energy to the particle mass
es.
, proposed in 1948 by Dutch
physicist
Hendrik B. G. Casimir
(Philips Research
), who considered the quantized electromagnetic field
between a pair of grounded, neutral metal plates. The vacuum energy contains contributions from all wavelengths, except those excluded by the spacing between plates. As the plates draw together, more wavelengths are excluded and the vacuum energy decreases. The decrease in energy means there must be a force doing work on the plates as they move. This force has been measured and found to be in good agreement with the theory. However, there is still some debate on whether vacuum energy is necessary to explain the Casimir effect. Robert Jaffe
of MIT argues that the Casimir force should not be considered evidence for vacuum energy, since it can be derived in QED
without reference to vacuum energy by considering charge-current interactions (the radiation-reaction picture).
The experimentally measured Lamb shift has been argued to be, in part, a zero-point energy effect.
, the zero-point energy offers an intriguing possibility for explaining the speculative positive values of the proposed cosmological constant
. In brief, if the energy is "really there", then it should exert a gravitational force. In general relativity
, mass
and energy are equivalent; both produce a gravitational field. One obvious difficulty with this association is that the zero-point energy of the vacuum is absurdly large. Naively, it is infinite, because it includes the energy of waves with arbitrarily short wavelengths. But since only differences in energy are physically measurable, the infinity can be removed by renormalization
. In all practical calculations, this is how the infinity is handled. It is also arguable that undiscovered physics relevant at the Planck scale
reduces or eliminates the energy of waves shorter than the Planck length, making the total zero-point energy finite.
.
The discovery of zero-point energy did not alter the implausibility of perpetual motion machines. Much attention has been given to reputable science suggesting that zero-point-energy density is infinite, but in quantum theory, zero-point energy is a minimum energy below which a thermodynamic system
can never go. Thus according to the standard quantum-theoretic viewpoint, none of this energy can be withdrawn without altering the system to a different form in which the system has a lower zero-point energy.
It is possible that the discovery of new physics will alter this conclusion. For example, in stochastic electrodynamics
, the zero-point field is viewed as simply a classical background isotropic noise wave field which excites all systems present in the vacuum and thus is responsible for their minimum-energy or "ground" states. The requirement of Lorentz invariance at a statistical level then implies that the energy density spectrum must increase with the third power of frequency, implying infinite energy density when integrated over all frequencies. If this theory is correct, there is no reason that energy, or for that matter, momentum, could not be extracted, and would of course still leave infinite energy density and infinite momentum density, isotropic in all directions simultaneously, remaining in the wave field.
According to NASA, "the concept of accessing a significant amount of useful energy from the ZPE gained much credibility when a major article on this topic was recently [March 1st, 2004] published in Aviation Week & Space Technology, a leading aerospace industry magazine".
The calculation that underlies the Casimir experiment, a calculation based on the formula predicting infinite vacuum energy, shows the zero-point energy of a system consisting of a vacuum between two plates will decrease at a finite rate as the two plates are drawn together. The vacuum energies are predicted to be infinite, but the changes are predicted to be finite. Casimir combined the projected rate of change in zero-point energy with the principle of conservation of energy to predict a force on the plates. The predicted force, which is very small and was experimentally measured to be within 5% of its predicted value, is finite. Even though the zero-point energy is theoretically infinite, there is as yet no practical evidence to suggest that infinite amounts of zero-point energy are available for use, that zero-point energy can be withdrawn for free, or that zero-point energy can be used in violation of conservation of energy.
Energy
In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
that a quantum mechanical physical system
Physical system
In physics, the word system has a technical meaning, namely, it is the portion of the physical universe chosen for analysis. Everything outside the system is known as the environment, which in analysis is ignored except for its effects on the system. The cut between system and the world is a free...
may have; it is the energy of its ground state
Ground state
The ground state of a quantum mechanical system is its lowest-energy state; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state...
. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave
Wave
In physics, a wave is a disturbance that travels through space and time, accompanied by the transfer of energy.Waves travel and the wave motion transfers energy from one point to another, often with no permanent displacement of the particles of the medium—that is, with little or no associated mass...
-like nature. The uncertainty principle
Uncertainty principle
In quantum mechanics, the Heisenberg uncertainty principle states a fundamental limit on the accuracy with which certain pairs of physical properties of a particle, such as position and momentum, can be simultaneously known...
requires every physical system to have a zero-point energy greater than the minimum of its classical potential well
Potential well
A potential well is the region surrounding a local minimum of potential energy. Energy captured in a potential well is unable to convert to another type of energy because it is captured in the local minimum of a potential well...
, even at absolute zero
Absolute zero
Absolute zero is the theoretical temperature at which entropy reaches its minimum value. The laws of thermodynamics state that absolute zero cannot be reached using only thermodynamic means....
. For example, liquid helium
Liquid helium
Helium exists in liquid form only at extremely low temperatures. The boiling point and critical point depend on the isotope of the helium; see the table below for values. The density of liquid helium-4 at its boiling point and 1 atmosphere is approximately 0.125 g/mL Helium-4 was first liquefied...
does not freeze under atmospheric pressure at any temperature because of its zero-point energy.
The concept of zero-point energy was developed in Germany by Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
and Otto Stern
Otto Stern
Otto Stern was a German physicist and Nobel laureate in physics.-Biography:Stern was born in Sohrau, now Żory in the German Empire's Kingdom of Prussia and studied at Breslau, now Wrocław in Lower Silesia....
in 1913, using a formula developed by Max Planck
Max Planck
Max Karl Ernst Ludwig Planck, ForMemRS, was a German physicist who actualized the quantum physics, initiating a revolution in natural science and philosophy. He is regarded as the founder of the quantum theory, for which he received the Nobel Prize in Physics in 1918.-Life and career:Planck came...
in 1900. The term zero-point energy originates
Calque
In linguistics, a calque or loan translation is a word or phrase borrowed from another language by literal, word-for-word or root-for-root translation.-Calque:...
from the German Nullpunktsenergie. The German name is also spelled Nullpunktenergie (without the "s").
Vacuum energy
Vacuum energy
Vacuum energy is an underlying background energy that exists in space even when the space is devoid of matter . The concept of vacuum energy has been deduced from the concept of virtual particles, which is itself derived from the energy-time uncertainty principle...
is the zero-point energy of all the field
Field (physics)
In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...
s in space, which in the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
includes the electromagnetic field
Electromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
, other gauge fields, fermionic field
Fermionic field
In quantum field theory, a fermionic field is a quantum field whose quanta are fermions; that is, they obey Fermi-Dirac statistics. Fermionic fields obey canonical anticommutation relations rather than the canonical commutation relations of bosonic fields....
s, and the Higgs field. It is the energy of the vacuum
Vacuum state
In quantum field theory, the vacuum state is the quantum state with the lowest possible energy. Generally, it contains no physical particles...
, which in quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
is defined not as empty space but as the ground state of the fields. In cosmology
Physical cosmology
Physical cosmology, as a branch of astronomy, is the study of the largest-scale structures and dynamics of the universe and is concerned with fundamental questions about its formation and evolution. For most of human history, it was a branch of metaphysics and religion...
, the vacuum energy is one possible explanation for the cosmological constant
Cosmological constant
In physical cosmology, the cosmological constant was proposed by Albert Einstein as a modification of his original theory of general relativity to achieve a stationary universe...
. A related term is zero-point field, which is the lowest energy state of a particular field.
History
In 1900, Max PlanckMax Planck
Max Karl Ernst Ludwig Planck, ForMemRS, was a German physicist who actualized the quantum physics, initiating a revolution in natural science and philosophy. He is regarded as the founder of the quantum theory, for which he received the Nobel Prize in Physics in 1918.-Life and career:Planck came...
derived the formula for the energy of a single energy radiator, e.g. a vibrating atomic unit:
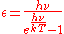
where
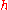is Planck's constant, is the frequencyFrequency
Frequency is the number of occurrences of a repeating event per unit time. It is also referred to as temporal frequency.The period is the duration of one cycle in a repeating event, so the period is the reciprocal of the frequency...
, k is Boltzmann's constant, and T is the absolute temperature
Temperature
Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
.
Then in 1913, using this formula as a basis, Albert Einstein
Albert Einstein
Albert Einstein was a German-born theoretical physicist who developed the theory of general relativity, effecting a revolution in physics. For this achievement, Einstein is often regarded as the father of modern physics and one of the most prolific intellects in human history...
and Otto Stern
Otto Stern
Otto Stern was a German physicist and Nobel laureate in physics.-Biography:Stern was born in Sohrau, now Żory in the German Empire's Kingdom of Prussia and studied at Breslau, now Wrocław in Lower Silesia....
published a paper of great significance in which they suggested for the first time the existence of a residual energy that all oscillators have at absolute zero. They called this residual energy Nullpunktsenergie (German), later translated
Calque
In linguistics, a calque or loan translation is a word or phrase borrowed from another language by literal, word-for-word or root-for-root translation.-Calque:...
as zero-point energy. They carried out an analysis of the specific heat of hydrogen gas at low temperature, and concluded that the data are best represented if the vibrational energy is
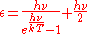
According to this expression, an atomic system at absolute zero
Absolute zero
Absolute zero is the theoretical temperature at which entropy reaches its minimum value. The laws of thermodynamics state that absolute zero cannot be reached using only thermodynamic means....
retains an energy of ½hν.
In 1916 Walther Nernst
Walther Nernst
Walther Hermann Nernst FRS was a German physical chemist and physicist who is known for his theories behind the calculation of chemical affinity as embodied in the third law of thermodynamics, for which he won the 1920 Nobel Prize in chemistry...
postulated that the vacuum of space is filled with zero-point electromagnetic radiation.
Relation to the uncertainty principle
Zero-point energy is fundamentally related to the Heisenberg uncertainty principle. Roughly speaking, the uncertainty principle states that complementary variables (such as a particle's position and momentum, or a field's value and derivative at a point in space) cannot simultaneously be defined precisely by any given quantum state. In particular, there cannot be a state in which the system sits motionless at the bottom of its potential well, for then its position and momentum would both be completely determined to arbitrarily great precision. Therefore, the lowest-energy state (the ground state) of the system must have a distribution in position and momentum that satisfies the uncertainty principle, which implies its energy must be greater than the minimum of the potential well.Near the bottom of a potential well
Potential well
A potential well is the region surrounding a local minimum of potential energy. Energy captured in a potential well is unable to convert to another type of energy because it is captured in the local minimum of a potential well...
, the Hamiltonian
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
of a system (the quantum-mechanical operator
Operator (physics)
In physics, an operator is a function acting on the space of physical states. As a resultof its application on a physical state, another physical state is obtained, very often along withsome extra relevant information....
giving its energy) can be approximated as
where
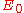is the minimum of the classical potential well. The uncertainty principle tells us that
making the expectation value
Expectation value (quantum mechanics)
In quantum mechanics, the expectation value is the predicted mean value of the result of an experiment. Despite the name, it is not the most probable value of a measurement...
s of the kinetic
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
and potential
Potential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
terms above satisfy
The expectation value of the energy must therefore be at least
where
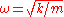is the angular frequencyAngular frequency
In physics, angular frequency ω is a scalar measure of rotation rate. Angular frequency is the magnitude of the vector quantity angular velocity...
at which the system oscillates.
A more thorough treatment, showing that the energy of the ground state actually is
requires solving for the ground state of the system. See quantum harmonic oscillatorQuantum harmonic oscillator
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...
for details.
Varieties
The concept of zero-point energy occurs in a number of situations.In ordinary quantum mechanics, the zero-point energy is the energy associated with the ground state
Ground state
The ground state of a quantum mechanical system is its lowest-energy state; the energy of the ground state is known as the zero-point energy of the system. An excited state is any state with energy greater than the ground state...
of the system. The professional physics literature tends to measure frequency, as denoted by
above, using angular frequencyAngular frequency
In physics, angular frequency ω is a scalar measure of rotation rate. Angular frequency is the magnitude of the vector quantity angular velocity...
, denoted with
and defined by = . This leads to a convention of writing Planck's constant 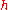with a bar through its top to denote the quantity 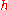/. In those terms, the most famous such example of zero-point energy is associated with the ground state of the quantum harmonic oscillatorQuantum harmonic oscillator
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...
. In quantum mechanical terms, the zero-point energy is the expectation value of the Hamiltonian
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
of the system in the ground state.
In quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
, the fabric of space is visualized as consisting of fields
Field (physics)
In physics, a field is a physical quantity associated with each point of spacetime. A field can be classified as a scalar field, a vector field, a spinor field, or a tensor field according to whether the value of the field at each point is a scalar, a vector, a spinor or, more generally, a tensor,...
, with the field at every point in space and time being a quantum harmonic oscillator
Quantum harmonic oscillator
The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...
, with neighboring oscillators interacting. In this case, one has a contribution of
from every point in space, resulting in a calculation of infinite zero-point energy in any finite volume; this is one reason renormalizationRenormalization
In quantum field theory, the statistical mechanics of fields, and the theory of self-similar geometric structures, renormalization is any of a collection of techniques used to treat infinities arising in calculated quantities....
is needed to make sense of quantum field theories. The zero-point energy is again the expectation value of the Hamiltonian; here, however, the phrase vacuum expectation value
Vacuum expectation value
In quantum field theory the vacuum expectation value of an operator is its average, expected value in the vacuum. The vacuum expectation value of an operator O is usually denoted by \langle O\rangle...
is more commonly used, and the energy is called the vacuum energy
Vacuum energy
Vacuum energy is an underlying background energy that exists in space even when the space is devoid of matter . The concept of vacuum energy has been deduced from the concept of virtual particles, which is itself derived from the energy-time uncertainty principle...
.
In quantum perturbation theory
Perturbation theory
Perturbation theory comprises mathematical methods that are used to find an approximate solution to a problem which cannot be solved exactly, by starting from the exact solution of a related problem...
, it is sometimes said that the contribution of one-loop and multi-loop Feynman diagram
Feynman diagram
Feynman diagrams are a pictorial representation scheme for the mathematical expressions governing the behavior of subatomic particles, first developed by the Nobel Prize-winning American physicist Richard Feynman, and first introduced in 1948...
s to elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
propagator
Propagator
In quantum mechanics and quantum field theory, the propagator gives the probability amplitude for a particle to travel from one place to another in a given time, or to travel with a certain energy and momentum. Propagators are used to represent the contribution of virtual particles on the internal...
s are the contribution of vacuum fluctuations or the zero-point energy to the particle mass
Mass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
es.
Experimental observations
A phenomenon that is commonly presented as evidence for the existence of zero-point energy in vacuum is the Casimir effectCasimir effect
In quantum field theory, the Casimir effect and the Casimir–Polder force are physical forces arising from a quantized field. The typical example is of two uncharged metallic plates in a vacuum, like capacitors placed a few micrometers apart, without any external electromagnetic field...
, proposed in 1948 by Dutch
Netherlands
The Netherlands is a constituent country of the Kingdom of the Netherlands, located mainly in North-West Europe and with several islands in the Caribbean. Mainland Netherlands borders the North Sea to the north and west, Belgium to the south, and Germany to the east, and shares maritime borders...
physicist
Physicist
A physicist is a scientist who studies or practices physics. Physicists study a wide range of physical phenomena in many branches of physics spanning all length scales: from sub-atomic particles of which all ordinary matter is made to the behavior of the material Universe as a whole...
Hendrik B. G. Casimir
Hendrik Casimir
Hendrik Brugt Gerhard Casimir FRS was a Dutch physicist best known for his research on the two-fluid model of superconductors in 1934 and the Casimir effect Hendrik Brugt Gerhard Casimir FRS (July 15, 1909 in The Hague, Netherlands – May 4, 2000 in Heeze) was a Dutch physicist best known...
(Philips Research
Philips Natuurkundig Laboratorium
The Philips Natuurkundig Laboratorium or NatLab was the Dutch section of the Philips research department, which did research for the product divisions of that company. Originally located in the Strijp district of Eindhoven, the facility moved to Waalre in the early 1960s...
), who considered the quantized electromagnetic field
Electromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
between a pair of grounded, neutral metal plates. The vacuum energy contains contributions from all wavelengths, except those excluded by the spacing between plates. As the plates draw together, more wavelengths are excluded and the vacuum energy decreases. The decrease in energy means there must be a force doing work on the plates as they move. This force has been measured and found to be in good agreement with the theory. However, there is still some debate on whether vacuum energy is necessary to explain the Casimir effect. Robert Jaffe
Robert Jaffe
Robert L. Jaffe is an American physicist and the Jane and Otto Morningstar Professor of Physics at the Massachusetts Institute of Technology . He was formerly director of the MIT Center for Theoretical Physics.-Biography:...
of MIT argues that the Casimir force should not be considered evidence for vacuum energy, since it can be derived in QED
Quantum electrodynamics
Quantum electrodynamics is the relativistic quantum field theory of electrodynamics. In essence, it describes how light and matter interact and is the first theory where full agreement between quantum mechanics and special relativity is achieved...
without reference to vacuum energy by considering charge-current interactions (the radiation-reaction picture).
The experimentally measured Lamb shift has been argued to be, in part, a zero-point energy effect.
Gravitation and cosmology
In cosmologyPhysical cosmology
Physical cosmology, as a branch of astronomy, is the study of the largest-scale structures and dynamics of the universe and is concerned with fundamental questions about its formation and evolution. For most of human history, it was a branch of metaphysics and religion...
, the zero-point energy offers an intriguing possibility for explaining the speculative positive values of the proposed cosmological constant
Cosmological constant
In physical cosmology, the cosmological constant was proposed by Albert Einstein as a modification of his original theory of general relativity to achieve a stationary universe...
. In brief, if the energy is "really there", then it should exert a gravitational force. In general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
, mass
Mass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
and energy are equivalent; both produce a gravitational field. One obvious difficulty with this association is that the zero-point energy of the vacuum is absurdly large. Naively, it is infinite, because it includes the energy of waves with arbitrarily short wavelengths. But since only differences in energy are physically measurable, the infinity can be removed by renormalization
Renormalization
In quantum field theory, the statistical mechanics of fields, and the theory of self-similar geometric structures, renormalization is any of a collection of techniques used to treat infinities arising in calculated quantities....
. In all practical calculations, this is how the infinity is handled. It is also arguable that undiscovered physics relevant at the Planck scale
Planck scale
In particle physics and physical cosmology, the Planck scale is an energy scale around 1.22 × 1019 GeV at which quantum effects of gravity become strong...
reduces or eliminates the energy of waves shorter than the Planck length, making the total zero-point energy finite.
Free-energy devices
As a scientific concept, the existence of zero-point energy is not controversial although the ability to harness it is. In particular, perpetual motion machines and other power generating devices supposedly based on zero-point energy are highly controversial and, in many cases, in violation of some of the fundamental laws of physics. No device claimed to operate using zero-point energy has been demonstrated to operate as claimed. No plausible description of a device drawing useful power from a source of zero-point energy has been given. Thus, current claims to zero-point-energy-based power generation systems have the status of pseudosciencePseudoscience
Pseudoscience is a claim, belief, or practice which is presented as scientific, but which does not adhere to a valid scientific method, lacks supporting evidence or plausibility, cannot be reliably tested, or otherwise lacks scientific status...
.
The discovery of zero-point energy did not alter the implausibility of perpetual motion machines. Much attention has been given to reputable science suggesting that zero-point-energy density is infinite, but in quantum theory, zero-point energy is a minimum energy below which a thermodynamic system
Thermodynamic system
A thermodynamic system is a precisely defined macroscopic region of the universe, often called a physical system, that is studied using the principles of thermodynamics....
can never go. Thus according to the standard quantum-theoretic viewpoint, none of this energy can be withdrawn without altering the system to a different form in which the system has a lower zero-point energy.
It is possible that the discovery of new physics will alter this conclusion. For example, in stochastic electrodynamics
Stochastic electrodynamics
In theoretical physics, Stochastic Electrodynamics is a variant of Classical Electrodynamics which posits the existence of a classical Lorentz Invariant radiation field having statistical properties similar to that of the electromagnetic zero-point field of Quantum Electrodynamics...
, the zero-point field is viewed as simply a classical background isotropic noise wave field which excites all systems present in the vacuum and thus is responsible for their minimum-energy or "ground" states. The requirement of Lorentz invariance at a statistical level then implies that the energy density spectrum must increase with the third power of frequency, implying infinite energy density when integrated over all frequencies. If this theory is correct, there is no reason that energy, or for that matter, momentum, could not be extracted, and would of course still leave infinite energy density and infinite momentum density, isotropic in all directions simultaneously, remaining in the wave field.
According to NASA, "the concept of accessing a significant amount of useful energy from the ZPE gained much credibility when a major article on this topic was recently [March 1st, 2004] published in Aviation Week & Space Technology, a leading aerospace industry magazine".
The calculation that underlies the Casimir experiment, a calculation based on the formula predicting infinite vacuum energy, shows the zero-point energy of a system consisting of a vacuum between two plates will decrease at a finite rate as the two plates are drawn together. The vacuum energies are predicted to be infinite, but the changes are predicted to be finite. Casimir combined the projected rate of change in zero-point energy with the principle of conservation of energy to predict a force on the plates. The predicted force, which is very small and was experimentally measured to be within 5% of its predicted value, is finite. Even though the zero-point energy is theoretically infinite, there is as yet no practical evidence to suggest that infinite amounts of zero-point energy are available for use, that zero-point energy can be withdrawn for free, or that zero-point energy can be used in violation of conservation of energy.
External links
- Zero-point energy? "Ask the Van" popular science FAQ at University of IllinoisUniversity of Illinois at Urbana-ChampaignThe University of Illinois at Urbana–Champaign is a large public research-intensive university in the state of Illinois, United States. It is the flagship campus of the University of Illinois system...
. - Philip Yam, "Exploiting Zero-point Energy", Scientific American Magazine, December 1997, pp. 82–85.
- Matt Visser, What is the 'zero-point energy' (or 'vacuum energy') in quantum physics? Is it really possible that we could harness this energy? from Scientific American Magazine, August 18, 1997
- Calphysics Institute "Zero Point Energy and Zero Point Field"