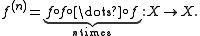Pushforward measure
Encyclopedia
In measure theory, a pushforward measure (also push forward, push-forward or image measure ) is obtained by transferring ("pushing forward") a measure
from one measurable space to another using a measurable function
.
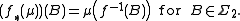
This definition applies mutatis mutandis
for a signed
or complex measure
.
can be pushed forward, the push-forward then becomes a linear operator, known as the transfer operator
or Frobenius–Perron operator. This operator typically satisfies the requirements of the Frobenius–Perron theorem, and the maximal eigenvalue of this theorem corresponds to the invariant measure. The adjoint to the push-forward is the pullback
; as an operator on measurable spaces, it is the composition operator
or Koopman operator.
Measure (mathematics)
In mathematical analysis, a measure on a set is a systematic way to assign to each suitable subset a number, intuitively interpreted as the size of the subset. In this sense, a measure is a generalization of the concepts of length, area, and volume...
from one measurable space to another using a measurable function
Measurable function
In mathematics, particularly in measure theory, measurable functions are structure-preserving functions between measurable spaces; as such, they form a natural context for the theory of integration...
.
Definition
Given measurable spaces (X1, Σ1) and (X2, Σ2), a measurable function f : X1 → X2 and a measure μ : Σ1 → [0, +∞], the pushforward of μ is defined to be the measure f∗(μ) : Σ2 → [0, +∞] given by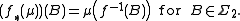
This definition applies mutatis mutandis
Mutatis mutandis
Mutatis mutandis is a Latin phrase meaning "by changing those things which need to be changed" or more simply "the necessary changes having been made"....
for a signed
Signed measure
In mathematics, signed measure is a generalization of the concept of measure by allowing it to have negative values. Some authors may call it a charge, by analogy with electric charge, which is a familiar distribution that takes on positive and negative values.-Definition:There are two slightly...
or complex measure
Complex measure
In mathematics, specifically measure theory, a complex measure generalizes the concept of measure by letting it have complex values. In other words, one allows for sets whose size is a complex number.-Definition:...
.
Examples and applications
- A natural "Lebesgue measureLebesgue measureIn measure theory, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of n-dimensional Euclidean space. For n = 1, 2, or 3, it coincides with the standard measure of length, area, or volume. In general, it is also called...
" on the unit circleUnit circleIn mathematics, a unit circle is a circle with a radius of one. Frequently, especially in trigonometry, "the" unit circle is the circle of radius one centered at the origin in the Cartesian coordinate system in the Euclidean plane...
S1 (here thought of as a subset of the complex planeComplex planeIn mathematics, the complex plane or z-plane is a geometric representation of the complex numbers established by the real axis and the orthogonal imaginary axis...
C) may be defined using a push-forward construction and Lebesgue measure λ on the real lineReal lineIn mathematics, the real line, or real number line is the line whose points are the real numbers. That is, the real line is the set of all real numbers, viewed as a geometric space, namely the Euclidean space of dimension one...
R. Let λ also denote the restriction of Lebesgue measure to the interval [0, 2π) and let f : [0, 2π) → S1 be the natural bijection defined by f(t) = exp(i t). The natural "Lebesgue measure" on S1 is then the push-forward measure f∗(λ). The measure f∗(λ) might also be called "arc lengthArc lengthDetermining the length of an irregular arc segment is also called rectification of a curve. Historically, many methods were used for specific curves...
measure" or "angle measure", since the f∗(λ)-measure of an arc in S1 is precisely its arc length (or, equivalently, the angle that it subtends at the centre of the circle.)
- The previous example extends nicely to give a natural "Lebesgue measure" on the n-dimensional torusTorusIn geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
Tn. The previous example is a special case, since S1 = T1. This Lebesgue measure on Tn is, up to normalization, the Haar measureHaar measureIn mathematical analysis, the Haar measure is a way to assign an "invariant volume" to subsets of locally compact topological groups and subsequently define an integral for functions on those groups....
for the compactCompact spaceIn mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, connectedConnected spaceIn topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint nonempty open subsets. Connectedness is one of the principal topological properties that is used to distinguish topological spaces...
Lie groupLie groupIn mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
Tn.
- Gaussian measureGaussian measureIn mathematics, Gaussian measure is a Borel measure on finite-dimensional Euclidean space Rn, closely related to the normal distribution in statistics. There is also a generalization to infinite-dimensional spaces...
s on infinite-dimensional vector spaces are defined using the push-forward and the standard Gaussian measure on the real line: a Borel measure γ on a separable Banach spaceBanach spaceIn mathematics, Banach spaces is the name for complete normed vector spaces, one of the central objects of study in functional analysis. A complete normed vector space is a vector space V with a norm ||·|| such that every Cauchy sequence in V has a limit in V In mathematics, Banach spaces is the...
X is called Gaussian if the push-forward of γ by any non-zero linear functionalLinear functionalIn linear algebra, a linear functional or linear form is a linear map from a vector space to its field of scalars. In Rn, if vectors are represented as column vectors, then linear functionals are represented as row vectors, and their action on vectors is given by the dot product, or the...
in the continuous dual space to X is a Gaussian measure on R.
- Consider a measurable function f : X → X and the compositionFunction compositionIn mathematics, function composition is the application of one function to the results of another. For instance, the functions and can be composed by computing the output of g when it has an argument of f instead of x...
of f with itself n times:
- This iterated functionIterated functionIn mathematics, an iterated function is a function which is composed with itself, possibly ad infinitum, in a process called iteration. In this process, starting from some initial value, the result of applying a given function is fed again in the function as input, and this process is repeated...
forms a dynamical systemDynamical systemA dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...
. It is often of interest in the study of such systems to find a measure μ on X that the map f leaves unchanged, a so-called invariant measureInvariant measureIn mathematics, an invariant measure is a measure that is preserved by some function. Ergodic theory is the study of invariant measures in dynamical systems...
, one for which f∗(μ) = μ.
- One can also consider quasi-invariant measureQuasi-invariant measureIn mathematics, a quasi-invariant measure μ with respect to a transformation T, from a measure space X to itself, is a measure which, roughly speaking, is multiplied by a numerical function by T...
s for such a dynamical system: a measure μ on X is called quasi-invariant under f if the push-forward of μ by f is merely equivalent to the original measure μ, not necessarily equal to it.
A generalization
In general, any measurable functionMeasurable function
In mathematics, particularly in measure theory, measurable functions are structure-preserving functions between measurable spaces; as such, they form a natural context for the theory of integration...
can be pushed forward, the push-forward then becomes a linear operator, known as the transfer operator
Transfer operator
In mathematics, the transfer operator encodes information about an iterated map and is frequently used to study the behavior of dynamical systems, statistical mechanics, quantum chaos and fractals...
or Frobenius–Perron operator. This operator typically satisfies the requirements of the Frobenius–Perron theorem, and the maximal eigenvalue of this theorem corresponds to the invariant measure. The adjoint to the push-forward is the pullback
Pullback
Suppose that φ:M→ N is a smooth map between smooth manifolds M and N; then there is an associated linear map from the space of 1-forms on N to the space of 1-forms on M. This linear map is known as the pullback , and is frequently denoted by φ*...
; as an operator on measurable spaces, it is the composition operator
Composition operator
In mathematics, the composition operator C_\phi with symbol \phi is a linear operator defined by the ruleC_\phi = f \circ\phiwhere f \circ\phi denotes function composition. In physics, and especially the area of dynamical systems, the composition operator is usually referred to as the Koopman...
or Koopman operator.