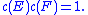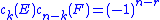Grassmannian
Encyclopedia
In mathematics
, a Grassmannian is a space which parameterizes all linear subspace
s of a vector space
V of a given dimension
. For example, the Grassmannian Gr(1, V) is the space of lines through the origin in V, so it is the same as the projective space
P(V). The Grassmanians are compact
, topological manifold
s. They are named in honor of Hermann Grassmann
.
choices of subspace. Though such concepts may seem strangely out of place they can coincide with things that one is interested in, and can describe ideas that could not be considered otherwise—or at least describe them more economically.
A natural example comes from tangent bundle
s of smooth manifolds embedded in Euclidean space
. Suppose we have a manifold M of dimension r embedded in Rn. At each point x in M, the tangent space to M can be considered as a subspace of the tangent space of Rn, which is just Rr. The map assigning to x its tangent space defines a map from M to Gr(r, n). (In order to do this, we have to translate the geometrical tangent space to M so that it passes through the origin rather than x, and hence defines a r-dimensional vector subspace. This idea is very similar to the Gauss map
for surfaces in a 3-dimensional space.)
This idea can with some effort be extended to all vector bundles over a manifold M, so that every vector bundle generates a continuous map from M to a suitably generalised grassmannian—although various embedding theorems must be proved to show this. We then find that the properties of our vector bundles are related to the properties of the corresponding maps viewed as continuous maps. In particular we find that vector bundles with maps that are homotopic are isomorphic. But the definition of homotopic relies on a notion of continuity, and hence a topology.
, as lines in projective 3-space, and he parameterized the space via Plücker coordinates
. Hermann Grassmann
generalized Plücker's work to general r-planes in n-space.
to it (and vice-versa); hence Gr(2, 3) ≅ Gr(1, 3) ≅ P2
.
k. The Grassmannian Gr(r, V) is the set of all r-dimensional linear subspaces of V. If V has dimension n, then the Grassmannian is also denoted Gr(r, n).
Vector subspaces of V are equivalent to linear subspaces of the projective space P(V), so it is equivalent to think of the Grassmannian as the set of all linear subspaces of P(V). When the Grassmannian is thought of this way, it is often written as Gr(r −1, P(V)) or Gr(r−1, n−1).
. First, recall that the general linear group
GL(V) acts
transitively on the r-dimensional subspaces of V. Therefore, if H is a stabilizer of this action, we have
If the underlying field is R or C and GL(V) is considered as a Lie group
, then this construction makes the Grassmannian into a smooth manifold. It also becomes possible to use other groups to make this construction. To do this, fix an inner product on V. Over R, one replaces GL(V) by the orthogonal group
O(V), and by restricting to orthonormal frames, one gets the identity
In particular, the dimension of the Grassmannian is r(n−r);.
Over C, one replaces GL(V) by the unitary group
U(V). This shows that the Grassmannian is compact. These constructions also make the Grassmannian into a metric space
: For a subspace W of V, let PW be the projection of V onto W. Then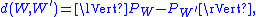
where
denotes the operator norm
, is a metric on Gr(r, V). The exact inner product used does not matter, because a different inner product will give an equivalent norm on V, and so give an equivalent metric.
If the ground field
k is arbitrary and GL(V) is considered as an algebraic group, then this construction shows that the Grassmannian is a non-singular
algebraic variety
. It can be shown that H is a parabolic subgroup, from which it follows that Gr(r, V) is complete
. It follows by the Veronese embedding that the Grassmannian is a projective variety, and more easily it follows from the Plücker embedding
.
, the Grassmannian can be constructed as a scheme
by expressing it as a representable functor
.
Suppose that W is an r-dimensional subspace of V. To define ψ(W), choose a basis w1, ..., wr of W, and let ψ(W) be the wedge product of these basis elements: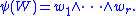
A different basis for W will give a different wedge product, but the two products will differ only by a non-zero scalar (the determinant of the change of basis matrix). Since the right-hand side takes values in a projective space, ψ is well-defined. To see that ψ is an embedding, notice that it is possible to recover W from ψ(W) as the set of all vectors w such that wΛψ(W) = 0.
The embedding of the Grassmannian satisfies some very simple quadratic polynomials called the Plücker relations. These show that the Grassmannian embeds as an algebraic subvariety of P(∧rV) and give another method of constructing the Grassmannian. To state the Plücker relations, choose two r-dimensional subspaces W and Z of V with bases w1, ..., wr and z1, ..., zr, respectively. Then, for any integer k ≥ 0, the following equation is true in the homogeneous coordinate ring
of P(∧rV):
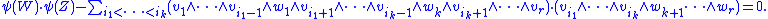
In the case that V has dimension 4, and r=2, the simplest Grassmannian which is not a projective space, the above reduces to a single equation. Denoting the coordinates of P(∧rV) by
, we have that Gr(2, V) is defined by the equation
In general, however, many more equations are needed to define the Plücker embedding of a Grassmannian in projective space.
A(r, n) and Gr(r, Rn) are homeomorphic, with a correspondence established by sending X ∈ A(r, n) to the column space of X.
Taking the dual
to each of these three spaces and linear transformations yields an inclusion of (V/W)* in V* with quotient W*: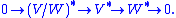
Using the natural isomorphism of a finite-dimensional vector space with its double dual shows that taking the dual again recovers the original short exact sequence. Consequently there is a one-to-one correspondence between r-dimensional subspaces of V and n−r-dimensional subspaces of V*. In terms of the Grassmannian, this is a canonical isomorphism
Choosing an isomorphism of V with V* therefore determines a (non-canonical) isomorphism of Gr(r, V) and Gr(n − r, V). An isomorphism of V with V* is equivalent to a choice of an inner product
, and with respect to the chosen inner product, this isomorphism of Grassmannians sends an r-dimensional subspace into its (n – r)-dimensional orthogonal complement.
s called Schubert cells, which were first applied in enumerative geometry
. The Schubert cells for Gr(r, n) are defined in terms of an auxiliary flag: take subspaces V1, V2, ..., Vr, with Vi contained in Vi + 1. Then we consider the corresponding subset of Gr(r, n), consisting of the W having intersection with Vi of dimension at least i, for i = 1 to r. The manipulation of Schubert cells is Schubert calculus
.
Here is an example of the technique. Consider the problem of determining the Euler characteristic of the Grassmannian of r-dimensional subspaces of Rn. Fix a one-dimensional subspace R ⊂ Rn and consider the partition of Gr(r, n) into those r-dimensional subspaces of Rn that contain R and those that do not. The former is Gr(r − 1, n − 1) and the latter is a r-dimensional vector bundle over Gr(r, n − 1). This gives recursive formulas: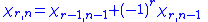
where by design
. If one solves this recurrence relation, you have the formula: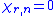 
if and only if n is even and r is odd. Otherwise: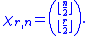
E which generalizes the tautological bundle
of a projective space
. Similarly the (n − r)-dimensional orthogonal complements of these planes yield an orthogonal vector bundle F. The integral cohomology
of the Grassmannians is generated, as a ring
, by the Chern class
es of E. In particular, all of the integral cohomology is at even degree as in the case of a projective space.
These generators are subject to a set of relations, which defines the ring. The relations merely state that the direct sum of the bundles E and F is trivial. Functoriality of the total Chern classes allows one to write this relation as
The quantum cohomology
ring was calculated by Edward Witten
in The Verlinde Algebra And The Cohomology Of The Grassmannian. The generators are identical to those of the classical cohomology ring, but the top relation is changed to
reflecting the existence in the corresponding quantum field theory of an instanton
with 2n fermionic zero-modes which violates the degree of the cohomology corresponding to a state by 2n units.
on the orthogonal group
O(n) and fix V in Gr(r, n). Then for a set A ⊆ Gr(r, n), define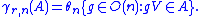
This measure is invariant under actions from the group O(n), that is, γr, n (g A) = γr, n (A) for all g in O(n). Since θn (O(n))=1, we have γr, n (Gr(r, n))=1. Moreover, γr, n is a Radon measure
with respect to the metric space topology and is uniform in the sense that every ball of the same radius (with respect to this metric) is of the same measure.
As a homogeneous space can be expressed as:
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a Grassmannian is a space which parameterizes all linear subspace
Linear subspace
The concept of a linear subspace is important in linear algebra and related fields of mathematics.A linear subspace is usually called simply a subspace when the context serves to distinguish it from other kinds of subspaces....
s of a vector space
Vector space
A vector space is a mathematical structure formed by a collection of vectors: objects that may be added together and multiplied by numbers, called scalars in this context. Scalars are often taken to be real numbers, but one may also consider vector spaces with scalar multiplication by complex...
V of a given dimension
Dimension
In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...
. For example, the Grassmannian Gr(1, V) is the space of lines through the origin in V, so it is the same as the projective space
Projective space
In mathematics a projective space is a set of elements similar to the set P of lines through the origin of a vector space V. The cases when V=R2 or V=R3 are the projective line and the projective plane, respectively....
P(V). The Grassmanians are compact
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, topological manifold
Topological manifold
In mathematics, a topological manifold is a topological space which looks locally like Euclidean space in a sense defined below...
s. They are named in honor of Hermann Grassmann
Hermann Grassmann
Hermann Günther Grassmann was a German polymath, renowned in his day as a linguist and now also admired as a mathematician. He was also a physicist, neohumanist, general scholar, and publisher...
.
Motivation
By giving subspaces a topological structure it is possible to talk about a continuous choice of subspace or open and closed collections of subspaces; by giving them the structure of a differential manifold one can talk about smoothSmooth
Smooth means having a texture that lacks friction. Not rough.Smooth may also refer to:-In mathematics:* Smooth function, a function that is infinitely differentiable; used in calculus and topology...
choices of subspace. Though such concepts may seem strangely out of place they can coincide with things that one is interested in, and can describe ideas that could not be considered otherwise—or at least describe them more economically.
A natural example comes from tangent bundle
Tangent bundle
In differential geometry, the tangent bundle of a differentiable manifold M is the disjoint unionThe disjoint union assures that for any two points x1 and x2 of manifold M the tangent spaces T1 and T2 have no common vector...
s of smooth manifolds embedded in Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
. Suppose we have a manifold M of dimension r embedded in Rn. At each point x in M, the tangent space to M can be considered as a subspace of the tangent space of Rn, which is just Rr. The map assigning to x its tangent space defines a map from M to Gr(r, n). (In order to do this, we have to translate the geometrical tangent space to M so that it passes through the origin rather than x, and hence defines a r-dimensional vector subspace. This idea is very similar to the Gauss map
Gauss map
In differential geometry, the Gauss map maps a surface in Euclidean space R3 to the unit sphere S2. Namely, given a surface X lying in R3, the Gauss map is a continuous map N: X → S2 such that N is a unit vector orthogonal to X at p, namely the normal vector to X at p.The Gauss map can be defined...
for surfaces in a 3-dimensional space.)
This idea can with some effort be extended to all vector bundles over a manifold M, so that every vector bundle generates a continuous map from M to a suitably generalised grassmannian—although various embedding theorems must be proved to show this. We then find that the properties of our vector bundles are related to the properties of the corresponding maps viewed as continuous maps. In particular we find that vector bundles with maps that are homotopic are isomorphic. But the definition of homotopic relies on a notion of continuity, and hence a topology.
History
The simplest Grassmannian that is not a projective space is Gr(2, 4). This was studied by Julius PlückerJulius Plücker
Julius Plücker was a German mathematician and physicist. He made fundamental contributions to the field of analytical geometry and was a pioneer in the investigations of cathode rays that led eventually to the discovery of the electron. He also vastly extended the study of Lamé curves.- Early...
, as lines in projective 3-space, and he parameterized the space via Plücker coordinates
Plücker coordinates
In geometry, Plücker coordinates, introduced by Julius Plücker in the 19th century, are a way to assign six homogenous coordinates to each line in projective 3-space, P3. Because they satisfy a quadratic constraint, they establish a one-to-one correspondence between the 4-dimensional space of lines...
. Hermann Grassmann
Hermann Grassmann
Hermann Günther Grassmann was a German polymath, renowned in his day as a linguist and now also admired as a mathematician. He was also a physicist, neohumanist, general scholar, and publisher...
generalized Plücker's work to general r-planes in n-space.
Low dimensions
When r = 2, the Grassmannian is the space of all planes through the origin. In Euclidean 3-space, a plane is completely characterized by the one and only line perpendicularPerpendicular
In geometry, two lines or planes are considered perpendicular to each other if they form congruent adjacent angles . The term may be used as a noun or adjective...
to it (and vice-versa); hence Gr(2, 3) ≅ Gr(1, 3) ≅ P2
Projective plane
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect...
.
The Grassmannian as a set
Let V be a finite-dimensional vector space over a fieldField (mathematics)
In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
k. The Grassmannian Gr(r, V) is the set of all r-dimensional linear subspaces of V. If V has dimension n, then the Grassmannian is also denoted Gr(r, n).
Vector subspaces of V are equivalent to linear subspaces of the projective space P(V), so it is equivalent to think of the Grassmannian as the set of all linear subspaces of P(V). When the Grassmannian is thought of this way, it is often written as Gr(r −1, P(V)) or Gr(r−1, n−1).
The Grassmannian as a homogeneous space
The quickest way of giving the Grassmannian a geometric structure is to express it as a homogeneous spaceHomogeneous space
In mathematics, particularly in the theories of Lie groups, algebraic groups and topological groups, a homogeneous space for a group G is a non-empty manifold or topological space X on which G acts continuously by symmetry in a transitive way. A special case of this is when the topological group,...
. First, recall that the general linear group
General linear group
In mathematics, the general linear group of degree n is the set of n×n invertible matrices, together with the operation of ordinary matrix multiplication. This forms a group, because the product of two invertible matrices is again invertible, and the inverse of an invertible matrix is invertible...
GL(V) acts
Group action
In algebra and geometry, a group action is a way of describing symmetries of objects using groups. The essential elements of the object are described by a set, and the symmetries of the object are described by the symmetry group of this set, which consists of bijective transformations of the set...
transitively on the r-dimensional subspaces of V. Therefore, if H is a stabilizer of this action, we have
- Gr(r, V) = GL(V)/H.
If the underlying field is R or C and GL(V) is considered as a Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
, then this construction makes the Grassmannian into a smooth manifold. It also becomes possible to use other groups to make this construction. To do this, fix an inner product on V. Over R, one replaces GL(V) by the orthogonal group
Orthogonal group
In mathematics, the orthogonal group of degree n over a field F is the group of n × n orthogonal matrices with entries from F, with the group operation of matrix multiplication...
O(V), and by restricting to orthonormal frames, one gets the identity
- Gr(r, n) = O(n)/(O(r) × O(n − r)).
In particular, the dimension of the Grassmannian is r(n−r);.
Over C, one replaces GL(V) by the unitary group
Unitary group
In mathematics, the unitary group of degree n, denoted U, is the group of n×n unitary matrices, with the group operation that of matrix multiplication. The unitary group is a subgroup of the general linear group GL...
U(V). This shows that the Grassmannian is compact. These constructions also make the Grassmannian into a metric space
Metric space
In mathematics, a metric space is a set where a notion of distance between elements of the set is defined.The metric space which most closely corresponds to our intuitive understanding of space is the 3-dimensional Euclidean space...
: For a subspace W of V, let PW be the projection of V onto W. Then
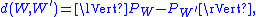
where
denotes the operator normOperator norm
In mathematics, the operator norm is a means to measure the "size" of certain linear operators. Formally, it is a norm defined on the space of bounded linear operators between two given normed vector spaces.- Introduction and definition :...
, is a metric on Gr(r, V). The exact inner product used does not matter, because a different inner product will give an equivalent norm on V, and so give an equivalent metric.
If the ground field
Ground field
In mathematics, a ground field is a field K fixed at the beginning of the discussion. It is used in various areas of algebra: for example in linear algebra where the concept of a vector space may be developed over any field; and in algebraic geometry, where in the foundational developments of André...
k is arbitrary and GL(V) is considered as an algebraic group, then this construction shows that the Grassmannian is a non-singular
Singular point of an algebraic variety
In mathematics, a singular point of an algebraic variety V is a point P that is 'special' , in the geometric sense that V is not locally flat there. In the case of an algebraic curve, a plane curve that has a double point, such as the cubic curveexhibits at , cannot simply be parametrized near the...
algebraic variety
Algebraic variety
In mathematics, an algebraic variety is the set of solutions of a system of polynomial equations. Algebraic varieties are one of the central objects of study in algebraic geometry...
. It can be shown that H is a parabolic subgroup, from which it follows that Gr(r, V) is complete
Complete algebraic variety
In mathematics, in particular in algebraic geometry, a complete algebraic variety is an algebraic variety X, such that for any variety Y the projection morphismis a closed map, i.e. maps closed sets onto closed sets....
. It follows by the Veronese embedding that the Grassmannian is a projective variety, and more easily it follows from the Plücker embedding
Plücker embedding
In mathematics, the Plücker embedding describes a method to realize the Grassmannian of all r-dimensional subspaces of a vector space V as a subvariety of the projective space of the rth exterior power of that vector space, P....
.
The Grassmannian as a scheme
In the realm of algebraic geometryAlgebraic geometry
Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, the Grassmannian can be constructed as a scheme
Scheme (mathematics)
In mathematics, a scheme is an important concept connecting the fields of algebraic geometry, commutative algebra and number theory. Schemes were introduced by Alexander Grothendieck so as to broaden the notion of algebraic variety; some consider schemes to be the basic object of study of modern...
by expressing it as a representable functor
Representable functor
In mathematics, particularly category theory, a representable functor is a functor of a special form from an arbitrary category into the category of sets. Such functors give representations of an abstract category in terms of known structures In mathematics, particularly category theory, a...
.
The Plücker embedding
The Plücker embedding is a natural embedding of a Grassmannian into a projective space:Suppose that W is an r-dimensional subspace of V. To define ψ(W), choose a basis w1, ..., wr of W, and let ψ(W) be the wedge product of these basis elements:
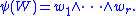
A different basis for W will give a different wedge product, but the two products will differ only by a non-zero scalar (the determinant of the change of basis matrix). Since the right-hand side takes values in a projective space, ψ is well-defined. To see that ψ is an embedding, notice that it is possible to recover W from ψ(W) as the set of all vectors w such that wΛψ(W) = 0.
The embedding of the Grassmannian satisfies some very simple quadratic polynomials called the Plücker relations. These show that the Grassmannian embeds as an algebraic subvariety of P(∧rV) and give another method of constructing the Grassmannian. To state the Plücker relations, choose two r-dimensional subspaces W and Z of V with bases w1, ..., wr and z1, ..., zr, respectively. Then, for any integer k ≥ 0, the following equation is true in the homogeneous coordinate ring
Homogeneous coordinate ring
In algebraic geometry, the homogeneous coordinate ring R of an algebraic variety V given as a subvariety of projective space of a given dimension N is by definition the quotient ring...
of P(∧rV):
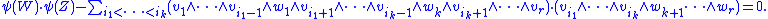
In the case that V has dimension 4, and r=2, the simplest Grassmannian which is not a projective space, the above reduces to a single equation. Denoting the coordinates of P(∧rV) by
, we have that Gr(2, V) is defined by the equation
-
.
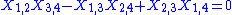In general, however, many more equations are needed to define the Plücker embedding of a Grassmannian in projective space.
The Grassmannian as a real affine algebraic variety
Let Gr(r, Rn) denote the Grassmannian of r-dimensional subspaces of Rn. Let M(n, R) denote the space of real n-by-n matrices. Consider the set of matrices A(r, n) ⊂ M(n, R) defined by X ∈ A (r, n) if and only if the three conditions are satisfied:-
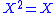(ie: it is a projection operator) -
(it is symmetric) -
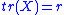(its trace is r)
A(r, n) and Gr(r, Rn) are homeomorphic, with a correspondence established by sending X ∈ A(r, n) to the column space of X.
Duality
Every r-dimensional subspace W of V determines an (n – r)-dimensional quotient space V/W of V. This can be written down quickly as a short exact sequence:Taking the dual
Dual space
In mathematics, any vector space, V, has a corresponding dual vector space consisting of all linear functionals on V. Dual vector spaces defined on finite-dimensional vector spaces can be used for defining tensors which are studied in tensor algebra...
to each of these three spaces and linear transformations yields an inclusion of (V/W)* in V* with quotient W*:
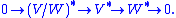
Using the natural isomorphism of a finite-dimensional vector space with its double dual shows that taking the dual again recovers the original short exact sequence. Consequently there is a one-to-one correspondence between r-dimensional subspaces of V and n−r-dimensional subspaces of V*. In terms of the Grassmannian, this is a canonical isomorphism
- Gr(r, V) ≅ Gr(n − r, V*).
Choosing an isomorphism of V with V* therefore determines a (non-canonical) isomorphism of Gr(r, V) and Gr(n − r, V). An isomorphism of V with V* is equivalent to a choice of an inner product
Inner product space
In mathematics, an inner product space is a vector space with an additional structure called an inner product. This additional structure associates each pair of vectors in the space with a scalar quantity known as the inner product of the vectors...
, and with respect to the chosen inner product, this isomorphism of Grassmannians sends an r-dimensional subspace into its (n – r)-dimensional orthogonal complement.
Schubert cells
The detailed study of the Grassmannians uses a decomposition into subsetSubset
In mathematics, especially in set theory, a set A is a subset of a set B if A is "contained" inside B. A and B may coincide. The relationship of one set being a subset of another is called inclusion or sometimes containment...
s called Schubert cells, which were first applied in enumerative geometry
Enumerative geometry
In mathematics, enumerative geometry is the branch of algebraic geometry concerned with counting numbers of solutions to geometric questions, mainly by means of intersection theory.-History:...
. The Schubert cells for Gr(r, n) are defined in terms of an auxiliary flag: take subspaces V1, V2, ..., Vr, with Vi contained in Vi + 1. Then we consider the corresponding subset of Gr(r, n), consisting of the W having intersection with Vi of dimension at least i, for i = 1 to r. The manipulation of Schubert cells is Schubert calculus
Schubert calculus
In mathematics, Schubert calculus is a branch of algebraic geometry introduced in the nineteenth century by Hermann Schubert, in order to solve various counting problems of projective geometry...
.
Here is an example of the technique. Consider the problem of determining the Euler characteristic of the Grassmannian of r-dimensional subspaces of Rn. Fix a one-dimensional subspace R ⊂ Rn and consider the partition of Gr(r, n) into those r-dimensional subspaces of Rn that contain R and those that do not. The former is Gr(r − 1, n − 1) and the latter is a r-dimensional vector bundle over Gr(r, n − 1). This gives recursive formulas:
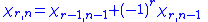
where by design
. If one solves this recurrence relation, you have the formula: 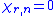if and only if n is even and r is odd. Otherwise: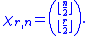Cohomology ring of the complex Grassmannian
Every point in the complex Grassmannian manifold Gr(r, n) defines an r-plane in n-space. Fibering these planes over the Grassmannian one arrives at the vector bundleVector bundle
In mathematics, a vector bundle is a topological construction that makes precise the idea of a family of vector spaces parameterized by another space X : to every point x of the space X we associate a vector space V in such a way that these vector spaces fit together...
E which generalizes the tautological bundle
Tautological bundle
In mathematics, tautological bundle is a term for a particularly natural vector bundle occurring over a Grassmannian, and more specially over projective space...
of a projective space
Projective space
In mathematics a projective space is a set of elements similar to the set P of lines through the origin of a vector space V. The cases when V=R2 or V=R3 are the projective line and the projective plane, respectively....
. Similarly the (n − r)-dimensional orthogonal complements of these planes yield an orthogonal vector bundle F. The integral cohomology
Cohomology
In mathematics, specifically in algebraic topology, cohomology is a general term for a sequence of abelian groups defined from a co-chain complex. That is, cohomology is defined as the abstract study of cochains, cocycles, and coboundaries...
of the Grassmannians is generated, as a ring
Ring (mathematics)
In mathematics, a ring is an algebraic structure consisting of a set together with two binary operations usually called addition and multiplication, where the set is an abelian group under addition and a semigroup under multiplication such that multiplication distributes over addition...
, by the Chern class
Chern class
In mathematics, in particular in algebraic topology and differential geometry, the Chern classes are characteristic classes associated to complex vector bundles.Chern classes were introduced by .-Basic idea and motivation:...
es of E. In particular, all of the integral cohomology is at even degree as in the case of a projective space.
These generators are subject to a set of relations, which defines the ring. The relations merely state that the direct sum of the bundles E and F is trivial. Functoriality of the total Chern classes allows one to write this relation as
The quantum cohomology
Quantum cohomology
In mathematics, specifically in symplectic topology and algebraic geometry, a quantum cohomology ring is an extension of the ordinary cohomology ring of a closed symplectic manifold. It comes in two versions, called small and big; in general, the latter is more complicated and contains more...
ring was calculated by Edward Witten
Edward Witten
Edward Witten is an American theoretical physicist with a focus on mathematical physics who is currently a professor of Mathematical Physics at the Institute for Advanced Study....
in The Verlinde Algebra And The Cohomology Of The Grassmannian. The generators are identical to those of the classical cohomology ring, but the top relation is changed to
reflecting the existence in the corresponding quantum field theory of an instanton
Instanton
An instanton is a notion appearing in theoretical and mathematical physics. Mathematically, a Yang–Mills instanton is a self-dual or anti-self-dual connection in a principal bundle over a four-dimensional Riemannian manifold that plays the role of physical space-time in non-abelian gauge theory...
with 2n fermionic zero-modes which violates the degree of the cohomology corresponding to a state by 2n units.
Associated measure
When V is n-dimensional Euclidean space, one may define a uniform measure on Gr(r, n) in the following way. Let θn be the unit Haar measureHaar measure
In mathematical analysis, the Haar measure is a way to assign an "invariant volume" to subsets of locally compact topological groups and subsequently define an integral for functions on those groups....
on the orthogonal group
Orthogonal group
In mathematics, the orthogonal group of degree n over a field F is the group of n × n orthogonal matrices with entries from F, with the group operation of matrix multiplication...
O(n) and fix V in Gr(r, n). Then for a set A ⊆ Gr(r, n), define
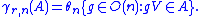
This measure is invariant under actions from the group O(n), that is, γr, n (g A) = γr, n (A) for all g in O(n). Since θn (O(n))=1, we have γr, n (Gr(r, n))=1. Moreover, γr, n is a Radon measure
Radon measure
In mathematics , a Radon measure, named after Johann Radon, is a measure on the σ-algebra of Borel sets of a Hausdorff topological space X that is locally finite and inner regular.-Motivation:...
with respect to the metric space topology and is uniform in the sense that every ball of the same radius (with respect to this metric) is of the same measure.
Oriented Grassmannian
This is the manifold consisting of all oriented r-dimensional subspaces of Rn. It is a double cover of Gr(r, n) and is denoted by:As a homogeneous space can be expressed as:
See also
- For an example of use of Grassmannians in differential geometry, see Gauss mapGauss mapIn differential geometry, the Gauss map maps a surface in Euclidean space R3 to the unit sphere S2. Namely, given a surface X lying in R3, the Gauss map is a continuous map N: X → S2 such that N is a unit vector orthogonal to X at p, namely the normal vector to X at p.The Gauss map can be defined...
and in projective geometryProjective geometryIn mathematics, projective geometry is the study of geometric properties that are invariant under projective transformations. This means that, compared to elementary geometry, projective geometry has a different setting, projective space, and a selective set of basic geometric concepts...
, see Plücker co-ordinates. - Flag manifoldFlag manifoldIn mathematics, a generalized flag variety is a homogeneous space whose points are flags in a finite-dimensional vector space V over a field F. When F is the real or complex numbers, a generalized flag variety is a smooth or complex manifold, called a real or complex flag manifold...
s are generalizations of Grassmannians and Stiefel manifoldStiefel manifoldIn mathematics, the Stiefel manifold Vk is the set of all orthonormal k-frames in Rn. That is, it is the set of ordered k-tuples of orthonormal vectors in Rn. It is named after Swiss mathematician Eduard Stiefel...
s are closely related. - Given a distinguished class of subspaces, one can define Grassmannians of these subspaces, such as the Lagrangian GrassmannianLagrangian GrassmannianIn mathematics, the Lagrangian Grassmannian is the smooth manifold of Lagrangian subspaces of a real symplectic vector space V. Its dimension is n/2 . It may be identified with the homogeneous space...
. - Grassmannians provide classifying spaceClassifying spaceIn mathematics, specifically in homotopy theory, a classifying space BG of a topological group G is the quotient of a weakly contractible space EG by a free action of G...
s in K-theoryK-theoryIn mathematics, K-theory originated as the study of a ring generated by vector bundles over a topological space or scheme. In algebraic topology, it is an extraordinary cohomology theory known as topological K-theory. In algebra and algebraic geometry, it is referred to as algebraic K-theory. It...
, notably the classifying space for U(n)Classifying space for U(n)In mathematics, the classifying space for the unitary group U is a space B together with a universal bundle E such that any hermitian bundle on a paracompact space X is the pull-back of E by a map X → B unique up to homotopy.This space with its universal fibration may be constructed as either# the...
. In the homotopy theory of schemes, the Grassmannian plays a similar rôle for algebraic K-theoryAlgebraic K-theoryIn mathematics, algebraic K-theory is an important part of homological algebra concerned with defining and applying a sequenceof functors from rings to abelian groups, for all integers n....
.