
Mathematical biology
Encyclopedia
Mathematical and theoretical biology is an interdisciplinary scientific research field with a range of applications in biology
, medicine
and biotechnology
. The field may be referred to as mathematical biology or biomathematics to stress the mathematical side, or as theoretical biology to stress the biological side.
It includes at least four major subfields: biological mathematical modeling, relational biology/complex systems biology (CSB), bioinformatics and computational biomodeling/biocomputing.
Mathematical biology aims at the mathematical representation, treatment and modeling of biological
processes, using a variety of applied mathematical
techniques and tools. It has both theoretical and practical applications in biological, biomedical and biotechnology research. For example, in cell biology, protein interactions are often represented as "cartoon" models, which, although easy to visualize, do not accurately describe the systems studied. In order to do this, precise mathematical models are required. By describing the systems in a quantitative manner, their behavior can be better simulated, and hence properties can be predicted that might not be evident to the experimenter.
Such mathematicial areas as calculus
, probability theory
, statistics
, linear algebra
, abstract algebra
, graph theory
, combinatorics
, algebraic geometry
, topology
, dynamical systems, differential equations and coding theory
are now being applied in biology.
theoretical biologists, physicists
, biophysicists, biochemists, bioengineers, engineers, biologists, physiologists, research physicians
, biomedical researchers, oncologists, molecular biologists, geneticists, embryologists
, zoologists
, chemists
, etc.
and evolutionary biology
have traditionally been the dominant fields of mathematical biology.
Evolutionary biology has been the subject of extensive mathematical theorizing. The overall name for this field is population genetics
. Most population genetics considers changes in the frequencies of allele
s at a small number of gene
loci
. When infinitesimal
effects at a large number of gene loci are considered, one derives quantitative genetics
. Ronald Fisher
made fundamental advances in statistics, such as analysis of variance
, via his work on quantitative genetics. Another important branch of population genetics concerns phylogenetics
. Phylogenetics is an area that deals with the reconstruction and analysis of phylogenetic (evolutionary) trees and networks based on inherited characteristics Traditional population genetic models deal with alleles and genotypes, and are frequently stochastic
. In evolutionary game theory
, developed first by John Maynard Smith
, evolutionary biology concepts may take a deterministic mathematical form, with selection acting directly on inherited phenotypes.
Many population genetics models assume that population sizes are constant. Variable population sizes, often in the absence of genetic variation, are treated by the field of population dynamics
. Work in this area dates back to the 19th century, and even as far as 1798 when Thomas Malthus formulated the first principle of population dynamics, which later became known as the Malthusian growth model
. The Lotka–Volterra predator-prey equations are another famous example. Population dynamics overlap with another active area of research in mathematical biology: mathematical epidemiology, the study of infectious disease affecting populations. Various models of the spread of infections have been proposed and analyzed, and provide important results that may be applied to health policy decisions.
in this area up to 1986, including subsections in the following areas: computer modeling in biology and medicine, arterial system models, neuron
models, biochemical and oscillation
networks, quantum automata, quantum computers in molecular biology
and genetics
, cancer modelling, neural nets, genetic networks, abstract categories in relational biology, metabolic-replication systems, category theory
applications in biology and medicine, automata theory
, cellular automata, tessallation models and complete self-reproduction, chaotic systems in organism
s, relational biology and organismic theories. This published report also includes 390 references to peer-reviewed articles by a large number of authors.
Modeling cell and molecular biology
This area has received a boost due to the growing importance of molecular biology
.
Modelling physiological systems
Molecular set theory (MST) is a mathematical formulation of the wide-sense chemical kinetics of biomolecular reactions in terms of sets of molecules and their chemical transformations represented by set-theoretical mappings between molecular sets. In a more general sense, MST is the theory of molecular categories defined as categories of molecular sets and their chemical transformations represented as set-theoretical mappings of molecular sets. The theory has also contributed to biostatistics and the formulation of clinical biochemistry problems in mathematical formulations of pathological, biochemical changes of interest to Physiology, Clinical Biochemistry and Medicine.
, described as the application of mathematics in biophysics, often involving specific physical/mathematical models of biosystems and their components or compartments.
The following is a list of mathematical descriptions and their assumptions.
Deterministic processes (dynamical system
s)
A fixed mapping between an initial state and a final state. Starting from an initial condition and moving forward in time, a deterministic process will always generate the same trajectory and no two trajectories cross in state space.
Stochastic
processes (random dynamical systems)
A random mapping between an initial state and a final state, making the state of the system a random variable
with a corresponding probability distribution
.
Spatial modelling
One classic work in this area is Alan Turing
's paper on morphogenesis
entitled The Chemical Basis of Morphogenesis
, published in 1952 in the Philosophical Transactions of the Royal Society
.
Relational biology
Abstract Relational Biology (ARB) is concerned with the study of general, relational models of complex biological systems, usually abstracting out specific morphological, or anatomical, structures. Some of the simplest models in ARB are the Metabolic-Replication, or (M,R)--systems introduced by Robert Rosen in 1957-1958 as abstract, relational models of cellular and organismal organization.
is very complex and is one of the most studied topics, since its misregulation leads to cancer
s.
It is possibly a good example of a mathematical model as it deals with simple calculus but gives valid results. Two research groups have produced several models of the cell cycle simulating several organisms. They have recently produced a generic eukaryotic cell cycle model which can represent a particular eukaryote depending on the values of the parameters, demonstrating that the idiosyncrasies of the individual cell cycles are due to different protein concentrations and affinities, while the underlying mechanisms are conserved (Csikasz-Nagy et al., 2006).
By means of a system of ordinary differential equation
s these models show the change in time (dynamical system
) of the protein inside a single typical cell; this type of model is called a deterministic process (whereas a model describing a statistical distribution of protein concentrations in a population of cells is called a stochastic process
).
To obtain these equations an iterative series of steps must be done: first the several models and observations are combined to form a consensus diagram and the appropriate kinetic laws are chosen to write the differential equations, such as rate kinetics
for stoichiometric reactions, Michaelis-Menten kinetics
for enzyme substrate reactions and Goldbeter–Koshland kinetics for ultrasensitive transcription factors, afterwards the parameters of the equations (rate constants, enzyme efficiency coefficients and Michealis constants) must be fitted to match observations; when they cannot be fitted the kinetic equation is revised and when that is not possible the wiring diagram is modified. The parameters are fitted and validated using observations of both wild type and mutants, such as protein half-life and cell size.
In order to fit the parameters the differential equations need to be studied. This can be done either by simulation or by analysis.
In a simulation, given a starting vector (list of the values of the variables), the progression of the system is calculated by solving the equations at each time-frame in small increments.
 In analysis, the proprieties of the equations are used to investigate the behavior of the system depending of the values of the parameters and variables. A system of differential equations can be represented as a vector field
In analysis, the proprieties of the equations are used to investigate the behavior of the system depending of the values of the parameters and variables. A system of differential equations can be represented as a vector field
, where each vector described the change (in concentration of two or more protein) determining where and how fast the trajectory (simulation) is heading. Vector fields can have several special points: a stable point
, called a sink, that attracts in all directions (forcing the concentrations to be at a certain value), an unstable point
, either a source or a saddle point
which repels (forcing the concentrations to change away from a certain value), and a limit cycle, a closed trajectory towards which several trajectories spiral towards (making the concentrations oscillate).
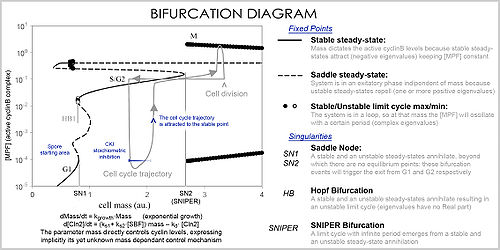
A better representation which can handle the large number of variables and parameters is called a bifurcation diagram
(Bifurcation theory
): the presence of these special steady-state points at certain values of a parameter (e.g. mass) is represented by a point and once the parameter passes a certain value, a qualitative change occurs, called a bifurcation, in which the nature of the space changes, with profound consequences for the protein concentrations: the cell cycle has phases (partially corresponding to G1 and G2) in which mass, via a stable point, controls cyclin levels, and phases (S and M phases) in which the concentrations change independently, but once the phase has changed at a bifurcation event (Cell cycle checkpoint
), the system cannot go back to the previous levels since at the current mass the vector field is profoundly different and the mass cannot be reversed back through the bifurcation event, making a checkpoint irreversible. In particular the S and M checkpoints are regulated by means of special bifurcations called a Hopf bifurcation
and an infinite period bifurcation.
Lists of references
Biology
Biology is a natural science concerned with the study of life and living organisms, including their structure, function, growth, origin, evolution, distribution, and taxonomy. Biology is a vast subject containing many subdivisions, topics, and disciplines...
, medicine
Medicine
Medicine is the science and art of healing. It encompasses a variety of health care practices evolved to maintain and restore health by the prevention and treatment of illness....
and biotechnology
Biotechnology
Biotechnology is a field of applied biology that involves the use of living organisms and bioprocesses in engineering, technology, medicine and other fields requiring bioproducts. Biotechnology also utilizes these products for manufacturing purpose...
. The field may be referred to as mathematical biology or biomathematics to stress the mathematical side, or as theoretical biology to stress the biological side.
It includes at least four major subfields: biological mathematical modeling, relational biology/complex systems biology (CSB), bioinformatics and computational biomodeling/biocomputing.
Mathematical biology aims at the mathematical representation, treatment and modeling of biological
Biology
Biology is a natural science concerned with the study of life and living organisms, including their structure, function, growth, origin, evolution, distribution, and taxonomy. Biology is a vast subject containing many subdivisions, topics, and disciplines...
processes, using a variety of applied mathematical
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
techniques and tools. It has both theoretical and practical applications in biological, biomedical and biotechnology research. For example, in cell biology, protein interactions are often represented as "cartoon" models, which, although easy to visualize, do not accurately describe the systems studied. In order to do this, precise mathematical models are required. By describing the systems in a quantitative manner, their behavior can be better simulated, and hence properties can be predicted that might not be evident to the experimenter.
Such mathematicial areas as calculus
Calculus
Calculus is a branch of mathematics focused on limits, functions, derivatives, integrals, and infinite series. This subject constitutes a major part of modern mathematics education. It has two major branches, differential calculus and integral calculus, which are related by the fundamental theorem...
, probability theory
Probability theory
Probability theory is the branch of mathematics concerned with analysis of random phenomena. The central objects of probability theory are random variables, stochastic processes, and events: mathematical abstractions of non-deterministic events or measured quantities that may either be single...
, statistics
Statistics
Statistics is the study of the collection, organization, analysis, and interpretation of data. It deals with all aspects of this, including the planning of data collection in terms of the design of surveys and experiments....
, linear algebra
Linear algebra
Linear algebra is a branch of mathematics that studies vector spaces, also called linear spaces, along with linear functions that input one vector and output another. Such functions are called linear maps and can be represented by matrices if a basis is given. Thus matrix theory is often...
, abstract algebra
Abstract algebra
Abstract algebra is the subject area of mathematics that studies algebraic structures, such as groups, rings, fields, modules, vector spaces, and algebras...
, graph theory
Graph theory
In mathematics and computer science, graph theory is the study of graphs, mathematical structures used to model pairwise relations between objects from a certain collection. A "graph" in this context refers to a collection of vertices or 'nodes' and a collection of edges that connect pairs of...
, combinatorics
Combinatorics
Combinatorics is a branch of mathematics concerning the study of finite or countable discrete structures. Aspects of combinatorics include counting the structures of a given kind and size , deciding when certain criteria can be met, and constructing and analyzing objects meeting the criteria ,...
, algebraic geometry
Algebraic geometry
Algebraic geometry is a branch of mathematics which combines techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry. It occupies a central place in modern mathematics and has multiple conceptual connections with such diverse fields as complex...
, topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
, dynamical systems, differential equations and coding theory
Coding theory
Coding theory is the study of the properties of codes and their fitness for a specific application. Codes are used for data compression, cryptography, error-correction and more recently also for network coding...
are now being applied in biology.
Importance
Applying mathematics to biology has a long history, but only recently has there been an explosion of interest in the field. Some reasons for this include:- the explosion of data-rich information sets, due to the genomicsGenomicsGenomics is a discipline in genetics concerning the study of the genomes of organisms. The field includes intensive efforts to determine the entire DNA sequence of organisms and fine-scale genetic mapping efforts. The field also includes studies of intragenomic phenomena such as heterosis,...
revolution, which are difficult to understand without the use of analytical tools, - recent development of mathematical tools such as chaos theoryChaos theoryChaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
to help understand complex, nonlinear mechanisms in biology, - an increase in computingComputerA computer is a programmable machine designed to sequentially and automatically carry out a sequence of arithmetic or logical operations. The particular sequence of operations can be changed readily, allowing the computer to solve more than one kind of problem...
power which enables calculations and simulationSimulationSimulation is the imitation of some real thing available, state of affairs, or process. The act of simulating something generally entails representing certain key characteristics or behaviours of a selected physical or abstract system....
s to be performed that were not previously possible, and - an increasing interest in in silicoIn silicoIn silico is an expression used to mean "performed on computer or via computer simulation." The phrase was coined in 1989 as an analogy to the Latin phrases in vivo and in vitro which are commonly used in biology and refer to experiments done in living organisms and outside of living organisms,...
experimentation due to ethical considerations, risk, unreliability and other complications involved in human and animal research.
Areas of research
Several areas of specialized research in mathematical and theoretical biology as well as external links to related projects in various universities are concisely presented in the following subsections, including also a large number of appropriate validating references from a list of several thousands of published authors contributing to this field. Many of the included examples are characterised by highly complex, nonlinear, and supercomplex mechanisms, as it is being increasingly recognised that the result of such interactions may only be understood through a combination of mathematical, logical, physical/chemical, molecular and computational models. Due to the wide diversity of specific knowledge involved, biomathematical research is often done in collaboration between mathematicians, biomathematicians,theoretical biologists, physicists
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, biophysicists, biochemists, bioengineers, engineers, biologists, physiologists, research physicians
Physiology
Physiology is the science of the function of living systems. This includes how organisms, organ systems, organs, cells, and bio-molecules carry out the chemical or physical functions that exist in a living system. The highest honor awarded in physiology is the Nobel Prize in Physiology or...
, biomedical researchers, oncologists, molecular biologists, geneticists, embryologists
Embryology
Embryology is a science which is about the development of an embryo from the fertilization of the ovum to the fetus stage...
, zoologists
Zoology
Zoology |zoölogy]]), is the branch of biology that relates to the animal kingdom, including the structure, embryology, evolution, classification, habits, and distribution of all animals, both living and extinct...
, chemists
Chemistry
Chemistry is the science of matter, especially its chemical reactions, but also its composition, structure and properties. Chemistry is concerned with atoms and their interactions with other atoms, and particularly with the properties of chemical bonds....
, etc.
Evolutionary biology
EcologyEcology
Ecology is the scientific study of the relations that living organisms have with respect to each other and their natural environment. Variables of interest to ecologists include the composition, distribution, amount , number, and changing states of organisms within and among ecosystems...
and evolutionary biology
Evolution
Evolution is any change across successive generations in the heritable characteristics of biological populations. Evolutionary processes give rise to diversity at every level of biological organisation, including species, individual organisms and molecules such as DNA and proteins.Life on Earth...
have traditionally been the dominant fields of mathematical biology.
Evolutionary biology has been the subject of extensive mathematical theorizing. The overall name for this field is population genetics
Population genetics
Population genetics is the study of allele frequency distribution and change under the influence of the four main evolutionary processes: natural selection, genetic drift, mutation and gene flow. It also takes into account the factors of recombination, population subdivision and population...
. Most population genetics considers changes in the frequencies of allele
Allele
An allele is one of two or more forms of a gene or a genetic locus . "Allel" is an abbreviation of allelomorph. Sometimes, different alleles can result in different observable phenotypic traits, such as different pigmentation...
s at a small number of gene
Gene
A gene is a molecular unit of heredity of a living organism. It is a name given to some stretches of DNA and RNA that code for a type of protein or for an RNA chain that has a function in the organism. Living beings depend on genes, as they specify all proteins and functional RNA chains...
loci
Locus (genetics)
In the fields of genetics and genetic computation, a locus is the specific location of a gene or DNA sequence on a chromosome. A variant of the DNA sequence at a given locus is called an allele. The ordered list of loci known for a particular genome is called a genetic map...
. When infinitesimal
Infinitesimal
Infinitesimals have been used to express the idea of objects so small that there is no way to see them or to measure them. The word infinitesimal comes from a 17th century Modern Latin coinage infinitesimus, which originally referred to the "infinite-th" item in a series.In common speech, an...
effects at a large number of gene loci are considered, one derives quantitative genetics
Quantitative genetics
Quantitative genetics is the study of continuous traits and their underlying mechanisms. It is effectively an extension of simple Mendelian inheritance in that the combined effects of one or more genes and the environments in which they are expressed give rise to continuous distributions of...
. Ronald Fisher
Ronald Fisher
Sir Ronald Aylmer Fisher FRS was an English statistician, evolutionary biologist, eugenicist and geneticist. Among other things, Fisher is well known for his contributions to statistics by creating Fisher's exact test and Fisher's equation...
made fundamental advances in statistics, such as analysis of variance
Analysis of variance
In statistics, analysis of variance is a collection of statistical models, and their associated procedures, in which the observed variance in a particular variable is partitioned into components attributable to different sources of variation...
, via his work on quantitative genetics. Another important branch of population genetics concerns phylogenetics
Computational phylogenetics
Computational phylogenetics is the application of computational algorithms, methods and programs to phylogenetic analyses. The goal is to assemble a phylogenetic tree representing a hypothesis about the evolutionary ancestry of a set of genes, species, or other taxa...
. Phylogenetics is an area that deals with the reconstruction and analysis of phylogenetic (evolutionary) trees and networks based on inherited characteristics Traditional population genetic models deal with alleles and genotypes, and are frequently stochastic
Stochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
. In evolutionary game theory
Evolutionary game theory
Evolutionary game theory is the application of Game Theory to evolving populations of lifeforms in biology. EGT is useful in this context by defining a framework of contests, strategies and analytics into which Darwinian competition can be modelled. It originated in 1973 with John Maynard Smith...
, developed first by John Maynard Smith
John Maynard Smith
John Maynard Smith,His surname was Maynard Smith, not Smith, nor was it hyphenated. F.R.S. was a British theoretical evolutionary biologist and geneticist. Originally an aeronautical engineer during the Second World War, he took a second degree in genetics under the well-known biologist J.B.S....
, evolutionary biology concepts may take a deterministic mathematical form, with selection acting directly on inherited phenotypes.
Many population genetics models assume that population sizes are constant. Variable population sizes, often in the absence of genetic variation, are treated by the field of population dynamics
Population dynamics
Population dynamics is the branch of life sciences that studies short-term and long-term changes in the size and age composition of populations, and the biological and environmental processes influencing those changes...
. Work in this area dates back to the 19th century, and even as far as 1798 when Thomas Malthus formulated the first principle of population dynamics, which later became known as the Malthusian growth model
Malthusian growth model
The Malthusian growth model, sometimes called the simple exponential growth model, is essentially exponential growth based on a constant rate of compound interest...
. The Lotka–Volterra predator-prey equations are another famous example. Population dynamics overlap with another active area of research in mathematical biology: mathematical epidemiology, the study of infectious disease affecting populations. Various models of the spread of infections have been proposed and analyzed, and provide important results that may be applied to health policy decisions.
Computer models and automata theory
A monograph on this topic summarizes an extensive amount of published researchin this area up to 1986, including subsections in the following areas: computer modeling in biology and medicine, arterial system models, neuron
Neuron
A neuron is an electrically excitable cell that processes and transmits information by electrical and chemical signaling. Chemical signaling occurs via synapses, specialized connections with other cells. Neurons connect to each other to form networks. Neurons are the core components of the nervous...
models, biochemical and oscillation
Oscillation
Oscillation is the repetitive variation, typically in time, of some measure about a central value or between two or more different states. Familiar examples include a swinging pendulum and AC power. The term vibration is sometimes used more narrowly to mean a mechanical oscillation but sometimes...
networks, quantum automata, quantum computers in molecular biology
Molecular biology
Molecular biology is the branch of biology that deals with the molecular basis of biological activity. This field overlaps with other areas of biology and chemistry, particularly genetics and biochemistry...
and genetics
Genetics
Genetics , a discipline of biology, is the science of genes, heredity, and variation in living organisms....
, cancer modelling, neural nets, genetic networks, abstract categories in relational biology, metabolic-replication systems, category theory
Category theory
Category theory is an area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows , where these collections satisfy certain basic conditions...
applications in biology and medicine, automata theory
Automata theory
In theoretical computer science, automata theory is the study of abstract machines and the computational problems that can be solved using these machines. These abstract machines are called automata...
, cellular automata, tessallation models and complete self-reproduction, chaotic systems in organism
Organism
In biology, an organism is any contiguous living system . In at least some form, all organisms are capable of response to stimuli, reproduction, growth and development, and maintenance of homoeostasis as a stable whole.An organism may either be unicellular or, as in the case of humans, comprise...
s, relational biology and organismic theories. This published report also includes 390 references to peer-reviewed articles by a large number of authors.
Modeling cell and molecular biology
This area has received a boost due to the growing importance of molecular biology
Molecular biology
Molecular biology is the branch of biology that deals with the molecular basis of biological activity. This field overlaps with other areas of biology and chemistry, particularly genetics and biochemistry...
.
- Mechanics of biological tissues
- Theoretical enzymology and enzyme kineticsEnzyme kineticsEnzyme kinetics is the study of the chemical reactions that are catalysed by enzymes. In enzyme kinetics, the reaction rate is measured and the effects of varying the conditions of the reaction investigated...
- CancerCancerCancer , known medically as a malignant neoplasm, is a large group of different diseases, all involving unregulated cell growth. In cancer, cells divide and grow uncontrollably, forming malignant tumors, and invade nearby parts of the body. The cancer may also spread to more distant parts of the...
modelling and simulation - Modelling the movement of interacting cell populations
- Mathematical modelling of scar tissue formation
- Mathematical modelling of intracellular dynamics
- Mathematical modelling of the cell cycle
Modelling physiological systems
- Modelling of arterialArteryArteries are blood vessels that carry blood away from the heart. This blood is normally oxygenated, exceptions made for the pulmonary and umbilical arteries....
disease - Multi-scale modelling of the heartHeartThe heart is a myogenic muscular organ found in all animals with a circulatory system , that is responsible for pumping blood throughout the blood vessels by repeated, rhythmic contractions...
Molecular set theory
Molecular set theory was introduced by Anthony Bartholomay, and its applications were developed in mathematical biology and especially in Mathematical Medicine.Molecular set theory (MST) is a mathematical formulation of the wide-sense chemical kinetics of biomolecular reactions in terms of sets of molecules and their chemical transformations represented by set-theoretical mappings between molecular sets. In a more general sense, MST is the theory of molecular categories defined as categories of molecular sets and their chemical transformations represented as set-theoretical mappings of molecular sets. The theory has also contributed to biostatistics and the formulation of clinical biochemistry problems in mathematical formulations of pathological, biochemical changes of interest to Physiology, Clinical Biochemistry and Medicine.
Mathematical methods
A model of a biological system is converted into a system of equations, although the word 'model' is often used synonymously with the system of corresponding equations. The solution of the equations, by either analytical or numerical means, describes how the biological system behaves either over time or at equilibrium. There are many different types of equations and the type of behavior that can occur is dependent on both the model and the equations used. The model often makes assumptions about the system. The equations may also make assumptions about the nature of what may occur.Mathematical biophysics
The earlier stages of mathematical biology were dominated by mathematical biophysicsBiophysics
Biophysics is an interdisciplinary science that uses the methods of physical science to study biological systems. Studies included under the branches of biophysics span all levels of biological organization, from the molecular scale to whole organisms and ecosystems...
, described as the application of mathematics in biophysics, often involving specific physical/mathematical models of biosystems and their components or compartments.
The following is a list of mathematical descriptions and their assumptions.
Deterministic processes (dynamical system
Dynamical system
A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...
s)
A fixed mapping between an initial state and a final state. Starting from an initial condition and moving forward in time, a deterministic process will always generate the same trajectory and no two trajectories cross in state space.
- Difference equations/Maps – discrete time, continuous state space.
- Ordinary differential equations – continuous time, continuous state space, no spatial derivatives. See also: Numerical ordinary differential equationsNumerical ordinary differential equationsNumerical ordinary differential equations is the part of numerical analysis which studies the numerical solution of ordinary differential equations...
. - Partial differential equations – continuous time, continuous state space, spatial derivatives. See also: Numerical partial differential equationsNumerical partial differential equationsNumerical partial differential equations is the branch of numerical analysis that studies the numerical solution of partial differential equations .Numerical techniques for solving PDEs include the following:...
.
Stochastic
Stochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
processes (random dynamical systems)
A random mapping between an initial state and a final state, making the state of the system a random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
with a corresponding probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
.
- Non-Markovian processes – generalized master equationMaster equationIn physics and chemistry and related fields, master equations are used to describe the time-evolution of a system that can be modelled as being in exactly one of countable number of states at any given time, and where switching between states is treated probabilistically...
– continuous time with memory of past events, discrete state space, waiting times of events (or transitions between states) discretely occur and have a generalized probability distributionProbability distributionIn probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
. - Jump Markov process – master equationMaster equationIn physics and chemistry and related fields, master equations are used to describe the time-evolution of a system that can be modelled as being in exactly one of countable number of states at any given time, and where switching between states is treated probabilistically...
– continuous time with no memory of past events, discrete state space, waiting times between events discretely occur and are exponentially distributed. See also: Monte Carlo methodMonte Carlo methodMonte Carlo methods are a class of computational algorithms that rely on repeated random sampling to compute their results. Monte Carlo methods are often used in computer simulations of physical and mathematical systems...
for numerical simulation methods, specifically dynamic Monte Carlo methodDynamic Monte Carlo methodIn chemistry, dynamic Monte Carlo is a method for modeling the dynamic behaviors of molecules by comparing the rates of individual steps with random numbers...
and Gillespie algorithmGillespie algorithmIn probability theory, the Gillespie algorithm generates a statistically correct trajectory of a stochastic equation. It was created by Joseph L...
. - Continuous Markov processMarkov processIn probability theory and statistics, a Markov process, named after the Russian mathematician Andrey Markov, is a time-varying random phenomenon for which a specific property holds...
– stochastic differential equationStochastic differential equationA stochastic differential equation is a differential equation in which one or more of the terms is a stochastic process, thus resulting in a solution which is itself a stochastic process....
s or a Fokker-Planck equationFokker-Planck equationThe Fokker–Planck equation describes the time evolution of the probability density function of the velocity of a particle, and can be generalized to other observables as well.It is named after Adriaan Fokkerand Max Planck...
– continuous time, continuous state space, events occur continuously according to a random Wiener processWiener processIn mathematics, the Wiener process is a continuous-time stochastic process named in honor of Norbert Wiener. It is often called standard Brownian motion, after Robert Brown...
.
Spatial modelling
One classic work in this area is Alan Turing
Alan Turing
Alan Mathison Turing, OBE, FRS , was an English mathematician, logician, cryptanalyst, and computer scientist. He was highly influential in the development of computer science, providing a formalisation of the concepts of "algorithm" and "computation" with the Turing machine, which played a...
's paper on morphogenesis
Morphogenesis
Morphogenesis , is the biological process that causes an organism to develop its shape...
entitled The Chemical Basis of Morphogenesis
The chemical basis of morphogenesis
The Chemical Basis of Morphogenesis is an article written by Alan Turing in 1951 describing the way in which non-uniformity may arise naturally out of a homogeneous, uniform state....
, published in 1952 in the Philosophical Transactions of the Royal Society
Philosophical Transactions of the Royal Society
The Philosophical Transactions of the Royal Society is a scientific journal published by the Royal Society of London. It was established in 1665, making it the first journal in the world exclusively devoted to science, and it has remained in continuous publication ever since, making it the world's...
.
- Travelling waves in a wound-healing assay
- Swarming behaviour
- A mechanochemical theory of morphogenesisMorphogenesisMorphogenesis , is the biological process that causes an organism to develop its shape...
- Biological pattern formation
- Spatial distribution modeling using plot samples
Relational biology
Abstract Relational Biology (ARB) is concerned with the study of general, relational models of complex biological systems, usually abstracting out specific morphological, or anatomical, structures. Some of the simplest models in ARB are the Metabolic-Replication, or (M,R)--systems introduced by Robert Rosen in 1957-1958 as abstract, relational models of cellular and organismal organization.
Model example: the cell cycle
The eukaryotic cell cycleCell cycle
The cell cycle, or cell-division cycle, is the series of events that takes place in a cell leading to its division and duplication . In cells without a nucleus , the cell cycle occurs via a process termed binary fission...
is very complex and is one of the most studied topics, since its misregulation leads to cancer
Cancer
Cancer , known medically as a malignant neoplasm, is a large group of different diseases, all involving unregulated cell growth. In cancer, cells divide and grow uncontrollably, forming malignant tumors, and invade nearby parts of the body. The cancer may also spread to more distant parts of the...
s.
It is possibly a good example of a mathematical model as it deals with simple calculus but gives valid results. Two research groups have produced several models of the cell cycle simulating several organisms. They have recently produced a generic eukaryotic cell cycle model which can represent a particular eukaryote depending on the values of the parameters, demonstrating that the idiosyncrasies of the individual cell cycles are due to different protein concentrations and affinities, while the underlying mechanisms are conserved (Csikasz-Nagy et al., 2006).
By means of a system of ordinary differential equation
Ordinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
s these models show the change in time (dynamical system
Dynamical system
A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...
) of the protein inside a single typical cell; this type of model is called a deterministic process (whereas a model describing a statistical distribution of protein concentrations in a population of cells is called a stochastic process
Stochastic process
In probability theory, a stochastic process , or sometimes random process, is the counterpart to a deterministic process...
).
To obtain these equations an iterative series of steps must be done: first the several models and observations are combined to form a consensus diagram and the appropriate kinetic laws are chosen to write the differential equations, such as rate kinetics
Reaction rate
The reaction rate or speed of reaction for a reactant or product in a particular reaction is intuitively defined as how fast or slow a reaction takes place...
for stoichiometric reactions, Michaelis-Menten kinetics
Michaelis-Menten kinetics
In biochemistry, Michaelis–Menten kinetics is one of the simplest and best-known models of enzyme kinetics. It is named after German biochemist Leonor Michaelis and Canadian physician Maud Menten. The model takes the form of an equation describing the rate of enzymatic reactions, by relating...
for enzyme substrate reactions and Goldbeter–Koshland kinetics for ultrasensitive transcription factors, afterwards the parameters of the equations (rate constants, enzyme efficiency coefficients and Michealis constants) must be fitted to match observations; when they cannot be fitted the kinetic equation is revised and when that is not possible the wiring diagram is modified. The parameters are fitted and validated using observations of both wild type and mutants, such as protein half-life and cell size.
In order to fit the parameters the differential equations need to be studied. This can be done either by simulation or by analysis.
In a simulation, given a starting vector (list of the values of the variables), the progression of the system is calculated by solving the equations at each time-frame in small increments.

Vector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
, where each vector described the change (in concentration of two or more protein) determining where and how fast the trajectory (simulation) is heading. Vector fields can have several special points: a stable point
Lyapunov stability
Various types of stability may be discussed for the solutions of differential equations describing dynamical systems. The most important type is that concerning the stability of solutions near to a point of equilibrium. This may be discussed by the theory of Lyapunov...
, called a sink, that attracts in all directions (forcing the concentrations to be at a certain value), an unstable point
Lyapunov stability
Various types of stability may be discussed for the solutions of differential equations describing dynamical systems. The most important type is that concerning the stability of solutions near to a point of equilibrium. This may be discussed by the theory of Lyapunov...
, either a source or a saddle point
Saddle point
In mathematics, a saddle point is a point in the domain of a function that is a stationary point but not a local extremum. The name derives from the fact that in two dimensions the surface resembles a saddle that curves up in one direction, and curves down in a different direction...
which repels (forcing the concentrations to change away from a certain value), and a limit cycle, a closed trajectory towards which several trajectories spiral towards (making the concentrations oscillate).
A better representation which can handle the large number of variables and parameters is called a bifurcation diagram
Bifurcation diagram
In mathematics, particularly in dynamical systems, a bifurcation diagram shows the possible long-term values of a system as a function of a bifurcation parameter in the system...
(Bifurcation theory
Bifurcation theory
Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations...
): the presence of these special steady-state points at certain values of a parameter (e.g. mass) is represented by a point and once the parameter passes a certain value, a qualitative change occurs, called a bifurcation, in which the nature of the space changes, with profound consequences for the protein concentrations: the cell cycle has phases (partially corresponding to G1 and G2) in which mass, via a stable point, controls cyclin levels, and phases (S and M phases) in which the concentrations change independently, but once the phase has changed at a bifurcation event (Cell cycle checkpoint
Cell cycle checkpoint
Cell cycle checkpoints are control mechanisms that ensure the fidelity of cell division in eukaryotic cells. These checkpoints verify whether the processes at each phase of the cell cycle have been accurately completed before progression into the next phase...
), the system cannot go back to the previous levels since at the current mass the vector field is profoundly different and the mass cannot be reversed back through the bifurcation event, making a checkpoint irreversible. In particular the S and M checkpoints are regulated by means of special bifurcations called a Hopf bifurcation
Hopf bifurcation
In the mathematical theory of bifurcations, a Hopf or Poincaré–Andronov–Hopf bifurcation, named after Henri Poincaré, Eberhard Hopf, and Aleksandr Andronov, is a local bifurcation in which a fixed point of a dynamical system loses stability as a pair of complex conjugate eigenvalues of...
and an infinite period bifurcation.
Societies and Institutes
- Division of Mathematical Biology at NIMR
- National Institute for Mathematical and Biological SynthesisNational Institute for Mathematical and Biological SynthesisThe National Institute for Mathematical and Biological Synthesis is a research institute focused on the science of mathematics and biology. Known by its acronym NIMBioS , the Institute opened in September 2008, arising from a collaborative agreement between the National Science Foundation, the U.S....
(NIMBioS) - Society for Mathematical BiologySociety for Mathematical BiologyThe Society for Mathematical Biology is an international association co-founded in 1972 in USA by Drs.George Karreman, Herbert Daniel Landahl and by Anthony Bartholomay for the furtherance of joint scientific activities between Mathematics and Biology research communities,...
- European Society for Mathematical and Theoretical Biology
External links
- The Society for Mathematical Biology
- Theoretical and mathematical biology website
- Complexity Discussion Group
- UCLA Biocybernetics Laboratory
- TUCS Computational Biomodelling Laboratory
- Nagoya University Division of Biomodeling
- Technische Universiteit Biomodeling and Informatics
- BioCybernetics Wiki, a vertical wiki on biomedical cybernetics and systems biology
- Bulletin of Mathematical Biology
- European Society for Mathematical and Theoretical Biology
- Journal of Mathematical Biology
- Biomathematics Research Centre at University of Canterbury
- Centre for Mathematical Biology at Oxford University
- Mathematical Biology at the National Institute for Medical Research
- Institute for Medical BioMathematics
- Mathematical Biology Systems of Differential Equations from EqWorld: The World of Mathematical Equations
- Systems Biology Workbench - a set of tools for modelling biochemical networks
- The Collection of Biostatistics Research Archive
- Statistical Applications in Genetics and Molecular Biology
- The International Journal of Biostatistics
- Theoretical Modeling of Cellular Physiology at Ecole Normale Superieure, Paris
Lists of references
- A general list of Theoretical biology/Mathematical biology references, including an updated list of actively contributing authors.
- A list of references for applications of category theory in relational biology.
- An updated list of publications of theoretical biologist Robert Rosen
- Theory of Biological Anthropology (Documents No. 9 and 10 in English)
- Drawing the Line Between Theoretical and Basic Biology (a forum article by Isidro T. Savillo)
Related journals
- Acta Biotheoretica
- Bioinformatics
- Biological Theory
- BioSystems
- Bulletin of Mathematical Biology
- Ecological Modelling
- Journal of Mathematical Biology
- Journal of Theoretical Biology
- Journal of the Royal Society Interface
- Mathematical Biosciences
- Medical Hypotheses
- Rivista di Biologia-Biology Forum
- Theoretical and Applied Genetics
- Theoretical Biology and Medical Modelling
- Theoretical Population Biology
- Theory in Biosciences (formerly: Biologisches Zentralblatt)

