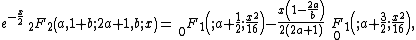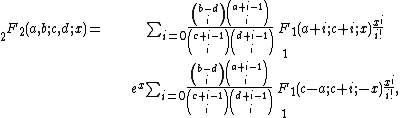Hypergeometric series
Encyclopedia
In mathematics
, a generalized hypergeometric series is a series in which the ratio of successive coefficient
s indexed by n is a rational function
of n. The series, if convergent, defines a generalized hypergeometric function, which may then be defined over a wider domain of the argument by analytic continuation
. The generalized hypergeometric series is sometimes just called the hypergeometric series, though this term also sometimes just refers to the Gaussian hypergeometric series. Generalized hypergeometric functions include the (Gaussian) hypergeometric function and the confluent hypergeometric function
as special cases, which in turn have many particular special functions
as special cases, such as elementary functions, Bessel function
s, and the classical orthogonal polynomials
.
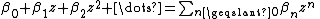
in which the ratio of successive coefficients is a rational function
of n. That is,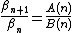
where A(n) and B(n) are polynomial
s in n.
For example, in the case of the series for the exponential function
,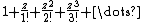 ,
,
βn = n!−1 and βn+1/βn = 1/(n+1). So this satisfies the definition with A(n) = 1 and B(n) = n+1.
It is customary to factor out the leading term, so β0 is assumed to be 1. The polynomials can be factored into linear factors of the form (aj+n) and (bk+n) respectively, where the aj and bk are complex numbers.
For historical reasons, it is assumed that (1+n) is a factor of B. If this is not already the case then both A and B can be multiplied by this factor; the factor cancels so the terms are unchanged and there is no loss of generality.
The ratio between consecutive coefficients now has the form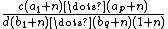 ,
,
where c and d are the leading coefficients of A and B. The series then has the form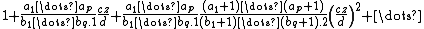 ,
,
or, by scaling z by the appropriate factor and rearranging,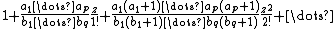 .
.
This has the form of an exponential generating function
. The standard notation for this series is or
or 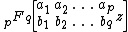
Using the rising factorial or Pochhammer symbol
: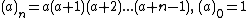 ,
,
this can be written
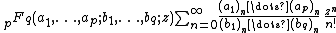
(Note that this use of the Pochhammer symbol is not standard, however it is the standard usage in this context.)
or the hypergeometric function; see the corresponding articles for examples.
Some of the functions related to more complicated hypergeometric functions include:
, then the series defines an analytic function
. Such a function, and its analytic continuation
s, is called the hypergeometric function.
The case when the radius of convergence is 0 yields many interesting series in mathematics, for example the incomplete gamma function
has the asymptotic expansion
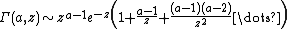
which could be written za−1e−z 2F0(1−a,1;;−z−1). However, the use of the term hypergeometric series is usually restricted to the case where the series defines an actual analytic function.
The ordinary hypergeometric series should not be confused with the basic hypergeometric series
, which, despite its name, is a rather more complicated and recondite series. The "basic" series is the q-analog
of the ordinary hypergeometric series. There are several such generalizations of the ordinary hypergeometric series, including the ones coming from zonal spherical function
s on Riemannian symmetric spaces
.
The series without the factor of n! in the denominator (summed over all integers n, including negative) is called the bilateral hypergeometric series
.
Excluding these cases, the ratio test can be applied to determine the radius of convergence.
The question of convergence for p=q+1 when z is on the unit circle is more difficult. It can be shown that the series converges absolutely at z=1 if .
.
 , or the order of the parameters
, or the order of the parameters 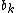 can be changed without changing the value of the function. Also, if any of the parameters
can be changed without changing the value of the function. Also, if any of the parameters  is equal to any of the parameters
is equal to any of the parameters 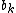 , then the matching parameters can be "cancelled out", with certain exceptions when the parameters are non-positive integers. For example,
, then the matching parameters can be "cancelled out", with certain exceptions when the parameters are non-positive integers. For example,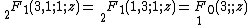 .
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a generalized hypergeometric series is a series in which the ratio of successive coefficient
Coefficient
In mathematics, a coefficient is a multiplicative factor in some term of an expression ; it is usually a number, but in any case does not involve any variables of the expression...
s indexed by n is a rational function
Rational function
In mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
of n. The series, if convergent, defines a generalized hypergeometric function, which may then be defined over a wider domain of the argument by analytic continuation
Analytic continuation
In complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which...
. The generalized hypergeometric series is sometimes just called the hypergeometric series, though this term also sometimes just refers to the Gaussian hypergeometric series. Generalized hypergeometric functions include the (Gaussian) hypergeometric function and the confluent hypergeometric function
Confluent hypergeometric function
In mathematics, a confluent hypergeometric function is a solution of a confluent hypergeometric equation, which is a degenerate form of a hypergeometric differential equation where two of the three regular singularities merge into an irregular singularity...
as special cases, which in turn have many particular special functions
Special functions
Special functions are particular mathematical functions which have more or less established names and notations due to their importance in mathematical analysis, functional analysis, physics, or other applications....
as special cases, such as elementary functions, Bessel function
Bessel function
In mathematics, Bessel functions, first defined by the mathematician Daniel Bernoulli and generalized by Friedrich Bessel, are canonical solutions y of Bessel's differential equation:...
s, and the classical orthogonal polynomials
Orthogonal polynomials
In mathematics, the classical orthogonal polynomials are the most widely used orthogonal polynomials, and consist of the Hermite polynomials, the Laguerre polynomials, the Jacobi polynomials together with their special cases the ultraspherical polynomials, the Chebyshev polynomials, and the...
.
Notation
A hypergeometric series is formally defined as a power series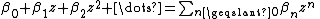
in which the ratio of successive coefficients is a rational function
Rational function
In mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
of n. That is,
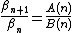
where A(n) and B(n) are polynomial
Polynomial
In mathematics, a polynomial is an expression of finite length constructed from variables and constants, using only the operations of addition, subtraction, multiplication, and non-negative integer exponents...
s in n.
For example, in the case of the series for the exponential function
Exponential function
In mathematics, the exponential function is the function ex, where e is the number such that the function ex is its own derivative. The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change In mathematics,...
,
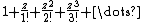 ,
,βn = n!−1 and βn+1/βn = 1/(n+1). So this satisfies the definition with A(n) = 1 and B(n) = n+1.
It is customary to factor out the leading term, so β0 is assumed to be 1. The polynomials can be factored into linear factors of the form (aj+n) and (bk+n) respectively, where the aj and bk are complex numbers.
For historical reasons, it is assumed that (1+n) is a factor of B. If this is not already the case then both A and B can be multiplied by this factor; the factor cancels so the terms are unchanged and there is no loss of generality.
The ratio between consecutive coefficients now has the form
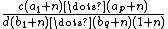 ,
,where c and d are the leading coefficients of A and B. The series then has the form
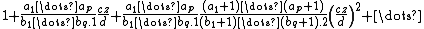 ,
,or, by scaling z by the appropriate factor and rearranging,
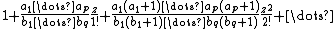 .
.This has the form of an exponential generating function
Generating function
In mathematics, a generating function is a formal power series in one indeterminate, whose coefficients encode information about a sequence of numbers an that is indexed by the natural numbers. Generating functions were first introduced by Abraham de Moivre in 1730, in order to solve the general...
. The standard notation for this series is
 or
or 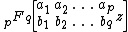
Using the rising factorial or Pochhammer symbol
Pochhammer symbol
In mathematics, the Pochhammer symbol introduced by Leo August Pochhammer is the notation ', where is a non-negative integer. Depending on the context the Pochhammer symbol may represent either the rising factorial or the falling factorial as defined below. Care needs to be taken to check which...
:
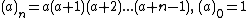 ,
,this can be written
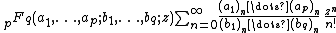
(Note that this use of the Pochhammer symbol is not standard, however it is the standard usage in this context.)
Special cases
Many of the special functions in mathematics are special cases of the confluent hypergeometric functionConfluent hypergeometric function
In mathematics, a confluent hypergeometric function is a solution of a confluent hypergeometric equation, which is a degenerate form of a hypergeometric differential equation where two of the three regular singularities merge into an irregular singularity...
or the hypergeometric function; see the corresponding articles for examples.
Some of the functions related to more complicated hypergeometric functions include:
- Dilogarithm:
-
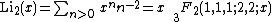
- Hahn polynomialsHahn polynomialsIn mathematics, the Hahn polynomials are a family of orthogonal polynomials in the Askey scheme of hypergeometric orthogonal polynomials, introduced by Chebyshev in 1875 and rediscovered by . The Hahn class is a name for special cases of Hahn polynomials, including Hahn polynomials, Meixner...
:
- Hahn polynomials
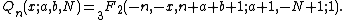
- Wilson polynomialsWilson polynomialsIn mathematics, Wilson polynomials are a family of orthogonal polynomials introduced by that generalize Jacobi polynomials, Hahn polynomials, and Charlier polynomials....
:
Terminology
When all the terms of the series are defined and it has a non-zero radius of convergenceRadius of convergence
In mathematics, the radius of convergence of a power series is a quantity, either a non-negative real number or ∞, that represents a domain in which the series will converge. Within the radius of convergence, a power series converges absolutely and uniformly on compacta as well...
, then the series defines an analytic function
Analytic function
In mathematics, an analytic function is a function that is locally given by a convergent power series. There exist both real analytic functions and complex analytic functions, categories that are similar in some ways, but different in others...
. Such a function, and its analytic continuation
Analytic continuation
In complex analysis, a branch of mathematics, analytic continuation is a technique to extend the domain of a given analytic function. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an infinite series representation in terms of which...
s, is called the hypergeometric function.
The case when the radius of convergence is 0 yields many interesting series in mathematics, for example the incomplete gamma function
Incomplete gamma function
In mathematics, the gamma function is defined by a definite integral. The incomplete gamma function is defined as an integral function of the same integrand. There are two varieties of the incomplete gamma function: the upper incomplete gamma function is for the case that the lower limit of...
has the asymptotic expansion
Asymptotic expansion
In mathematics an asymptotic expansion, asymptotic series or Poincaré expansion is a formal series of functions which has the property that truncating the series after a finite number of terms provides an approximation to a given function as the argument of the function tends towards a particular,...
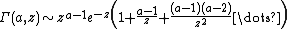
which could be written za−1e−z 2F0(1−a,1;;−z−1). However, the use of the term hypergeometric series is usually restricted to the case where the series defines an actual analytic function.
The ordinary hypergeometric series should not be confused with the basic hypergeometric series
Basic hypergeometric series
In mathematics, Heine's basic hypergeometric series, or hypergeometric q-series, are q-analog generalizations of generalized hypergeometric series, and are in turn generalized by elliptic hypergeometric series....
, which, despite its name, is a rather more complicated and recondite series. The "basic" series is the q-analog
Q-analog
Roughly speaking, in mathematics, specifically in the areas of combinatorics and special functions, a q-analog of a theorem, identity or expression is a generalization involving a new parameter q that returns the original theorem, identity or expression in the limit as q → 1...
of the ordinary hypergeometric series. There are several such generalizations of the ordinary hypergeometric series, including the ones coming from zonal spherical function
Zonal spherical function
In mathematics, a zonal spherical function or often just spherical function is a function on a locally compact group G with compact subgroup K that arises as the matrix coefficient of a K-invariant vector in an irreducible representation of G...
s on Riemannian symmetric spaces
Symmetric space
A symmetric space is, in differential geometry and representation theory, a smooth manifold whose group of symmetries contains an "inversion symmetry" about every point...
.
The series without the factor of n! in the denominator (summed over all integers n, including negative) is called the bilateral hypergeometric series
Bilateral hypergeometric series
In mathematics, a bilateral hypergeometric series is a series Σan summed over all integers n, and such that the ratioof two terms is a rational function of n...
.
Convergence conditions
There are certain values of the aj and bk for which the numerator or the denominator of the coefficients is 0.- If any aj is a non-positive integer (0, −1, −2, etc.) then the series only has a finite number of terms and is, in fact, a polynomial of degree −aj.
- If any bk is a non-positive integer (excepting the previous case with −bk < aj) then the denominators become 0 and the series is undefined.
Excluding these cases, the ratio test can be applied to determine the radius of convergence.
- If p ≤ q then the ratio of coefficients tends to zero. This implies that the series converges for any finite value of z. An example is the power series for the exponential function.
- If p = q+1 then the ratio of coefficients tends to one. This implies that the series converges for |z|<1 and diverges for |z|>1. Analytic continuation can be employed for larger values of z.
- If p > q+1 then the ratio of coefficients grows without bound. This implies that, besides z=0, the series diverges. This is then a divergent or asymptotic series, or it can be interpreted as a symbolic shorthand for a differential equation that the sum satisfies.
The question of convergence for p=q+1 when z is on the unit circle is more difficult. It can be shown that the series converges absolutely at z=1 if
 .
.Basic properties
It is immediate from the definition that the order of the parameters , or the order of the parameters
, or the order of the parameters  can be changed without changing the value of the function. Also, if any of the parameters
can be changed without changing the value of the function. Also, if any of the parameters  is equal to any of the parameters
is equal to any of the parameters 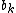 , then the matching parameters can be "cancelled out", with certain exceptions when the parameters are non-positive integers. For example,
, then the matching parameters can be "cancelled out", with certain exceptions when the parameters are non-positive integers. For example,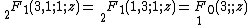 .
.Euler's integral transform
The following basic identity is very useful as it relates the higher-order hypergeometric functions in terms of integrals over the lower order ones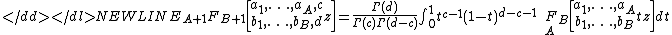
Differentiation
The generalized hypergeometric function satisfies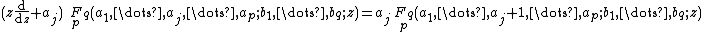 .
.
and .
.
Combining these gives a differential equation satisfied by w = pFq: .
.
Contiguous function and related identities
Let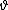 be the operator
be the operator 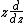 . From the differentiation formulas given above, the linear space spanned by
. From the differentiation formulas given above, the linear space spanned by  and
and 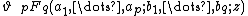 contains each of
contains each of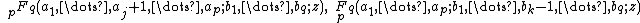 ,
,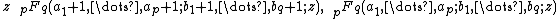 .
.
Since the space has dimension 2, any three of these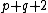 functions are linearly dependent. These dependencies can be written out to generate a large number of identities involving
functions are linearly dependent. These dependencies can be written out to generate a large number of identities involving  .
.
For example, in the simplest non-trivial case,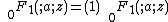 ,
,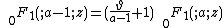 ,
, ,
,
So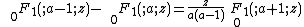 .
.
This, and other important examples,
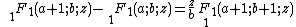 ,
,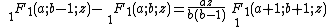 ,
,
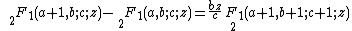 ,
, ,
,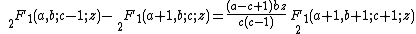 ,
,
can be used to generate continued fractionContinued fractionIn mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer part and another reciprocal, and so on...
expressions known as Gauss's continued fraction.
Similarly, by applying the differentiation formulas twice, there are such functions contained in
such functions contained in 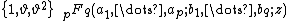 , which has dimension three so any four are linearly dependent. This generates more identities and the process can be continued. The identities thus generated can be combined with each other to produce new ones in a different way.
, which has dimension three so any four are linearly dependent. This generates more identities and the process can be continued. The identities thus generated can be combined with each other to produce new ones in a different way.
A function obtained by adding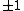 to exactly one of the parameters
to exactly one of the parameters 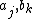 in
in
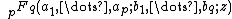 is called contiguous to
is called contiguous to  . Using the technique outlined above, an identity relating
. Using the technique outlined above, an identity relating 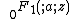 and its two contiguous functions can be given, six identities relating
and its two contiguous functions can be given, six identities relating  and any two of its four contiguous functions, and fifteen identities relating
and any two of its four contiguous functions, and fifteen identities relating  and any two of its six contiguous functions have been found. (The first one was derived in the previous paragraph. The last fifteen were given by Gauss in his 1812 paper.)
and any two of its six contiguous functions have been found. (The first one was derived in the previous paragraph. The last fifteen were given by Gauss in his 1812 paper.)
Identities
A number of other hypergeometric function identities were discovered in the nineteenth and twentieth centuries.
Saalschütz's theorem
Saalschütz's theorem is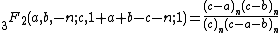
for n a positive integer
, where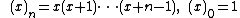
is the Pochhammer symbolPochhammer symbolIn mathematics, the Pochhammer symbol introduced by Leo August Pochhammer is the notation ', where is a non-negative integer. Depending on the context the Pochhammer symbol may represent either the rising factorial or the falling factorial as defined below. Care needs to be taken to check which...
.
Dixon's identity
Dixon's identity, first proved by , gives the sum of a well-poised 3F2 at 1: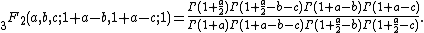
Dougall's formula
Dougall's formula gives the sum of a terminating
well-poised series: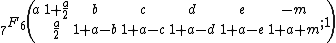
provided that m is a non-negative integer (so that the series terminates) and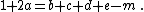
Many of the other formulas for special values of hypergeometric functions can be derived from this as special or limiting cases.
Generalization of Kummer's transformations and identities for
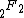
where ;
;
which links Bessel functions to ; this reduces to Kummer's second formula for
; this reduces to Kummer's second formula for 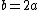 :
:
-
 .
.
which is a finite sum if b-d is a non-negative integer.
Kummer's relation
Kummer's relation is
Clausen's formula
Clausen's formula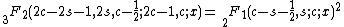
was used by de BrangesLouis de Branges de BourciaLouis de Branges de Bourcia is a French-American mathematician. He is the Edward C. Elliott Distinguished Professor of Mathematics at Purdue University in West Lafayette, Indiana. He is best known for proving the long-standing Bieberbach conjecture in 1984, now called de Branges' theorem...
to prove the Bieberbach conjecture.
The series 0F0
As noted earlier, . The differential equation for this function is
. The differential equation for this function is 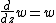 , which has solutions
, which has solutions  where k is a constant.
where k is a constant.
The series 1F0
Also as noted earlier,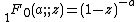 . The differential equation for this function is
. The differential equation for this function is  , or
, or 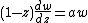 , which has solutions
, which has solutions 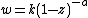 where k is a constant.
where k is a constant.
The series 0F1
The functions of the form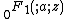 are called confluent hypergeometric limit functions and are closely related to Bessel functions. The relationship is:
are called confluent hypergeometric limit functions and are closely related to Bessel functions. The relationship is: 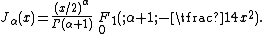 The differential equation for this function is
The differential equation for this function is 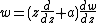 or
or 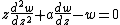 . When a is not a positive integer, the substitution
. When a is not a positive integer, the substitution 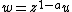 , gives a linearly independent solution
, gives a linearly independent solution 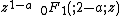 , so the general solution is
, so the general solution is
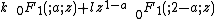 where k, l are constants. (If a is a positive integer, the independent solution is given by the appropriate Bessel function of the second kind.)
where k, l are constants. (If a is a positive integer, the independent solution is given by the appropriate Bessel function of the second kind.)
The series 1F1
The functions of the form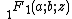 are called confluent hypergeometric functions of the first kind, also written
are called confluent hypergeometric functions of the first kind, also written  . The incomplete gamma function
. The incomplete gamma function  is a special case.
is a special case.
The differential equation for this function is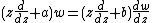 or
or
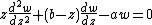 . When b is not a positive integer, the substitution
. When b is not a positive integer, the substitution 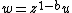 , gives a linearly independent solution
, gives a linearly independent solution 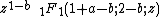 , so the general solution is
, so the general solution is
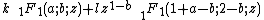 where k, l are constants.
where k, l are constants.
When a is a non-positive integer, −n, is a polynomial. Up to constant factors, these are the
is a polynomial. Up to constant factors, these are the
Laguerre polynomialsLaguerre polynomialsIn mathematics, the Laguerre polynomials, named after Edmond Laguerre ,are the canonical solutions of Laguerre's equation:x\,y + \,y' + n\,y = 0\,which is a second-order linear differential equation....
. This implies Hermite polynomialsHermite polynomialsIn mathematics, the Hermite polynomials are a classical orthogonal polynomial sequence that arise in probability, such as the Edgeworth series; in combinatorics, as an example of an Appell sequence, obeying the umbral calculus; in numerical analysis as Gaussian quadrature; and in physics, where...
can be expressed in terms of as well.
as well.
The series 2F0
This occurs in connection with the exponential integral function Ei(z).
The series 2F1
Historically, the most important are the functions of the form . These are sometimes called Gauss' hypergeometric functions, classical standard hypergeometric or often simply hypergeometric functions. The term Generalized hypergeometric function is used for the functions
. These are sometimes called Gauss' hypergeometric functions, classical standard hypergeometric or often simply hypergeometric functions. The term Generalized hypergeometric function is used for the functions 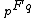 if there is risk of confusion.
if there is risk of confusion.
This function was first studied in detail by Carl Friedrich GaussCarl Friedrich GaussJohann Carl Friedrich Gauss was a German mathematician and scientist who contributed significantly to many fields, including number theory, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy and optics.Sometimes referred to as the Princeps mathematicorum...
, who explored the conditions for its convergence.
The differential equation for this function is

or

It is known as the hypergeometric differential equationHypergeometric differential equationIn mathematics, the Gaussian or ordinary hypergeometric function 2F1 is a special function represented by the hypergeometric series, that includes many other special functions as specific or limiting cases. It is a solution of a second-order linear ordinary differential equation...
. When c is not a positive integer, the substitution

gives a linearly independent solution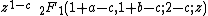 , so the general solution for
, so the general solution for 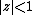 is
is

where k, l are constants. Different solutions can be derived for other values of z. In fact there are 24 solutions, known as the KummerErnst KummerErnst Eduard Kummer was a German mathematician. Skilled in applied mathematics, Kummer trained German army officers in ballistics; afterwards, he taught for 10 years in a gymnasium, the German equivalent of high school, where he inspired the mathematical career of Leopold Kronecker.-Life:Kummer...
solutions, derivable using various identities, valid in different regions of the complex plane.
When a is a non-positive integer, −n,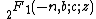 is a polynomial. Up to constant factors and scaling, these are the Jacobi polynomialsJacobi polynomialsIn mathematics, Jacobi polynomials are a class of classical orthogonal polynomials. They are orthogonal with respect to the weight ^\alpha ^\beta on the interval [-1, 1]...
is a polynomial. Up to constant factors and scaling, these are the Jacobi polynomialsJacobi polynomialsIn mathematics, Jacobi polynomials are a class of classical orthogonal polynomials. They are orthogonal with respect to the weight ^\alpha ^\beta on the interval [-1, 1]...
. Several other classes of orthogonal polynomials, up to constant factors, are special cases of Jacobi polynomials, so these can be expressed using as well. This includes Legendre polynomials and Chebyshev polynomials.
as well. This includes Legendre polynomials and Chebyshev polynomials.
A wide range of integrals of elementary functions can be expressed using the hypergeometric function, e.g.: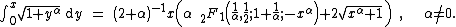
The series 3F1
This occurs in the theory of Bessel functions. It provides a way to compute Bessel functions of large arguments.
Generalizations
The generalized hypergeometric function is linked to the Meijer G-functionMeijer G-FunctionIn mathematics, the G-function was introduced by as a very general function intended to include most of the known special functions as particular cases. This was not the only attempt of its kind: the generalized hypergeometric function and the MacRobert E-function had the same aim, but Meijer's...
and the MacRobert E-function. Hypergeometric series were generalised to several variables, for example by Paul Emile AppellPaul Émile AppellPaul Appell , also known as Paul Émile Appel, was a French mathematician and Rector of the University of Paris...
; but a comparable general theory took long to emerge. Many identities were found, some quite remarkable. A generalization, the q-series analogues, called the basic hypergeometric seriesBasic hypergeometric seriesIn mathematics, Heine's basic hypergeometric series, or hypergeometric q-series, are q-analog generalizations of generalized hypergeometric series, and are in turn generalized by elliptic hypergeometric series....
, were given by Eduard HeineEduard HeineHeinrich Eduard Heine was a German mathematician.Heine became known for results on special functions and in real analysis. In particular, he authored an important treatise on spherical harmonics and Legendre functions . He also investigated basic hypergeometric series...
in the late nineteenth century. Here, the ratios considered of successive terms, instead of a rational function of n, are a rational function of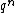 . Another generalization, the elliptic hypergeometric seriesElliptic hypergeometric seriesIn mathematics, an elliptic hypergeometric series is a series Σcn such that the ratiocn/cn−1 is an elliptic function of n, analogous to generalized hypergeometric series where the ratio is a rational function of n, and basic hypergeometric series where the ratio is a periodic function of the...
. Another generalization, the elliptic hypergeometric seriesElliptic hypergeometric seriesIn mathematics, an elliptic hypergeometric series is a series Σcn such that the ratiocn/cn−1 is an elliptic function of n, analogous to generalized hypergeometric series where the ratio is a rational function of n, and basic hypergeometric series where the ratio is a periodic function of the...
, are those series where the ratio of terms is an elliptic functionElliptic functionIn complex analysis, an elliptic function is a function defined on the complex plane that is periodic in two directions and at the same time is meromorphic...
(a doubly periodic meromorphic functionMeromorphic functionIn complex analysis, a meromorphic function on an open subset D of the complex plane is a function that is holomorphic on all D except a set of isolated points, which are poles for the function...
) of n.
During the twentieth century this was a fruitful area of combinatorial mathematics, with numerous connections to other fields. There are a number of new definitions of general hypergeometric functionGeneral hypergeometric functionIn mathematics, a general hypergeometric function or Aomoto–Gelfand hypergeometric function is a generalization of the hypergeometric function that was introduced by...
s, by Aomoto, Israel GelfandIsrael GelfandIsrael Moiseevich Gelfand, also written Israïl Moyseyovich Gel'fand, or Izrail M. Gelfand was a Soviet mathematician who made major contributions to many branches of mathematics, including group theory, representation theory and functional analysis...
and others; and applications for example to the combinatorics of arranging a number of hyperplaneHyperplaneA hyperplane is a concept in geometry. It is a generalization of the plane into a different number of dimensions.A hyperplane of an n-dimensional space is a flat subset with dimension n − 1...
s in complex N-space (see arrangement of hyperplanesArrangement of hyperplanesIn geometry and combinatorics, an arrangement of hyperplanes is a finite set A of hyperplanes in a linear, affine, or projective space S....
).
Special hypergeometric functions occur as zonal spherical functionZonal spherical functionIn mathematics, a zonal spherical function or often just spherical function is a function on a locally compact group G with compact subgroup K that arises as the matrix coefficient of a K-invariant vector in an irreducible representation of G...
s on Riemannian symmetric spacesSymmetric spaceA symmetric space is, in differential geometry and representation theory, a smooth manifold whose group of symmetries contains an "inversion symmetry" about every point...
and semi-simple Lie groupLie groupIn mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
s. Their importance and role can be understood through the following example: the hypergeometric series 2F1 has the Legendre polynomials as a special case, and when considered in the form of spherical harmonicsSpherical harmonicsIn mathematics, spherical harmonics are the angular portion of a set of solutions to Laplace's equation. Represented in a system of spherical coordinates, Laplace's spherical harmonics Y_\ell^m are a specific set of spherical harmonics that forms an orthogonal system, first introduced by Pierre...
, these polynomials reflect, in a certain sense, the symmetry properties of the two-sphere or, equivalently, the rotations given by the Lie group SO(3)Rotation groupIn mechanics and geometry, the rotation group is the group of all rotations about the origin of three-dimensional Euclidean space R3 under the operation of composition. By definition, a rotation about the origin is a linear transformation that preserves length of vectors and preserves orientation ...
. In tensor product decompositions of concrete representations of this group Clebsch-Gordan coefficientsClebsch-Gordan coefficientsIn physics, the Clebsch–Gordan coefficients are sets of numbers that arise in angular momentum coupling under the laws of quantum mechanics.In more mathematical terms, the CG coefficients are used in representation theory, particularly of compact Lie groups, to perform the explicit direct sum...
are met, which can be written as 3F2 hypergeometric series.
Bilateral hypergeometric seriesBilateral hypergeometric seriesIn mathematics, a bilateral hypergeometric series is a series Σan summed over all integers n, and such that the ratioof two terms is a rational function of n...
are a generalization of hypergeometric functions where one sums over all integers, not just the positive ones.
External links
- The book "A = B", this book is freely downloadable from the internet.
- MathWorldMathWorldMathWorld is an online mathematics reference work, created and largely written by Eric W. Weisstein. It is sponsored by and licensed to Wolfram Research, Inc. and was partially funded by the National Science Foundation's National Science Digital Library grant to the University of Illinois at...
-