
Pro-finite group
Encyclopedia
In mathematics
, profinite groups are topological group
s that are in a certain sense assembled from finite group
s; they share many properties with their finite quotients.
, compact
, and totally disconnected topological group
: that is, a topological group that is also a Stone space. Equivalently, one can define a profinite group to be a topological group that is isomorphic
to the inverse limit
of an inverse system
of discrete
finite group
s. In categorical
terms, this is a special case of a (co)filtered limit
construction.
s in G of finite index
(these normal subgroups are partially ordered by inclusion, which translates into an inverse system of natural homomorphisms between the quotients). There is a natural homomorphism η : G → G^, and the image of G under this homomorphism is dense
in G^. The homomorphism η is injective if and only if the group G is residually finite
(i.e.,
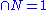 , where the intersection runs through all normal subgroups of finite index).
, where the intersection runs through all normal subgroups of finite index).
The homomorphism η is characterized by the following universal property
: given any profinite group H and any group homomorphism f : G → H, there exists a unique continuous group homomorphism g : G^ → H with f = gη.
to profinite groups; i.e. a group G is ind-finite if it is the direct limit
of an inductive system of finite groups. The usual terminology is different: a group G is called locally finite
if every finitely-generated
subgroup
is finite. This is equivalent, in fact, to being 'ind-finite'.
By applying Pontryagin duality
, one can see that abelian
profinite groups are in duality with locally finite discrete abelian groups. The latter are just the abelian torsion groups.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, profinite groups are topological group
Topological group
In mathematics, a topological group is a group G together with a topology on G such that the group's binary operation and the group's inverse function are continuous functions with respect to the topology. A topological group is a mathematical object with both an algebraic structure and a...
s that are in a certain sense assembled from finite group
Finite group
In mathematics and abstract algebra, a finite group is a group whose underlying set G has finitely many elements. During the twentieth century, mathematicians investigated certain aspects of the theory of finite groups in great depth, especially the local theory of finite groups, and the theory of...
s; they share many properties with their finite quotients.
Definition
Formally, a profinite group is a HausdorffHausdorff space
In topology and related branches of mathematics, a Hausdorff space, separated space or T2 space is a topological space in which distinct points have disjoint neighbourhoods. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" is the most frequently...
, compact
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, and totally disconnected topological group
Topological group
In mathematics, a topological group is a group G together with a topology on G such that the group's binary operation and the group's inverse function are continuous functions with respect to the topology. A topological group is a mathematical object with both an algebraic structure and a...
: that is, a topological group that is also a Stone space. Equivalently, one can define a profinite group to be a topological group that is isomorphic
Isomorphism
In abstract algebra, an isomorphism is a mapping between objects that shows a relationship between two properties or operations. If there exists an isomorphism between two structures, the two structures are said to be isomorphic. In a certain sense, isomorphic structures are...
to the inverse limit
Inverse limit
In mathematics, the inverse limit is a construction which allows one to "glue together" several related objects, the precise manner of the gluing process being specified by morphisms between the objects...
of an inverse system
Inverse system
In mathematics, an inverse system in a category C is a functor from a small cofiltered category I to C. An inverse system is sometimes called a pro-object in C. The dual concept is a direct system.-The category of inverse systems:...
of discrete
Discrete space
In topology, a discrete space is a particularly simple example of a topological space or similar structure, one in which the points are "isolated" from each other in a certain sense.- Definitions :Given a set X:...
finite group
Finite group
In mathematics and abstract algebra, a finite group is a group whose underlying set G has finitely many elements. During the twentieth century, mathematicians investigated certain aspects of the theory of finite groups in great depth, especially the local theory of finite groups, and the theory of...
s. In categorical
Category theory
Category theory is an area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows , where these collections satisfy certain basic conditions...
terms, this is a special case of a (co)filtered limit
Filtered category
In category theory, filtered categories generalize the notion of directed set understood as a category .A category J is filtered when...
construction.
Examples
- Finite groups are profinite, if given the discrete topology.
- The group of p-adic integersP-adic numberIn mathematics, and chiefly number theory, the p-adic number system for any prime number p extends the ordinary arithmetic of the rational numbers in a way different from the extension of the rational number system to the real and complex number systems...
Zp under addition is profinite (in fact procyclic). It is the inverse limit of the finite groups Z/pnZ where n ranges over all natural numbers and the natural maps Z/pnZ → Z/pmZ (n≥m) are used for the limit process. The topology on this profinite group is the same as the topology arising from the p-adic valuation on Zp.
- The Galois theoryGalois theoryIn mathematics, more specifically in abstract algebra, Galois theory, named after Évariste Galois, provides a connection between field theory and group theory...
of field extensionField extensionIn abstract algebra, field extensions are the main object of study in field theory. The general idea is to start with a base field and construct in some manner a larger field which contains the base field and satisfies additional properties...
s of infinite degree gives rise naturally to Galois groups that are profinite. Specifically, if L/K is a Galois extensionGalois extensionIn mathematics, a Galois extension is an algebraic field extension E/F satisfying certain conditions ; one also says that the extension is Galois. The significance of being a Galois extension is that the extension has a Galois group and obeys the fundamental theorem of Galois theory.The definition...
, we consider the group G = Gal(L/K) consisting of all field automorphisms of L which keep all elements of K fixed. This group is the inverse limit of the finite groups Gal(F/K), where F ranges over all intermediate fields such that F/K is a finite Galois extension. For the limit process, we use the restriction homomorphisms Gal(F1/K) → Gal(F2/K), where F2 ⊆ F1. The topology we obtain on Gal(L/K) is known as the Krull topology after Wolfgang KrullWolfgang KrullWolfgang Krull was a German mathematician working in the field of commutative algebra.He was born in Baden-Baden, Imperial Germany and died in Bonn, West Germany.- See also :* Krull dimension* Krull topology...
. Waterhouse showed that every profinite group is isomorphic to one arising from the Galois theory of some field K; but one cannot (yet) control which field K will be in this case. In fact, for many fields K one does not know in general precisely which finite groupFinite groupIn mathematics and abstract algebra, a finite group is a group whose underlying set G has finitely many elements. During the twentieth century, mathematicians investigated certain aspects of the theory of finite groups in great depth, especially the local theory of finite groups, and the theory of...
s occur as Galois groups over K. This is the inverse Galois problemInverse Galois problemIn Galois theory, the inverse Galois problem concerns whether or not every finite group appears as the Galois group of some Galois extension of the rational numbers Q. This problem, first posed in the 19th century, is unsolved....
for a field K. (For some fields K the inverse Galois problem is settled, such as the field of rational functions in one variable over the complex numbers.)
- The fundamental groups considered in algebraic geometryÉtale fundamental groupThe étale fundamental group is an analogue in algebraic geometry, for schemes, of the usual fundamental group of topological spaces.-Topological analogue:In algebraic topology, the fundamental group\pi_1...
are also profinite groups, roughly speaking because the algebra can only 'see' finite coverings of an algebraic varietyAlgebraic varietyIn mathematics, an algebraic variety is the set of solutions of a system of polynomial equations. Algebraic varieties are one of the central objects of study in algebraic geometry...
. The fundamental groupFundamental groupIn mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
s of algebraic topologyAlgebraic topologyAlgebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
, however, are in general not profinite.
Properties and facts
- Every productDirect product of groupsIn the mathematical field of group theory, the direct product is an operation that takes two groups and and constructs a new group, usually denoted...
of (arbitrarily many) profinite groups is profinite; the topology arising from the profiniteness agrees with the product topologyProduct topologyIn topology and related areas of mathematics, a product space is the cartesian product of a family of topological spaces equipped with a natural topology called the product topology...
. The inverse limit of an inverse system of profinite groups with continuous transition maps is profinite and the inverse limit functor is exact on the category of profinite groups. Further, being profinite is an extension property.
- Every closedClosed setIn geometry, topology, and related branches of mathematics, a closed set is a set whose complement is an open set. In a topological space, a closed set can be defined as a set which contains all its limit points...
subgroup of a profinite group is itself profinite; the topology arising from the profiniteness agrees with the subspace topology. If N is a closed normal subgroup of a profinite group G, then the factor group G/N is profinite; the topology arising from the profiniteness agrees with the quotient topology. - Since every profinite group G is compact Hausdorff, we have a Haar measureHaar measureIn mathematical analysis, the Haar measure is a way to assign an "invariant volume" to subsets of locally compact topological groups and subsequently define an integral for functions on those groups....
on G, which allows us to measure the "size" of subsets of G, compute certain probabilities, and integrate functions on G. - A subgroup of a profinite group is open if and only if it is closed and has finite indexIndex of a subgroupIn mathematics, specifically group theory, the index of a subgroup H in a group G is the "relative size" of H in G: equivalently, the number of "copies" of H that fill up G. For example, if H has index 2 in G, then intuitively "half" of the elements of G lie in H...
. - According to a theorem of Nikolay NikolovNikolay Nikolov (mathematician)Nikolay Nikolov is a Bulgarian mathematician working in abstract algebra and currently a Reader at Imperial College London.He obtained his PhD in 2002 under the supervision of Dan Segal.In 2007, he was awarded the LMS Whitehead Prize....
and Dan SegalDan SegalDaniel Segal is a British mathematician, currently a Professor of Mathematics at the University of Oxford. He specialises in algebra and group theory....
, in any topologically finitely-generated profinite group (that is, a profinite group that has a denseDense setIn topology and related areas of mathematics, a subset A of a topological space X is called dense if any point x in X belongs to A or is a limit point of A...
finitely-generated subgroup) the subgroups of finite index are open. This generalizes an earlier analogous result of Jean-Pierre SerreJean-Pierre SerreJean-Pierre Serre is a French mathematician. He has made contributions in the fields of algebraic geometry, number theory, and topology.-Early years:...
for topologically finitely-generated pro-p groupPro-p groupIn mathematics, a pro-p group is a profinite group G such that for any open normal subgroup N\triangleleft G the quotient group G/N is a p-group...
s. The proof uses the classification of finite simple groupsClassification of finite simple groupsIn mathematics, the classification of the finite simple groups is a theorem stating that every finite simple group belongs to one of four categories described below. These groups can be seen as the basic building blocks of all finite groups, in much the same way as the prime numbers are the basic...
. - As an easy corollary of the Nikolov-Segal result above, any surjective discrete group homomorphism φ: G → H between profinite groups G and H is continuous as long as G is topologically finitely-generated. Indeed, any open subgroup of H is of finite index, so its preimage in G is also of finite index, hence it must be open.
- Suppose G and H are topologically finitely-generated profinite groups which are isomorphic as discrete groups by an isomorphism ι. Then ι is bijective and continuous by the above result. Furthermore, ι−1 is also continuous, so ι is a homeomorphism. Therefore the topology on a topologically finitely-generated profinite group is uniquely determined by its algebraic structure.
Profinite completion
Given an arbitrary group G, there is a related profinite group G^, the profinite completion of G. It is defined as the inverse limit of the groups G/N, where N runs through the normal subgroupNormal subgroup
In abstract algebra, a normal subgroup is a subgroup which is invariant under conjugation by members of the group. Normal subgroups can be used to construct quotient groups from a given group....
s in G of finite index
Subgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
(these normal subgroups are partially ordered by inclusion, which translates into an inverse system of natural homomorphisms between the quotients). There is a natural homomorphism η : G → G^, and the image of G under this homomorphism is dense
Dense set
In topology and related areas of mathematics, a subset A of a topological space X is called dense if any point x in X belongs to A or is a limit point of A...
in G^. The homomorphism η is injective if and only if the group G is residually finite
Residually finite group
In the mathematical field of group theory, a group G is residually finite or finitely approximable if for every nontrivial element g in G there is a homomorphism h from G to a finite group, such thath \neq 1.\,...
(i.e.,
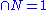 , where the intersection runs through all normal subgroups of finite index).
, where the intersection runs through all normal subgroups of finite index).The homomorphism η is characterized by the following universal property
Universal property
In various branches of mathematics, a useful construction is often viewed as the “most efficient solution” to a certain problem. The definition of a universal property uses the language of category theory to make this notion precise and to study it abstractly.This article gives a general treatment...
: given any profinite group H and any group homomorphism f : G → H, there exists a unique continuous group homomorphism g : G^ → H with f = gη.
Ind-finite groups
There is a notion of ind-finite group, which is the concept dualDual (category theory)
In category theory, a branch of mathematics, duality is a correspondence between properties of a category C and so-called dual properties of the opposite category Cop...
to profinite groups; i.e. a group G is ind-finite if it is the direct limit
Direct limit
In mathematics, a direct limit is a colimit of a "directed family of objects". We will first give the definition for algebraic structures like groups and modules, and then the general definition which can be used in any category.- Algebraic objects :In this section objects are understood to be...
of an inductive system of finite groups. The usual terminology is different: a group G is called locally finite
Locally finite group
In mathematics, in the field of group theory, a locally finite group is a type of group that can be studied in ways analogous to a finite group. Sylow subgroups, Carter subgroups, and abelian subgroups of locally finite groups have been studied....
if every finitely-generated
Generating set of a group
In abstract algebra, a generating set of a group is a subset that is not contained in any proper subgroup of the group. Equivalently, a generating set of a group is a subset such that every element of the group can be expressed as the combination of finitely many elements of the subset and their...
subgroup
Subgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
is finite. This is equivalent, in fact, to being 'ind-finite'.
By applying Pontryagin duality
Pontryagin duality
In mathematics, specifically in harmonic analysis and the theory of topological groups, Pontryagin duality explains the general properties of the Fourier transform on locally compact groups, such as R, the circle or finite cyclic groups.-Introduction:...
, one can see that abelian
Abelian group
In abstract algebra, an abelian group, also called a commutative group, is a group in which the result of applying the group operation to two group elements does not depend on their order . Abelian groups generalize the arithmetic of addition of integers...
profinite groups are in duality with locally finite discrete abelian groups. The latter are just the abelian torsion groups.

