
Filtered category
Encyclopedia
In category theory
, filtered categories generalize the notion of directed set
understood as a category (hence called a directed category; while some use directed category as a synonym for a filtered category).
A category
 is filtered when
is filtered when
A diagram is said to be of cardinality if the morphism set of its domain is of cardinality
if the morphism set of its domain is of cardinality  . A category
. A category  is filtered if and only if there is a cone over any finite diagram
is filtered if and only if there is a cone over any finite diagram  ; more generally, for a regular cardinal
; more generally, for a regular cardinal  , a category
, a category  is said to be
is said to be  -filtered if for every diagram
-filtered if for every diagram  in
in  of cardinality smaller than
of cardinality smaller than  there is a cone over
there is a cone over  .
.
A filtered colimit is a colimit of a functor
 where
where  is a filtered category. This readily generalizes to
is a filtered category. This readily generalizes to  -filtered limits. An ind-object in a category
-filtered limits. An ind-object in a category  is a presheaf of sets
is a presheaf of sets 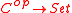 which is a small filtered colimit of representable presheaves. Ind-objects in a category
which is a small filtered colimit of representable presheaves. Ind-objects in a category  form a full subcategory
form a full subcategory 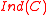 in the category of functors
in the category of functors 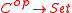 . The category
. The category  of pro-objects in
of pro-objects in  is the opposite of the category of ind-objects in the opposite category
is the opposite of the category of ind-objects in the opposite category 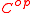 .
.
 is cofiltered if the opposite category
is cofiltered if the opposite category
 is filtered. In detail, a category is cofiltered when
is filtered. In detail, a category is cofiltered when
A cofiltered limit is a limit
of a functor
 where
where  is a cofiltered category.
is a cofiltered category.
Category theory
Category theory is an area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows , where these collections satisfy certain basic conditions...
, filtered categories generalize the notion of directed set
Directed set
In mathematics, a directed set is a nonempty set A together with a reflexive and transitive binary relation ≤ , with the additional property that every pair of elements has an upper bound: In other words, for any a and b in A there must exist a c in A with a ≤ c and b ≤...
understood as a category (hence called a directed category; while some use directed category as a synonym for a filtered category).
A category
Category (mathematics)
In mathematics, a category is an algebraic structure that comprises "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose...
 is filtered when
is filtered when
- it is not empty,
- for every two objects
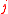 and
and 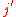 in
in  there exists an object
there exists an object  and two arrows
and two arrows  and
and  in
in  ,
, - for every two parallel arrows
 in
in  , there exists an object
, there exists an object  and an arrow
and an arrow  such that
such that  .
.
A diagram is said to be of cardinality
 if the morphism set of its domain is of cardinality
if the morphism set of its domain is of cardinality  . A category
. A category  is filtered if and only if there is a cone over any finite diagram
is filtered if and only if there is a cone over any finite diagram  ; more generally, for a regular cardinal
; more generally, for a regular cardinal  , a category
, a category  is said to be
is said to be  -filtered if for every diagram
-filtered if for every diagram  in
in  of cardinality smaller than
of cardinality smaller than  there is a cone over
there is a cone over  .
.A filtered colimit is a colimit of a functor
Functor
In category theory, a branch of mathematics, a functor is a special type of mapping between categories. Functors can be thought of as homomorphisms between categories, or morphisms when in the category of small categories....
 where
where  is a filtered category. This readily generalizes to
is a filtered category. This readily generalizes to  -filtered limits. An ind-object in a category
-filtered limits. An ind-object in a category  is a presheaf of sets
is a presheaf of sets 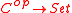 which is a small filtered colimit of representable presheaves. Ind-objects in a category
which is a small filtered colimit of representable presheaves. Ind-objects in a category  form a full subcategory
form a full subcategory 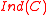 in the category of functors
in the category of functors 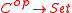 . The category
. The category  of pro-objects in
of pro-objects in  is the opposite of the category of ind-objects in the opposite category
is the opposite of the category of ind-objects in the opposite category 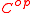 .
.Cofiltered categories
There is a dual notion of cofiltered category. A category is cofiltered if the opposite category
is cofiltered if the opposite categoryOpposite category
In category theory, a branch of mathematics, the opposite category or dual category Cop of a given category C is formed by reversing the morphisms, i.e. interchanging the source and target of each morphism. Doing the reversal twice yields the original category, so the opposite of an opposite...
 is filtered. In detail, a category is cofiltered when
is filtered. In detail, a category is cofiltered when
- it is not empty
- for every two objects
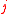 and
and 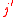 in
in  there exists an object
there exists an object  and two arrows
and two arrows  and
and 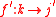 in
in  ,
, - for every two parallel arrows
 in
in  , there exists an object
, there exists an object  and an arrow
and an arrow  such that
such that  .
.
A cofiltered limit is a limit
Limit (category theory)
In category theory, a branch of mathematics, the abstract notion of a limit captures the essential properties of universal constructions such as products and inverse limits....
of a functor
Functor
In category theory, a branch of mathematics, a functor is a special type of mapping between categories. Functors can be thought of as homomorphisms between categories, or morphisms when in the category of small categories....
 where
where  is a cofiltered category.
is a cofiltered category.

