Slope stability analysis - AbsoluteAstronomy.com

The slope stability
Slope stability
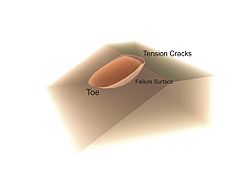
The field of slope stability encompasses the analysis of static and dynamic stability of slopes of earth and rock-fill dams, slopes of other types of embankments, excavated slopes, and natural slopes in soil and soft rock...

analyses
Engineering analysis
Engineering analysis involves the application of scientific analytic principles and processes to reveal the properties and state of the system, device or mechanism under study...

are performed to assess the safe and economic design of a human-made or natural slope

Slope

In mathematics, the slope or gradient of a line describes its steepness, incline, or grade. A higher slope value indicates a steeper incline....

s (e.g. embankments

Embankment (transportation)

To keep a road or railway line straight or flat, and where the comparative cost or practicality of alternate solutions is prohibitive, the land over which the road or rail line will travel is built up to form an embankment. An embankment is therefore in some sense the opposite of a cutting, and...

, road cuts, open-pit mining

Open-pit mining

Open-pit mining or opencast mining refers to a method of extracting rock or minerals from the earth by their removal from an open pit or borrow....

, excavations, landfill

Landfill

A landfill site , is a site for the disposal of waste materials by burial and is the oldest form of waste treatment...

s etc.) and the equilibrium conditions. The term slope stability may be defined as the resistance of inclined surface to failure

Failure

Failure refers to the state or condition of not meeting a desirable or intended objective, and may be viewed as the opposite of success. Product failure ranges from failure to sell the product to fracture of the product, in the worst cases leading to personal injury, the province of forensic...

by sliding

Landslide

A landslide or landslip is a geological phenomenon which includes a wide range of ground movement, such as rockfalls, deep failure of slopes and shallow debris flows, which can occur in offshore, coastal and onshore environments...

or collapsing. The main objectives of slope stability analysis are finding endangered areas, investigation of potential failure mechanisms, determination of the slope sensitivity to different triggering mechanisms, designing of optimal slopes with regard to safety

Safety

Safety is the state of being "safe" , the condition of being protected against physical, social, spiritual, financial, political, emotional, occupational, psychological, educational or other types or consequences of failure, damage, error, accidents, harm or any other event which could be...

, reliability

Reliability engineering

Reliability engineering is an engineering field, that deals with the study, evaluation, and life-cycle management of reliability: the ability of a system or component to perform its required functions under stated conditions for a specified period of time. It is often measured as a probability of...

and economics

Economics

Economics is the social science that analyzes the production, distribution, and consumption of goods and services. The term economics comes from the Ancient Greek from + , hence "rules of the house"...

, designing possible remedial measures, e.g. barriers and stabilization

Stabilization (architecture)

Stabilization is the retrofitting of platforms or foundations as constructed for the purpose of improving the bearing capacity and levelness of the supported building....

.

Successful design

Design

Design as a noun informally refers to a plan or convention for the construction of an object or a system while “to design” refers to making this plan...

of the slope requires geological information and site characteristics, e.g. properties of soil

Soil

Soil is a natural body consisting of layers of mineral constituents of variable thicknesses, which differ from the parent materials in their morphological, physical, chemical, and mineralogical characteristics...

/rock mass, slope geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, groundwater

Groundwater

Groundwater is water located beneath the ground surface in soil pore spaces and in the fractures of rock formations. A unit of rock or an unconsolidated deposit is called an aquifer when it can yield a usable quantity of water. The depth at which soil pore spaces or fractures and voids in rock...

conditions, alternation of materials by faulting, joint or discontinuity systems, movements and tension in joints, earthquake activity etc. Choice of correct analysis technique depends on both site conditions and the potential mode of failure, with careful consideration being given to the varying strengths

Strength of materials

In materials science, the strength of a material is its ability to withstand an applied stress without failure. The applied stress may be tensile, compressive, or shear. Strength of materials is a subject which deals with loads, deformations and the forces acting on a material. A load applied to a...

, weaknesses and limitations inherent in each methodology

Methodology

Methodology is generally a guideline for solving a problem, with specificcomponents such as phases, tasks, methods, techniques and tools . It can be defined also as follows:...

.

Before the computer age stability analysis was performed graphically or using hand-held calculator. Today engineer

Engineer

An engineer is a professional practitioner of engineering, concerned with applying scientific knowledge, mathematics and ingenuity to develop solutions for technical problems. Engineers design materials, structures, machines and systems while considering the limitations imposed by practicality,...

s have a lot of possibilities to use analysis software, ranges from simple limit equilibrium techniques through computational limit analysis approaches (e.g. Finite element limit analysis

Finite element limit analysis

A finite element limit analysis utilises optimisation techniques to directly compute the upper or lower bound plastic collapse load for a mechanical system rather than time stepping to a collapse load, as might be undertaken with conventional non-linear finite element techniques...

, Discontinuity layout optimization

Discontinuity layout optimization

Discontinuity layout optimization is an engineering analysis procedure which can be used to directly establish the amount of load that can be carried by a solid or structure prior to collapse...

) to complex and sophisticated numerical solutions
Numerical analysis
Numerical analysis is the study of algorithms that use numerical approximation for the problems of mathematical analysis ....

(finite-

Finite element method

The finite element method is a numerical technique for finding approximate solutions of partial differential equations as well as integral equations...

/distinct

Discrete element method

A discrete element method , also called a distinct element method is any of family of numerical methods for computing the motion of a large number of particles of micrometre-scale size and above...

-element codes). The engineer must fully understand limitations of each technique. For example, limit equilibrium is most commonly used and simple solution method, but it can become inadequate if the slope fails by complex mechanisms (e.g. internal deformation

Deformation (mechanics)

Deformation in continuum mechanics is the transformation of a body from a reference configuration to a current configuration. A configuration is a set containing the positions of all particles of the body...

and brittle fracture, progressive creep

Creep (deformation)

In materials science, creep is the tendency of a solid material to slowly move or deform permanently under the influence of stresses. It occurs as a result of long term exposure to high levels of stress that are below the yield strength of the material....

, liquefaction

Soil liquefaction

Soil liquefaction describes a phenomenon whereby a saturated soil substantially loses strength and stiffness in response to an applied stress, usually earthquake shaking or other sudden change in stress condition, causing it to behave like a liquid....

of weaker soil layers, etc.). In these cases more sophisticated numerical modelling

Numerical analysis

Numerical analysis is the study of algorithms that use numerical approximation for the problems of mathematical analysis ....

techniques should be utilised. In addition, the use of the risk assessment

Risk assessment

Risk assessment is a step in a risk management procedure. Risk assessment is the determination of quantitative or qualitative value of risk related to a concrete situation and a recognized threat...

concept is increasing today. Risk assessment is concerned with both the consequence of slope failure and the probability

Probability

Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...

of failure (both require an understanding of the failure mechanism).

Within the last decade (2003) Slope Stability Radar

Slope stability radar

The Slope Stability Radar is a new application for the monitoring of slope stability at open-cut mines. It is a system currently in use across the global mining and civil industries....

has been developed to remotely scan a rock slope to monitor the spatial deformation of the face. Small movements of a rough wall can be detected with sub-millimeter accuracy by using interferometry techniques.

Conventional methods of analysis

Most of the slope stability analysis computer program

Computer program

A computer program is a sequence of instructions written to perform a specified task with a computer. A computer requires programs to function, typically executing the program's instructions in a central processor. The program has an executable form that the computer can use directly to execute...

s are based on the limit equilibrium concept for a two- or three-dimensional

Three-dimensional space

Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...

model. In rock slope stability analysis conventional methods can be divided into three groups: kinematic

Kinematics

Kinematics is the branch of classical mechanics that describes the motion of bodies and systems without consideration of the forces that cause the motion....

analysis, limit equilibrium and rock

Rock (geology)

In geology, rock or stone is a naturally occurring solid aggregate of minerals and/or mineraloids.The Earth's outer solid layer, the lithosphere, is made of rock. In general rocks are of three types, namely, igneous, sedimentary, and metamorphic...

fall simulators.

Limit equilibrium analysis

The conventional limit equilibrium methods investigate the equilibrium of the soil mass tending to slide down under the influence of gravity. Transitional or rotational movement is considered on assumed or known potential slip surface below soil or rock

Rock (geology)

mass. In rock slope engineering, methods may be highly significant to simple block failure along distinct discontinuities. All methods are based on comparison of force

Force

In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...

s (moments

Torque

Torque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....

or stresses) resisting instability

Instability

In numerous fields of study, the component of instability within a system is generally characterized by some of the outputs or internal states growing without bounds...

of the mass and those that causing instability

Instability

In numerous fields of study, the component of instability within a system is generally characterized by some of the outputs or internal states growing without bounds...

(disturbing forces). Two-dimensional sections are analyzed assuming plain strain conditions. These methods assume that the shear strength

Shear strength

Shear strength in engineering is a term used to describe the strength of a material or component against the type of yield or structural failure where the material or component fails in shear. A shear load is a force that tends to produce a sliding failure on a material along a plane that is...

s of the materials along the potential failure surface are governed by linear (Mohr-Coulomb
Mohr-Coulomb theory
Mohr–Coulomb theory is a mathematical model describing the response of brittle materials such as concrete, or rubble piles, to shear stress as well as normal stress. Most of the classical engineering materials somehow follow this rule in at least a portion of their shear failure envelope...

) or non-linear relationships between shear strength and the normal stress on the failure surface. analysis provides a factor of safety

Factor of safety

Factor of safety , also known as safety factor , is a term describing the structural capacity of a system beyond the expected loads or actual loads. Essentially, how much stronger the system is than it usually needs to be for an intended load...

, defined as a ratio

Ratio

In mathematics, a ratio is a relationship between two numbers of the same kind , usually expressed as "a to b" or a:b, sometimes expressed arithmetically as a dimensionless quotient of the two which explicitly indicates how many times the first number contains the second In mathematics, a ratio is...

of available shear resistance (capacity) to that required for equilibrium. If the value of factor of safety is less than 1.0, slope is unstable. The most common limit equilibrium techniques are methods of slices where soil mass is discretized into vertical slices (Fig. 2). Results (factor of safety) of particular methods can vary because methods differs in assumption

Assumption

In logic an assumption is a proposition that is taken for granted, as if it were true based upon presupposition without preponderance of the facts...

s and satisfied equilibrium conditions.
Functional slope design considers calculation

Calculation

A calculation is a deliberate process for transforming one or more inputs into one or more results, with variable change.The term is used in a variety of senses, from the very definite arithmetical calculation of using an algorithm to the vague heuristics of calculating a strategy in a competition...

with the critical slip surface where is the lowest value of factor of safety. Locating failure surface can be made with the help of computer program

Computer program

s using search optimization

Optimization (mathematics)

In mathematics, computational science, or management science, mathematical optimization refers to the selection of a best element from some set of available alternatives....

techniques. Wide variety of slope stability software using limit equilibrium concept is available including search of critical slip surface. The program

Program (machine)

A program is a list of instructions written in a programming language that is used to control the behavior of a machine, often a computer ....

analyses the stability of generally layered soil slopes, mainly embankments, earth cuts and anchored sheeting structures

Architectural structure

An architectural structure is a free-standing, immobile outdoor constructed element. The structure may be temporary or permanent.Structures include buildings and nonbuilding structures . Examples of building structures include houses, town halls, libraries, and skyscrapers...

. Fast optimization of circular

Circle

A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....

and polygonal slip surfaces provides the lowest factor of safety. Earthquake effects, external loading

Structural load

Structural loads or actions are forces, deformations or accelerations applied to a structure or its components.Loads cause stresses, deformations and displacements in structures. Assessment of their effects is carried out by the methods of structural analysis...

, groundwater conditions, stabilization

Stabilization (architecture)

Stabilization is the retrofitting of platforms or foundations as constructed for the purpose of improving the bearing capacity and levelness of the supported building....

forces (i.e. anchors, georeinforcements etc.) can be also included. The software uses solution according to various methods of slices (Fig. 2), such as Bishop simplified, Ordinary method of slices (Swedish circle method/Petterson/Fellenius), Spencer, Sarma
Sarma method
The Sarma method is a method used primarily to assess the stability of soil slopes under seismic conditions. Using appropriate assumptions the method can also be employed for static slope stability analysis. It was proposed by Sarada K...

etc.

Sarma
Sarma method
The Sarma method is a method used primarily to assess the stability of soil slopes under seismic conditions. Using appropriate assumptions the method can also be employed for static slope stability analysis. It was proposed by Sarada K...

and Spencer are called as rigorous methods because they satisfy all three conditions of equilibrium: force equilibrium in horizontal and vertical direction and moment equilibrium condition. Rigorous methods can provide more accurate

ACCURATE

ACCURATE was established in 2005 by a group of computer scientists, psychologists and policy experts to address problems with electronic voting...

results than non-rigorous methods. Bishop simplified or Fellenius are non-rigorous methods satisfying only some of the equilibrium conditions and making some simplifying assumption

Assumption

In logic an assumption is a proposition that is taken for granted, as if it were true based upon presupposition without preponderance of the facts...

s.

Another limit equilibrium program SLIDE provides 2D stability calculations in rocks or soils using these rigorous analysis methods: Spencer, Morgenstern-Price/General limit equilibrium; and non-rigorous methods: Bishop simplified, Corps of Engineers, Janbu simplified/corrected, Lowe-Karafiath and Ordinary/Fellenius. Searching of the critical slip surface is realized with the help of a grid or as a slope search in user-defined area. Program includes also probabilistic analysis using Monte Carlo
Monte Carlo method
Monte Carlo methods are a class of computational algorithms that rely on repeated random sampling to compute their results. Monte Carlo methods are often used in computer simulations of physical and mathematical systems...

or Latin Hypercube simulation
Latin hypercube sampling
Latin hypercube sampling is a statistical method for generating a distribution of plausible collections of parameter values from a multidimensional distribution. The sampling method is often applied in uncertainty analysis....

techniques where any input parameter

Parameter

Parameter from Ancient Greek παρά also “para” meaning “beside, subsidiary” and μέτρον also “metron” meaning “measure”, can be interpreted in mathematics, logic, linguistics, environmental science and other disciplines....

can be defined as a random variable

Random variable

In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...

. Probabilistic analysis determine the probability of failure and reliability

Reliability engineering

index, which gives better representation of the level

Level

Level or levels may refer to:-Places:*Levél, Győr-Moson-Sopron, Hungary*Level, Ohio, United States*Level Valley*Levels, West Virginia-Engineering-related:*Floor, or storey, of a building or a mine...

of safety. Back analysis serves for calculation of a reinforcement load

Structural load

with a given required factor of safety. Program enables finite element groundwater seepage analysis.

Program

Program (machine)

A program is a list of instructions written in a programming language that is used to control the behavior of a machine, often a computer ....

SLOPE/W is formulated in terms of moment and force equilibrium factor of safety equations. Limit equilibrium methods include Morgenstern-Price, General limit equilibrium, Spencer, Bishop, Ordinary, Janbu etc. This program allows integration

Enterprise application integration

Enterprise Application Integration is defined as the use of software and computer systems architectural principles to integrate a set of enterprise computer applications.- Overview :...

with other applications. For example finite element computed stresses from SIGMA/W or QUAKE/W can be used to calculate a stability factor by computing total shear resistance and mobilized shear stress

Shear stress

A shear stress, denoted \tau\, , is defined as the component of stress coplanar with a material cross section. Shear stress arises from the force vector component parallel to the cross section...

along the entire slip surface. Then a local stability factor for each slice is obtained. Using a Monte Carlo
Monte Carlo
Monte Carlo is an administrative area of the Principality of Monaco....

approach, program computes the probability of failure in addition to the conventional factor of safety.

STABL WV is a limit equilibrium-based, Windows software based on the stabl family of algorithms. It allows analysis using Bishop's, Spencer's and Janbu's method. Regular slopes as well as slopes with various types of inclusions may be analyzed.

SVSlope is formulated in terms of moment and force equilibrium factor of safety equations. Limit equilibrium methods include Morgenstern-Price, General limit equilibrium, Spencer, Bishop, Ordinary, Kulhawy and others This program allows integration with other applications in the geotechnical software suite. For example finite element computed stresses from SVSolid or pore-water pressures from SVFlux can be used to calculate the factor of safety by computing total shear resistance and mobilized shear stress

Shear stress

A shear stress, denoted \tau\, , is defined as the component of stress coplanar with a material cross section. Shear stress arises from the force vector component parallel to the cross section...

along the entire slip surface. The software also utilizes Monte Carlo
Monte Carlo
Monte Carlo is an administrative area of the Principality of Monaco....

,Latin Hypercube, and the APEM probabilistic approaches. Spatial variability through random fields computations may also be included in the analysis.

Some other programs based on limit equilibrium concept:

GALENA - includes stability analysis, back analysis, and probability analysis, using the Bishop, Spencer-Wright and Sarma methods.
GSLOPE - provides limit equilibrium slope stability analysis of existing natural slopes, unreinforced man-made slopes, or slopes with soil reinforcement, using Bishop’s Modified method and Janbu’s Simplified method applied to circular, composite or non-circular surfaces.
CLARA-W - three-dimensional
Three-dimensional space
Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...

slope stability program includes calculation with the help of these methods: Bishop simplified, Janbu simplified, Spencer and Morgenstern-Price. Problem configurations can involve rotational or non-rotational sliding surfaces, ellipsoids, wedges, compound surfaces, fully specified surfaces and searches.
TSLOPE3 - two- or three-dimensional
Three-dimensional space
Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...

analyses of soil and rock slopes using Spencer method.

Rock slope stability analysis based on limit equilibrium techniques may consider following modes of failure:

Planar failure -> case of rock mass sliding on a single surface (special case of general wedge type of failure); two-dimensional analysis may be used according to the concept of a block resisting on an inclined plane at limit equilibrium
Polygonal failure -> sliding of a nature rock usually takes place on polygonally-shaped surfaces; calculation is based on a certain assumption
Assumption
In logic an assumption is a proposition that is taken for granted, as if it were true based upon presupposition without preponderance of the facts...

s (e.g. sliding on a polygonal surface which is composed from N parts is kinematically possible only in case of development at least (N - 1) internal shear surfaces; rock mass is divided into blocks by internal shear surfaces; blocks are considered to be rigid; no tensile strength is permitted etc.)
Wedge failure -> three-dimensional
Three-dimensional space
Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...

analysis enables modelling of the wedge sliding on two planes in a direction along the line of intersection
Toppling failure -> long thin rock columns formed by the steeply dipping discontinuities may rotate about a pivot point located at the lowest corner of the block; the sum of the moments causing toppling of a block (i.e. horizontal weight component of the block and the sum of the driving forces from adjacent blocks behind the block under consideration) is compared to the sum of the moments resisting toppling (i.e. vertical weight component of the block and the sum of the resisting forces from adjacent blocks in front of the block under consideration); toppling occur if driving moments exceed resisting moments

Stereographic and kinematic analysis

Kinematic analysis examines which modes of failure can possibly occur in the rock mass. Analysis requires the detailed evaluation of rock mass structure and the geometry of existing discontinuities contributing to block instability

Instability

In numerous fields of study, the component of instability within a system is generally characterized by some of the outputs or internal states growing without bounds...

. Stereographic

Stereographic projection

The stereographic projection, in geometry, is a particular mapping that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it...

representation (stereonets) of the planes and lines is used. Stereonets are useful for analyzing discontinuous rock blocks. Program DIPS allows for visualization structural data using stereonets, determination of the kinematic feasibility of rock mass and statistical analysis of the discontinuity properties.

Rockfall simulators

Rock slope stability analysis may design protective measures near or around structures endangered by the falling blocks. Rockfall

Rockfall

Rockfall or rock-fall refers to quantities of rock falling freely from a cliff face. A rockfall is a fragment of rock detached by sliding, toppling, or falling, that falls along a vertical or sub-vertical cliff, proceeds down slope by bouncing and flying along ballistic trajectories or by rolling...

simulators determine travel paths and trajectories of unstable blocks separated from a rock slope face. Analytical solution method described by Hungr & Evans assumes rock block as a point with mass and velocity

Velocity

In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...

moving on a ballistic trajectory with regard to potential contact with slope surface. Calculation requires two restitution coefficients that depend on fragment shape, slope surface roughness, momentum and deformational properties and on the chance of certain conditions in a given impact.

Program ROCFALL provides a statistical analysis of trajectory of falling blocks. Method rely on velocity

Velocity

changes as a rock blocks roll, slide or bounce on various materials. Energy

Energy

In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...

, velocity]], bounce height and location of rock endpoints are determined and may be analyzed statistically. The program can assist in determining remedial measures by computing kinetic energy

Kinetic energy

The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...

and location of impact on a barrier. This can help determine the capacity, size and location of barriers.

Rock mass classification

Various rock mass classification

Rock mass classification

Rock mass classification systems are used for various engineering design and stability analysis. These are based on empirical relations between rock mass parameters and engineering applications, such as tunnels, slopes, foundations, and excavatability...

systems exist for the design of slopes and to assess the stability of slopes. The systems are based on empirical relations between rock mass parameters and various slope parameters such as height and slope dip.

Numerical methods of analysis

Numerical modelling techniques provide an approximate solution to problems which otherwise cannot be solved by conventional methods, e.g. complex geometry, material anisotropy

Anisotropy

Anisotropy is the property of being directionally dependent, as opposed to isotropy, which implies identical properties in all directions. It can be defined as a difference, when measured along different axes, in a material's physical or mechanical properties An example of anisotropy is the light...

, non-linear behaviour, in situ stresses. Numerical analysis

Numerical analysis

Numerical analysis is the study of algorithms that use numerical approximation for the problems of mathematical analysis ....

allows for material deformation

Deformation (mechanics)

and failure, modelling of pore pressures, creep deformation

Creep (deformation)

, dynamic loading, assessing effects of parameter variations etc. However, numerical modelling is restricted by some limitations. For example, input parameters are not usually measured and availability of these data is generally poor. Analysis must be executed by well trained user with good modelling practise. User also should be aware of boundary effects, meshing errors, hardware memory and time restrictions. Numerical methods used for slope stability analysis can be divided into three main groups: continuum

Continuum mechanics

Continuum mechanics is a branch of mechanics that deals with the analysis of the kinematics and the mechanical behavior of materials modelled as a continuous mass rather than as discrete particles...

, discontinuum and hybrid modelling.

Continuum modelling

Modelling of the continuum

Continuum mechanics

Continuum mechanics is a branch of mechanics that deals with the analysis of the kinematics and the mechanical behavior of materials modelled as a continuous mass rather than as discrete particles...

is suitable for the analysis of soil slopes, massive intact rock or heavily jointed rock masses. This approach includes the finite-difference
Finite difference method
In mathematics, finite-difference methods are numerical methods for approximating the solutions to differential equations using finite difference equations to approximate derivatives.- Derivation from Taylor's polynomial :...

and finite element methods that discretize

Discretization

In mathematics, discretization concerns the process of transferring continuous models and equations into discrete counterparts. This process is usually carried out as a first step toward making them suitable for numerical evaluation and implementation on digital computers...

the whole mass to finite number of elements with the help of generated mesh (Fig. 3). In finite-difference
Finite difference method
In mathematics, finite-difference methods are numerical methods for approximating the solutions to differential equations using finite difference equations to approximate derivatives.- Derivation from Taylor's polynomial :...

method (FDM) differential

Differential equation

A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...

equilibrium equations (i.e. strain-displacement and stress-strain relations) are solved. finite element method (FEM) uses the approximations to the connectivity of elements, continuity of displacements

Displacement field (mechanics)

A displacement field is an assignment of displacement vectors for all points in a region or body that is displaced from one state to another. A displacement vector specifies the position of a point or a particle in reference to an origin or to a previous position...

and stresses between elements. Most of numerical codes allows modelling of discrete fracture

Fracture

A fracture is the separation of an object or material into two, or more, pieces under the action of stress.The word fracture is often applied to bones of living creatures , or to crystals or crystalline materials, such as gemstones or metal...

s, e.g. bedding planes, faults. Several constitutive models are usually available, e.g. elasticity

Elasticity (physics)

In physics, elasticity is the physical property of a material that returns to its original shape after the stress that made it deform or distort is removed. The relative amount of deformation is called the strain....

, elasto-plasticity, strain-softening, elasto-viscoplasticity etc.

Discontinuum modelling

Discontinuum approach is useful for rock slopes controlled by discontinuity behaviour. Rock mass is considered as an aggregation of distinct, interacting blocks subjected to external loads and assumed to undergo motion with time. This methodology is collectively called the discrete-element
Discrete element method
A discrete element method , also called a distinct element method is any of family of numerical methods for computing the motion of a large number of particles of micrometre-scale size and above...

method (DEM). Discontinuum modelling allows for sliding between the blocks or particles. The DEM is based on solution of dynamic equation of equilibrium for each block repeatedly until the boundary conditions and laws of contact and motion

Newton's laws of motion

Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...

are satisfied. Discontinuum modelling belongs to the most commonly applied numerical approach to rock slope analysis and following variations of the DEM exist:

distinct-element method
discontinuous deformation analysis
Discontinuous Deformation Analysis
Discontinuous Deformation Analysis is a type of discrete element method originally proposed by Shi in 1988. DDA is somewhat similar to the finite element method for solving stress-displacement problems, but accounts for the interaction of independent particles along discontinuities in fractured...

(DDA)
particle flow codes

The distinct-element
Discrete element method
A discrete element method , also called a distinct element method is any of family of numerical methods for computing the motion of a large number of particles of micrometre-scale size and above...

approach describes mechanical behaviour of both, the discontinuities and the solid material. This methodology is based on a force-displacement law (specifying the interaction between the deformable rock blocks) and a law of motion

Newton's laws of motion

Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...

(determining displacements caused in the blocks by out-of-balance forces). Joints are treated as [boundary conditions. Deformable blocks are discretized into internal constant-strain elements.

Discontinuum program UDEC (Universal distinct element code) is suitable for high jointed rock slopes subjected to static or dynamic loading. Two-dimensional analysis of translational failure mechanism allows for simulating large displacements, modelling deformation or material yielding. Three-dimensional discontinuum code 3DEC contains modelling of multiple intersecting discontinuities and therefore it is suitable for analysis of wedge instabilities or influence of rock support (e.g. rockbolts, cables).

In discontinuous deformation analysis
Discontinuous Deformation Analysis
Discontinuous Deformation Analysis is a type of discrete element method originally proposed by Shi in 1988. DDA is somewhat similar to the finite element method for solving stress-displacement problems, but accounts for the interaction of independent particles along discontinuities in fractured...

(DDA) displacements are unknowns and equilibrium equations are then solved analogous to finite element method. Each unit of finite element type mesh represents an isolated block bounded by discontinuities. Advantage of this methodology is possibility to model large deformations, rigid body movements, coupling or failure states between rock blocks.

Discontinuous rock mass can be modelled with the help of distinct-element
Discrete element method
A discrete element method , also called a distinct element method is any of family of numerical methods for computing the motion of a large number of particles of micrometre-scale size and above...

methodology in the form of particle flow code, e.g.
program PFC2D/3D. Spherical particles interact through frictional sliding contacts. Simulation of joint bounded blocks may be realized through specified bond strengths. Law of motion is repeatedly applied to each particle and force-displacement law to each contact. Particle flow methodology enables modelling of granular flow, fracture of intact rock, transitional block movements, dynamic response to blasting or seismicity, deformation between particles caused by shear or tensile forces. These codes also allow to model subsequent failure processes of rock slope, e.g. simulation of rock

Hybrid/coupled modelling

Hybrid codes involve the coupling of various methodologies to maximize their key advantages, e.g. limit equilibrium analysis combined with finite element groundwater flow and stress analysis adopted in the SVOFFICE or GEO-STUDIO suites of software; coupled particle flow and finite-difference
Finite difference method
In mathematics, finite-difference methods are numerical methods for approximating the solutions to differential equations using finite difference equations to approximate derivatives.- Derivation from Taylor's polynomial :...

analyses used in PF3D and FLAC3D. Hybrid techniques allows investigation of piping slope failures and the influence of high groundwater pressures on the failure of weak rock slope. Coupled finite-/distinct-element
Discrete element method
A discrete element method , also called a distinct element method is any of family of numerical methods for computing the motion of a large number of particles of micrometre-scale size and above...

codes, e.g. ELFEN, provide for the modelling of both intact rock behaviour and the development and behaviour of fractures.

External links

The source of this article is wikipedia, the free encyclopedia. The text of this article is licensed under the GFDL.

Conventional methods of analysis

Limit equilibrium analysis

Stereographic and kinematic analysis

Rockfall simulators

Rock mass classification

Numerical methods of analysis

Continuum modelling

Discontinuum modelling

Hybrid/coupled modelling

See also

External links