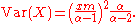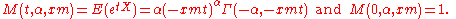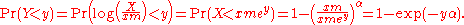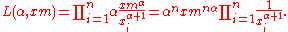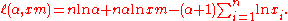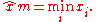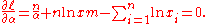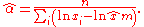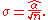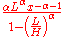
Pareto distribution
Encyclopedia
The Pareto distribution, named after the Italian economist
Vilfredo Pareto
, is a power law
probability distribution
that coincides with social
, scientific, geophysical, actuarial
, and many other types of observable phenomena. Outside the field of economics it is sometimes referred to as the Bradford distribution
.
with a Pareto (Type I) distribution, then the probability that X is greater than some number x is given by

where xm is the (necessarily positive) minimum possible value of X, and α is a positive parameter. The family of Pareto distributions is parameterized by two quantities, xm and α. When this distribution is used to model the distribution of wealth, then the parameter α is called the Pareto index
.
It follows from the above that the cumulative distribution function of a Pareto random variable with parameters α and xm is
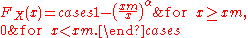
. All segments of the curve are self-similar (subject to appropriate scaling factors).
When plotted on logarithm
ic scales (both axes logarithmic), the distribution is represented by a straight line.
) that the probability density function is
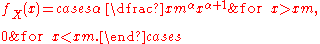
is a limiting case of the Pareto density:
s whose probability distribution is supported on the interval[ xm, ∞) for some xm > 0. Suppose that for all n, the two random variables min{ X1, ..., Xn } and (X1 + ... + Xn)/min{ X1, ..., Xn } are independent. Then the common distribution is a Pareto distribution.
originally used this distribution to describe the allocation of wealth
among individuals since it seemed to show rather well the way that a larger portion of the wealth of any society is owned by a smaller percentage of the people in that society. He also used it to describe distribution of income. This idea is sometimes expressed more simply as the Pareto principle
or the "80-20 rule" which says that 20% of the population controls 80% of the wealth. However, the 80-20 rule corresponds to a particular value of α, and in fact, Pareto's data on British income taxes in his Cours d'économie politique indicates that about 30% of the population had about 70% of the income. The probability density function
(PDF) graph at the beginning of this article shows that the "probability" or fraction of the population that owns a small amount of wealth per person is rather high, and then decreases steadily as wealth increases. This distribution is not limited to describing wealth or income, but to many situations in which an equilibrium is found in the distribution of the "small" to the "large". The following examples are sometimes seen as approximately Pareto-distributed:
and normal distribution. (Both of these latter two distributions are "basic" in the sense that the logarithms of their density functions are linear and quadratic, respectively, functions of the observed values.)
s.
as follows. If X is Pareto-distributed with minimum xm and index α, then
is exponentially distributed
with intensity α. Equivalently, if Y is exponentially distributed with intensity α, then
is Pareto-distributed with minimum xm and index α.
This can be shown using the standard change of variable techniques:
The last expression is the cumulative distribution function of an exponential distribution with intensity α.
This does not apply only to income, but also to wealth, or to anything else that can be modeled by this distribution.
This excludes Pareto distributions in which 0 < α ≤ 1, which, as noted above, have infinite expected value, and so cannot reasonably model income distribution.
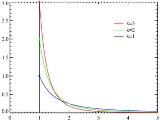
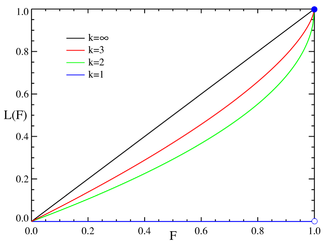 The Lorenz curve
The Lorenz curve
is often used to characterize income and wealth distributions. For any distribution, the Lorenz curve L(F) is written in terms of the PDF ƒ or the CDF F as
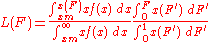
where x(F) is the inverse of the CDF. For the Pareto distribution,

and the Lorenz curve is calculated to be
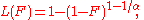
where α must be greater than or equal to unity, since the denominator in the expression for L(F) is just the mean value of x. Examples of the Lorenz curve for a number of Pareto distributions are shown in the graph on the right.
The Gini coefficient
is a measure of the deviation of the Lorenz curve from the equidistribution line which is a line connecting [0, 0] and [1, 1], which is shown in black (α = ∞) in the Lorenz plot on the right. Specifically, the Gini coefficient is twice the area between the Lorenz curve and the equidistribution line. The Gini coefficient for the Pareto distribution is then calculated to be
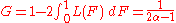
(see Aaberge 2005).
for the Pareto distribution parameters α and xm, given a sample
x = (x1, x2, ..., xn), is
Therefore, the logarithmic likelihood function is
It can be seen that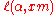 is monotonically increasing with
is monotonically increasing with  , that is, the greater the value of
, that is, the greater the value of  , the greater the value of the likelihood function. Hence, since
, the greater the value of the likelihood function. Hence, since 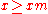 , we conclude that
, we conclude that
To find the estimator
for α, we compute the corresponding partial derivative and determine where it is zero:
Thus the maximum likelihood
estimator for α is:
The expected statistical error is:
' distribution when plotted on a linear scale, masks the underlying simplicity of the function when plotted on a log-log graph
, which then takes the form of a straight line with negative gradient.
on the unit interval (0, 1), the variate T given by
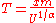
is Pareto-distributed.
(The Variance in the table on the right should be interpreted as 2nd Moment).
The probability density function
is
where L ≤ x ≤ H, and α > 0.
on
(0, 1) , then
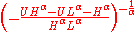
is bounded Pareto-distributed.
:
Economist
An economist is a professional in the social science discipline of economics. The individual may also study, develop, and apply theories and concepts from economics and write about economic policy...
Vilfredo Pareto
Vilfredo Pareto
Vilfredo Federico Damaso Pareto , born Wilfried Fritz Pareto, was an Italian engineer, sociologist, economist, political scientist and philosopher. He made several important contributions to economics, particularly in the study of income distribution and in the analysis of individuals' choices....
, is a power law
Power law
A power law is a special kind of mathematical relationship between two quantities. When the frequency of an event varies as a power of some attribute of that event , the frequency is said to follow a power law. For instance, the number of cities having a certain population size is found to vary...
probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
that coincides with social
Social sciences
Social science is the field of study concerned with society. "Social science" is commonly used as an umbrella term to refer to a plurality of fields outside of the natural sciences usually exclusive of the administrative or managerial sciences...
, scientific, geophysical, actuarial
Actuarial science
Actuarial science is the discipline that applies mathematical and statistical methods to assess risk in the insurance and finance industries. Actuaries are professionals who are qualified in this field through education and experience...
, and many other types of observable phenomena. Outside the field of economics it is sometimes referred to as the Bradford distribution
Bradford's law
Bradford's law is a pattern first described by Samuel C. Bradford in 1934 that estimates the exponentially diminishing returns of extending a search for references in science journals...
.
Definition
If X is a random variableRandom variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
with a Pareto (Type I) distribution, then the probability that X is greater than some number x is given by

where xm is the (necessarily positive) minimum possible value of X, and α is a positive parameter. The family of Pareto distributions is parameterized by two quantities, xm and α. When this distribution is used to model the distribution of wealth, then the parameter α is called the Pareto index
Pareto index
In economics the Pareto index, named after the Italian economist and sociologist Vilfredo Pareto, is a measure of the breadth of income or wealth distribution. It is one of the parameters specifying a Pareto distribution and embodies the Pareto principle...
.
It follows from the above that the cumulative distribution function of a Pareto random variable with parameters α and xm is
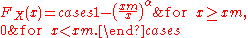
Graphical representation
When plotted on linear axes, the distribution assumes the familiar J-shaped curve which approaches each of the orthogonal axes asymptoticallyAsymptote
In analytic geometry, an asymptote of a curve is a line such that the distance between the curve and the line approaches zero as they tend to infinity. Some sources include the requirement that the curve may not cross the line infinitely often, but this is unusual for modern authors...
. All segments of the curve are self-similar (subject to appropriate scaling factors).
When plotted on logarithm
Logarithm
The logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: More generally, if x = by, then y is the logarithm of x to base b, and is written...
ic scales (both axes logarithmic), the distribution is represented by a straight line.
Density function
It follows (by differentiationDerivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
) that the probability density function is
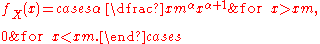
Moments and characteristic function
- The expected valueExpected valueIn probability theory, the expected value of a random variable is the weighted average of all possible values that this random variable can take on...
of a random variableRandom variableIn probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
following a Pareto distribution with α > 1 is
-
-
- (if α ≤ 1, the expected value does not exist).
- The varianceVarianceIn probability theory and statistics, the variance is a measure of how far a set of numbers is spread out. It is one of several descriptors of a probability distribution, describing how far the numbers lie from the mean . In particular, the variance is one of the moments of a distribution...
is
-
-
- (If α ≤ 2, the variance does not exist.)
- The raw momentsMoment (mathematics)In mathematics, a moment is, loosely speaking, a quantitative measure of the shape of a set of points. The "second moment", for example, is widely used and measures the "width" of a set of points in one dimension or in higher dimensions measures the shape of a cloud of points as it could be fit by...
are
-
-
- but the nth moment exists only for n < α.
- The moment generating functionMoment-generating functionIn probability theory and statistics, the moment-generating function of any random variable is an alternative definition of its probability distribution. Thus, it provides the basis of an alternative route to analytical results compared with working directly with probability density functions or...
is only defined for non-positive values t ≤ 0 as
- The characteristic functionCharacteristic function (probability theory)In probability theory and statistics, the characteristic function of any random variable completely defines its probability distribution. Thus it provides the basis of an alternative route to analytical results compared with working directly with probability density functions or cumulative...
is given by
-
-
- where Γ(a, x) is the incomplete gamma functionIncomplete gamma functionIn mathematics, the gamma function is defined by a definite integral. The incomplete gamma function is defined as an integral function of the same integrand. There are two varieties of the incomplete gamma function: the upper incomplete gamma function is for the case that the lower limit of...
.
Degenerate case
The Dirac delta functionDirac delta function
The Dirac delta function, or δ function, is a generalized function depending on a real parameter such that it is zero for all values of the parameter except when the parameter is zero, and its integral over the parameter from −∞ to ∞ is equal to one. It was introduced by theoretical...
is a limiting case of the Pareto density:
Conditional distributions
The conditional probability distribution of a Pareto-distributed random variable, given the event that it is greater than or equal to a particular number x1 exceeding xm, is a Pareto distribution with the same Pareto index α but with minimum x1 instead of xm.A characterization theorem
Suppose Xi, i = 1, 2, 3, ... are independent identically distributed random variableRandom variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
s whose probability distribution is supported on the interval
Applications
ParetoVilfredo Pareto
Vilfredo Federico Damaso Pareto , born Wilfried Fritz Pareto, was an Italian engineer, sociologist, economist, political scientist and philosopher. He made several important contributions to economics, particularly in the study of income distribution and in the analysis of individuals' choices....
originally used this distribution to describe the allocation of wealth
Distribution of wealth
The distribution of wealth is a comparison of the wealth of various members or groups in a society. It differs from the distribution of income in that it looks at the distribution of ownership of the assets in a society, rather than the current income of members of that society.-Definition of...
among individuals since it seemed to show rather well the way that a larger portion of the wealth of any society is owned by a smaller percentage of the people in that society. He also used it to describe distribution of income. This idea is sometimes expressed more simply as the Pareto principle
Pareto principle
The Pareto principle states that, for many events, roughly 80% of the effects come from 20% of the causes.Business-management consultant Joseph M...
or the "80-20 rule" which says that 20% of the population controls 80% of the wealth. However, the 80-20 rule corresponds to a particular value of α, and in fact, Pareto's data on British income taxes in his Cours d'économie politique indicates that about 30% of the population had about 70% of the income. The probability density function
Probability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
(PDF) graph at the beginning of this article shows that the "probability" or fraction of the population that owns a small amount of wealth per person is rather high, and then decreases steadily as wealth increases. This distribution is not limited to describing wealth or income, but to many situations in which an equilibrium is found in the distribution of the "small" to the "large". The following examples are sometimes seen as approximately Pareto-distributed:
- The sizes of human settlements (few cities, many hamlets/villages)
- File size distribution of Internet traffic which uses the TCP protocol (many smaller files, few larger ones)
- Hard disk drive error rates
- Clusters of Bose–Einstein condensate near absolute zeroAbsolute zeroAbsolute zero is the theoretical temperature at which entropy reaches its minimum value. The laws of thermodynamics state that absolute zero cannot be reached using only thermodynamic means....
- The values of oil reservesOil reservesThe total estimated amount of oil in an oil reservoir, including both producible and non-producible oil, is called oil in place. However, because of reservoir characteristics and limitations in petroleum extraction technologies, only a fraction of this oil can be brought to the surface, and it is...
in oil fields (a few large fields, many small fields) - The length distribution in jobs assigned supercomputers (a few large ones, many small ones)
- The standardized price returns on individual stocks
- Sizes of sand particles
- Sizes of meteorites
- Numbers of species per genus (There is subjectivity involved: The tendency to divide a genus into two or more increases with the number of species in it)
- Areas burnt in forest fires
- Severity of large casualtyCasualty (person)A casualty is a person who is the victim of an accident, injury, or trauma. The word casualties is most often used by the news media to describe deaths and injuries resulting from wars or disasters...
losses for certain lines of business such as general liability, commercial auto, and workers compensation. - In hydrologyHydrologyHydrology is the study of the movement, distribution, and quality of water on Earth and other planets, including the hydrologic cycle, water resources and environmental watershed sustainability...
the Pareto distribution is applied to extreme events such as annually maximum one-day rainfalls and river discharges. The blue picture illustrates an example of fitting the Pareto distribution to ranked annually maximum one-day rainfalls showing also the 90% confidence belt based on the binomial distribution. The rainfall data are represented by plotting positions as part of the cumulative frequency analysisCumulative frequency analysisCumulative frequency analysis is the applcation of estimation theory to exceedance probability . The complement, the non-exceedance probability concerns the frequency of occurrence of values of a phenomenon staying below a reference value. The phenomenon may be time or space dependent...
.
Relation to the log-normal distribution
Note that the Pareto distribution and log-normal distribution are alternative distributions for describing the same types of quantities. One of the connections between the two is that they are both the distributions of the exponential of random variables distributed according to other common distributions, respectively the exponential distributionExponential distribution
In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
and normal distribution. (Both of these latter two distributions are "basic" in the sense that the logarithms of their density functions are linear and quadratic, respectively, functions of the observed values.)
Relation to the generalized Pareto distribution
The Pareto distribution is a special case of the generalized Pareto distribution, which is a family of distributions of similar form, but containing an extra parameter in such a way that the support of the distribution is either bounded below (at a variable point), or bounded both above and below (where both are variable). This family also contains both the unshifted and shifted exponential distributionExponential distribution
In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
s.
Relation to the exponential distribution
The Pareto distribution is related to the exponential distributionExponential distribution
In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
as follows. If X is Pareto-distributed with minimum xm and index α, then
is exponentially distributed
Exponential distribution
In probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
with intensity α. Equivalently, if Y is exponentially distributed with intensity α, then
is Pareto-distributed with minimum xm and index α.
This can be shown using the standard change of variable techniques:
The last expression is the cumulative distribution function of an exponential distribution with intensity α.
Relation to Zipf's law
Pareto distributions are continuous probability distributions. Zipf's law, also sometimes called the zeta distribution, may be thought of as a discrete counterpart of the Pareto distribution.Relation to the "Pareto principle"
The "80-20 law", according to which 20% of all people receive 80% of all income, and 20% of the most affluent 20% receive 80% of that 80%, and so on, holds precisely when the Pareto index is α = log45, approximately 1.161. Moreover, the following have been shown to be mathematically equivalent:- Income is distributed according to a Pareto distribution with index α > 1.
- There is some number 0 ≤ p ≤ 1/2 such that 100p% of all people receive 100(1 − p)% of all income, and similarly for every real (not necessarily integer) n > 0, 100pn% of all people receive 100(1 − p)n% of all income.
This does not apply only to income, but also to wealth, or to anything else that can be modeled by this distribution.
This excludes Pareto distributions in which 0 < α ≤ 1, which, as noted above, have infinite expected value, and so cannot reasonably model income distribution.
Pareto, Lorenz, and Gini

Lorenz curve
In economics, the Lorenz curve is a graphical representation of the cumulative distribution function of the empirical probability distribution of wealth; it is a graph showing the proportion of the distribution assumed by the bottom y% of the values...
is often used to characterize income and wealth distributions. For any distribution, the Lorenz curve L(F) is written in terms of the PDF ƒ or the CDF F as
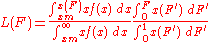
where x(F) is the inverse of the CDF. For the Pareto distribution,

and the Lorenz curve is calculated to be
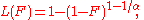
where α must be greater than or equal to unity, since the denominator in the expression for L(F) is just the mean value of x. Examples of the Lorenz curve for a number of Pareto distributions are shown in the graph on the right.
The Gini coefficient
Gini coefficient
The Gini coefficient is a measure of statistical dispersion developed by the Italian statistician and sociologist Corrado Gini and published in his 1912 paper "Variability and Mutability" ....
is a measure of the deviation of the Lorenz curve from the equidistribution line which is a line connecting [0, 0] and [1, 1], which is shown in black (α = ∞) in the Lorenz plot on the right. Specifically, the Gini coefficient is twice the area between the Lorenz curve and the equidistribution line. The Gini coefficient for the Pareto distribution is then calculated to be
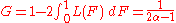
(see Aaberge 2005).
Parameter estimation
The likelihood functionLikelihood function
In statistics, a likelihood function is a function of the parameters of a statistical model, defined as follows: the likelihood of a set of parameter values given some observed outcomes is equal to the probability of those observed outcomes given those parameter values...
for the Pareto distribution parameters α and xm, given a sample
Sample (statistics)
In statistics, a sample is a subset of a population. Typically, the population is very large, making a census or a complete enumeration of all the values in the population impractical or impossible. The sample represents a subset of manageable size...
x = (x1, x2, ..., xn), is
Therefore, the logarithmic likelihood function is
It can be seen that
 is monotonically increasing with
is monotonically increasing with  , that is, the greater the value of
, that is, the greater the value of  , the greater the value of the likelihood function. Hence, since
, the greater the value of the likelihood function. Hence, since 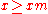 , we conclude that
, we conclude thatTo find the estimator
Estimator
In statistics, an estimator is a rule for calculating an estimate of a given quantity based on observed data: thus the rule and its result are distinguished....
for α, we compute the corresponding partial derivative and determine where it is zero:
Thus the maximum likelihood
Maximum likelihood
In statistics, maximum-likelihood estimation is a method of estimating the parameters of a statistical model. When applied to a data set and given a statistical model, maximum-likelihood estimation provides estimates for the model's parameters....
estimator for α is:
The expected statistical error is:
Graphical representation
The characteristic curved 'long tailLong tail
Long tail may refer to:*The Long Tail, a consumer demographic in business*Power law's long tail, a statistics term describing certain kinds of distribution*Long-tail boat, a type of watercraft native to Southeast Asia...
' distribution when plotted on a linear scale, masks the underlying simplicity of the function when plotted on a log-log graph
Log-log graph
In science and engineering, a log-log graph or log-log plot is a two-dimensional graph of numerical data that uses logarithmic scales on both the horizontal and vertical axes...
, which then takes the form of a straight line with negative gradient.
Generating a random sample from Pareto distribution
Random samples can be generated using inverse transform sampling. Given a random variate U drawn from the uniform distributionUniform distribution (continuous)
In probability theory and statistics, the continuous uniform distribution or rectangular distribution is a family of probability distributions such that for each member of the family, all intervals of the same length on the distribution's support are equally probable. The support is defined by...
on the unit interval (0, 1), the variate T given by
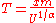
is Pareto-distributed.
Bounded Pareto distribution
The bounded Pareto distribution has three parameters α, L and H. As in the standard Pareto distribution α determines the shape. L denotes the minimal value, and H denotes the maximal value.(The Variance in the table on the right should be interpreted as 2nd Moment).
The probability density function
Probability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
is
where L ≤ x ≤ H, and α > 0.
Generating bounded Pareto random variables
If U is uniformly distributedUniform distribution (continuous)
In probability theory and statistics, the continuous uniform distribution or rectangular distribution is a family of probability distributions such that for each member of the family, all intervals of the same length on the distribution's support are equally probable. The support is defined by...
on
(0, 1
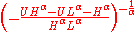
is bounded Pareto-distributed.
Symmetric Pareto distribution
The symmetric Pareto distribution can be defined by the probability density functionProbability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
:
-
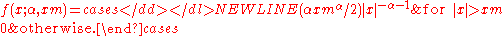
The density is zero for x between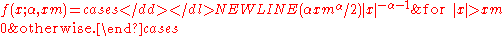 and
and  . It has a similar shape to a Pareto distribution for
. It has a similar shape to a Pareto distribution for 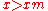 while looking like an inverted Pareto distribution for
while looking like an inverted Pareto distribution for 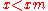
See also
- Cumulative frequency analysisCumulative frequency analysisCumulative frequency analysis is the applcation of estimation theory to exceedance probability . The complement, the non-exceedance probability concerns the frequency of occurrence of values of a phenomenon staying below a reference value. The phenomenon may be time or space dependent...
- Pareto analysisPareto analysisPareto analysis is a statistical technique in decision making that is used for selection of a limited number of tasks that produce significant overall effect. It uses the Pareto principle – the idea that by doing 20% of work, 80% of the advantage of doing the entire job can be generated...
- Pareto efficiencyPareto efficiencyPareto efficiency, or Pareto optimality, is a concept in economics with applications in engineering and social sciences. The term is named after Vilfredo Pareto, an Italian economist who used the concept in his studies of economic efficiency and income distribution.Given an initial allocation of...
- Pareto interpolationPareto interpolationPareto interpolation is a method of estimating the median and other properties of a population that follows a Pareto distribution. It is used in economics when analysing the distribution of incomes in a population, when one must base estimates on a relatively small random sample taken from the...
- Pareto principlePareto principleThe Pareto principle states that, for many events, roughly 80% of the effects come from 20% of the causes.Business-management consultant Joseph M...
- The Long TailThe Long TailThe Long Tail or long tail refers to the statistical property that a larger share of population rests within the tail of a probability distribution than observed under a 'normal' or Gaussian distribution...
- Traffic generation model
External links
- The Pareto, Zipf and other power laws / William J. Reed – PDF
- Gini's Nuclear Family / Rolf Aabergé. – In: International Conference to Honor Two Eminent Social Scientists, May, 2005 – PDF
- syntraf1.c is a C program to generate synthetic packet traffic with bounded Pareto burst size and exponential interburst time.
- "Self-Similarity in World Wide Web Traffic: Evidence and Possible Causes" /Mark E. Crovella and Azer Bestavros
- Cumulative frequency analysis