
Hamilton–Jacobi equation
Encyclopedia
In mathematics, the Hamilton–Jacobi equation is a necessary condition describing extremal geometry
in generalizations of problems from the calculus of variations
. In physics
, the Hamilton–Jacobi equation (HJE) is a reformulation of classical mechanics
and, thus, equivalent to other formulations such as Newton's laws of motion
, Lagrangian mechanics
and Hamiltonian mechanics
. The Hamilton–Jacobi equation is particularly useful in identifying conserved quantities for mechanical systems, which may be possible even when the mechanical problem itself cannot be solved completely.
The HJE is also the only formulation of mechanics in which the motion of a particle can be represented as a wave. In this sense, the HJE fulfilled a long-held goal of theoretical physics (dating at least to Johann Bernoulli
in the 18th century) of finding an analogy between the propagation of light and the motion of a particle. The wave equation followed by mechanical systems is similar to, but not identical with, Schrödinger's equation, as described below; for this reason, the HJE is considered the "closest approach" of classical mechanics
to quantum mechanics
.
for a function called Hamilton's principal function
called Hamilton's principal function
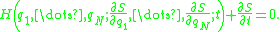
As described below, this equation may be derived from Hamiltonian mechanics
by treating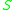 as the generating function for a canonical transformation
as the generating function for a canonical transformation
of the classical Hamiltonian
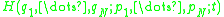 . The conjugate momenta correspond to the first derivatives of
. The conjugate momenta correspond to the first derivatives of 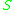 with respect to the generalized coordinates
with respect to the generalized coordinates
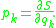
As a solution to the Hamilton-Jacobi equation, the principal function contains N+1 undetermined constants, the first N of them denoted as , and the last one coming from the integration of
, and the last one coming from the integration of 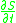 . The relationship then between p and q describes the orbit in phase space
. The relationship then between p and q describes the orbit in phase space
in terms of these constants of motion. Furthermore, the quantities
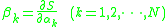
are also constants of motion, and these equations can be inverted to find q as a function of all the and
and 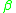 constants and time.
constants and time.
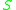 of the
of the 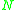 generalized coordinates
generalized coordinates 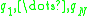 and the time
and the time  . The generalized momenta do not appear, except as derivatives of
. The generalized momenta do not appear, except as derivatives of 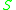 . Remarkably, the function
. Remarkably, the function 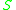 is equal to the classical action
is equal to the classical action
.
For comparison, in the equivalent Euler–Lagrange equations of motion of Lagrangian mechanics
, the conjugate momenta also do not appear; however, those equations are a system of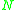 , generally second-order equations for the time evolution of the generalized coordinates. Similarly, Hamilton's equations of motion are another system of
, generally second-order equations for the time evolution of the generalized coordinates. Similarly, Hamilton's equations of motion are another system of 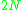 first-order equations for the time evolution of the generalized coordinates and their conjugate momenta
first-order equations for the time evolution of the generalized coordinates and their conjugate momenta 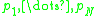 .
.
Since the HJE is an equivalent expression of an integral minimization problem such as Hamilton's principle
, the HJE can be useful in other problems of the calculus of variations
and, more generally, in other branches of mathematics
and physics
, such as dynamical systems, symplectic geometry and quantum chaos
. For example, the Hamilton–Jacobi equations can be used to determine the geodesic
s on a Riemannian manifold
, an important variational problem
in Riemannian geometry
.
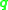 to represent the list of
to represent the list of 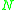 generalized coordinates
generalized coordinates
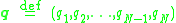
that need not transform like a vector under rotation
. The dot product
is defined here as the sum of the products of corresponding components, i.e.,
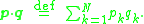
involving a type-2 generating function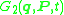 leads to the relations
leads to the relations
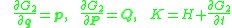
(See the canonical transformation
article for more details.)
To derive the HJE, we choose a generating function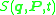 that makes the new Hamiltonian
that makes the new Hamiltonian  identically zero. Hence, all its derivatives are also zero, and Hamilton's equations become trivial
identically zero. Hence, all its derivatives are also zero, and Hamilton's equations become trivial

i.e., the new generalized coordinates and momenta are constants of motion
. The new generalized momenta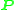 are usually denoted
are usually denoted 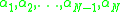 , i.e.,
, i.e., 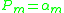 .
.
The equation for the transformed Hamiltonian
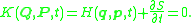
Let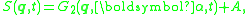
where A is an arbitrary constant, then S satisfies HJE
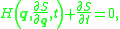
since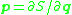 .
.
The new generalized coordinates
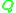 are also constants, typically denoted as
are also constants, typically denoted as 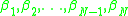 . Once we have solved for
. Once we have solved for 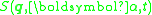 , these also give useful equations
, these also give useful equations
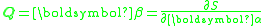
or written in components for clarity
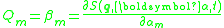
Ideally, these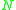 equations can be inverted to find the original generalized coordinates
equations can be inverted to find the original generalized coordinates
 as a function of the constants
as a function of the constants 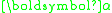 and
and 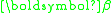 , thus solving the original problem.
, thus solving the original problem.
.
The time derivative of S is
therefore
so S is actually classical action plus an undetermined constant.
When H does not explicitly depend on time,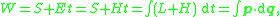
in this case W is the same as abbreviated action.
, which directly identifies constants of motion
. For example, the time can be separated if the Hamiltonian does not depend on time explicitly. In that case, the time derivative
can be separated if the Hamiltonian does not depend on time explicitly. In that case, the time derivative  in the HJE must be a constant (usually denoted ), giving the separated solution
in the HJE must be a constant (usually denoted ), giving the separated solution
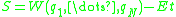
where the time-independent function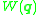 is sometimes called Hamilton's characteristic function. The reduced Hamilton–Jacobi equation can then be written
is sometimes called Hamilton's characteristic function. The reduced Hamilton–Jacobi equation can then be written
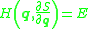
To illustrate separability for other variables, we assume that a certain generalized coordinate and its derivative
and its derivative 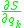 appear together in the Hamiltonian as a single function
appear together in the Hamiltonian as a single function 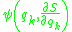

In that case, the function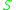 can be partitioned into two functions, one that depends only on
can be partitioned into two functions, one that depends only on  and another that depends only on the remaining generalized coordinates
and another that depends only on the remaining generalized coordinates
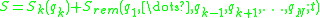
Substitution of these formulae into the Hamilton–Jacobi equation shows that the function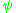 must be a constant (denoted here as
must be a constant (denoted here as 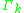 ), yielding a first-order ordinary differential equation
), yielding a first-order ordinary differential equation
for
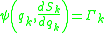
In fortunate cases, the function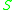 can be separated completely into
can be separated completely into 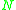 functions
functions 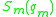
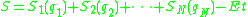
In such a case, the problem devolves to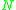 ordinary differential equation
ordinary differential equation
s.
The separability of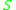 depends both on the Hamiltonian and on the choice of generalized coordinates. For orthogonal coordinates
depends both on the Hamiltonian and on the choice of generalized coordinates. For orthogonal coordinates
and Hamiltonians that have no time dependence and are quadratic
in the generalized momenta, will be completely separable if the potential energy is additively separable in each coordinate, where the potential energy term for each coordinate is multiplied by the coordinate-dependent factor in the corresponding momentum term of the Hamiltonian (the Staeckel conditions). For illustration, several examples in orthogonal coordinates
will be completely separable if the potential energy is additively separable in each coordinate, where the potential energy term for each coordinate is multiplied by the coordinate-dependent factor in the corresponding momentum term of the Hamiltonian (the Staeckel conditions). For illustration, several examples in orthogonal coordinates
are worked in the next sections.
 can be written
can be written
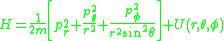
The Hamilton–Jacobi equation is completely separable in these coordinates provided that there exist functions ,
, 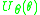 and
and 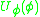 such that
such that 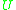 can be written in the analogous form
can be written in the analogous form
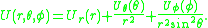
Substitution of the completely separated solution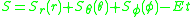 into the HJE yields
into the HJE yields
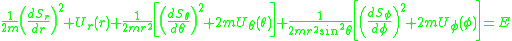
This equation may be solved by successive integrations of ordinary differential equation
s, beginning with the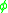 equation
equation
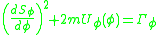
where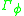 is a constant of the motion
is a constant of the motion
that eliminates the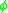 dependence from the Hamilton–Jacobi equation
dependence from the Hamilton–Jacobi equation
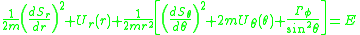
The next ordinary differential equation
involves the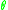 generalized coordinate
generalized coordinate
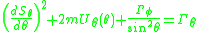
where is again a constant of the motion
is again a constant of the motion
that eliminates the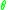 dependence and reduces the HJE to the final ordinary differential equation
dependence and reduces the HJE to the final ordinary differential equation

whose integration completes the solution for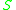 .
.
can be written
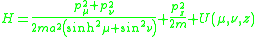
where the foci
of the ellipse
s are located at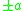 on the
on the  -axis. The Hamilton–Jacobi equation is completely separable in these coordinates provided that
-axis. The Hamilton–Jacobi equation is completely separable in these coordinates provided that 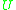 has an analogous form
has an analogous form
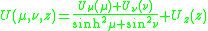
where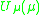 ,
, 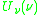 and
and  are arbitrary functions. Substitution of the completely separated solution
are arbitrary functions. Substitution of the completely separated solution 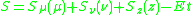 into the HJE yields
into the HJE yields
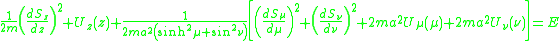
Separating the first ordinary differential equation

yields the reduced Hamilton–Jacobi equation (after re-arrangement and multiplication of both sides by the denominator)
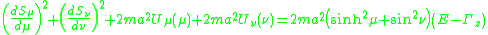
which itself may be separated into two independent ordinary differential equations
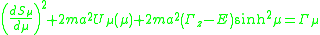
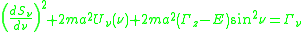
that, when solved, provide a complete solution for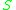 .
.
can be written
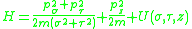
The Hamilton–Jacobi equation is completely separable in these coordinates provided that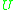 has an analogous form
has an analogous form
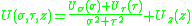
where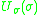 ,
, 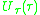 and
and 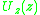 are arbitrary functions. Substitution of the completely separated solution
are arbitrary functions. Substitution of the completely separated solution 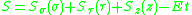 into the HJE yields
into the HJE yields
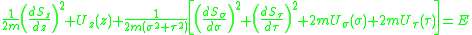
Separating the first ordinary differential equation
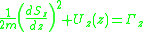
yields the reduced Hamilton–Jacobi equation (after re-arrangement and multiplication of both sides by the denominator)
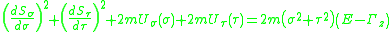
which itself may be separated into two independent ordinary differential equations
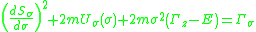
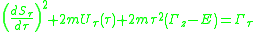
that, when solved, provide a complete solution for .
.
s of the function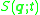 can be determined at any time
can be determined at any time  . The motion of an
. The motion of an 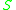 -isosurface as a function of time is defined by the motions of the particles beginning at the points
-isosurface as a function of time is defined by the motions of the particles beginning at the points 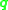 on the isosurface. The motion of such an isosurface can be thought of as a wave
on the isosurface. The motion of such an isosurface can be thought of as a wave
moving through space, although it does not obey the wave equation
space, although it does not obey the wave equation
exactly. To show this, let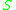 represent the phase
represent the phase
of a wave
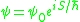
where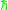 is a constant introduced to make the exponential argument unitless; changes in the amplitude
is a constant introduced to make the exponential argument unitless; changes in the amplitude
of the wave
can be represented by having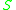 be a complex number. We may then rewrite the Hamilton–Jacobi equation as
be a complex number. We may then rewrite the Hamilton–Jacobi equation as
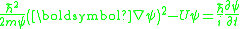
which is a nonlinear variant of the Schrödinger equation
.
Conversely, starting with the Schrödinger equation
and our Ansatz
for , we arrive at
, we arrive at

The classical limit ( ) of the Schrödinger equation
) of the Schrödinger equation
above becomes identical to the following variant of the Hamilton–Jacobi equation,
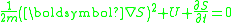
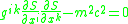
where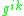 are the contravariant coordinates of the metric tensor
are the contravariant coordinates of the metric tensor
, m is the rest mass of the particle and c is the speed of light
.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
in generalizations of problems from the calculus of variations
Calculus of variations
Calculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
. In physics
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, the Hamilton–Jacobi equation (HJE) is a reformulation of classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
and, thus, equivalent to other formulations such as Newton's laws of motion
Newton's laws of motion
Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...
, Lagrangian mechanics
Lagrangian mechanics
Lagrangian mechanics is a re-formulation of classical mechanics that combines conservation of momentum with conservation of energy. It was introduced by the Italian-French mathematician Joseph-Louis Lagrange in 1788....
and Hamiltonian mechanics
Hamiltonian mechanics
Hamiltonian mechanics is a reformulation of classical mechanics that was introduced in 1833 by Irish mathematician William Rowan Hamilton.It arose from Lagrangian mechanics, a previous reformulation of classical mechanics introduced by Joseph Louis Lagrange in 1788, but can be formulated without...
. The Hamilton–Jacobi equation is particularly useful in identifying conserved quantities for mechanical systems, which may be possible even when the mechanical problem itself cannot be solved completely.
The HJE is also the only formulation of mechanics in which the motion of a particle can be represented as a wave. In this sense, the HJE fulfilled a long-held goal of theoretical physics (dating at least to Johann Bernoulli
Johann Bernoulli
Johann Bernoulli was a Swiss mathematician and was one of the many prominent mathematicians in the Bernoulli family...
in the 18th century) of finding an analogy between the propagation of light and the motion of a particle. The wave equation followed by mechanical systems is similar to, but not identical with, Schrödinger's equation, as described below; for this reason, the HJE is considered the "closest approach" of classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
to quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
.
Mathematical formulation
The Hamilton–Jacobi equation is a first-order, non-linear partial differential equationPartial differential equation
In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...
for a function
 called Hamilton's principal function
called Hamilton's principal function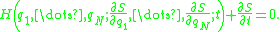
As described below, this equation may be derived from Hamiltonian mechanics
Hamiltonian mechanics
Hamiltonian mechanics is a reformulation of classical mechanics that was introduced in 1833 by Irish mathematician William Rowan Hamilton.It arose from Lagrangian mechanics, a previous reformulation of classical mechanics introduced by Joseph Louis Lagrange in 1788, but can be formulated without...
by treating
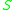 as the generating function for a canonical transformation
as the generating function for a canonical transformationCanonical transformation
In Hamiltonian mechanics, a canonical transformation is a change of canonical coordinates → that preserves the form of Hamilton's equations , although it...
of the classical Hamiltonian
Hamiltonian mechanics
Hamiltonian mechanics is a reformulation of classical mechanics that was introduced in 1833 by Irish mathematician William Rowan Hamilton.It arose from Lagrangian mechanics, a previous reformulation of classical mechanics introduced by Joseph Louis Lagrange in 1788, but can be formulated without...
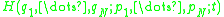 . The conjugate momenta correspond to the first derivatives of
. The conjugate momenta correspond to the first derivatives of 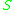 with respect to the generalized coordinates
with respect to the generalized coordinates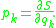
As a solution to the Hamilton-Jacobi equation, the principal function contains N+1 undetermined constants, the first N of them denoted as
 , and the last one coming from the integration of
, and the last one coming from the integration of 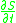 . The relationship then between p and q describes the orbit in phase space
. The relationship then between p and q describes the orbit in phase spacePhase space
In mathematics and physics, a phase space, introduced by Willard Gibbs in 1901, is a space in which all possible states of a system are represented, with each possible state of the system corresponding to one unique point in the phase space...
in terms of these constants of motion. Furthermore, the quantities
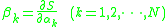
are also constants of motion, and these equations can be inverted to find q as a function of all the
 and
and 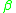 constants and time.
constants and time.Comparison with other formulations of mechanics
The HJE is a single, first-order partial differential equation for the function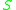 of the
of the 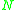 generalized coordinates
generalized coordinates 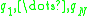 and the time
and the time  . The generalized momenta do not appear, except as derivatives of
. The generalized momenta do not appear, except as derivatives of 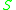 . Remarkably, the function
. Remarkably, the function 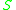 is equal to the classical action
is equal to the classical actionAction (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
.
For comparison, in the equivalent Euler–Lagrange equations of motion of Lagrangian mechanics
Lagrangian mechanics
Lagrangian mechanics is a re-formulation of classical mechanics that combines conservation of momentum with conservation of energy. It was introduced by the Italian-French mathematician Joseph-Louis Lagrange in 1788....
, the conjugate momenta also do not appear; however, those equations are a system of
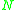 , generally second-order equations for the time evolution of the generalized coordinates. Similarly, Hamilton's equations of motion are another system of
, generally second-order equations for the time evolution of the generalized coordinates. Similarly, Hamilton's equations of motion are another system of 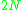 first-order equations for the time evolution of the generalized coordinates and their conjugate momenta
first-order equations for the time evolution of the generalized coordinates and their conjugate momenta 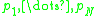 .
.Since the HJE is an equivalent expression of an integral minimization problem such as Hamilton's principle
Hamilton's principle
In physics, Hamilton's principle is William Rowan Hamilton's formulation of the principle of stationary action...
, the HJE can be useful in other problems of the calculus of variations
Calculus of variations
Calculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
and, more generally, in other branches of mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
and physics
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, such as dynamical systems, symplectic geometry and quantum chaos
Quantum chaos
Quantum chaos is a branch of physics which studies how chaotic classical dynamical systems can be described in terms of quantum theory. The primary question that quantum chaos seeks to answer is, "What is the relationship between quantum mechanics and classical chaos?" The correspondence principle...
. For example, the Hamilton–Jacobi equations can be used to determine the geodesic
Geodesic
In mathematics, a geodesic is a generalization of the notion of a "straight line" to "curved spaces". In the presence of a Riemannian metric, geodesics are defined to be the shortest path between points in the space...
s on a Riemannian manifold
Riemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
, an important variational problem
Calculus of variations
Calculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
in Riemannian geometry
Riemannian geometry
Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point which varies smoothly from point to point. This gives, in particular, local notions of angle, length...
.
Notation
For brevity, we use boldface variables such as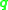 to represent the list of
to represent the list of 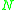 generalized coordinates
generalized coordinatesGeneralized coordinates
In the study of multibody systems, generalized coordinates are a set of coordinates used to describe the configuration of a system relative to some reference configuration....
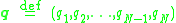
that need not transform like a vector under rotation
Rotation
A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...
. The dot product
Dot product
In mathematics, the dot product or scalar product is an algebraic operation that takes two equal-length sequences of numbers and returns a single number obtained by multiplying corresponding entries and then summing those products...
is defined here as the sum of the products of corresponding components, i.e.,
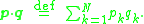
Derivation
Any canonical transformationCanonical transformation
In Hamiltonian mechanics, a canonical transformation is a change of canonical coordinates → that preserves the form of Hamilton's equations , although it...
involving a type-2 generating function
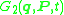 leads to the relations
leads to the relations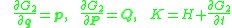
(See the canonical transformation
Canonical transformation
In Hamiltonian mechanics, a canonical transformation is a change of canonical coordinates → that preserves the form of Hamilton's equations , although it...
article for more details.)
To derive the HJE, we choose a generating function
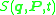 that makes the new Hamiltonian
that makes the new Hamiltonian  identically zero. Hence, all its derivatives are also zero, and Hamilton's equations become trivial
identically zero. Hence, all its derivatives are also zero, and Hamilton's equations become trivial
i.e., the new generalized coordinates and momenta are constants of motion
Constant of motion
In mechanics, a constant of motion is a quantity that is conserved throughout the motion, imposing in effect a constraint on the motion. However, it is a mathematical constraint, the natural consequence of the equations of motion, rather than a physical constraint...
. The new generalized momenta
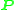 are usually denoted
are usually denoted 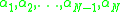 , i.e.,
, i.e., 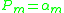 .
.The equation for the transformed Hamiltonian

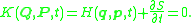
Let
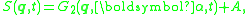
where A is an arbitrary constant, then S satisfies HJE
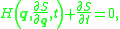
since
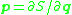 .
.The new generalized coordinates
Generalized coordinates
In the study of multibody systems, generalized coordinates are a set of coordinates used to describe the configuration of a system relative to some reference configuration....
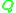 are also constants, typically denoted as
are also constants, typically denoted as 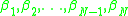 . Once we have solved for
. Once we have solved for 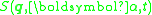 , these also give useful equations
, these also give useful equations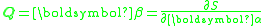
or written in components for clarity
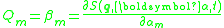
Ideally, these
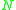 equations can be inverted to find the original generalized coordinates
equations can be inverted to find the original generalized coordinatesGeneralized coordinates
In the study of multibody systems, generalized coordinates are a set of coordinates used to describe the configuration of a system relative to some reference configuration....
 as a function of the constants
as a function of the constants 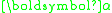 and
and 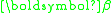 , thus solving the original problem.
, thus solving the original problem.Action
Both Hamilton principal function S and characteristic function are closely related to actionAction (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
.
The time derivative of S is

therefore

so S is actually classical action plus an undetermined constant.
When H does not explicitly depend on time,
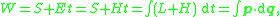
in this case W is the same as abbreviated action.
Separation of variables
The HJE is most useful when it can be solved via additive separation of variablesSeparation of variables
In mathematics, separation of variables is any of several methods for solving ordinary and partial differential equations, in which algebra allows one to rewrite an equation so that each of two variables occurs on a different side of the equation....
, which directly identifies constants of motion
Constant of motion
In mechanics, a constant of motion is a quantity that is conserved throughout the motion, imposing in effect a constraint on the motion. However, it is a mathematical constraint, the natural consequence of the equations of motion, rather than a physical constraint...
. For example, the time
 can be separated if the Hamiltonian does not depend on time explicitly. In that case, the time derivative
can be separated if the Hamiltonian does not depend on time explicitly. In that case, the time derivative  in the HJE must be a constant (usually denoted ), giving the separated solution
in the HJE must be a constant (usually denoted ), giving the separated solution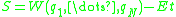
where the time-independent function
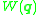 is sometimes called Hamilton's characteristic function. The reduced Hamilton–Jacobi equation can then be written
is sometimes called Hamilton's characteristic function. The reduced Hamilton–Jacobi equation can then be written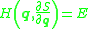
To illustrate separability for other variables, we assume that a certain generalized coordinate
 and its derivative
and its derivative 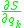 appear together in the Hamiltonian as a single function
appear together in the Hamiltonian as a single function 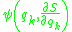

In that case, the function
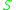 can be partitioned into two functions, one that depends only on
can be partitioned into two functions, one that depends only on  and another that depends only on the remaining generalized coordinates
and another that depends only on the remaining generalized coordinates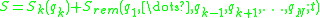
Substitution of these formulae into the Hamilton–Jacobi equation shows that the function
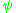 must be a constant (denoted here as
must be a constant (denoted here as 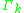 ), yielding a first-order ordinary differential equation
), yielding a first-order ordinary differential equationOrdinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
for

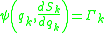
In fortunate cases, the function
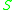 can be separated completely into
can be separated completely into 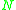 functions
functions 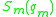
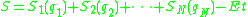
In such a case, the problem devolves to
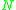 ordinary differential equation
ordinary differential equationOrdinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
s.
The separability of
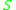 depends both on the Hamiltonian and on the choice of generalized coordinates. For orthogonal coordinates
depends both on the Hamiltonian and on the choice of generalized coordinates. For orthogonal coordinatesOrthogonal coordinates
In mathematics, orthogonal coordinates are defined as a set of d coordinates q = in which the coordinate surfaces all meet at right angles . A coordinate surface for a particular coordinate qk is the curve, surface, or hypersurface on which qk is a constant...
and Hamiltonians that have no time dependence and are quadratic
Quadratic
In mathematics, the term quadratic describes something that pertains to squares, to the operation of squaring, to terms of the second degree, or equations or formulas that involve such terms...
in the generalized momenta,
 will be completely separable if the potential energy is additively separable in each coordinate, where the potential energy term for each coordinate is multiplied by the coordinate-dependent factor in the corresponding momentum term of the Hamiltonian (the Staeckel conditions). For illustration, several examples in orthogonal coordinates
will be completely separable if the potential energy is additively separable in each coordinate, where the potential energy term for each coordinate is multiplied by the coordinate-dependent factor in the corresponding momentum term of the Hamiltonian (the Staeckel conditions). For illustration, several examples in orthogonal coordinatesOrthogonal coordinates
In mathematics, orthogonal coordinates are defined as a set of d coordinates q = in which the coordinate surfaces all meet at right angles . A coordinate surface for a particular coordinate qk is the curve, surface, or hypersurface on which qk is a constant...
are worked in the next sections.
Example of spherical coordinates
In spherical coordinates the Hamiltonian of a free particle moving in a conservative potential can be written
can be written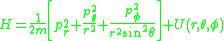
The Hamilton–Jacobi equation is completely separable in these coordinates provided that there exist functions
 ,
, 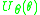 and
and 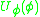 such that
such that 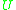 can be written in the analogous form
can be written in the analogous form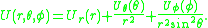
Substitution of the completely separated solution
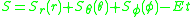 into the HJE yields
into the HJE yields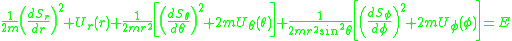
This equation may be solved by successive integrations of ordinary differential equation
Ordinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
s, beginning with the
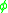 equation
equation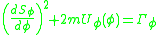
where
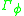 is a constant of the motion
is a constant of the motionConstant of motion
In mechanics, a constant of motion is a quantity that is conserved throughout the motion, imposing in effect a constraint on the motion. However, it is a mathematical constraint, the natural consequence of the equations of motion, rather than a physical constraint...
that eliminates the
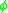 dependence from the Hamilton–Jacobi equation
dependence from the Hamilton–Jacobi equation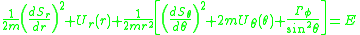
The next ordinary differential equation
Ordinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
involves the
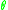 generalized coordinate
generalized coordinate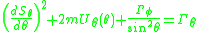
where
 is again a constant of the motion
is again a constant of the motionConstant of motion
In mechanics, a constant of motion is a quantity that is conserved throughout the motion, imposing in effect a constraint on the motion. However, it is a mathematical constraint, the natural consequence of the equations of motion, rather than a physical constraint...
that eliminates the
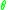 dependence and reduces the HJE to the final ordinary differential equation
dependence and reduces the HJE to the final ordinary differential equationOrdinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....

whose integration completes the solution for
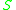 .
.Example of elliptic cylindrical coordinates
The Hamiltonian in elliptic cylindrical coordinatesElliptic cylindrical coordinates
Elliptic cylindrical coordinates are a three-dimensional orthogonal coordinate system that results from projecting the two-dimensional elliptic coordinate system in the...
can be written
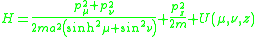
where the foci
Focus (geometry)
In geometry, the foci are a pair of special points with reference to which any of a variety of curves is constructed. For example, foci can be used in defining conic sections, the four types of which are the circle, ellipse, parabola, and hyperbola...
of the ellipse
Ellipse
In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
s are located at
 on the
on the  -axis. The Hamilton–Jacobi equation is completely separable in these coordinates provided that
-axis. The Hamilton–Jacobi equation is completely separable in these coordinates provided that 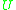 has an analogous form
has an analogous form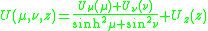
where
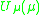 ,
, 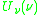 and
and  are arbitrary functions. Substitution of the completely separated solution
are arbitrary functions. Substitution of the completely separated solution 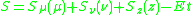 into the HJE yields
into the HJE yields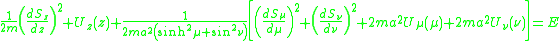
Separating the first ordinary differential equation
Ordinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....

yields the reduced Hamilton–Jacobi equation (after re-arrangement and multiplication of both sides by the denominator)
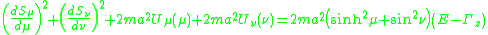
which itself may be separated into two independent ordinary differential equations
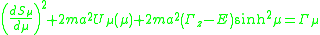
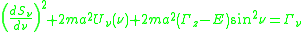
that, when solved, provide a complete solution for
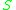 .
.Example of parabolic cylindrical coordinates
The Hamiltonian in parabolic cylindrical coordinatesParabolic cylindrical coordinates
In mathematics, parabolic cylindrical coordinates are a three-dimensional orthogonal coordinate system that results from projecting the two-dimensional parabolic coordinate system in the...
can be written
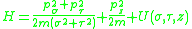
The Hamilton–Jacobi equation is completely separable in these coordinates provided that
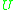 has an analogous form
has an analogous form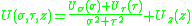
where
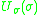 ,
, 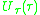 and
and 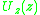 are arbitrary functions. Substitution of the completely separated solution
are arbitrary functions. Substitution of the completely separated solution 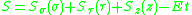 into the HJE yields
into the HJE yields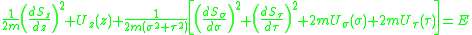
Separating the first ordinary differential equation
Ordinary differential equation
In mathematics, an ordinary differential equation is a relation that contains functions of only one independent variable, and one or more of their derivatives with respect to that variable....
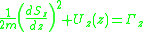
yields the reduced Hamilton–Jacobi equation (after re-arrangement and multiplication of both sides by the denominator)
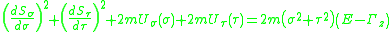
which itself may be separated into two independent ordinary differential equations
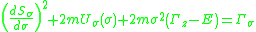
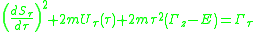
that, when solved, provide a complete solution for
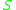 .
.Eikonal approximation and relationship to the Schrödinger equation
The isosurfaceIsosurface
An isosurface is a three-dimensional analog of an isoline. It is a surface that represents points of a constant value within a volume of space; in other words, it is a level set of a continuous function whose domain is 3D-space.Isosurfaces are normally displayed using computer graphics, and are...
s of the function
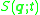 can be determined at any time
can be determined at any time  . The motion of an
. The motion of an 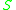 -isosurface as a function of time is defined by the motions of the particles beginning at the points
-isosurface as a function of time is defined by the motions of the particles beginning at the points 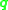 on the isosurface. The motion of such an isosurface can be thought of as a wave
on the isosurface. The motion of such an isosurface can be thought of as a waveWave
In physics, a wave is a disturbance that travels through space and time, accompanied by the transfer of energy.Waves travel and the wave motion transfers energy from one point to another, often with no permanent displacement of the particles of the medium—that is, with little or no associated mass...
moving through
 space, although it does not obey the wave equation
space, although it does not obey the wave equationWave equation
The wave equation is an important second-order linear partial differential equation for the description of waves – as they occur in physics – such as sound waves, light waves and water waves. It arises in fields like acoustics, electromagnetics, and fluid dynamics...
exactly. To show this, let
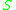 represent the phase
represent the phasePhase (waves)
Phase in waves is the fraction of a wave cycle which has elapsed relative to an arbitrary point.-Formula:The phase of an oscillation or wave refers to a sinusoidal function such as the following:...
of a wave
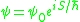
where
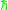 is a constant introduced to make the exponential argument unitless; changes in the amplitude
is a constant introduced to make the exponential argument unitless; changes in the amplitudeAmplitude
Amplitude is the magnitude of change in the oscillating variable with each oscillation within an oscillating system. For example, sound waves in air are oscillations in atmospheric pressure and their amplitudes are proportional to the change in pressure during one oscillation...
of the wave
Wave
In physics, a wave is a disturbance that travels through space and time, accompanied by the transfer of energy.Waves travel and the wave motion transfers energy from one point to another, often with no permanent displacement of the particles of the medium—that is, with little or no associated mass...
can be represented by having
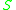 be a complex number. We may then rewrite the Hamilton–Jacobi equation as
be a complex number. We may then rewrite the Hamilton–Jacobi equation as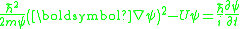
which is a nonlinear variant of the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
.
Conversely, starting with the Schrödinger equation
Schrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
and our Ansatz
Ansatz
Ansatz is a German noun with several meanings in the English language.It is widely encountered in physics and mathematics literature.Since ansatz is a noun, in German texts the initial a of this word is always capitalised.-Definition:...
for
 , we arrive at
, we arrive at
The classical limit (
 ) of the Schrödinger equation
) of the Schrödinger equationSchrödinger equation
The Schrödinger equation was formulated in 1926 by Austrian physicist Erwin Schrödinger. Used in physics , it is an equation that describes how the quantum state of a physical system changes in time....
above becomes identical to the following variant of the Hamilton–Jacobi equation,
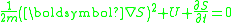
The Hamilton–Jacobi equation in the gravitational field
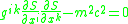
where
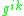 are the contravariant coordinates of the metric tensor
are the contravariant coordinates of the metric tensorMetric tensor
In the mathematical field of differential geometry, a metric tensor is a type of function defined on a manifold which takes as input a pair of tangent vectors v and w and produces a real number g in a way that generalizes many of the familiar properties of the dot product of vectors in Euclidean...
, m is the rest mass of the particle and c is the speed of light
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
.
See also
- Hamilton's principal function
- Canonical transformationCanonical transformationIn Hamiltonian mechanics, a canonical transformation is a change of canonical coordinates → that preserves the form of Hamilton's equations , although it...
- Constant of motionConstant of motionIn mechanics, a constant of motion is a quantity that is conserved throughout the motion, imposing in effect a constraint on the motion. However, it is a mathematical constraint, the natural consequence of the equations of motion, rather than a physical constraint...
- Hamiltonian vector fieldHamiltonian vector fieldIn mathematics and physics, a Hamiltonian vector field on a symplectic manifold is a vector field, defined for any energy function or Hamiltonian. Named after the physicist and mathematician Sir William Rowan Hamilton, a Hamiltonian vector field is a geometric manifestation of Hamilton's equations...
- Hamilton–Jacobi–Bellman equation in control theory
- WKB approximationWKB approximationIn mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear partial differential equations with spatially varying coefficients...
- William Rowan HamiltonWilliam Rowan HamiltonSir William Rowan Hamilton was an Irish physicist, astronomer, and mathematician, who made important contributions to classical mechanics, optics, and algebra. His studies of mechanical and optical systems led him to discover new mathematical concepts and techniques...
- Carl Gustav Jacob Jacobi
- Action-angle coordinatesAction-angle coordinatesIn classical mechanics, action-angle coordinates are a set of canonical coordinates useful in solving many integrable systems. The method of action-angles is useful for obtaining the frequencies of oscillatory or rotational motion without solving the equations of motion. Action-angle coordinates...

