Kaluza–Klein theory
Encyclopedia
In physics
, Kaluza–Klein theory (KK theory) is a model that seeks to unify the two fundamental forces
of gravitation
and electromagnetism
. The theory was first published in 1921. It was proposed by the mathematician Theodor Kaluza
who extended general relativity
to a five-dimensional spacetime. The resulting equations can be separated into further sets of equations, one of which is equivalent to Einstein field equations
, another set equivalent to Maxwell's equations
for the electromagnetic field
and the final part an extra scalar field
now termed the "radion".
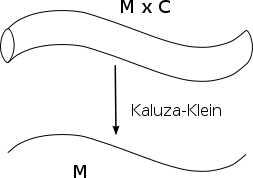A splitting of five-dimensional spacetime
into the Einstein equations and Maxwell equations in four dimensions was first discovered by Gunnar Nordström
in 1914, in the context of his theory
of gravity, but subsequently forgotten. Kaluza published his derivation in 1921 as an attempt to unify electromagnetism with Einstein's general relativity.
In 1926, Oskar Klein
proposed that the fourth spatial dimension is curled up in a circle
of very small radius
, so that a particle
moving a short distance along that axis would return to where it began. The distance a particle can travel before reaching its initial position is said to be the size of the dimension. This extra dimension is a compact set, and the phenomenon of having a space-time with compact dimensions is referred to as compactification
.
In modern geometry, the extra fifth dimension can be understood to be the circle group U(1), as electromagnetism
can essentially be formulated as a gauge theory
on a fiber bundle
, the circle bundle, with gauge group U(1). Once this geometrical interpretation is understood, it is relatively straightforward to replace U(1) by a general Lie group
. Such generalizations are often called Yang–Mills theories. If a distinction is drawn, then it is that Yang–Mills theories occur on a flat space-time, whereas Kaluza–Klein treats the more general case of curved spacetime. The base space of Kaluza–Klein theory need not be four-dimensional space-time; it can be any (pseudo-
)Riemannian manifold
, or even a supersymmetric
manifold or orbifold
or even a noncommutative space.
As an approach to the unification of the forces, it is straightforward to apply the Kaluza–Klein theory in an attempt to unify gravity with the strong and electroweak forces by using the symmetry group of the Standard Model
, SU(3) × SU(2) × U(1). However, an attempt to convert this interesting geometrical construction into a bona-fide model of reality flounders on a number of issues, including the fact that the fermion
s must be introduced in an artificial way (in nonsupersymmetric models). Nonetheless, KK remains an important touchstone
in theoretical physics and is often embedded in more sophisticated theories. It is studied in its own right as an object of geometric interest in K-theory
.
Even in the absence of a completely satisfying theoretical physics framework, the idea of exploring extra, compactified, dimensions is of considerable interest in the experimental physics
and astrophysics
communities. A variety of predictions, with real experimental consequences, can be made (in the case of large extra dimension
s/warped models). For example, on the simplest of principles, one might expect to have standing wave
s in the extra compactified dimension(s). If a spatial extra dimension is of radius R, the invariant mass
of such standing waves would be
with n an integer
, h being Planck's constant and c the speed of light
. This set of possible mass values is often called the Kaluza–Klein tower. Similarly, in Thermal quantum field theory
a compactification of the euclidean time dimension leads to the Matsubara frequencies and thus to a discretized thermal energy spectrum.
Examples of experimental pursuits include work by the CDF
collaboration, which has re-analyzed particle collider data for the signature of effects associated with large extra dimensions/warped models.
Brandenberger and Vafa have speculated that in the early universe, cosmic inflation
causes three of the space dimensions to expand to cosmological size while the remaining dimensions of space remained microscopic.
-time
-matter
theory or induced matter theory, chiefly promulgated by Paul Wesson
and other members of the so-called Space-Time-Matter Consortium. In this version of the theory, it is noted that solutions to the equation
with
the five-dimensional Ricci curvature
, may be re-expressed so that in four dimensions, these solutions satisfy Einstein's equations
with the precise form of the
following from the Ricci-flat condition on the five-dimensional space. Since the energy-momentum tensor 
is normally understood to be due to concentrations of matter in four-dimensional space, the above result is interpreted as saying that four-dimensional matter is induced from geometry in five-dimensional space.
In particular, the soliton
solutions of
can be shown to contain the Friedmann-Lemaitre-Robertson-Walker metric in both radiation-dominated (early universe) and matter-dominated (later universe) forms. The general equations can be shown to be sufficiently consistent with classical tests of general relativity
to be acceptable on physical principles, while still leaving considerable freedom to also provide interesting cosmological models.
, by applying the variational principle
to a certain action
. Let M be a (pseudo-
)Riemannian manifold
, which may be taken as the spacetime
of general relativity
. If g is the metric
on this manifold, one defines the action
as
where R(g) is the scalar curvature
and vol(g) is the volume element
. By applying the variational principle
to the action
one obtains precisely the Einstein equations for free space:
Here,
is the Ricci tensor.
can be understood to be the Hodge equations
of a principal U(1)-bundle
or circle bundle
with fiber U(1). That is, the electromagnetic field
F is a harmonic 2-form in the space
of differentiable 2-forms on the manifold M. In the absence of charges and currents, the free-field Maxwell equations are
and 
where
is the Hodge star.
that is the fiber of the U(1)-bundle of electromagnetism. In this discussion, an invariant metric is simply one that is invariant under rotations of the circle. Suppose this metric gives the circle a total length of 
. One then considers metrics 
on the bundle P that are consistent with both the fiber metric, and the metric on the underlying manifold M. The consistency conditions are:
The Kaluza–Klein action for such a metric is given by
The scalar curvature, written in components, then expands to
where
is the pullback of the fiber bundle projection 
. The connection A on the fiber bundle is related to the electromagnetic field strength as
That there always exists such a connection, even for fiber bundles of arbitrarily complex topology, is a result from homology
and specifically, K-theory
. Applying Fubini's theorem
and integrating on the fiber, one gets
Varying the action with respect to the component A, one regains the Maxwell equations. Applying the variational principle to the base metric g, one gets the Einstein equations
with the stress-energy tensor
being given by
,
sometimes called the Maxwell stress tensor
.
The original theory identifies
with the fiber metric 
, and allows 
to vary from fiber to fiber. In this case, the coupling between gravity and the electromagnetic field is not constant, but has its own dynamical field, the radion.
acts as a coupling constant between the gravitational field and the electromagnetic field. If the base manifold is four-dimensional, the Kaluza–Klein manifold P is five-dimensional. The fifth dimension is a compact space
, and is called the compact dimension. The technique of introducing compact dimensions to obtain a higher-dimensional manifold is referred to as compactification
. Compactification does not produce group actions on chiral fermions except in very specific cases: the dimension of the total space must be 2 mod 8 and the G-index of the Dirac operator of the compact space must be nonzero.
The above development generalizes in a more-or-less straightforward fashion to general principal G-bundles for some arbitrary Lie group
G taking the place of U(1). In such a case, the theory is often referred to as a Yang-Mills theory, and is sometimes taken to be synonymous. If the underlying manifold is supersymmetric, the resulting theory is a supersymmetric Yang–Mills theory.
have been officially reported. An analysis of results from the Large Hadron Collider
in December 2010 severely constrains theories with large extra dimensions.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, Kaluza–Klein theory (KK theory) is a model that seeks to unify the two fundamental forces
Fundamental interaction
In particle physics, fundamental interactions are the ways that elementary particles interact with one another...
of gravitation
Gravitation
Gravitation, or gravity, is a natural phenomenon by which physical bodies attract with a force proportional to their mass. Gravitation is most familiar as the agent that gives weight to objects with mass and causes them to fall to the ground when dropped...
and electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
. The theory was first published in 1921. It was proposed by the mathematician Theodor Kaluza
Theodor Kaluza
Theodor Franz Eduard Kaluza was a German mathematician and physicist known for the Kaluza-Klein theory involving field equations in five-dimensional space...
who extended general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
to a five-dimensional spacetime. The resulting equations can be separated into further sets of equations, one of which is equivalent to Einstein field equations
Einstein field equations
The Einstein field equations or Einstein's equations are a set of ten equations in Albert Einstein's general theory of relativity which describe the fundamental interaction of gravitation as a result of spacetime being curved by matter and energy...
, another set equivalent to Maxwell's equations
Maxwell's equations
Maxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
for the electromagnetic field
Electromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
and the final part an extra scalar field
Scalar field theory
In theoretical physics, scalar field theory can refer to a classical or quantum theory of scalar fields. A field which is invariant under any Lorentz transformation is called a "scalar", in contrast to a vector or tensor field...
now termed the "radion".
Overview
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
into the Einstein equations and Maxwell equations in four dimensions was first discovered by Gunnar Nordström
Gunnar Nordström
Gunnar Nordström was a Finnish theoretical physicist best remembered for his theory of gravitation, which was an early competitor of general relativity...
in 1914, in the context of his theory
Nordström's theory of gravitation
In theoretical physics, Nordström's theory of gravitation was a predecessor of general relativity. Strictly speaking, there were actually two distinct theories proposed by the Finnish theoretical physicist Gunnar Nordström, in 1912 and 1913 respectively...
of gravity, but subsequently forgotten. Kaluza published his derivation in 1921 as an attempt to unify electromagnetism with Einstein's general relativity.
In 1926, Oskar Klein
Oskar Klein
Oskar Benjamin Klein was a Swedish theoretical physicist.Klein was born in Danderyd outside Stockholm, son of the chief rabbi of Stockholm, Dr. Gottlieb Klein from Homonna in Hungary and Antonie Levy...
proposed that the fourth spatial dimension is curled up in a circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
of very small radius
Radius
In classical geometry, a radius of a circle or sphere is any line segment from its center to its perimeter. By extension, the radius of a circle or sphere is the length of any such segment, which is half the diameter. If the object does not have an obvious center, the term may refer to its...
, so that a particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
moving a short distance along that axis would return to where it began. The distance a particle can travel before reaching its initial position is said to be the size of the dimension. This extra dimension is a compact set, and the phenomenon of having a space-time with compact dimensions is referred to as compactification
Compactification (physics)
In physics, compactification means changing a theory with respect to one of its space-time dimensions. Instead of having a theory with this dimension being infinite, one changes the theory so that this dimension has a finite length, and may also be periodic....
.
In modern geometry, the extra fifth dimension can be understood to be the circle group U(1), as electromagnetism
Electromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
can essentially be formulated as a gauge theory
Gauge theory
In physics, gauge invariance is the property of a field theory in which different configurations of the underlying fundamental but unobservable fields result in identical observable quantities. A theory with such a property is called a gauge theory...
on a fiber bundle
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
, the circle bundle, with gauge group U(1). Once this geometrical interpretation is understood, it is relatively straightforward to replace U(1) by a general Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
. Such generalizations are often called Yang–Mills theories. If a distinction is drawn, then it is that Yang–Mills theories occur on a flat space-time, whereas Kaluza–Klein treats the more general case of curved spacetime. The base space of Kaluza–Klein theory need not be four-dimensional space-time; it can be any (pseudo-
Pseudo-Riemannian manifold
In differential geometry, a pseudo-Riemannian manifold is a generalization of a Riemannian manifold. It is one of many mathematical objects named after Bernhard Riemann. The key difference between a Riemannian manifold and a pseudo-Riemannian manifold is that on a pseudo-Riemannian manifold the...
)Riemannian manifold
Riemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
, or even a supersymmetric
Supersymmetry
In particle physics, supersymmetry is a symmetry that relates elementary particles of one spin to other particles that differ by half a unit of spin and are known as superpartners...
manifold or orbifold
Orbifold
In the mathematical disciplines of topology, geometry, and geometric group theory, an orbifold is a generalization of a manifold...
or even a noncommutative space.
As an approach to the unification of the forces, it is straightforward to apply the Kaluza–Klein theory in an attempt to unify gravity with the strong and electroweak forces by using the symmetry group of the Standard Model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
, SU(3) × SU(2) × U(1). However, an attempt to convert this interesting geometrical construction into a bona-fide model of reality flounders on a number of issues, including the fact that the fermion
Fermion
In particle physics, a fermion is any particle which obeys the Fermi–Dirac statistics . Fermions contrast with bosons which obey Bose–Einstein statistics....
s must be introduced in an artificial way (in nonsupersymmetric models). Nonetheless, KK remains an important touchstone
Touchstone (metaphor)
As a metaphor, a touchstone refers to any physical or intellectual measure by which the validity or merit of a concept can be tested. It is similar in use to an acid test, litmus test in politics, and a shibboleth.-Touchstone in literature:...
in theoretical physics and is often embedded in more sophisticated theories. It is studied in its own right as an object of geometric interest in K-theory
K-theory
In mathematics, K-theory originated as the study of a ring generated by vector bundles over a topological space or scheme. In algebraic topology, it is an extraordinary cohomology theory known as topological K-theory. In algebra and algebraic geometry, it is referred to as algebraic K-theory. It...
.
Even in the absence of a completely satisfying theoretical physics framework, the idea of exploring extra, compactified, dimensions is of considerable interest in the experimental physics
Experimental physics
Within the field of physics, experimental physics is the category of disciplines and sub-disciplines concerned with the observation of physical phenomena in order to gather data about the universe...
and astrophysics
Astrophysics
Astrophysics is the branch of astronomy that deals with the physics of the universe, including the physical properties of celestial objects, as well as their interactions and behavior...
communities. A variety of predictions, with real experimental consequences, can be made (in the case of large extra dimension
Large extra dimension
In particle physics, models with universal extra dimensions propose that there are one or more additional dimensions beyond the three spatial dimensions and one temporal dimension that are observed...
s/warped models). For example, on the simplest of principles, one might expect to have standing wave
Standing wave
In physics, a standing wave – also known as a stationary wave – is a wave that remains in a constant position.This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling...
s in the extra compactified dimension(s). If a spatial extra dimension is of radius R, the invariant mass
Mass
Mass can be defined as a quantitive measure of the resistance an object has to change in its velocity.In physics, mass commonly refers to any of the following three properties of matter, which have been shown experimentally to be equivalent:...
of such standing waves would be
with n an integerInteger
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
, h being Planck's constant and c the speed of light
Speed of light
The speed of light in vacuum, usually denoted by c, is a physical constant important in many areas of physics. Its value is 299,792,458 metres per second, a figure that is exact since the length of the metre is defined from this constant and the international standard for time...
. This set of possible mass values is often called the Kaluza–Klein tower. Similarly, in Thermal quantum field theory
Thermal quantum field theory
In theoretical physics, thermal quantum field theory or finite temperature field theory is a set of methods to calculate expectation values of physical observables of a quantum field theory at finite temperature....
a compactification of the euclidean time dimension leads to the Matsubara frequencies and thus to a discretized thermal energy spectrum.
Examples of experimental pursuits include work by the CDF
Collider Detector at Fermilab
The Collider Detector at Fermilab experimental collaboration studies high energy particle collisions at the Tevatron,the world's former highest-energy particle accelerator...
collaboration, which has re-analyzed particle collider data for the signature of effects associated with large extra dimensions/warped models.
Brandenberger and Vafa have speculated that in the early universe, cosmic inflation
Cosmic inflation
In physical cosmology, cosmic inflation, cosmological inflation or just inflation is the theorized extremely rapid exponential expansion of the early universe by a factor of at least 1078 in volume, driven by a negative-pressure vacuum energy density. The inflationary epoch comprises the first part...
causes three of the space dimensions to expand to cosmological size while the remaining dimensions of space remained microscopic.
Space-time-matter theory
One particular variant of Kaluza–Klein theory is spaceSpace
Space is the boundless, three-dimensional extent in which objects and events occur and have relative position and direction. Physical space is often conceived in three linear dimensions, although modern physicists usually consider it, with time, to be part of a boundless four-dimensional continuum...
-time
Time
Time is a part of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify rates of change such as the motions of objects....
-matter
Matter
Matter is a general term for the substance of which all physical objects consist. Typically, matter includes atoms and other particles which have mass. A common way of defining matter is as anything that has mass and occupies volume...
theory or induced matter theory, chiefly promulgated by Paul Wesson
Paul S. Wesson
Paul S. Wesson, B.Sc., Ph.D., D.Sc., F.R.A.S, is a professor of astrophysics and theoretical physics. He was educated at the Universities of London and Cambridge in England, and spent most of his career at the University of Waterloo in Canada...
and other members of the so-called Space-Time-Matter Consortium. In this version of the theory, it is noted that solutions to the equation
with
the five-dimensional Ricci curvatureRicci curvature
In differential geometry, the Ricci curvature tensor, named after Gregorio Ricci-Curbastro, represents the amount by which the volume element of a geodesic ball in a curved Riemannian manifold deviates from that of the standard ball in Euclidean space...
, may be re-expressed so that in four dimensions, these solutions satisfy Einstein's equations
with the precise form of the
following from the Ricci-flat condition on the five-dimensional space. Since the energy-momentum tensor is normally understood to be due to concentrations of matter in four-dimensional space, the above result is interpreted as saying that four-dimensional matter is induced from geometry in five-dimensional space.In particular, the soliton
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
solutions of
can be shown to contain the Friedmann-Lemaitre-Robertson-Walker metric in both radiation-dominated (early universe) and matter-dominated (later universe) forms. The general equations can be shown to be sufficiently consistent with classical tests of general relativityTests of general relativity
At its introduction in 1915, the general theory of relativity did not have a solid empirical foundation. It was known that it correctly accounted for the "anomalous" precession of the perihelion of Mercury and on philosophical grounds it was considered satisfying that it was able to unify Newton's...
to be acceptable on physical principles, while still leaving considerable freedom to also provide interesting cosmological models.
Geometric interpretation
The Kaluza–Klein theory is striking because it has a particularly elegant presentation in terms of geometry. In a certain sense, it looks just like ordinary gravity in free space, except that it is phrased in five dimensions instead of four.The Einstein equations
The equations governing ordinary gravity in free space can be obtained from an actionAction (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
, by applying the variational principle
Variational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
to a certain action
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
. Let M be a (pseudo-
Pseudo-Riemannian manifold
In differential geometry, a pseudo-Riemannian manifold is a generalization of a Riemannian manifold. It is one of many mathematical objects named after Bernhard Riemann. The key difference between a Riemannian manifold and a pseudo-Riemannian manifold is that on a pseudo-Riemannian manifold the...
)Riemannian manifold
Riemannian manifold
In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...
, which may be taken as the spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
of general relativity
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
. If g is the metric
Metric (mathematics)
In mathematics, a metric or distance function is a function which defines a distance between elements of a set. A set with a metric is called a metric space. A metric induces a topology on a set but not all topologies can be generated by a metric...
on this manifold, one defines the action
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
aswhere R(g) is the scalar curvature
Scalar curvature
In Riemannian geometry, the scalar curvature is the simplest curvature invariant of a Riemannian manifold. To each point on a Riemannian manifold, it assigns a single real number determined by the intrinsic geometry of the manifold near that point...
and vol(g) is the volume element
Volume element
In mathematics, a volume element provides a means for integrating a function with respect to volume in various coordinate systems such as spherical coordinates and cylindrical coordinates...
. By applying the variational principle
Variational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
to the action
one obtains precisely the Einstein equations for free space:
Here,
is the Ricci tensor.The Maxwell equations
By contrast, the Maxwell equations describing electromagnetismElectromagnetism
Electromagnetism is one of the four fundamental interactions in nature. The other three are the strong interaction, the weak interaction and gravitation...
can be understood to be the Hodge equations
De Rham cohomology
In mathematics, de Rham cohomology is a tool belonging both to algebraic topology and to differential topology, capable of expressing basic topological information about smooth manifolds in a form particularly adapted to computation and the concrete representation of cohomology classes...
of a principal U(1)-bundle
Principal bundle
In mathematics, a principal bundle is a mathematical object which formalizes some of the essential features of the Cartesian product X × G of a space X with a group G...
or circle bundle
with fiber U(1). That is, the electromagnetic fieldElectromagnetic field
An electromagnetic field is a physical field produced by moving electrically charged objects. It affects the behavior of charged objects in the vicinity of the field. The electromagnetic field extends indefinitely throughout space and describes the electromagnetic interaction...
F is a harmonic 2-form in the space
of differentiable 2-forms on the manifold M. In the absence of charges and currents, the free-field Maxwell equations are and where
is the Hodge star.The Kaluza–Klein geometry
To build the Kaluza–Klein theory, one picks an invariant metric on the circle that is the fiber of the U(1)-bundle of electromagnetism. In this discussion, an invariant metric is simply one that is invariant under rotations of the circle. Suppose this metric gives the circle a total length of . One then considers metrics on the bundle P that are consistent with both the fiber metric, and the metric on the underlying manifold M. The consistency conditions are:- The projection of
to the vertical subspaceVertical bundleThe vertical bundle of a smooth fiber bundle is the subbundle of the tangent bundle that consists of all vectors which are tangent to the fibers...
needs to agree with metric on the fiber over a point in the manifold M.
- The projection of
to the horizontal subspaceHorizontal bundleIn mathematics, in the field of differential topology, givena smooth fiber bundle over a smooth manifold M, then the vertical bundle VE of E is the subbundle of the tangent bundle TE consisting of the vectors which are tangent to the fibers of E over M...
of the tangent spaceTangent spaceIn mathematics, the tangent space of a manifold facilitates the generalization of vectors from affine spaces to general manifolds, since in the latter case one cannot simply subtract two points to obtain a vector pointing from one to the other....
at point
must be isomorphic to the metric g on M at 
.
The Kaluza–Klein action for such a metric is given by
The scalar curvature, written in components, then expands to
where
is the pullback of the fiber bundle projection . The connection A on the fiber bundle is related to the electromagnetic field strength asThat there always exists such a connection, even for fiber bundles of arbitrarily complex topology, is a result from homology
Homology (mathematics)
In mathematics , homology is a certain general procedure to associate a sequence of abelian groups or modules with a given mathematical object such as a topological space or a group...
and specifically, K-theory
K-theory
In mathematics, K-theory originated as the study of a ring generated by vector bundles over a topological space or scheme. In algebraic topology, it is an extraordinary cohomology theory known as topological K-theory. In algebra and algebraic geometry, it is referred to as algebraic K-theory. It...
. Applying Fubini's theorem
Fubini's theorem
In mathematical analysis Fubini's theorem, named after Guido Fubini, is a result which gives conditions under which it is possible to compute a double integral using iterated integrals. As a consequence it allows the order of integration to be changed in iterated integrals.-Theorem...
and integrating on the fiber, one gets
Varying the action with respect to the component A, one regains the Maxwell equations. Applying the variational principle to the base metric g, one gets the Einstein equations
with the stress-energy tensor
Stress-energy tensor
The stress–energy tensor is a tensor quantity in physics that describes the density and flux of energy and momentum in spacetime, generalizing the stress tensor of Newtonian physics. It is an attribute of matter, radiation, and non-gravitational force fields...
being given by
,sometimes called the Maxwell stress tensor
Maxwell stress tensor
The Maxwell Stress Tensor is a mathematical object in physics, more precisely it is a second rank tensor used in classical electromagnetism to represent the interaction between electric/magnetic forces and mechanical momentum...
.
The original theory identifies
with the fiber metric , and allows to vary from fiber to fiber. In this case, the coupling between gravity and the electromagnetic field is not constant, but has its own dynamical field, the radion.Commentary and generalizations
In the above, the size of the loop acts as a coupling constant between the gravitational field and the electromagnetic field. If the base manifold is four-dimensional, the Kaluza–Klein manifold P is five-dimensional. The fifth dimension is a compact spaceCompact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
, and is called the compact dimension. The technique of introducing compact dimensions to obtain a higher-dimensional manifold is referred to as compactification
Compactification (physics)
In physics, compactification means changing a theory with respect to one of its space-time dimensions. Instead of having a theory with this dimension being infinite, one changes the theory so that this dimension has a finite length, and may also be periodic....
. Compactification does not produce group actions on chiral fermions except in very specific cases: the dimension of the total space must be 2 mod 8 and the G-index of the Dirac operator of the compact space must be nonzero.
The above development generalizes in a more-or-less straightforward fashion to general principal G-bundles for some arbitrary Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
G taking the place of U(1). In such a case, the theory is often referred to as a Yang-Mills theory, and is sometimes taken to be synonymous. If the underlying manifold is supersymmetric, the resulting theory is a supersymmetric Yang–Mills theory.
Empirical tests
Up to now, no experimental or observational signs of extra dimensionsExtra dimensions
Several speculative physical theories have introduced extra dimensions of space for various reasons:*Kaluza-Klein theory introduces extra dimensions to explain the fundamental forces other than gravity ....
have been officially reported. An analysis of results from the Large Hadron Collider
Large Hadron Collider
The Large Hadron Collider is the world's largest and highest-energy particle accelerator. It is expected to address some of the most fundamental questions of physics, advancing the understanding of the deepest laws of nature....
in December 2010 severely constrains theories with large extra dimensions.
See also
- Classical theories of gravitationClassical theories of gravitationThe current Gold Standard Theory of Gravitation is the general theory of relativity. This is a classical, relativistic field theory of gravitation...
- DGP modelDGP modelThe DGP model is a model of gravity proposed by Gia Dvali, Gregory Gabadadze, and Massimo Porrati in 2000. The model is popular among some model builders, but has resisted being embedded into string theory....
- Randall-Sundrum modelRandall-Sundrum modelIn physics, Randall–Sundrum models imagine that the real world is a higher-dimensional Universe described by warped geometry...
- SupergravitySupergravityIn theoretical physics, supergravity is a field theory that combines the principles of supersymmetry and general relativity. Together, these imply that, in supergravity, the supersymmetry is a local symmetry...
- Superstring theorySuperstring theorySuperstring theory is an attempt to explain all of the particles and fundamental forces of nature in one theory by modelling them as vibrations of tiny supersymmetric strings...
- Why 10 dimensions?
- Quantum gravityQuantum gravityQuantum gravity is the field of theoretical physics which attempts to develop scientific models that unify quantum mechanics with general relativity...
Further reading
- Kaku, Michio and Robert O'Keefe. Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth DimensionHyperspace (book)Hyperspace: A Scientific Odyssey Through Parallel Universes, Time Warps, and the Tenth Dimension is a book by Michio Kaku, a theoretical physicist from the City College of New York. It focuses on Kaku's studies of higher dimensions referred to as hyperspace...
. New York: Oxford University PressOxford University PressOxford University Press is the largest university press in the world. It is a department of the University of Oxford and is governed by a group of 15 academics appointed by the Vice-Chancellor known as the Delegates of the Press. They are headed by the Secretary to the Delegates, who serves as...
, 1994. ISBN 0-19-286189-1 - The CDF Collaboration, Search for Extra Dimensions using Missing Energy at CDF, (2004) (A simplified presentation of the search made for extra dimensions at the Collider Detector at FermilabCollider Detector at FermilabThe Collider Detector at Fermilab experimental collaboration studies high energy particle collisions at the Tevatron,the world's former highest-energy particle accelerator...
(CDF) particle physics facility.) - John M. Pierre, SUPERSTRINGS! Extra Dimensions, (2003).
- TeV scale gravity, mirror universe, and ... dinosaurs Article from Acta Physica Polonica B by Z.K. Silagadze.
- Chris Pope, Lectures on Kaluza-Klein Theory.