Brunnian link
Encyclopedia
In knot theory
, a branch of mathematics
, a Brunnian link is a nontrivial link
that becomes trivial if any component is removed. In other words, cutting any loop frees all the other loops (so that no two loops can be directly linked
).
The name Brunnian is after Hermann Brunn
. Brunn's 1892 article Über Verkettung included examples of such links.
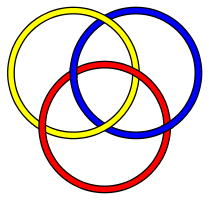The best-known and simplest possible Brunnian link is the Borromean rings
, a link of three unknot
s. However for every number three or above, there are an infinite number of links with the Brunnian property containing that number of loops. Here are some relatively simple three-component Brunnian links which are not the same as the Borromean rings:
An example of a n-component Brunnian link is given by the "Rubberband" Brunnian Links, where each component is looped around the next as aba−1b−1, with the last looping around the first, forming a circle.
in , and the invariants he introduced are now called Milnor invariants.
A (n + 1)-component Brunnian link can be thought of as an element of the link group
– which in this case (but not in general) is the fundamental group
of the link complement – of the n-component unlink, since by Brunnianness removing the last link unlinks the others. The link group of the n-component unlink is the free group
on n generators, Fn, as the link group of a single link is the knot group
of the unknot
, which is the integers, and the link group of an unlinked union is the free product
of the link groups of the components.
Not every element of the link group gives a Brunnian link, as removing any other component must also unlink the remaining n elements. Milnor showed that the group elements that do correspond to Brunnian links are related to the graded Lie algebra
of the lower central series of the free group, which can be interpreted as "relations" in the free Lie algebra
.
via Massey product
s: a Massey product is an n-fold product which is only defined if all (n − 1)-fold products of its terms vanish. This corresponds to the Brunnian property of all (n − 1)-component sublinks being unlinked, but the overall n-component link being non-trivially linked.
A Brunnian braid
is a braid that becomes trivial upon removal of any one of its strings. Brunnian braids form a subgroup
of the braid group
. Brunnian braids over the 2-sphere
that are not Brunnian over the 2-disk
give rise to non-trivial elements in the homotopy groups of the 2-sphere. For example, the "standard" braid corresponding to the Borromean rings gives rise to the Hopf fibration S3 → S2, and iterations of this (as in everyday braiding) is likewise Brunnian.
s and some mechanical puzzles are variants of Brunnian Links, with the goal being to free a single piece only partially linked to the rest, thus dismantling the structure.
Knot theory
In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...
, a branch of mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a Brunnian link is a nontrivial link
Link (knot theory)
In mathematics, a link is a collection of knots which do not intersect, but which may be linked together. A knot can be described as a link with one component. Links and knots are studied in a branch of mathematics called knot theory...
that becomes trivial if any component is removed. In other words, cutting any loop frees all the other loops (so that no two loops can be directly linked
Hopf link
thumb|right|[[Skein relation]] for the Hopf link.In mathematical knot theory, the Hopf link, named after Heinz Hopf, is the simplest nontrivial link with more than one component. It consists of two circles linked together exactly once...
).
The name Brunnian is after Hermann Brunn
Hermann Brunn
Karl Hermann Brunn was a German mathematician, known for his work in convex geometry and in knot theory .-Life and work:...
. Brunn's 1892 article Über Verkettung included examples of such links.
Examples
Borromean rings
In mathematics, the Borromean rings consist of three topological circles which are linked and form a Brunnian link, i.e., removing any ring results in two unlinked rings.- Mathematical properties :...
, a link of three unknot
Unknot
The unknot arises in the mathematical theory of knots. Intuitively, the unknot is a closed loop of rope without a knot in it. A knot theorist would describe the unknot as an image of any embedding that can be deformed, i.e. ambient-isotoped, to the standard unknot, i.e. the embedding of the...
s. However for every number three or above, there are an infinite number of links with the Brunnian property containing that number of loops. Here are some relatively simple three-component Brunnian links which are not the same as the Borromean rings:
An example of a n-component Brunnian link is given by the "Rubberband" Brunnian Links, where each component is looped around the next as aba−1b−1, with the last looping around the first, forming a circle.
Classification
Brunnian links were classified by John MilnorJohn Milnor
John Willard Milnor is an American mathematician known for his work in differential topology, K-theory and dynamical systems. He won the Fields Medal in 1962, the Wolf Prize in 1989, and the Abel Prize in 2011. Milnor is a distinguished professor at Stony Brook University...
in , and the invariants he introduced are now called Milnor invariants.
A (n + 1)-component Brunnian link can be thought of as an element of the link group
Link group
In knot theory, an area of mathematics, the link group of a link is an analog of the knot group of a knot. They were described by John Milnor in his Bachelor's thesis, .- Definition :...
– which in this case (but not in general) is the fundamental group
Fundamental group
In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
of the link complement – of the n-component unlink, since by Brunnianness removing the last link unlinks the others. The link group of the n-component unlink is the free group
Free group
In mathematics, a group G is called free if there is a subset S of G such that any element of G can be written in one and only one way as a product of finitely many elements of S and their inverses...
on n generators, Fn, as the link group of a single link is the knot group
Knot group
In mathematics, a knot is an embedding of a circle into 3-dimensional Euclidean space. The knot group of a knot K is defined as the fundamental group of the knot complement of K in R3,\pi_1....
of the unknot
Unknot
The unknot arises in the mathematical theory of knots. Intuitively, the unknot is a closed loop of rope without a knot in it. A knot theorist would describe the unknot as an image of any embedding that can be deformed, i.e. ambient-isotoped, to the standard unknot, i.e. the embedding of the...
, which is the integers, and the link group of an unlinked union is the free product
Free product
In mathematics, specifically group theory, the free product is an operation that takes two groups G and H and constructs a new group G ∗ H. The result contains both G and H as subgroups, is generated by the elements of these subgroups, and is the “most general” group having these properties...
of the link groups of the components.
Not every element of the link group gives a Brunnian link, as removing any other component must also unlink the remaining n elements. Milnor showed that the group elements that do correspond to Brunnian links are related to the graded Lie algebra
Graded Lie algebra
In mathematics, a graded Lie algebra is a Lie algebra endowed with a gradation which is compatible with the Lie bracket. In other words, a graded Lie algebra is a Lie algebra which is also a nonassociative graded algebra under the bracket operation. A choice of Cartan decomposition endows any...
of the lower central series of the free group, which can be interpreted as "relations" in the free Lie algebra
Free Lie algebra
In mathematics, a free Lie algebra, over a given field K, is a Lie algebra generated by a set X, without any imposed relations.-Definition:Given a set X, one can show that there exists a unique free Lie algebra L generated by X....
.
Massey products
Brunnian links can be understood in algebraic topologyAlgebraic topology
Algebraic topology is a branch of mathematics which uses tools from abstract algebra to study topological spaces. The basic goal is to find algebraic invariants that classify topological spaces up to homeomorphism, though usually most classify up to homotopy equivalence.Although algebraic topology...
via Massey product
Massey product
In algebraic topology, the Massey product is a cohomology operation of higher order introduced in , which generalizes the cup product.-Massey triple product:...
s: a Massey product is an n-fold product which is only defined if all (n − 1)-fold products of its terms vanish. This corresponds to the Brunnian property of all (n − 1)-component sublinks being unlinked, but the overall n-component link being non-trivially linked.
Brunnian braids
Braid theory
In topology, a branch of mathematics, braid theory is an abstract geometric theory studying the everyday braid concept, and some generalizations. The idea is that braids can be organized into groups, in which the group operation is 'do the first braid on a set of strings, and then follow it with a...
is a braid that becomes trivial upon removal of any one of its strings. Brunnian braids form a subgroup
Subgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
of the braid group
Braid group
In mathematics, the braid group on n strands, denoted by Bn, is a group which has an intuitive geometrical representation, and in a sense generalizes the symmetric group Sn. Here, n is a natural number; if n > 1, then Bn is an infinite group...
. Brunnian braids over the 2-sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
that are not Brunnian over the 2-disk
Disk (mathematics)
In geometry, a disk is the region in a plane bounded by a circle.A disk is said to be closed or open according to whether or not it contains the circle that constitutes its boundary...
give rise to non-trivial elements in the homotopy groups of the 2-sphere. For example, the "standard" braid corresponding to the Borromean rings gives rise to the Hopf fibration S3 → S2, and iterations of this (as in everyday braiding) is likewise Brunnian.
Real world examples
Many disentanglement puzzleDisentanglement puzzle
A disentanglement puzzle is a type of mechanical puzzle that involves disentangling one piece or set of pieces from another piece or set of pieces. The reverse problem of reassembling the puzzle can be as hard as—or even harder than—disentanglement...
s and some mechanical puzzles are variants of Brunnian Links, with the goal being to free a single piece only partially linked to the rest, thus dismantling the structure.
External links
- "Are Borromean Links so Rare?", by Slavik Jablan (also available in its original form as published in the journal Forma here (PDF file)).
- Brunnian link at The Knot Atlas