Braid theory - AbsoluteAstronomy.com

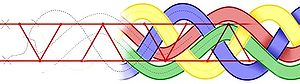
Topology

Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...

, a branch of mathematics, braid theory is an abstract geometric

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

theory

Theory

The English word theory was derived from a technical term in Ancient Greek philosophy. The word theoria, , meant "a looking at, viewing, beholding", and referring to contemplation or speculation, as opposed to action...

studying the everyday braid

Braid

A braid is a complex structure or pattern formed by intertwining three or more strands of flexible material such as textile fibres, wire, or human hair...

concept, and some generalizations. The idea is that braids can be organized into group

Group (mathematics)

In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...

s, in which the group operation is 'do the first braid on a set of strings, and then follow it with a second on the twisted strings'. Such groups may be described by explicit presentation

Presentation of a group

In mathematics, one method of defining a group is by a presentation. One specifies a set S of generators so that every element of the group can be written as a product of powers of some of these generators, and a set R of relations among those generators...

s, as was shown by . For an elementary treatment along these lines, see the article on braid group

Braid group

In mathematics, the braid group on n strands, denoted by Bn, is a group which has an intuitive geometrical representation, and in a sense generalizes the symmetric group Sn. Here, n is a natural number; if n > 1, then Bn is an infinite group...

s. Braid groups may also be given a deeper mathematical interpretation: as the fundamental group

Fundamental group

In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...

of certain configuration space

Configuration space

- Configuration space in physics :In classical mechanics, the configuration space is the space of possible positions that a physical system may attain, possibly subject to external constraints...

Braids as fundamental groups

To explain how to reduce a braid group in the sense of Artin to a fundamental group, we consider a connected manifold

Manifold

In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....

X of dimension at least 2. The symmetric product of n copies of X means the quotient of Xⁿ, the n-fold Cartesian product

Cartesian product

In mathematics, a Cartesian product is a construction to build a new set out of a number of given sets. Each member of the Cartesian product corresponds to the selection of one element each in every one of those sets...

of X with itself, by the permutation action of the symmetric group

Symmetric group

In mathematics, the symmetric group Sn on a finite set of n symbols is the group whose elements are all the permutations of the n symbols, and whose group operation is the composition of such permutations, which are treated as bijective functions from the set of symbols to itself...

on n letters operating on the indices of coordinates. That is, an ordered n-tuple is in the same orbit as any other that is a re-ordered version of it.

A path in the n-fold symmetric product is the abstract way of discussing n points of X, considered as an unordered n-tuple, independently tracing out n strings. Since we must require that the strings never pass through each other, it is necessary that we pass to the subspace Y of the symmetric product, of orbits of n-tuples of distinct points. That is, we remove all the subspaces of Xⁿ defined by conditions x_i = x_j. This is invariant under the symmetric group, and Y is the quotient by the symmetric group of the non-excluded n-tuples. Under the dimension condition Y will be connected.

With this definition, then, we can call the braid group
Braid group
In mathematics, the braid group on n strands, denoted by Bn, is a group which has an intuitive geometrical representation, and in a sense generalizes the symmetric group Sn. Here, n is a natural number; if n > 1, then Bn is an infinite group...

of X with n strings the fundamental group of Y (for any choice of base point – this is well-defined up to

Up to

In mathematics, the phrase "up to x" means "disregarding a possible difference in x".For instance, when calculating an indefinite integral, one could say that the solution is f "up to addition by a constant," meaning it differs from f, if at all, only by some constant.It indicates that...

isomorphism). The case where X is the Euclidean plane is the original one of Artin. In some cases it can be shown that the higher homotopy group

Homotopy group

In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, which records information about loops in a space...

s of Y are trivial.

Closed braids

When X is the plane, the braid can be closed, i.e., corresponding ends can be connected in pairs, to form a link

Link (knot theory)

In mathematics, a link is a collection of knots which do not intersect, but which may be linked together. A knot can be described as a link with one component. Links and knots are studied in a branch of mathematics called knot theory...

, i.e., a possibly intertwined union of possibly knotted loops in three dimensions. The number of components of the link can be anything from 1 to n, depending on the permutation of strands determined by the link. A theorem of J. W. Alexander

James Waddell Alexander II

James Waddell Alexander II was a mathematician and topologist of the pre-World War II era and part of an influential Princeton topology elite, which included Oswald Veblen, Solomon Lefschetz, and others...

demonstrates that every link can be obtained in this way as the "closure" of a braid. Compare with string links.

Different braids can give rise to the same link, just as different crossing diagrams can give rise to the same knot

Knot theory

In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...

. describes two moves on braid diagrams that yield equivalence in the corresponding closed braids. A single-move version of Markov's theorem, was published by .

Vaughan Jones

Vaughan Jones

Sir Vaughan Frederick Randal Jones, KNZM, FRS, FRSNZ is a New Zealand mathematician, known for his work on von Neumann algebras, knot polynomials and conformal field theory. He was awarded a Fields Medal in 1990, and famously wore a New Zealand rugby jersey when he accepted the prize...

originally defined his polynomial as a braid invariant and then showed that it depended only on the class of the closed braid.

Applications

Braid theory has recently been applied to fluid mechanics

Fluid mechanics

Fluid mechanics is the study of fluids and the forces on them. Fluid mechanics can be divided into fluid statics, the study of fluids at rest; fluid kinematics, the study of fluids in motion; and fluid dynamics, the study of the effect of forces on fluid motion...

, specifically to the field of chaotic mixing

Chaotic mixing

In chaos theory and fluid dynamics, chaotic mixing is a processby which flow tracers develop into complex fractals under the actionof a time-varying fluid flow.The flow is characterized by an exponential growth of fluid filaments....

in fluid flows. The braiding of (2+1) dimensional space-time trajectories formed by motion of physical rods, periodic orbits or "ghost rods", and almost-invariant sets has been used to estimate the topological entropy

Topological entropy

In mathematics, the topological entropy of a topological dynamical system is a nonnegative real number that measures the complexity of the system. Topological entropy was first introduced in 1965 by Adler, Konheim and McAndrew. Their definition was modelled after the definition of the...

of several engineered and naturally occurring fluid systems, via the use of Nielsen–Thurston classification.

Braids as fundamental groups

Closed braids

Applications

See also