Orbital resonance
Encyclopedia
In celestial mechanics
, an orbital resonance occurs when two orbit
ing bodies exert a regular, periodic gravitational influence on each other, usually due to their orbital period
s being related by a ratio of two small integer
s. Orbital resonances greatly enhance the mutual gravitational influence of the bodies, i.e., their ability to alter or constrain each others' orbits. In most cases, this results in an unstable interaction, in which the bodies exchange momentum
and shift orbits until the resonance no longer exists. Under some circumstances, a resonant system can be stable and self correcting, so that the bodies remain in resonance. Examples are the 1:2:4 resonance of Jupiter
's moons Ganymede
, Europa
and Io
, and the 2:3 resonance between Pluto
and Neptune
. Unstable resonances with Saturn
's inner moons give rise to gaps in the rings of Saturn
. The special case of 1:1 resonance (between bodies with similar orbital radii) causes large Solar System
bodies to eject most other bodies sharing their orbits; this is part of the much more extensive process of clearing the neighbourhood
, an effect that is used in the current definition of a planet
.
Except as noted in the Laplace resonance figure (below), a resonance ratio in this article should be interpreted as the ratio of number of orbits completed in the same time interval, rather than as the ratio of orbital periods (which would be the inverse ratio). The 2:3 ratio above means Pluto completes 2 orbits in the time it takes Neptune to complete 3.
in the 17th century, the stability of the solar system has preoccupied many mathematicians, starting with Laplace
. The stable orbits that arise in a two-body approximation
ignore the influence of other bodies. The effect of these added interactions on the stability of the Solar System
is very small, but at first it was not known whether they might add up over longer periods to significantly change the orbital parameters and lead to a completely different configuration, or whether some other stabilising effects might maintain the configuration of the orbits of the planets.
It was Laplace who found the first answers explaining the remarkable dance of the Galilean moons (see below). It is fair to say that this general field of study has remained very active since then, with plenty more yet to be understood (e.g. how interactions of moonlets with particles of the rings of giant planets result in maintaining the rings).
A mean motion orbital resonance occurs when two bodies have periods of revolution
that are a simple integer ratio of each other. Depending on the details, this can either stabilize or destabilize the orbit.
Stabilization occurs when the two bodies move in such a synchronised fashion that they never closely approach. For instance:
Orbital resonances can also destabilize one of the orbits. For small bodies, destabilization is actually far more likely. For instance:
A Laplace resonance occurs when three or more orbiting bodies have a simple integer ratio between their orbital periods. For example, Jupiter's moons Ganymede
, Europa
and Io
are in a 1:2:4 orbital resonance. The extrasolar planet
s Gliese 876
e, Gliese 876
b and Gliese 876
c are also in a 1:2:4 orbital resonance (with periods of 124.3, 61.1 and 30.0 days).
A Lindblad resonance
drives spiral density waves
both in galaxies (where stars are subject to forcing
by the spiral arms themselves) and in Saturn's rings
(where ring particles are subject to forcing by Saturn's moons).
A secular resonance
occurs when the precession of two orbits is synchronised (usually a precession of the perihelion or ascending node). A small body in secular resonance with a much larger one (e.g. a planet
) will precess at the same rate as the large body. Over long times (a million years, or so) a secular resonance will change the eccentricity and inclination
of the small body.
Several prominent examples of secular resonance involve Saturn. A resonance between the precession of Saturn's rotational axis and that of Neptune's orbital axis (both of which have periods of about 1.87 million years) has been identified as the likely source of Saturn's large axial tilt
(26.7°). Initially, Saturn probably had a tilt closer to that of Jupiter (3.1°). The gradual depletion of the Kuiper belt would have decreased the precession rate of Neptune's orbit; eventually, the frequencies matched, and Saturn's axial precession was captured into the spin-orbit resonance, leading to an increase in Saturn's obliquity. (The angular momentum of Neptune's orbit is 104 times that of that of Saturn's spin, and thus dominates the interaction.)
The ν6 secular resonance between asteroid
s and Saturn
helps shape the asteroid belt. Asteroids which approach it have their eccentricity slowly increased until they become Mars-crossers, at which point they are usually ejected from the asteroid belt
by a close pass to Mars
. This resonance forms the inner and "side" boundaries of the main asteroid belt
around 2 AU
, and at inclination
s of about 20°.
The Titan Ringlet within Saturn's C Ring exemplifies another type of resonance in which the rate of apsidal precession of one orbit exactly matches the speed of revolution of another. The outer end of this eccentric ringlet always points towards Saturn's major moon Titan
.
A Kozai resonance occurs when the inclination and eccentricity of a perturbed
orbit oscillate synchronously (increasing eccentricity while decreasing inclination and vice versa). This resonance applies only to bodies on highly inclined orbits; as a consequence, such orbits tend to be unstable, since the growing eccentricity would result in small pericenters
, typically leading to a collision or (for large moons) destruction by tidal forces.
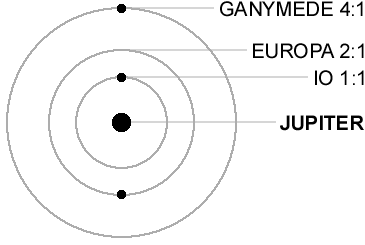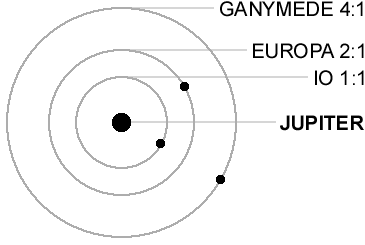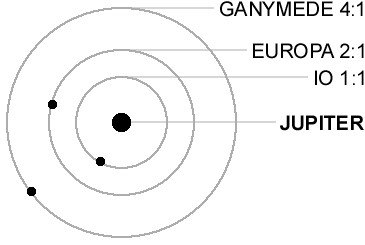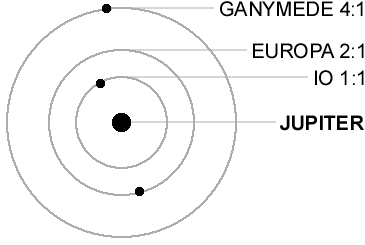There are only a few known mean motion resonances in the Solar System
involving planets, dwarf planets or larger satellite
s (a much greater number involve asteroid
s, smaller Kuiper belt
objects, planetary ring
s and moonlet
s).
The simple integer ratios between periods are a convenient simplification hiding more complex
relations:
As illustration of the latter, consider the well known 2:1 resonance of Io-Europa. If the orbiting periods were in this relation, the mean motion
s
(inverse of periods, often expressed in degrees per day) would satisfy the following
Substituting the data (from Wikipedia) one will get −0.7395° day−1, a value substantially different from zero!
Actually, the resonance is perfect but it involves also the precession of perijove (the point closest to Jupiter),
. The correct equation (part of the Laplace equations) is:
In other words, the mean motion of Io is indeed double of that of Europa taking into account the precession of the perijove. An observer sitting on the (drifting) perijove will see the moons coming into conjunction in the same place (elongation).
The other pairs listed above satisfy the same type of equation with the exception of Mimas-Tethys resonance. In this case, the resonance satisfies the equation
The point of conjunctions librates around the midpoint between the nodes
of the two moons.
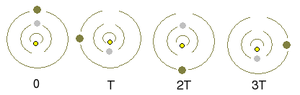The most remarkable resonance involving Io-Europa-Ganymede includes the following relation locking the orbital phase of the moons:
where
are mean longitude
s of the moons.
This relation makes a triple conjunction impossible. The graph illustrates the positions of the moons after 1, 2 and 3 Io periods. (The Laplace resonance in the Gliese 876
system, in contrast, is associated with one triple conjunction per orbit of the outermost planet.)
Pluto
is following an orbit trapped in a web of resonances with Neptune
. The resonances include:
One consequence of these resonances is that a separation of at least 30 AU is maintained when Pluto crosses Neptune's orbit. The minimum separation between the two bodies overall is 17 AU, while the minimum separation between Pluto and Uranus
is just 11 AU (see Pluto's orbit for detailed explanation and graphs).
The next largest body in a similar 2:3 resonance with Neptune is the candidate dwarf planet Orcus
. Orcus has an orbit similar in inclination and eccentricity to Pluto's. However, the two are constrained by their mutual resonance with Neptune to always be in opposite phases of their orbits; Orcus is thus sometimes described as the "anti-Pluto".
-ratio relationships between the orbital frequencies of the planets or major moons are sometimes pointed out (see list below). However, these have no dynamical significance because there is no appropriate precession of perihelion or other libration to make the resonance perfect (see the detailed discussion in the section above). Such near resonances are dynamically insignificant even if the mismatch is quite small because (unlike a true resonance), after each cycle the relative position of the bodies shifts. When averaged over astronomically short timescales, their relative position is random, just like bodies that are nowhere near resonance. For example, consider the orbits of Earth and Venus, which arrive at almost the same configuration after 8 Earth orbits and 13 Venus orbits. The actual ratio is 0.61518624, which is only 0.032% away from exactly 8:13. The mismatch after 8 years is only 1.5° of Venus' orbital movement. Still, this is enough that Venus and Earth find themselves in the opposite relative orientation to the original every 120 such cycles, which is 960 years. Therefore, on timescales of thousands of years or more (still tiny by astronomical standards), their relative position is effectively random.
The presence of a near resonance may reflect that a perfect resonance existed in the past, or that the system is evolving towards one in the future.
Some orbital frequency coincidences include:
The most remarkable (least probable) orbital correlation in the list is that between Callisto and Ganymede, followed in second place by that between Hydra and Charon.
The two near resonances listed for Earth and Venus are reflected in the timing of transits of Venus
, which occur in pairs 8 years apart, in a cycle that repeats every 243 years.
The near 1:12 resonance between Jupiter and Earth causes the Alinda asteroids
, which occupy (or are close to) the 3:1 resonance with Jupiter, to be close to a 1:4 resonance with Earth.
by Alessandro Morbidelli
of the Observatoire de la Côte d'Azur
in Nice
suggested that the formation of a 1:2 resonance between Jupiter and Saturn (due to interactions with planetesimals that caused them to migrate inward and outward, respectively) created a gravitational push that propelled both Uranus and Neptune into higher orbits, and in some scenarios caused them to switch places, which would have doubled Neptune's distance from the Sun. The resultant expulsion of objects from the proto-Kuiper belt as Neptune moved outwards could explain the Late Heavy Bombardment
600 million years after the Solar System's formation and the origin of Jupiter's Trojan asteroid
s. An outward migration of Neptune could also explain the current occupancy of some of its resonances (particularly the 2:5 resonance) within the Kuiper belt.
While Saturn's mid-sized moons Dione and Tethys are not close to an exact resonance now, they may have been in a 2:3 resonance early in the Solar System's history. This would have led to orbital eccentricity and tidal heating that may have warmed Tethys' interior enough to form a subsurface ocean. Subsequent freezing of the ocean after the moons escaped from the resonance may have generated the extensional stresses that created the enormous graben
system of Ithaca Chasma
on Tethys.
The satellite system of Uranus is notably different from those of Jupiter and Saturn in that it lacks precise resonances among the larger moons, while the majority of the larger moons of Jupiter (3 of the 4 largest) and of Saturn (6 of the 8 largest) are in mean motion resonances. In all three satellite systems, moons were likely captured into mean motion resonances in the past as their orbits shifted due to tidal dissipation
(a process by which satellites gain orbital energy at the expense of the primary's rotational energy, affecting inner moons disproportionately). In the Uranus System, however, due to the planet's lesser degree of oblateness, and the larger relative size of its satellites, escape from a mean motion resonance is much easier. Lower oblateness of the primary alters its gravitational field in such a way that different possible resonances are spaced more closely together. A larger relative satellite size increases the strength of their interactions. Both factors lead to more chaotic orbital behavior at or near mean motion resonances. Escape from a resonance may be associated with capture into a secondary resonance, and/or tidal evolution-driven increases in orbital eccentricity
or inclination
.
Mean motion resonances that probably once existed in the Uranus System include (3:5) Ariel-Miranda, (1:3) Umbriel-Miranda, (3:5) Umbriel-Ariel, and (1:4) Titania-Ariel. Evidence for such past resonances includes the relatively high eccentricities of the orbits of Uranus' inner satellites, and the anomalously high orbital inclination of Miranda. High past orbital eccentricities associated with the (1:3) Umbriel-Miranda and (1:4) Titania-Ariel resonances may have led to tidal heating of the interiors of Miranda and Ariel, respectively. Miranda probably escaped from its resonance with Umbriel via a secondary resonance, and the mechanism of this escape is believed to explain why its orbital inclination is more than 10 times those of the other regular
Uranian moons (see Uranus' natural satellites
).
In the case of Pluto
's satellites, it has been proposed that the present near resonances are relics of a previous precise resonance that was disrupted by tidal damping of the eccentricity of Charon's orbit (see Pluto's natural satellites
for details). The near resonances may be maintained by a 15% local fluctuation in the Pluto-Charon gravitational field. Thus, these near resonances may not be coincidental.
Plutoid Haumea's smaller inner moon Namaka
is a tenth the mass of Hiiaka
, orbiting Haumea in 18 days in a highly elliptical, non-Keplerian
orbit, and as of 2008 is inclined 13° from the larger moon, which perturbs
its orbit. Over the timescale of the system, it should have been tidally damped into a more circular orbit. It appears that it has been disturbed by resonances with the more massive Hiiaka, due to converging orbits as it moved outward from Haumea because of tidal dissipation. The moons may have been caught in and then escaped from orbital resonance several times; they currently are in or at least close to an 8:3 resonance. This strongly perturbs Namaka's orbit, with a current precession of ~20°.
Celestial mechanics
Celestial mechanics is the branch of astronomy that deals with the motions of celestial objects. The field applies principles of physics, historically classical mechanics, to astronomical objects such as stars and planets to produce ephemeris data. Orbital mechanics is a subfield which focuses on...
, an orbital resonance occurs when two orbit
Orbit
In physics, an orbit is the gravitationally curved path of an object around a point in space, for example the orbit of a planet around the center of a star system, such as the Solar System...
ing bodies exert a regular, periodic gravitational influence on each other, usually due to their orbital period
Orbital period
The orbital period is the time taken for a given object to make one complete orbit about another object.When mentioned without further qualification in astronomy this refers to the sidereal period of an astronomical object, which is calculated with respect to the stars.There are several kinds of...
s being related by a ratio of two small integer
Integer
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
s. Orbital resonances greatly enhance the mutual gravitational influence of the bodies, i.e., their ability to alter or constrain each others' orbits. In most cases, this results in an unstable interaction, in which the bodies exchange momentum
Momentum
In classical mechanics, linear momentum or translational momentum is the product of the mass and velocity of an object...
and shift orbits until the resonance no longer exists. Under some circumstances, a resonant system can be stable and self correcting, so that the bodies remain in resonance. Examples are the 1:2:4 resonance of Jupiter
Jupiter
Jupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,...
's moons Ganymede
Ganymede (moon)
Ganymede is a satellite of Jupiter and the largest moon in the Solar System. It is the seventh moon and third Galilean satellite outward from Jupiter. Completing an orbit in roughly seven days, Ganymede participates in a 1:2:4 orbital resonance with the moons Europa and Io, respectively...
, Europa
Europa (moon)
Europa Slightly smaller than Earth's Moon, Europa is primarily made of silicate rock and probably has an iron core. It has a tenuous atmosphere composed primarily of oxygen. Its surface is composed of ice and is one of the smoothest in the Solar System. This surface is striated by cracks and...
and Io
Io (moon)
Io ) is the innermost of the four Galilean moons of the planet Jupiter and, with a diameter of , the fourth-largest moon in the Solar System. It was named after the mythological character of Io, a priestess of Hera who became one of the lovers of Zeus....
, and the 2:3 resonance between Pluto
Pluto
Pluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
and Neptune
Neptune
Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times...
. Unstable resonances with Saturn
Saturn
Saturn is the sixth planet from the Sun and the second largest planet in the Solar System, after Jupiter. Saturn is named after the Roman god Saturn, equated to the Greek Cronus , the Babylonian Ninurta and the Hindu Shani. Saturn's astronomical symbol represents the Roman god's sickle.Saturn,...
's inner moons give rise to gaps in the rings of Saturn
Rings of Saturn
The rings of Saturn are the most extensive planetary ring system of any planet in the Solar System. They consist of countless small particles, ranging in size from micrometres to metres, that form clumps that in turn orbit about Saturn...
. The special case of 1:1 resonance (between bodies with similar orbital radii) causes large Solar System
Solar System
The Solar System consists of the Sun and the astronomical objects gravitationally bound in orbit around it, all of which formed from the collapse of a giant molecular cloud approximately 4.6 billion years ago. The vast majority of the system's mass is in the Sun...
bodies to eject most other bodies sharing their orbits; this is part of the much more extensive process of clearing the neighbourhood
Clearing the neighbourhood
"Clearing the neighbourhood of its orbit" is a criterion for a celestial body to be considered a planet in the Solar System. This was one of the three criteria adopted by the International Astronomical Union in its 2006 definition of planet....
, an effect that is used in the current definition of a planet
Definition of planet
The definition of planet, since the word was coined by the ancient Greeks, has included within its scope a wide range of celestial bodies. Greek astronomers employed the term asteres planetai , "wandering stars", for objects which apparently move over the sky...
.
Except as noted in the Laplace resonance figure (below), a resonance ratio in this article should be interpreted as the ratio of number of orbits completed in the same time interval, rather than as the ratio of orbital periods (which would be the inverse ratio). The 2:3 ratio above means Pluto completes 2 orbits in the time it takes Neptune to complete 3.
History
Since the discovery of Newton's law of universal gravitationNewton's law of universal gravitation
Newton's law of universal gravitation states that every point mass in the universe attracts every other point mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them...
in the 17th century, the stability of the solar system has preoccupied many mathematicians, starting with Laplace
Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace was a French mathematician and astronomer whose work was pivotal to the development of mathematical astronomy and statistics. He summarized and extended the work of his predecessors in his five volume Mécanique Céleste...
. The stable orbits that arise in a two-body approximation
N-body problem
The n-body problem is the problem of predicting the motion of a group of celestial objects that interact with each other gravitationally. Solving this problem has been motivated by the need to understand the motion of the Sun, planets and the visible stars...
ignore the influence of other bodies. The effect of these added interactions on the stability of the Solar System
Solar System
The Solar System consists of the Sun and the astronomical objects gravitationally bound in orbit around it, all of which formed from the collapse of a giant molecular cloud approximately 4.6 billion years ago. The vast majority of the system's mass is in the Sun...
is very small, but at first it was not known whether they might add up over longer periods to significantly change the orbital parameters and lead to a completely different configuration, or whether some other stabilising effects might maintain the configuration of the orbits of the planets.
It was Laplace who found the first answers explaining the remarkable dance of the Galilean moons (see below). It is fair to say that this general field of study has remained very active since then, with plenty more yet to be understood (e.g. how interactions of moonlets with particles of the rings of giant planets result in maintaining the rings).
Types of resonance
In general, an orbital resonance may- involve one or any combination of the orbit parameters (e.g. eccentricity versus semimajor axis, or eccentricity versus orbit inclination).
- act on any time scale from short term, commensurable with the orbit periods, to secularSecular variations of the planetary orbitsThe secular variations of the planetary orbits is a concept describing long-term changes in the orbits of the planets Mercury to Neptune. If one ignores the gravitational attraction between the planets and only models the attraction between the Sun and the planets, then with some further...
, measured in 104 to 106 years. - lead to either long term stabilization of the orbits or be the cause of their destabilization.
A mean motion orbital resonance occurs when two bodies have periods of revolution
Revolution
A revolution is a fundamental change in power or organizational structures that takes place in a relatively short period of time.Aristotle described two types of political revolution:...
that are a simple integer ratio of each other. Depending on the details, this can either stabilize or destabilize the orbit.
Stabilization occurs when the two bodies move in such a synchronised fashion that they never closely approach. For instance:
- The orbits of PlutoPlutoPluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
and the plutinoPlutinoIn astronomy, a plutino is a trans-Neptunian object in 2:3 mean motion resonance with Neptune. For every 2 orbits that a plutino makes, Neptune orbits 3 times. Plutinos are named after Pluto, which follows an orbit trapped in the same resonance, with the Italian diminutive suffix -ino...
s are stable, despite crossing that of much larger NeptuneNeptuneNeptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times...
, because they are in a 2:3 resonance with it. The resonance ensures that, when they approach perihelion and Neptune's orbit, Neptune is consistently distant (averaging a quarter of its orbit away). Other (much more numerous) Neptune-crossing bodies that were not in resonance were ejected from that region by strong perturbationPerturbation (astronomy)Perturbation is a term used in astronomy in connection with descriptions of the complex motion of a massive body which is subject to appreciable gravitational effects from more than one other massive body....
s due to Neptune. There are also smaller but significant groups of resonant trans-Neptunian objectResonant trans-Neptunian objectIn astronomy, a resonant trans-Neptunian object is a trans-Neptunian object in mean motion orbital resonance with Neptune. The orbital periods of the resonant objects are in a simple integer relations with the period of Neptune e.g. 1:2, 2:3 etc...
s occupying the 1:1 (Neptune trojanNeptune TrojanNeptune trojans are Kuiper belt object-like bodies in solar orbit that have the same orbital period as Neptune and follow roughly the same orbital path...
s), 3:5, 4:7, 1:2 (twotinos) and 2:5 resonances with respect to Neptune. - In the asteroid beltAsteroid beltThe asteroid belt is the region of the Solar System located roughly between the orbits of the planets Mars and Jupiter. It is occupied by numerous irregularly shaped bodies called asteroids or minor planets...
beyond 3.5 AU from the Sun, the 3:2, 4:3 and 1:1 resonances with JupiterJupiterJupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,...
are populated by clumps of asteroids (the Hilda familyHilda familyThe Hilda asteroids consists of asteroids with a semi-major axis between 3.7 AU and 4.2 AU, an eccentricity less than 0.3, and an inclination less than 20°. They do not form a true asteroid family, in the sense that they do not descend from a common parent object. Instead, this is a dynamical...
, 279 Thule279 Thule279 Thule is a large asteroid from the asteroid belt. It is classified as a D-type asteroid and is probably composed of organic-rich silicates, carbon and anhydrous silicates....
, and the Trojan asteroidTrojan asteroidThe Jupiter Trojans, commonly called Trojans or Trojan asteroids, are a large group of objects that share the orbit of the planet Jupiter around the Sun. Relative to Jupiter, each Trojan librates around one of the planet's two Lagrangian points of stability, and , that respectively lie 60° ahead...
s, respectively).
Orbital resonances can also destabilize one of the orbits. For small bodies, destabilization is actually far more likely. For instance:
- In the asteroid beltAsteroid beltThe asteroid belt is the region of the Solar System located roughly between the orbits of the planets Mars and Jupiter. It is occupied by numerous irregularly shaped bodies called asteroids or minor planets...
within 3.5 AU from the Sun, the major mean-motion resonances with JupiterJupiterJupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,...
are locations of gaps in the asteroid distribution, the Kirkwood gapKirkwood gapA Kirkwood gap is a gap or dip in the distribution of main-belt asteroids with semi-major axis , as seen in the histogram below...
s (most notably at the 3:1, 5:2, 7:3 and 2:1 resonances). AsteroidAsteroidAsteroids are a class of small Solar System bodies in orbit around the Sun. They have also been called planetoids, especially the larger ones...
s have been ejected from these almost empty lanes by repeated perturbations. However, there are still populations of asteroids temporarily present in or near these resonances. For example, asteroids of the Alinda familyAlinda familyThe Alinda asteroids are a group of asteroids with a semi-major axis of about 2.5 AU and an orbital eccentricity approximately between 0.4 and 0.65. The namesake is 887 Alinda, discovered by Max Wolf in 1918....
are in or close to the 3:1 resonance, with their orbital eccentricity steadily increased by interactions with Jupiter until they eventually have a close encounter with an inner planet that ejects them from the resonance. - In the rings of SaturnRings of SaturnThe rings of Saturn are the most extensive planetary ring system of any planet in the Solar System. They consist of countless small particles, ranging in size from micrometres to metres, that form clumps that in turn orbit about Saturn...
, the Cassini Division is a gap between the inner B Ring and the outer A Ring that has been cleared by a 2:1 resonance with the moon MimasMimas (moon)Mimas is a moon of Saturn which was discovered in 1789 by William Herschel. It is named after Mimas, a son of Gaia in Greek mythology, and is also designated Saturn I....
. (More specifically, the site of the resonance is the Huygens Gap, which bounds the outer edge of the B Ring.) - In the rings of Saturn, the Encke and Keeler gaps within the A Ring are cleared by 1:1 resonances with the embedded moonlets PanPan (moon)Pan is the innermost moon of Saturn. It is a walnut-shaped small moon about 35 kilometres across and 23 km high that orbits within the Encke Gap in Saturn's A Ring. Pan acts as a ring shepherd and is responsible for keeping the Encke Gap free of ring particles.It was discovered by Mark R...
and DaphnisDaphnis (moon)Daphnis is an inner satellite of Saturn. It is also known as '; its provisional designation was '. Daphnis is about 8 kilometres in diameter, and orbits the planet in the Keeler Gap within the A ring.- Naming :...
, respectively. The A Ring's outer edge is maintained by a destabilizing 7:6 resonance with the moon JanusJanus (moon)Janus is an inner satellite of Saturn. It is also known as Saturn X . It is named after the mythological Janus.-Discovery and orbit:Janus occupies practically the same orbit as the moon Epimetheus...
.
A Laplace resonance occurs when three or more orbiting bodies have a simple integer ratio between their orbital periods. For example, Jupiter's moons Ganymede
Ganymede (moon)
Ganymede is a satellite of Jupiter and the largest moon in the Solar System. It is the seventh moon and third Galilean satellite outward from Jupiter. Completing an orbit in roughly seven days, Ganymede participates in a 1:2:4 orbital resonance with the moons Europa and Io, respectively...
, Europa
Europa (moon)
Europa Slightly smaller than Earth's Moon, Europa is primarily made of silicate rock and probably has an iron core. It has a tenuous atmosphere composed primarily of oxygen. Its surface is composed of ice and is one of the smoothest in the Solar System. This surface is striated by cracks and...
and Io
Io (moon)
Io ) is the innermost of the four Galilean moons of the planet Jupiter and, with a diameter of , the fourth-largest moon in the Solar System. It was named after the mythological character of Io, a priestess of Hera who became one of the lovers of Zeus....
are in a 1:2:4 orbital resonance. The extrasolar planet
Extrasolar planet
An extrasolar planet, or exoplanet, is a planet outside the Solar System. A total of such planets have been identified as of . It is now known that a substantial fraction of stars have planets, including perhaps half of all Sun-like stars...
s Gliese 876
Gliese 876
Gliese 876 is a red dwarf star approximately 15 light-years away from Earth in the constellation of Aquarius. As of 2011, it has been confirmed that four extrasolar planets orbit the star...
e, Gliese 876
Gliese 876
Gliese 876 is a red dwarf star approximately 15 light-years away from Earth in the constellation of Aquarius. As of 2011, it has been confirmed that four extrasolar planets orbit the star...
b and Gliese 876
Gliese 876
Gliese 876 is a red dwarf star approximately 15 light-years away from Earth in the constellation of Aquarius. As of 2011, it has been confirmed that four extrasolar planets orbit the star...
c are also in a 1:2:4 orbital resonance (with periods of 124.3, 61.1 and 30.0 days).
A Lindblad resonance
Lindblad resonance
A Lindblad resonance, named for the Swedish galactic astronomer Bertil Lindblad, is an orbital resonance in which an object's epicyclic frequency is a simple multiple of some forcing frequency...
drives spiral density waves
Density wave theory
Density wave theory or the Lin-Shu density wave theory is a theory proposed by C.C. Lin and Frank Shu in the mid-1960s to explain spiral arm structure of spiral galaxies. Their theory introduces the idea of long-lived quasistatic density waves , which are sections of the galactic disk that have...
both in galaxies (where stars are subject to forcing
Harmonic oscillator
In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force, F, proportional to the displacement, x: \vec F = -k \vec x \, where k is a positive constant....
by the spiral arms themselves) and in Saturn's rings
Rings of Saturn
The rings of Saturn are the most extensive planetary ring system of any planet in the Solar System. They consist of countless small particles, ranging in size from micrometres to metres, that form clumps that in turn orbit about Saturn...
(where ring particles are subject to forcing by Saturn's moons).
A secular resonance
Secular resonance
A secular resonance is a type of orbital resonance.Secular resonances occur when the precession of two orbits is synchronised . A small body in secular resonance with a much larger one will precess at the same rate as the large body...
occurs when the precession of two orbits is synchronised (usually a precession of the perihelion or ascending node). A small body in secular resonance with a much larger one (e.g. a planet
Planet
A planet is a celestial body orbiting a star or stellar remnant that is massive enough to be rounded by its own gravity, is not massive enough to cause thermonuclear fusion, and has cleared its neighbouring region of planetesimals.The term planet is ancient, with ties to history, science,...
) will precess at the same rate as the large body. Over long times (a million years, or so) a secular resonance will change the eccentricity and inclination
Inclination
Inclination in general is the angle between a reference plane and another plane or axis of direction.-Orbits:The inclination is one of the six orbital parameters describing the shape and orientation of a celestial orbit...
of the small body.
Several prominent examples of secular resonance involve Saturn. A resonance between the precession of Saturn's rotational axis and that of Neptune's orbital axis (both of which have periods of about 1.87 million years) has been identified as the likely source of Saturn's large axial tilt
Axial tilt
In astronomy, axial tilt is the angle between an object's rotational axis, and a line perpendicular to its orbital plane...
(26.7°). Initially, Saturn probably had a tilt closer to that of Jupiter (3.1°). The gradual depletion of the Kuiper belt would have decreased the precession rate of Neptune's orbit; eventually, the frequencies matched, and Saturn's axial precession was captured into the spin-orbit resonance, leading to an increase in Saturn's obliquity. (The angular momentum of Neptune's orbit is 104 times that of that of Saturn's spin, and thus dominates the interaction.)
The ν6 secular resonance between asteroid
Asteroid
Asteroids are a class of small Solar System bodies in orbit around the Sun. They have also been called planetoids, especially the larger ones...
s and Saturn
Saturn
Saturn is the sixth planet from the Sun and the second largest planet in the Solar System, after Jupiter. Saturn is named after the Roman god Saturn, equated to the Greek Cronus , the Babylonian Ninurta and the Hindu Shani. Saturn's astronomical symbol represents the Roman god's sickle.Saturn,...
helps shape the asteroid belt. Asteroids which approach it have their eccentricity slowly increased until they become Mars-crossers, at which point they are usually ejected from the asteroid belt
Asteroid belt
The asteroid belt is the region of the Solar System located roughly between the orbits of the planets Mars and Jupiter. It is occupied by numerous irregularly shaped bodies called asteroids or minor planets...
by a close pass to Mars
Mars
Mars is the fourth planet from the Sun in the Solar System. The planet is named after the Roman god of war, Mars. It is often described as the "Red Planet", as the iron oxide prevalent on its surface gives it a reddish appearance...
. This resonance forms the inner and "side" boundaries of the main asteroid belt
Asteroid belt
The asteroid belt is the region of the Solar System located roughly between the orbits of the planets Mars and Jupiter. It is occupied by numerous irregularly shaped bodies called asteroids or minor planets...
around 2 AU
Astronomical unit
An astronomical unit is a unit of length equal to about or approximately the mean Earth–Sun distance....
, and at inclination
Inclination
Inclination in general is the angle between a reference plane and another plane or axis of direction.-Orbits:The inclination is one of the six orbital parameters describing the shape and orientation of a celestial orbit...
s of about 20°.
The Titan Ringlet within Saturn's C Ring exemplifies another type of resonance in which the rate of apsidal precession of one orbit exactly matches the speed of revolution of another. The outer end of this eccentric ringlet always points towards Saturn's major moon Titan
Titan (moon)
Titan , or Saturn VI, is the largest moon of Saturn, the only natural satellite known to have a dense atmosphere, and the only object other than Earth for which clear evidence of stable bodies of surface liquid has been found....
.
A Kozai resonance occurs when the inclination and eccentricity of a perturbed
Perturbation theory
Perturbation theory comprises mathematical methods that are used to find an approximate solution to a problem which cannot be solved exactly, by starting from the exact solution of a related problem...
orbit oscillate synchronously (increasing eccentricity while decreasing inclination and vice versa). This resonance applies only to bodies on highly inclined orbits; as a consequence, such orbits tend to be unstable, since the growing eccentricity would result in small pericenters
Apsis
An apsis , plural apsides , is the point of greatest or least distance of a body from one of the foci of its elliptical orbit. In modern celestial mechanics this focus is also the center of attraction, which is usually the center of mass of the system...
, typically leading to a collision or (for large moons) destruction by tidal forces.
Mean motion resonances in the Solar System
Solar System
The Solar System consists of the Sun and the astronomical objects gravitationally bound in orbit around it, all of which formed from the collapse of a giant molecular cloud approximately 4.6 billion years ago. The vast majority of the system's mass is in the Sun...
involving planets, dwarf planets or larger satellite
Natural satellite
A natural satellite or moon is a celestial body that orbits a planet or smaller body, which is called its primary. The two terms are used synonymously for non-artificial satellites of planets, of dwarf planets, and of minor planets....
s (a much greater number involve asteroid
Asteroid
Asteroids are a class of small Solar System bodies in orbit around the Sun. They have also been called planetoids, especially the larger ones...
s, smaller Kuiper belt
Kuiper belt
The Kuiper belt , sometimes called the Edgeworth–Kuiper belt, is a region of the Solar System beyond the planets extending from the orbit of Neptune to approximately 50 AU from the Sun. It is similar to the asteroid belt, although it is far larger—20 times as wide and 20 to 200 times as massive...
objects, planetary ring
Planetary ring
A planetary ring is a ring of cosmic dust and other small particles orbiting around a planet in a flat disc-shaped region.The most notable planetary rings known in Earth's solar system are those around Saturn, but the other three gas giants of the solar system possess ring systems of their...
s and moonlet
Inner satellite
In astronomy, an inner moon is a natural satellite following a prograde, low inclination orbit inwards of the large satellites of the parent planet. They are generally thought to have been formed in situ at the same time as the coalescence of the original planet...
s).
- 2:3 PlutoPlutoPluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
-NeptuneNeptuneNeptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times... - 2:4 TethysTethys (moon)Tethys or Saturn III is a mid-sized moon of Saturn about across. It was discovered by G. D. Cassini in 1684 and is named after titan Tethys of Greek mythology. Tethys is pronounced |Odysseus]] is about 400 km in diameter, while the largest graben—Ithaca Chasma is about 100 km wide and...
-MimasMimas (moon)Mimas is a moon of Saturn which was discovered in 1789 by William Herschel. It is named after Mimas, a son of Gaia in Greek mythology, and is also designated Saturn I....
(Saturn’s moons) - 1:2 DioneDione (moon)Dione is a moon of Saturn discovered by Cassini in 1684. It is named after the titan Dione of Greek mythology. It is also designated Saturn IV.- Name :...
-EnceladusEnceladus (moon)Enceladus is the sixth-largest of the moons of Saturn. It was discovered in 1789 by William Herschel. Until the two Voyager spacecraft passed near it in the early 1980s very little was known about this small moon besides the identification of water ice on its surface...
(Saturn’s moons) - 3:4 HyperionHyperion (moon)Hyperion , also known as Saturn VII, is a moon of Saturn discovered by William Cranch Bond, George Phillips Bond and William Lassell in 1848. It is distinguished by its irregular shape, its chaotic rotation, and its unexplained sponge-like appearance...
-TitanTitan (moon)Titan , or Saturn VI, is the largest moon of Saturn, the only natural satellite known to have a dense atmosphere, and the only object other than Earth for which clear evidence of stable bodies of surface liquid has been found....
(Saturn's moons) - 1:2:4 GanymedeGanymede (moon)Ganymede is a satellite of Jupiter and the largest moon in the Solar System. It is the seventh moon and third Galilean satellite outward from Jupiter. Completing an orbit in roughly seven days, Ganymede participates in a 1:2:4 orbital resonance with the moons Europa and Io, respectively...
-EuropaEuropa (moon)Europa Slightly smaller than Earth's Moon, Europa is primarily made of silicate rock and probably has an iron core. It has a tenuous atmosphere composed primarily of oxygen. Its surface is composed of ice and is one of the smoothest in the Solar System. This surface is striated by cracks and...
-IoIo (moon)Io ) is the innermost of the four Galilean moons of the planet Jupiter and, with a diameter of , the fourth-largest moon in the Solar System. It was named after the mythological character of Io, a priestess of Hera who became one of the lovers of Zeus....
(Jupiter’s moons).
The simple integer ratios between periods are a convenient simplification hiding more complex
relations:
- the point of conjunction can oscillate (librateLibrationIn astronomy, libration is an oscillating motion of orbiting bodies relative to each other, notably including the motion of the Moon relative to Earth, or of Trojan asteroids relative to planets.-Lunar libration:...
) around an equilibrium point defined by the resonance. - given non-zero eccentricities, the nodeOrbital nodeAn orbital node is one of the two points where an orbit crosses a plane of reference to which it is inclined. An orbit which is contained in the plane of reference has no nodes.-Planes of reference:...
s or periapsides can drift (a resonance related, short period, not secular precession).
As illustration of the latter, consider the well known 2:1 resonance of Io-Europa. If the orbiting periods were in this relation, the mean motion
Mean motion
Mean motion, n\,\!, is a measure of how fast a satellite progresses around its elliptical orbit. Unless the orbit is circular, the mean motion is only an average value, and does not represent the instantaneous angular rate....
s
(inverse of periods, often expressed in degrees per day) would satisfy the following
Substituting the data (from Wikipedia) one will get −0.7395° day−1, a value substantially different from zero!
Actually, the resonance is perfect but it involves also the precession of perijove (the point closest to Jupiter),
. The correct equation (part of the Laplace equations) is:
In other words, the mean motion of Io is indeed double of that of Europa taking into account the precession of the perijove. An observer sitting on the (drifting) perijove will see the moons coming into conjunction in the same place (elongation).
The other pairs listed above satisfy the same type of equation with the exception of Mimas-Tethys resonance. In this case, the resonance satisfies the equation
The point of conjunctions librates around the midpoint between the nodes
Orbital node
An orbital node is one of the two points where an orbit crosses a plane of reference to which it is inclined. An orbit which is contained in the plane of reference has no nodes.-Planes of reference:...
of the two moons.
The Laplace resonance
where
are mean longitudeMean longitude
In astrodynamics or celestial dynamics, mean longitude is the longitude at which an orbiting body could be found if its orbit were circular, and free of perturbations, and if its inclination were zero. Both the mean longitude and the true longitude of the body in such an orbit would change at a...
s of the moons.
This relation makes a triple conjunction impossible. The graph illustrates the positions of the moons after 1, 2 and 3 Io periods. (The Laplace resonance in the Gliese 876
Gliese 876
Gliese 876 is a red dwarf star approximately 15 light-years away from Earth in the constellation of Aquarius. As of 2011, it has been confirmed that four extrasolar planets orbit the star...
system, in contrast, is associated with one triple conjunction per orbit of the outermost planet.)
Pluto resonances
The dwarf planetDwarf planet
A dwarf planet, as defined by the International Astronomical Union , is a celestial body orbiting the Sun that is massive enough to be spherical as a result of its own gravity but has not cleared its neighboring region of planetesimals and is not a satellite...
Pluto
Pluto
Pluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
is following an orbit trapped in a web of resonances with Neptune
Neptune
Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times...
. The resonances include:
- A mean motion resonance of 2:3
- The resonance of the perihelion (libration around 90°), keeping the perihelion above the eclipticEclipticThe ecliptic is the plane of the earth's orbit around the sun. In more accurate terms, it is the intersection of the celestial sphere with the ecliptic plane, which is the geometric plane containing the mean orbit of the Earth around the Sun...
- The resonance of the longitude of the perihelion in relation to that of Neptune
One consequence of these resonances is that a separation of at least 30 AU is maintained when Pluto crosses Neptune's orbit. The minimum separation between the two bodies overall is 17 AU, while the minimum separation between Pluto and Uranus
Uranus
Uranus is the seventh planet from the Sun. It has the third-largest planetary radius and fourth-largest planetary mass in the Solar System. It is named after the ancient Greek deity of the sky Uranus , the father of Cronus and grandfather of Zeus...
is just 11 AU (see Pluto's orbit for detailed explanation and graphs).
The next largest body in a similar 2:3 resonance with Neptune is the candidate dwarf planet Orcus
90482 Orcus
90482 Orcus is a trans-Neptunian object in the Kuiper belt with a large moon. It was discovered on February 17, 2004 by Michael Brown of Caltech, Chad Trujillo of the Gemini Observatory, and David Rabinowitz of Yale University. Precovery images as early as November 8, 1951 were later identified...
. Orcus has an orbit similar in inclination and eccentricity to Pluto's. However, the two are constrained by their mutual resonance with Neptune to always be in opposite phases of their orbits; Orcus is thus sometimes described as the "anti-Pluto".
Coincidental 'near' ratios of mean motion
A number of near-integerInteger
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
-ratio relationships between the orbital frequencies of the planets or major moons are sometimes pointed out (see list below). However, these have no dynamical significance because there is no appropriate precession of perihelion or other libration to make the resonance perfect (see the detailed discussion in the section above). Such near resonances are dynamically insignificant even if the mismatch is quite small because (unlike a true resonance), after each cycle the relative position of the bodies shifts. When averaged over astronomically short timescales, their relative position is random, just like bodies that are nowhere near resonance. For example, consider the orbits of Earth and Venus, which arrive at almost the same configuration after 8 Earth orbits and 13 Venus orbits. The actual ratio is 0.61518624, which is only 0.032% away from exactly 8:13. The mismatch after 8 years is only 1.5° of Venus' orbital movement. Still, this is enough that Venus and Earth find themselves in the opposite relative orientation to the original every 120 such cycles, which is 960 years. Therefore, on timescales of thousands of years or more (still tiny by astronomical standards), their relative position is effectively random.
The presence of a near resonance may reflect that a perfect resonance existed in the past, or that the system is evolving towards one in the future.
Some orbital frequency coincidences include:
| (Ratio) and Bodies | Mismatch after one cycle | Randomization time | Probability |
|---|---|---|---|
| Planets | |||
| (9:23) Venus Venus Venus is the second planet from the Sun, orbiting it every 224.7 Earth days. The planet is named after Venus, the Roman goddess of love and beauty. After the Moon, it is the brightest natural object in the night sky, reaching an apparent magnitude of −4.6, bright enough to cast shadows... − Mercury Mercury (planet) Mercury is the innermost and smallest planet in the Solar System, orbiting the Sun once every 87.969 Earth days. The orbit of Mercury has the highest eccentricity of all the Solar System planets, and it has the smallest axial tilt. It completes three rotations about its axis for every two orbits... |
4.0° | 200 y Year A year is the orbital period of the Earth moving around the Sun. For an observer on Earth, this corresponds to the period it takes the Sun to complete one course throughout the zodiac along the ecliptic.... |
0.19 |
| (8:13) Earth Earth Earth is the third planet from the Sun, and the densest and fifth-largest of the eight planets in the Solar System. It is also the largest of the Solar System's four terrestrial planets... − Venus Venus Venus is the second planet from the Sun, orbiting it every 224.7 Earth days. The planet is named after Venus, the Roman goddess of love and beauty. After the Moon, it is the brightest natural object in the night sky, reaching an apparent magnitude of −4.6, bright enough to cast shadows... |
1.5° | 1000 y Year A year is the orbital period of the Earth moving around the Sun. For an observer on Earth, this corresponds to the period it takes the Sun to complete one course throughout the zodiac along the ecliptic.... |
0.065 |
| (243:395) Earth Earth Earth is the third planet from the Sun, and the densest and fifth-largest of the eight planets in the Solar System. It is also the largest of the Solar System's four terrestrial planets... − Venus Venus Venus is the second planet from the Sun, orbiting it every 224.7 Earth days. The planet is named after Venus, the Roman goddess of love and beauty. After the Moon, it is the brightest natural object in the night sky, reaching an apparent magnitude of −4.6, bright enough to cast shadows... |
0.8° | 50,000 y Year A year is the orbital period of the Earth moving around the Sun. For an observer on Earth, this corresponds to the period it takes the Sun to complete one course throughout the zodiac along the ecliptic.... |
0.68 |
| (1:3) Mars Mars Mars is the fourth planet from the Sun in the Solar System. The planet is named after the Roman god of war, Mars. It is often described as the "Red Planet", as the iron oxide prevalent on its surface gives it a reddish appearance... − Venus Venus Venus is the second planet from the Sun, orbiting it every 224.7 Earth days. The planet is named after Venus, the Roman goddess of love and beauty. After the Moon, it is the brightest natural object in the night sky, reaching an apparent magnitude of −4.6, bright enough to cast shadows... |
20.6° | 20 y | 0.11 |
| (1:2) Mars Mars Mars is the fourth planet from the Sun in the Solar System. The planet is named after the Roman god of war, Mars. It is often described as the "Red Planet", as the iron oxide prevalent on its surface gives it a reddish appearance... − Earth Earth Earth is the third planet from the Sun, and the densest and fifth-largest of the eight planets in the Solar System. It is also the largest of the Solar System's four terrestrial planets... |
42.9° | 8 y | 0.24 |
| (1:12) Jupiter Jupiter Jupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,... − Earth Earth Earth is the third planet from the Sun, and the densest and fifth-largest of the eight planets in the Solar System. It is also the largest of the Solar System's four terrestrial planets... |
49.1° | 40 y | 0.27 |
| (2:5) Saturn Saturn Saturn is the sixth planet from the Sun and the second largest planet in the Solar System, after Jupiter. Saturn is named after the Roman god Saturn, equated to the Greek Cronus , the Babylonian Ninurta and the Hindu Shani. Saturn's astronomical symbol represents the Roman god's sickle.Saturn,... − Jupiter Jupiter Jupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,... |
12.8° | 800 y | 0.14 |
| (1:7) Uranus Uranus Uranus is the seventh planet from the Sun. It has the third-largest planetary radius and fourth-largest planetary mass in the Solar System. It is named after the ancient Greek deity of the sky Uranus , the father of Cronus and grandfather of Zeus... − Jupiter Jupiter Jupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,... |
31.1° | 500 y | 0.17 |
| (7:20) Uranus Uranus Uranus is the seventh planet from the Sun. It has the third-largest planetary radius and fourth-largest planetary mass in the Solar System. It is named after the ancient Greek deity of the sky Uranus , the father of Cronus and grandfather of Zeus... − Saturn Saturn Saturn is the sixth planet from the Sun and the second largest planet in the Solar System, after Jupiter. Saturn is named after the Roman god Saturn, equated to the Greek Cronus , the Babylonian Ninurta and the Hindu Shani. Saturn's astronomical symbol represents the Roman god's sickle.Saturn,... |
5.7° | 20,000 y | 0.20 |
| (5:28) Neptune Neptune Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times... − Saturn Saturn Saturn is the sixth planet from the Sun and the second largest planet in the Solar System, after Jupiter. Saturn is named after the Roman god Saturn, equated to the Greek Cronus , the Babylonian Ninurta and the Hindu Shani. Saturn's astronomical symbol represents the Roman god's sickle.Saturn,... |
1.9° | 80,000 y | 0.052 |
| (1:2) Neptune Neptune Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times... − Uranus Uranus Uranus is the seventh planet from the Sun. It has the third-largest planetary radius and fourth-largest planetary mass in the Solar System. It is named after the ancient Greek deity of the sky Uranus , the father of Cronus and grandfather of Zeus... |
14.0° | 2000 y | 0.078 |
| Mars System | |||
| (1:4) Deimos Deimos (moon) Deimos is the smaller and outer of Mars's two moons . It is named after Deimos, a figure representing dread in Greek Mythology. Its systematic designation is '.-Discovery:Deimos was discovered by Asaph Hall, Sr... − Phobos Phobos (moon) Phobos is the larger and closer of the two natural satellites of Mars. Both moons were discovered in 1877. With a mean radius of , Phobos is 7.24 times as massive as Deimos... |
14.9° | 0.04 y | 0.083 |
| Jupiter System | |||
| (3:7) Callisto Callisto (moon) Callisto named after the Greek mythological figure of Callisto) is a moon of the planet Jupiter. It was discovered in 1610 by Galileo Galilei. It is the third-largest moon in the Solar System and the second largest in the Jovian system, after Ganymede. Callisto has about 99% the diameter of the... − Ganymede Ganymede (moon) Ganymede is a satellite of Jupiter and the largest moon in the Solar System. It is the seventh moon and third Galilean satellite outward from Jupiter. Completing an orbit in roughly seven days, Ganymede participates in a 1:2:4 orbital resonance with the moons Europa and Io, respectively... |
0.7° | 30 y | 0.012 |
| Saturn System | |||
| (2:3) Enceladus Enceladus (moon) Enceladus is the sixth-largest of the moons of Saturn. It was discovered in 1789 by William Herschel. Until the two Voyager spacecraft passed near it in the early 1980s very little was known about this small moon besides the identification of water ice on its surface... − Mimas Mimas (moon) Mimas is a moon of Saturn which was discovered in 1789 by William Herschel. It is named after Mimas, a son of Gaia in Greek mythology, and is also designated Saturn I.... |
33.2° | 0.04 y | 0.33 |
| (2:3) Dione Dione (moon) Dione is a moon of Saturn discovered by Cassini in 1684. It is named after the titan Dione of Greek mythology. It is also designated Saturn IV.- Name :... − Tethys Tethys (moon) Tethys or Saturn III is a mid-sized moon of Saturn about across. It was discovered by G. D. Cassini in 1684 and is named after titan Tethys of Greek mythology. Tethys is pronounced |Odysseus]] is about 400 km in diameter, while the largest graben—Ithaca Chasma is about 100 km wide and... |
36.2° | 0.07 y | 0.36 |
| (3:5) Rhea Rhea (moon) Rhea is the second-largest moon of Saturn and the ninth largest moon in the Solar System. It was discovered in 1672 by Giovanni Domenico Cassini.-Name:Rhea is named after the Titan Rhea of Greek mythology, "mother of the gods"... − Dione Dione (moon) Dione is a moon of Saturn discovered by Cassini in 1684. It is named after the titan Dione of Greek mythology. It is also designated Saturn IV.- Name :... |
17.1° | 0.4 y | 0.26 |
| (2:7) Titan Titan (moon) Titan , or Saturn VI, is the largest moon of Saturn, the only natural satellite known to have a dense atmosphere, and the only object other than Earth for which clear evidence of stable bodies of surface liquid has been found.... − Rhea Rhea (moon) Rhea is the second-largest moon of Saturn and the ninth largest moon in the Solar System. It was discovered in 1672 by Giovanni Domenico Cassini.-Name:Rhea is named after the Titan Rhea of Greek mythology, "mother of the gods"... |
21.0° | 0.7 y | 0.22 |
| (1:5) Iapetus Iapetus (moon) Iapetus ), occasionally Japetus , is the third-largest moon of Saturn, and eleventh in the Solar System. It was discovered by Giovanni Domenico Cassini in 1671... − Titan Titan (moon) Titan , or Saturn VI, is the largest moon of Saturn, the only natural satellite known to have a dense atmosphere, and the only object other than Earth for which clear evidence of stable bodies of surface liquid has been found.... |
9.2° | 4.0 y | 0.051 |
| Uranus System | |||
| (1:3) Umbriel Umbriel (moon) Umbriel is a moon of Uranus discovered on October 24, 1851, by William Lassell. It was discovered at the same time as Ariel and named after a character in Alexander Pope's poem The Rape of the Lock. Umbriel consists mainly of ice with a substantial fraction of rock, and may be differentiated into a... − Miranda Miranda (moon) -External links:* at * at The Nine8 Planets* at Views of the Solar System* * from the... |
24.5° | 0.08 y | 0.14 |
| (3:5) Umbriel Umbriel (moon) Umbriel is a moon of Uranus discovered on October 24, 1851, by William Lassell. It was discovered at the same time as Ariel and named after a character in Alexander Pope's poem The Rape of the Lock. Umbriel consists mainly of ice with a substantial fraction of rock, and may be differentiated into a... − Ariel Ariel (moon) Ariel is the brightest and fourth-largest of the 27 known moons of Uranus. Ariel orbits and rotates in the equatorial plane of Uranus, which is almost perpendicular to the orbit of Uranus, and so has an extreme seasonal cycle.... |
24.2° | 0.3 y | 0.35 |
| (1:2) Titania Titania (moon) Titania is the largest of the moons of Uranus and the eighth largest moon in the Solar System at a diameter of 1578 km. Discovered by William Herschel in 1787, Titania is named after the queen of the fairies in Shakespeare's A Midsummer Night's Dream... − Umbriel Umbriel (moon) Umbriel is a moon of Uranus discovered on October 24, 1851, by William Lassell. It was discovered at the same time as Ariel and named after a character in Alexander Pope's poem The Rape of the Lock. Umbriel consists mainly of ice with a substantial fraction of rock, and may be differentiated into a... |
36.3° | 0.1 y | 0.20 |
| (2:3) Oberon Oberon (moon) Oberon , also designated ', is the outermost major moon of the planet Uranus. It is the second largest and second most massive of the Uranian moons, and the ninth most massive moon in the Solar System. Discovered by William Herschel in 1787, Oberon is named after the mythical king of the fairies... − Titania Titania (moon) Titania is the largest of the moons of Uranus and the eighth largest moon in the Solar System at a diameter of 1578 km. Discovered by William Herschel in 1787, Titania is named after the queen of the fairies in Shakespeare's A Midsummer Night's Dream... |
33.4° | 0.4 y | 0.34 |
| Pluto System | |||
| (1:4) Nix Nix (moon) Nix is a natural satellite of Pluto. It was discovered along with Hydra in June 2005, and is to be visited along with Pluto by the New Horizons mission in July 2015.- Discovery :... − Charon Charon (moon) Charon is the largest satellite of the dwarf planet Pluto. It was discovered in 1978 at the United States Naval Observatory Flagstaff Station. Following the 2005 discovery of two other natural satellites of Pluto , Charon may also be referred to as Pluto I... |
39.1° | 0.3 y | 0.22 |
| (1:5) P4 S/2011 P 1 S/2011 P 1 is a small natural satellite of Pluto whose existence was announced on July 20, 2011... − Charon Charon (moon) Charon is the largest satellite of the dwarf planet Pluto. It was discovered in 1978 at the United States Naval Observatory Flagstaff Station. Following the 2005 discovery of two other natural satellites of Pluto , Charon may also be referred to as Pluto I... |
? | ? | ? |
| (1:6) Hydra Hydra (moon) Hydra is the second outermost known natural satellite of Pluto. It was discovered along with Nix in June 2005, and is to be visited along with Pluto by the New Horizons mission in July 2015.- Discovery :... − Charon Charon (moon) Charon is the largest satellite of the dwarf planet Pluto. It was discovered in 1978 at the United States Naval Observatory Flagstaff Station. Following the 2005 discovery of two other natural satellites of Pluto , Charon may also be referred to as Pluto I... |
6.6° | 3.0 y | 0.037 |
The most remarkable (least probable) orbital correlation in the list is that between Callisto and Ganymede, followed in second place by that between Hydra and Charon.
The two near resonances listed for Earth and Venus are reflected in the timing of transits of Venus
Transit of Venus
A transit of Venus across the Sun takes place when the planet Venus passes directly between the Sun and Earth, becoming visible against the solar disk. During a transit, Venus can be seen from Earth as a small black disk moving across the face of the Sun...
, which occur in pairs 8 years apart, in a cycle that repeats every 243 years.
The near 1:12 resonance between Jupiter and Earth causes the Alinda asteroids
Alinda family
The Alinda asteroids are a group of asteroids with a semi-major axis of about 2.5 AU and an orbital eccentricity approximately between 0.4 and 0.65. The namesake is 887 Alinda, discovered by Max Wolf in 1918....
, which occupy (or are close to) the 3:1 resonance with Jupiter, to be close to a 1:4 resonance with Earth.
Possible past mean motion resonances
A past resonance between Jupiter and Saturn may have played a dramatic role in early Solar System history. A 2004 computer modelNice model
The Nice model is a scenario for the dynamical evolution of the Solar System. It is named for the location of the Observatoire de la Côte d'Azur, where it was initially developed, in Nice, France. It proposes the migration of the giant planets from an initial compact configuration into their...
by Alessandro Morbidelli
Alessandro Morbidelli (astronomer)
Alessandro Morbidelli, born May 2, 1966, is an Italian astronomer and planetary scientist currently employed by the Observatoire de la Cote d'Azur in Nice....
of the Observatoire de la Côte d'Azur
Côte d'Azur Observatory
The Observatoire de la Côte d'Azur originated in 1988 with the merger of two observatories:# Observatoire de Nice# The CERGA - External links :*...
in Nice
Nice
Nice is the fifth most populous city in France, after Paris, Marseille, Lyon and Toulouse, with a population of 348,721 within its administrative limits on a land area of . The urban area of Nice extends beyond the administrative city limits with a population of more than 955,000 on an area of...
suggested that the formation of a 1:2 resonance between Jupiter and Saturn (due to interactions with planetesimals that caused them to migrate inward and outward, respectively) created a gravitational push that propelled both Uranus and Neptune into higher orbits, and in some scenarios caused them to switch places, which would have doubled Neptune's distance from the Sun. The resultant expulsion of objects from the proto-Kuiper belt as Neptune moved outwards could explain the Late Heavy Bombardment
Late Heavy Bombardment
The Late Heavy Bombardment is a period of time approximately 4.1 to 3.8 billion years ago during which a large number of impact craters are believed to have formed on the Moon, and by inference on Earth, Mercury, Venus, and Mars as well...
600 million years after the Solar System's formation and the origin of Jupiter's Trojan asteroid
Trojan asteroid
The Jupiter Trojans, commonly called Trojans or Trojan asteroids, are a large group of objects that share the orbit of the planet Jupiter around the Sun. Relative to Jupiter, each Trojan librates around one of the planet's two Lagrangian points of stability, and , that respectively lie 60° ahead...
s. An outward migration of Neptune could also explain the current occupancy of some of its resonances (particularly the 2:5 resonance) within the Kuiper belt.
While Saturn's mid-sized moons Dione and Tethys are not close to an exact resonance now, they may have been in a 2:3 resonance early in the Solar System's history. This would have led to orbital eccentricity and tidal heating that may have warmed Tethys' interior enough to form a subsurface ocean. Subsequent freezing of the ocean after the moons escaped from the resonance may have generated the extensional stresses that created the enormous graben
Graben
In geology, a graben is a depressed block of land bordered by parallel faults. Graben is German for ditch. Graben is used for both the singular and plural....
system of Ithaca Chasma
Ithaca Chasma
Ithaca Chasma is a valley on Saturn's moon Tethys, named after the island of Ithaca, in Greece. It is on average 100 km wide, 3 to 5 km deep and 2,000 km long, running approximately three-quarters of the way around Tethys' circumference, making it one of the longer valleys in the...
on Tethys.
The satellite system of Uranus is notably different from those of Jupiter and Saturn in that it lacks precise resonances among the larger moons, while the majority of the larger moons of Jupiter (3 of the 4 largest) and of Saturn (6 of the 8 largest) are in mean motion resonances. In all three satellite systems, moons were likely captured into mean motion resonances in the past as their orbits shifted due to tidal dissipation
Tidal acceleration
Tidal acceleration is an effect of the tidal forces between an orbiting natural satellite , and the primary planet that it orbits . The "acceleration" is usually negative, as it causes a gradual slowing and recession of a satellite in a prograde orbit away from the primary, and a corresponding...
(a process by which satellites gain orbital energy at the expense of the primary's rotational energy, affecting inner moons disproportionately). In the Uranus System, however, due to the planet's lesser degree of oblateness, and the larger relative size of its satellites, escape from a mean motion resonance is much easier. Lower oblateness of the primary alters its gravitational field in such a way that different possible resonances are spaced more closely together. A larger relative satellite size increases the strength of their interactions. Both factors lead to more chaotic orbital behavior at or near mean motion resonances. Escape from a resonance may be associated with capture into a secondary resonance, and/or tidal evolution-driven increases in orbital eccentricity
Orbital eccentricity
The orbital eccentricity of an astronomical body is the amount by which its orbit deviates from a perfect circle, where 0 is perfectly circular, and 1.0 is a parabola, and no longer a closed orbit...
or inclination
Inclination
Inclination in general is the angle between a reference plane and another plane or axis of direction.-Orbits:The inclination is one of the six orbital parameters describing the shape and orientation of a celestial orbit...
.
Mean motion resonances that probably once existed in the Uranus System include (3:5) Ariel-Miranda, (1:3) Umbriel-Miranda, (3:5) Umbriel-Ariel, and (1:4) Titania-Ariel. Evidence for such past resonances includes the relatively high eccentricities of the orbits of Uranus' inner satellites, and the anomalously high orbital inclination of Miranda. High past orbital eccentricities associated with the (1:3) Umbriel-Miranda and (1:4) Titania-Ariel resonances may have led to tidal heating of the interiors of Miranda and Ariel, respectively. Miranda probably escaped from its resonance with Umbriel via a secondary resonance, and the mechanism of this escape is believed to explain why its orbital inclination is more than 10 times those of the other regular
Regular moon
In astronomy, a regular moon is a natural satellite following a relatively close and generally prograde orbit with little orbital inclination or eccentricity...
Uranian moons (see Uranus' natural satellites
Uranus' natural satellites
Uranus, the seventh planet of the Solar System, has 27 known moons, all of which are named after characters from the works of William Shakespeare and Alexander Pope. William Herschel discovered the first two moons, Titania and Oberon, in 1787, and the other spherical moons were discovered in 1851...
).
In the case of Pluto
Pluto
Pluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
's satellites, it has been proposed that the present near resonances are relics of a previous precise resonance that was disrupted by tidal damping of the eccentricity of Charon's orbit (see Pluto's natural satellites
Pluto's natural satellites
Pluto has four known moons. The largest, Charon, is proportionally larger, compared to its primary, than any other satellite of a known planet or dwarf planet in the Solar System. The other moons, Nix, Hydra, and S/2011 P 1 are much smaller...
for details). The near resonances may be maintained by a 15% local fluctuation in the Pluto-Charon gravitational field. Thus, these near resonances may not be coincidental.
Plutoid Haumea's smaller inner moon Namaka
Namaka (moon)
Namaka is the smaller, inner moon of the dwarf planet Haumea. It is named after Nāmaka, one of the daughters of Haumea, the goddess of the sea in Hawaiian mythology.- Discovery :Namaka was discovered on 30 June 2005 and announced on November 29, 2005...
is a tenth the mass of Hiiaka
Hi'iaka (moon)
Hiiaka is the larger, outer moon of the dwarf planet Haumea.- Discovery and naming :Hiiaka was the first satellite discovered around Haumea. It is named after one of the daughters of Haumea, Hiiaka, the patron goddess of the Big Island of Hawaii, though at first it had gone by the nickname...
, orbiting Haumea in 18 days in a highly elliptical, non-Keplerian
Osculating orbit
In astronomy, and in particular in astrodynamics, the osculating orbit of an object in space is the gravitational Kepler orbit In astronomy, and in particular in astrodynamics, the osculating orbit of an object in space (at a given moment of time) is the gravitational Kepler orbit In astronomy,...
orbit, and as of 2008 is inclined 13° from the larger moon, which perturbs
Perturbation (astronomy)
Perturbation is a term used in astronomy in connection with descriptions of the complex motion of a massive body which is subject to appreciable gravitational effects from more than one other massive body....
its orbit. Over the timescale of the system, it should have been tidally damped into a more circular orbit. It appears that it has been disturbed by resonances with the more massive Hiiaka, due to converging orbits as it moved outward from Haumea because of tidal dissipation. The moons may have been caught in and then escaped from orbital resonance several times; they currently are in or at least close to an 8:3 resonance. This strongly perturbs Namaka's orbit, with a current precession of ~20°.
See also
- 3753 Cruithne3753 Cruithne3753 Cruithne is an asteroid in orbit around the Sun in approximate 1:1 orbital resonance with the Earth. It is a periodic inclusion planetoid orbiting the Sun in an apparent horseshoe orbit. It has been incorrectly called "Earth's second moon", but it is only a quasi-satellite. Cruithne never...
, an asteroid in approximate 1:1 orbital resonance with the Earth - Resonant trans-Neptunian objectResonant trans-Neptunian objectIn astronomy, a resonant trans-Neptunian object is a trans-Neptunian object in mean motion orbital resonance with Neptune. The orbital periods of the resonant objects are in a simple integer relations with the period of Neptune e.g. 1:2, 2:3 etc...
- Commensurability (astronomy)Commensurability (astronomy)Commensurability is the property of two orbiting objects, such as planets, satellites, or asteroids, whose orbital periods are in a rational proportion....
- Kozai resonanceKozai mechanismIn celestial mechanics, the Kozai mechanism, or the Lidov-Kozai mechanism, causes a periodic exchange between the inclination and eccentricity of an orbit...
- Dermott's Law
- Lagrangian pointLagrangian pointThe Lagrangian points are the five positions in an orbital configuration where a small object affected only by gravity can theoretically be stationary relative to two larger objects...
s - Mercury, which has a 3:2 spin-orbit resonance
- Tidal lockingTidal lockingTidal locking occurs when the gravitational gradient makes one side of an astronomical body always face another; for example, the same side of the Earth's Moon always faces the Earth. A tidally locked body takes just as long to rotate around its own axis as it does to revolve around its partner...
- Tidal resonanceTidal resonanceIn oceanography, a tidal resonance occurs when the tide excites one of the resonant modes of the ocean.The effect is most striking when a continental shelf is about a quarter wavelength wide...
- Titius-Bode lawTitius-Bode lawThe Titius–Bode law is a hypothesis that the bodies in some orbital systems, including the Sun's, orbit at semi-major axes in a function of planetary sequence...
- Trojan objectTrojan (astronomy)In astronomy, a Trojan is a minor planet or natural satellite that shares an orbit with a larger planet or moon, but does not collide with it because it orbits around one of the two Lagrangian points of stability , and , which lie approximately 60° ahead of and behind the larger body,...
, a body in a type of 1:1 resonance - Horseshoe orbitHorseshoe orbitA horseshoe orbit is a type of co-orbital motion of a small orbiting body relative to a larger orbiting body . The orbital period of the smaller body is very nearly the same as for the larger body, and its path appears to have a horseshoe shape in a rotating reference frame as viewed from the...
, followed by an object in another type of 1:1 resonance - Musica universalisMusica universalisMusica universalis is an ancient philosophical concept that regards proportions in the movements of celestial bodies—the Sun, Moon, and planets—as a form of musica . This 'music' is not usually thought to be literally audible, but a harmonic and/or mathematical and/or religious concept...
("music of the spheres")
External links
- Locations of Solar System Planetary Mean-Motion Resonances. Web calculator that plots distributions of the semimajor axes (or in one case the perihelion distances) of the minor planets in relation to mean motion resonances of the planets (website maintained by M.A. Murison).