Basil Hiley
Encyclopedia
Basil Hiley, born 1935, is a British
quantum
physicist
and professor emeritus of the University of London
.
of a macromolecule
, which was followed by further papers on the Ising model
, and lattice constant
systems defined in graph theoretical
terms.
After completing his PhD in condensed matter physics
under the supervision of Cyril Domb
and Michael Fisher
, Hiley was appointed assistant lecturer at Birbeck College. He reports of himself that he was particularly fascinated by John Wheeler
’s “sum over three geometries” ideas that he was using to quantise gravity and felt that the “Einstein-Schrödinger equation”, as Wheeler called it, might be found by studying the full implications of the model which had been proposed by David Bohm
in 1952.
Hiley worked with Bohm over many years on fundamental problems of theoretical physics
, resulting in a book published 1993, which is considered the major reference for Bohm's interpretation of quantum theory
.
In 1995, Basil Hiley was appointed to a chair in physics at Birkbeck College at the University of London
.
leads to the notion of an “unbroken wholeness of the entire universe”, and they proposed possible routes to a generalization of the approach to general relativity by means of a novel concept of time. In 1979 they discussed the Aharonov-Bohm effect
which had recently found experimental confirmation. Bohm and Hiley together covered topics such as quantum nonlocality and the measurement process, as well as the notion of beables (introduced by John Bell
in contrast to observable
s). Hiley and co-workers showed how, based on the quantum potential approach, ensembles of particle trajectories could be deduced that could account for the interference fringes in the double-split experiment.
Bohm and Hiley interpreted Bell's theorem
as a test of spontaneous localization, meaning a tendency of a many-body system to factorize into a product of localized states of its constituent particles, pointing out that such spontaneous localization removes the need for a fundamental role of the measuring apparatus in quantum theory.
Bohm and Hiley called attention to the importance of the early work of Louis de Broglie on pilot wave
s and his insight and physical intuition; according to them, extensions of de Broglie's ideas were being developed that aimed at a better understanding than mathematical formalism alone.
Introducing the concept of active information, they showed how measurement, with the collapse of the wave function, could be understood in terms of the quantum potential approach, and that this approach could be extended to relativistic field theories
. They described the measurement process and the impossibility of measuring position and momentum simultaneously as follows: “The ѱ field itself changes since it must satisfy the Schrödinger equation, which now contains the interaction between the particle and apparatus, and it is this change that makes it impossible to measure position and momentum together”. The collapse of the wave function of the Copenhagen interpretation of quantum theory is explained in the quantum potential approach by the demonstration that “all the packets of the multi-dimensional wave function that do not correspond to the actual result of measurement have no effect on the particle” from then on. With P.N. Kaloyerou, he extended the quantum potential approach to quantum field theory in Minkowski space
time. Hiley and a co-worker later extended it further to curved spacetime.
They demonstrated that the non-locality of quantum theory can be understood as limit case of a purely local theory, provided the transmission of active information is allowed to be greater than the speed of light, and that this limit case yields approximations to both quantum theory and relativity. In work of 1987 and their book of 1993, they laid out why they considered the quantum potential to be an information potential. The quantum potential (information potential) does not act mechanically on the system, but rather influences the form of processes and is itself shaped by the environment.
Hiley emphasised three aspects that regard the quantum potential of a quantum particle: it
He explained further:
The quantum potential (information potential) links the quantum system under investigation to the measuring apparatus, thereby giving that system a significance within the context defined by the apparatus. In a system consisting of multiple particles, active information is transferred from one particle to another, and this transfer is mediated by the non-local quantum potential.
of Roger Penrose
as a Clifford algebra
, thereby describing structure and forms of ordinary space as an explicit order that unfolds from an implicate order
, the latter representing a pre-space as proposed by Wheeler
. More generally, Bohm and Hiley worked towards representing the implicate order in form of an appropriate algebra
or other pregeometry
. They considered spacetime
itself as part of an explicit order that is connected to pre-space as implicit order. The spacetime manifold and properties of locality
and nonlocality
then arise from an order in such pre-space. A. M. Frescura and Hiley suggested that an implicate order could be carried by an algebra, with the explicate order being contained in the various representations of this algebra.
Much of Bohm and Hiley's work expanded on the notion of implicate, explicate and generative orders proposed by Bohm. In the view of Bohm and Hiley, “things, such as particles, objects, and indeed subjects, are considered as semi-autonomous quasi-local features of this underlying activity”. These features can be considered to be independent only up to a certain level of approximation in which certain criteria are fulfilled. In this picture, the classical limit
for quantum phenomena, in terms of a condition that the action function
is not much greater than Planck's constant, indicates one such criterion. Bohm and Hiley used the word holomovement
for the underlying activity in the various orders together. This term is intended extend beyond the notion of process, covering movement in a wide context such as for instance the movement of a symphony: a “a total ordering which involves the whole movement, past and anticipated, at any one moment”. This concept, which avowedly has similarities with the notion of organic mechanism of Alfred North Whitehead
, underlies Bohm and Hiley´s efforts towards establishing algebraic structures that relate to quantum physics as well as their investigations into an ordering that relates to thought processes and the mind.
In 1985, Bohm and Hiley showed that Wheeler's delayed choice experiment
does not require the existence of the past
to be limited to its recording in the present. Hiley confirmed this in later work in terms of an investigation into welcher Weg experiments. Hiley has pursued work on algebraic structures in quantum theory throughout his scientific career. After Bohm's death in 1992, he published several papers on how different formulations of quantum physics, including Bohm's, can be brought in context, as well as on the relation between classical and quantum physics. He pursued further work on the thought experiment
s set out by Einstein-Podolsky-Rosen
and by Lucien Hardy
, concerning in particular on aspects relevant to the framework of special relativity. Bohm and Hiley had demonstrated a relation between the Wigner–Moyal approach
and Bohm's theory, allowing to avoid the problem of negative probabilities
, and Hiley emphasized its relevance to constructing a quantum geometry
. Together with Melvin Brown, he showed that the Bohm interpretation of quantum physics need not rely on a formulation in terms of ordinary space (
-space), but could also, alternatively, be formulated in terms of momentum space (
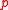-space); Hiley thereby gave further evidence to the notion that the ontology of implicate and explicate orders could be understood as a process described in terms of a non-commutative algebra, from which spacetime could be abstracted, namely as one of several possible representations. Hiley identifies the algebraic structure with an implicate order, their shadow manifolds (projections) with the sets of explicate orders that are consistent with that implicate order. Recently, based on a description of the dynamics of the Schrödinger, Pauli and Dirac particles as a hierarchy of Clifford algebras, he presented a complete relativistic version of the Bohm model for the Dirac particle.
He has shown how the energy-momentum-relations in the Bohm model can be obtained directly from the energy-momentum tensor of quantum field theory
. He has referred to this as “a remarkable discovery, so obvious that I am surprised we didn't spot it sooner” and has pointed out that, on this basis, the quantum potential constitutes the missing energy term that is required for local energy-momentum conservation.
Hiley has repeatedly discussed the reasons for which the Bohm interpretation has met resistance, these reasons relating mainly to assumptions on particle trajectories. In a recent work on the quantum Zeno effect
, he showed that in Bohm's model a continuously observed trajectory is identical to the classical particle trajectory.
He has stated that his recent focus on noncommutative geometry
appears to be very much in line with the work of Fred van Oystaeyen
on noncommutative topology
.
addressed the question of the relation between mind and matter by the hypothesis of an active information contributing to quantum potential
.
Bohm's and Hiley's work on implicate and explicate order
is based on the thought that matter and of the content of thought are displayed in explicate orders, while deeper quantum movements as well as the process of thinking, feeling, etc. occur through the activity of unfolding and enfolding in an implicate order. Hiley worked closely with biologist Brian Goodwin
on a process view of biological life, with an alternate view on Darwinism. Hiley aims at finding “an algebraic description of those aspects of this implicate order where mind and matter have their origins”.
British people
The British are citizens of the United Kingdom, of the Isle of Man, any of the Channel Islands, or of any of the British overseas territories, and their descendants...
quantum
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
physicist
Physicist
A physicist is a scientist who studies or practices physics. Physicists study a wide range of physical phenomena in many branches of physics spanning all length scales: from sub-atomic particles of which all ordinary matter is made to the behavior of the material Universe as a whole...
and professor emeritus of the University of London
University of London
-20th century:Shortly after 6 Burlington Gardens was vacated, the University went through a period of rapid expansion. Bedford College, Royal Holloway and the London School of Economics all joined in 1900, Regent's Park College, which had affiliated in 1841 became an official divinity school of the...
.
Work
Hiley published a paper in 1961 on the random walkRandom walk
A random walk, sometimes denoted RW, is a mathematical formalisation of a trajectory that consists of taking successive random steps. For example, the path traced by a molecule as it travels in a liquid or a gas, the search path of a foraging animal, the price of a fluctuating stock and the...
of a macromolecule
Macromolecule
A macromolecule is a very large molecule commonly created by some form of polymerization. In biochemistry, the term is applied to the four conventional biopolymers , as well as non-polymeric molecules with large molecular mass such as macrocycles...
, which was followed by further papers on the Ising model
Ising model
The Ising model is a mathematical model of ferromagnetism in statistical mechanics. The model consists of discrete variables called spins that can be in one of two states . The spins are arranged in a graph , and each spin interacts with its nearest neighbors...
, and lattice constant
Lattice constant
The lattice constant [or lattice parameter] refers to the constant distance between unit cells in a crystal lattice. Lattices in three dimensions generally have three lattice constants, referred to as a, b, and c. However, in the special case of cubic crystal structures, all of the constants are...
systems defined in graph theoretical
Graph theory
In mathematics and computer science, graph theory is the study of graphs, mathematical structures used to model pairwise relations between objects from a certain collection. A "graph" in this context refers to a collection of vertices or 'nodes' and a collection of edges that connect pairs of...
terms.
After completing his PhD in condensed matter physics
Condensed matter physics
Condensed matter physics deals with the physical properties of condensed phases of matter. These properties appear when a number of atoms at the supramolecular and macromolecular scale interact strongly and adhere to each other or are otherwise highly concentrated in a system. The most familiar...
under the supervision of Cyril Domb
Cyril Domb
Cyril Domb is a physicist best known for his lecturing and writing on the theory of phase transitions and critical phenomena of fluids. He is also known in the Orthodox Jewish world for his writings on Science and Judaism...
and Michael Fisher
Michael Fisher
Michael Ellis Fisher is an English physicist, as well as chemist and mathematician, known for his many seminal contributions...
, Hiley was appointed assistant lecturer at Birbeck College. He reports of himself that he was particularly fascinated by John Wheeler
John Archibald Wheeler
John Archibald Wheeler was an American theoretical physicist who was largely responsible for reviving interest in general relativity in the United States after World War II. Wheeler also worked with Niels Bohr in explaining the basic principles behind nuclear fission...
’s “sum over three geometries” ideas that he was using to quantise gravity and felt that the “Einstein-Schrödinger equation”, as Wheeler called it, might be found by studying the full implications of the model which had been proposed by David Bohm
David Bohm
David Joseph Bohm FRS was an American-born British quantum physicist who contributed to theoretical physics, philosophy, neuropsychology, and the Manhattan Project.-Youth and college:...
in 1952.
Hiley worked with Bohm over many years on fundamental problems of theoretical physics
Theoretical physics
Theoretical physics is a branch of physics which employs mathematical models and abstractions of physics to rationalize, explain and predict natural phenomena...
, resulting in a book published 1993, which is considered the major reference for Bohm's interpretation of quantum theory
Quantum theory
Quantum theory may mean:In science:*Quantum mechanics: a subset of quantum physics explaining the physical behaviours at atomic and sub-atomic levels*Old quantum theory under the Bohr model...
.
In 1995, Basil Hiley was appointed to a chair in physics at Birkbeck College at the University of London
University of London
-20th century:Shortly after 6 Burlington Gardens was vacated, the University went through a period of rapid expansion. Bedford College, Royal Holloway and the London School of Economics all joined in 1900, Regent's Park College, which had affiliated in 1841 became an official divinity school of the...
.
Quantum potential and active information
Building on the theory presented by Bohm in 1952, Bohm and Hiley co-authored numerous articles. In 1975, they proposed that the fundamental new quality introduced by quantum physics is nonlocality, they presented how in the causal interpretation of the quantum theory introduced by Bohm in 1952 the concept of a quantum potentialQuantum potential
The quantum potential is a central concept of the de Broglie–Bohm formulation of quantum mechanics, introduced by David Bohm in 1952.Initially presented under the name quantum-mechanical potential, subsequently quantum potential, it was later elaborated upon by Bohm and Basil Hiley in its...
leads to the notion of an “unbroken wholeness of the entire universe”, and they proposed possible routes to a generalization of the approach to general relativity by means of a novel concept of time. In 1979 they discussed the Aharonov-Bohm effect
Aharonov-Bohm effect
The Aharonov–Bohm effect, sometimes called the Ehrenberg–Siday–Aharonov–Bohm effect, is a quantum mechanical phenomenon in which an electrically charged particle is affected by an electromagnetic field , despite being confined to a region in which both the magnetic field B and electric field E are...
which had recently found experimental confirmation. Bohm and Hiley together covered topics such as quantum nonlocality and the measurement process, as well as the notion of beables (introduced by John Bell
John Bell
- Law and politics :* John Bell , English barrister* John Bell , professor of law and Fellow of Pembroke College, Cambridge* John Bell , Member of Parliament from Thirsk...
in contrast to observable
Observable
In physics, particularly in quantum physics, a system observable is a property of the system state that can be determined by some sequence of physical operations. For example, these operations might involve submitting the system to various electromagnetic fields and eventually reading a value off...
s). Hiley and co-workers showed how, based on the quantum potential approach, ensembles of particle trajectories could be deduced that could account for the interference fringes in the double-split experiment.
Bohm and Hiley interpreted Bell's theorem
Bell's theorem
In theoretical physics, Bell's theorem is a no-go theorem, loosely stating that:The theorem has great importance for physics and the philosophy of science, as it implies that quantum physics must necessarily violate either the principle of locality or counterfactual definiteness...
as a test of spontaneous localization, meaning a tendency of a many-body system to factorize into a product of localized states of its constituent particles, pointing out that such spontaneous localization removes the need for a fundamental role of the measuring apparatus in quantum theory.
Bohm and Hiley called attention to the importance of the early work of Louis de Broglie on pilot wave
Pilot wave
In theoretical physics, the Pilot Wave theory was the first known example of a hidden variable theory, presented by Louis de Broglie in 1927. Its more modern version, the Bohm interpretation,...
s and his insight and physical intuition; according to them, extensions of de Broglie's ideas were being developed that aimed at a better understanding than mathematical formalism alone.
Introducing the concept of active information, they showed how measurement, with the collapse of the wave function, could be understood in terms of the quantum potential approach, and that this approach could be extended to relativistic field theories
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
. They described the measurement process and the impossibility of measuring position and momentum simultaneously as follows: “The ѱ field itself changes since it must satisfy the Schrödinger equation, which now contains the interaction between the particle and apparatus, and it is this change that makes it impossible to measure position and momentum together”. The collapse of the wave function of the Copenhagen interpretation of quantum theory is explained in the quantum potential approach by the demonstration that “all the packets of the multi-dimensional wave function that do not correspond to the actual result of measurement have no effect on the particle” from then on. With P.N. Kaloyerou, he extended the quantum potential approach to quantum field theory in Minkowski space
Minkowski space
In physics and mathematics, Minkowski space or Minkowski spacetime is the mathematical setting in which Einstein's theory of special relativity is most conveniently formulated...
time. Hiley and a co-worker later extended it further to curved spacetime.
They demonstrated that the non-locality of quantum theory can be understood as limit case of a purely local theory, provided the transmission of active information is allowed to be greater than the speed of light, and that this limit case yields approximations to both quantum theory and relativity. In work of 1987 and their book of 1993, they laid out why they considered the quantum potential to be an information potential. The quantum potential (information potential) does not act mechanically on the system, but rather influences the form of processes and is itself shaped by the environment.
Hiley emphasised three aspects that regard the quantum potential of a quantum particle: it
- is derived mathematically from the real part of the wave function and is not derived from a Hamiltonian or other external source, and could rather said to be involved in a self-organising process involving a basic underlying field;
- is independent of field intensity, thus fulfilling a precondition for nonlocality;
- carries information about the whole experimental arrangement in which the particle finds itself.
He explained further:
- “These properties show how the quantum potential is essentially different from a classical particle, so different that we must conclude that it does not give rise to a mechanical force in the Newtonian sense. Thus while the Newtonian potential drives the particle along the trajectory, the quantum potential organises the form of the trajectories in response to the experimental conditions.”
The quantum potential (information potential) links the quantum system under investigation to the measuring apparatus, thereby giving that system a significance within the context defined by the apparatus. In a system consisting of multiple particles, active information is transferred from one particle to another, and this transfer is mediated by the non-local quantum potential.
Implicate and explicate orders
Starting from the concept that “relativistic quantum mechanics can be expressed completely through the interweaving of three basic algebras, the bosonic, the fermionic and the Clifford” and that in this manner “the whole of relativisic quantum mechanics can also be put into an implicate order” as known from earlier publications of Bohm of 1973 and 1980, Bohm and Hiley expressed the twistor theoryTwistor theory
In theoretical and mathematical physics, twistor theory maps the geometric objects of conventional 3+1 space-time into geometric objects in a 4 dimensional space with metric signature...
of Roger Penrose
Roger Penrose
Sir Roger Penrose OM FRS is an English mathematical physicist and Emeritus Rouse Ball Professor of Mathematics at the Mathematical Institute, University of Oxford and Emeritus Fellow of Wadham College...
as a Clifford algebra
Clifford algebra
In mathematics, Clifford algebras are a type of associative algebra. As K-algebras, they generalize the real numbers, complex numbers, quaternions and several other hypercomplex number systems. The theory of Clifford algebras is intimately connected with the theory of quadratic forms and orthogonal...
, thereby describing structure and forms of ordinary space as an explicit order that unfolds from an implicate order
Implicate and explicate order according to David Bohm
According to David Bohm's theory, implicate and explicate orders are characterised by:-David Bohm's challenges to some generally prevailing views:...
, the latter representing a pre-space as proposed by Wheeler
John Archibald Wheeler
John Archibald Wheeler was an American theoretical physicist who was largely responsible for reviving interest in general relativity in the United States after World War II. Wheeler also worked with Niels Bohr in explaining the basic principles behind nuclear fission...
. More generally, Bohm and Hiley worked towards representing the implicate order in form of an appropriate algebra
Algebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
or other pregeometry
Pregeometry (physics)
In physics, a pregeometry is a structure from which geometry develops. The term was championed by John Archibald Wheeler in the 1960s and 1970s as a possible route to a theory of quantum gravity...
. They considered spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
itself as part of an explicit order that is connected to pre-space as implicit order. The spacetime manifold and properties of locality
Principle of locality
In physics, the principle of locality states that an object is influenced directly only by its immediate surroundings. Experiments have shown that quantum mechanically entangled particles must violate either the principle of locality or the form of philosophical realism known as counterfactual...
and nonlocality
Nonlocality
In Classical physics, nonlocality is the direct influence of one object on another, distant object. In Quantum mechanics, nonlocality refers to the absence of a local, realist model in agreement with quantum mechanical predictions.Nonlocality may refer to:...
then arise from an order in such pre-space. A. M. Frescura and Hiley suggested that an implicate order could be carried by an algebra, with the explicate order being contained in the various representations of this algebra.
Much of Bohm and Hiley's work expanded on the notion of implicate, explicate and generative orders proposed by Bohm. In the view of Bohm and Hiley, “things, such as particles, objects, and indeed subjects, are considered as semi-autonomous quasi-local features of this underlying activity”. These features can be considered to be independent only up to a certain level of approximation in which certain criteria are fulfilled. In this picture, the classical limit
Classical limit
The classical limit or correspondence limit is the ability of a physical theory to approximate or "recover" classical mechanics when considered over special values of its parameters. The classical limit is used with physical theories that predict non-classical behavior...
for quantum phenomena, in terms of a condition that the action function
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
is not much greater than Planck's constant, indicates one such criterion. Bohm and Hiley used the word holomovement
Holomovement
The holomovement is a key concept in David Bohm's interpretation of quantum mechanics and for his overall wordview. It brings together the holistic principle of "undivided wholeness" with the idea that everything is in a state of process or becoming...
for the underlying activity in the various orders together. This term is intended extend beyond the notion of process, covering movement in a wide context such as for instance the movement of a symphony: a “a total ordering which involves the whole movement, past and anticipated, at any one moment”. This concept, which avowedly has similarities with the notion of organic mechanism of Alfred North Whitehead
Alfred North Whitehead
Alfred North Whitehead, OM FRS was an English mathematician who became a philosopher. He wrote on algebra, logic, foundations of mathematics, philosophy of science, physics, metaphysics, and education...
, underlies Bohm and Hiley´s efforts towards establishing algebraic structures that relate to quantum physics as well as their investigations into an ordering that relates to thought processes and the mind.
In 1985, Bohm and Hiley showed that Wheeler's delayed choice experiment
Wheeler's delayed choice experiment
Wheeler's delayed choice experiment is a thought experiment proposed by John Archibald Wheeler in 1978, and later confirmed. Wheeler proposed a variation of the famous double-slit experiment of quantum physics, one in which the method of detection can be changed after the photon passes the double...
does not require the existence of the past
Past
Most generally, the past is a term used to indicate the totality of events which occurred before a given point in time. The past is contrasted with and defined by the present and the future. The concept of the past is derived from the linear fashion in which human observers experience time, and is...
to be limited to its recording in the present. Hiley confirmed this in later work in terms of an investigation into welcher Weg experiments. Hiley has pursued work on algebraic structures in quantum theory throughout his scientific career. After Bohm's death in 1992, he published several papers on how different formulations of quantum physics, including Bohm's, can be brought in context, as well as on the relation between classical and quantum physics. He pursued further work on the thought experiment
Thought experiment
A thought experiment or Gedankenexperiment considers some hypothesis, theory, or principle for the purpose of thinking through its consequences...
s set out by Einstein-Podolsky-Rosen
EPR paradox
The EPR paradox is a topic in quantum physics and the philosophy of science concerning the measurement and description of microscopic systems by the methods of quantum physics...
and by Lucien Hardy
Hardy's paradox
Hardy's paradox is a thought experiment in quantum mechanics devised by Lucien Hardy in which a particle and its antiparticle may interact without annihilating each other...
, concerning in particular on aspects relevant to the framework of special relativity. Bohm and Hiley had demonstrated a relation between the Wigner–Moyal approach
Wigner quasi-probability distribution
The Wigner quasi-probability distribution is a quasi-probability distribution. It was introduced by Eugene Wigner in 1932 to study quantum corrections to classical statistical mechanics...
and Bohm's theory, allowing to avoid the problem of negative probabilities
Negative probability
In 1942, Paul Dirac wrote a paper "The Physical Interpretation of Quantum Mechanics" where he introduced the concept of negative energies and negative probabilities:...
, and Hiley emphasized its relevance to constructing a quantum geometry
Quantum geometry
In theoretical physics, quantum geometry is the set of new mathematical concepts generalizing the concepts of geometry whose understanding is necessary to describe the physical phenomena at very short distance scales...
. Together with Melvin Brown, he showed that the Bohm interpretation of quantum physics need not rely on a formulation in terms of ordinary space (
-space), but could also, alternatively, be formulated in terms of momentum space (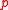-space); Hiley thereby gave further evidence to the notion that the ontology of implicate and explicate orders could be understood as a process described in terms of a non-commutative algebra, from which spacetime could be abstracted, namely as one of several possible representations. Hiley identifies the algebraic structure with an implicate order, their shadow manifolds (projections) with the sets of explicate orders that are consistent with that implicate order. Recently, based on a description of the dynamics of the Schrödinger, Pauli and Dirac particles as a hierarchy of Clifford algebras, he presented a complete relativistic version of the Bohm model for the Dirac particle.He has shown how the energy-momentum-relations in the Bohm model can be obtained directly from the energy-momentum tensor of quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
. He has referred to this as “a remarkable discovery, so obvious that I am surprised we didn't spot it sooner” and has pointed out that, on this basis, the quantum potential constitutes the missing energy term that is required for local energy-momentum conservation.
Hiley has repeatedly discussed the reasons for which the Bohm interpretation has met resistance, these reasons relating mainly to assumptions on particle trajectories. In a recent work on the quantum Zeno effect
Quantum Zeno effect
The quantum Zeno effect is a name coined by George Sudarshan and Baidyanath Misra of the University of Texas in 1977 in their analysis of the situation in which an unstable particle, if observed continuously, will never decay. One can nearly "freeze" the evolution of the system by measuring it...
, he showed that in Bohm's model a continuously observed trajectory is identical to the classical particle trajectory.
He has stated that his recent focus on noncommutative geometry
Noncommutative geometry
Noncommutative geometry is a branch of mathematics concerned with geometric approach to noncommutative algebras, and with construction of spaces which are locally presented by noncommutative algebras of functions...
appears to be very much in line with the work of Fred van Oystaeyen
Fred Van Oystaeyen
Fred Van Oystaeyen , also Freddy van Oystaeyen, is a mathematician and professor of mathematics at the University of Antwerp.He has pioneered work on noncommutative geometry, in particular noncommutative algebraic geometry....
on noncommutative topology
Noncommutative topology
Noncommutative topology in mathematics is a term applied to the strictly C*-algebraic part of the noncommutative geometry program. The program has its origins in the Gel'fand duality between the topology of locally compact spaces and the algebraic structure of commutative C*-algebras.Several...
.
Mind and matter
Hiley and Paavo PylkkänenPaavo Pylkkänen
Paavo Pylkkänen is a Finnish philosopher of mind. He is associate professor in philosophy at Skövde University College and adjunct professor of philosophy at the University of Helsinki...
addressed the question of the relation between mind and matter by the hypothesis of an active information contributing to quantum potential
Quantum potential
The quantum potential is a central concept of the de Broglie–Bohm formulation of quantum mechanics, introduced by David Bohm in 1952.Initially presented under the name quantum-mechanical potential, subsequently quantum potential, it was later elaborated upon by Bohm and Basil Hiley in its...
.
Bohm's and Hiley's work on implicate and explicate order
Implicate and explicate order according to David Bohm
According to David Bohm's theory, implicate and explicate orders are characterised by:-David Bohm's challenges to some generally prevailing views:...
is based on the thought that matter and of the content of thought are displayed in explicate orders, while deeper quantum movements as well as the process of thinking, feeling, etc. occur through the activity of unfolding and enfolding in an implicate order. Hiley worked closely with biologist Brian Goodwin
Brian Goodwin
Brian Carey Goodwin was a Canadian mathematician and biologist, a Professor Emeritus at the Open University and a key founder of the field of theoretical biology.He made key contributions to the foundations of biomathematics, complex systems and generative models in developmental biology...
on a process view of biological life, with an alternate view on Darwinism. Hiley aims at finding “an algebraic description of those aspects of this implicate order where mind and matter have their origins”.
Books
- F. David PeatF. David PeatF. David Peat was born in Waterloo, England and is a holistic physicist and author who has carried out research in solid state physics and the foundation of quantum theory....
(Editor) and Basil Hiley (Editor): Quantum Implications: Essays in Honour of David Bohm, Routledge & Kegan Paul Ltd, London & New York, 1987 - David Bohm, Basil Hiley: The Undivided Universe: An Ontological Interpretation of Quantum Theory, Routledge, 1993, ISBN 0-415-06588-7
Articles
- Basil J. Hiley: Bohm's Approach to the EPR Paradox, Compendium of Quantum Physics, 2009, 55-58, DOI: 10.1007/978-3-540-70626-7_17 (abstract)
- Basil J. Hiley, Paavo Pylkkänen: Can mind affect matter via active information, Mind & Matter, volume 3, number 2, pp. 7–27, Imprint Academic, 2005 (abstract)
- B. J. Hiley, R.E Callaghan, O. Maroney: Quantum trajectories, real, surreal or an approximation to a deeper process? (submitted on 5 Oct 2000, version of 5 Nov 2000 - abstract, PDF)
- O. Maroney, B. J. Hiley: Quantum State Teleportation Understood Through the Bohm Interpretation, Foundations of Physics, Volume 29, Number 9, pp. 1403–1415, 1999, DOI: 10.1023/A:1018861226606 (abstract)
- D. Bohm and B. J. Hiley: Statistical mechanics and the ontological interpretation, Foundations of Physics, Volume 26, Number 6, pp. 823–846, 1996, DOI: 10.1007/BF02058636, Part III. Invited Papers Dedicated to Max Jammer (abstract)
- O. Cohen, B. J. Hiley: Elements of reality, Lorentz invariance, and the product rule, Foundations of Physics, Volume 26, Number 1, pp. 1–15, 1996, DOI: 10.1007/BF02058886 (abstract)
- D. Bohm, B. J. Hiley: Unbroken Quantum Realism, from Microscopic to Macroscopic Levels, Physics Review Letters, volume 55, number 23, pp. 2511–2514, 1985, DOI: 10.1103/PhysRevLett.55.2511 (abstract)
- C. Dewdney, B. J. Hiley: A quantum potential description of one-dimensional time-dependent scattering from square barriers and square wells, Foundations of Physics, Volume 12, Number 1, pp. 27–48, 1982, DOI: 10.1007/BF00726873 (abstract)
- D. Bohm, B. J. Hiley: Nonlocality in quantum theory understood in terms of Einstein's nonlinear field approach, Foundations of Physics, Volume 11, Numbers 7-8, pp. 529-546, 1981, DOI: 10.1007/BF00726935 (abstract)
- D. Bohm, B. J. Hiley: On a quantum algebraic approach to a generalized phase space, Foundations of Physics, volume 11, numbers 3-4, pp. 179–203, 1981, DOI: 10.1007/BF00726266 (abstract)
External links
- Basil Hiley, Birkbeck College - Publications on algebraic structures in quantum theory - Recent publications
- Publications by Basil Hiley, Spires, High-Energy Physics Literature Database
- Articles by B. J. Hiley at the Center for Process Studies (ctr4process.org):
- Basil J. Hiley: Process and the Implicate Order: their relevance to Quantum Theory and Mind. (PDF)
- Interviews with Basil Hiley:
- The measurement problem in physics, a List of In Our Time programmes discussion programme, 5 March 2009
- Interview with Basil Hiley conducted by M. Perus
- David Bohm Quantum theory versus Copenhagen Interpretation, YouTube
- David Bohm, Wholistic Universe, quantum physics, YouTube
- Taher Gozel interview with Basil Hiley (part 1), YouTube
- Lectures by Basil Hiley:
- Towards a quantum geometry: Groupoids, Clifford algebras and shadow manifolds (slides)