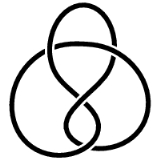
Amphichiral knot
Encyclopedia
In the mathematical
field of knot theory
, a chiral knot is a knot
that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphichiral knot, also called an achiral knot or amphicheiral knot. The chirality of a knot is a knot invariant
. A knot's chirality can be further classified depending on whether or not it is invertible
.
in 1914. P. G. Tait conjectured that all amphichiral knots had even crossing number
, but a counterexample was found by Morwen Thistlethwaite
et al. in 1998. However, Tait's conjecture was proven true for prime
, alternating knot
s.
, which was shown to be chiral by Max Dehn
. All torus knot
s are chiral. The Alexander polynomial
cannot detect the chirality of a knot, but the Jones polynomial can in some cases; if Vk(q) ≠ Vk(q−1), then the knot is chiral, however the converse is not true. The HOMFLY polynomial
is even better at detecting chirality, but no knot invariant
is known which can fully detect chirality.
is classified as a reversible knot.
or its mirror image, it is a fully chiral knot.
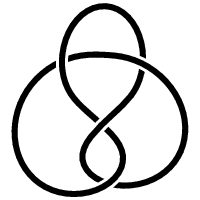 An amphichiral knot is one which has an orientation
An amphichiral knot is one which has an orientation
-reversing self-homeomorphism
of the 3-sphere
, α, fixing the knot set-wise.
All amphichiral alternating knot
s have even crossing number
. The only known amphichiral knot with odd crossing number is a 15-crossing knot discovered by Hoste
et al.
to both its reverse and its mirror image, it is fully amphichiral. The simplest knot with this property is the figure eight knot.
 If the self-homeomorphism, α, reverses the orientation of the knot, it is said to be negative amphichiral. This is equivalent to the knot being isotopic to the reverse of its mirror image. The knot with this property that has the fewest crossings is the knot 817.
If the self-homeomorphism, α, reverses the orientation of the knot, it is said to be negative amphichiral. This is equivalent to the knot being isotopic to the reverse of its mirror image. The knot with this property that has the fewest crossings is the knot 817.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
field of knot theory
Knot theory
In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...
, a chiral knot is a knot
Knot (mathematics)
In mathematics, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3, considered up to continuous deformations . A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a...
that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphichiral knot, also called an achiral knot or amphicheiral knot. The chirality of a knot is a knot invariant
Knot invariant
In the mathematical field of knot theory, a knot invariant is a quantity defined for each knot which is the same for equivalent knots. The equivalence is often given by ambient isotopy but can be given by homeomorphism. Some invariants are indeed numbers, but invariants can range from the...
. A knot's chirality can be further classified depending on whether or not it is invertible
Invertible knot
In mathematics, especially in the area of topology known as knot theory, an invertible knot is a knot that can be continuously deformed to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant...
.
Background
The chirality of certain knots was long suspected, and was proven by Max DehnMax Dehn
Max Dehn was a German American mathematician and a student of David Hilbert. He is most famous for his work in geometry, topology and geometric group theory...
in 1914. P. G. Tait conjectured that all amphichiral knots had even crossing number
Crossing number (knot theory)
In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....
, but a counterexample was found by Morwen Thistlethwaite
Morwen Thistlethwaite
Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...
et al. in 1998. However, Tait's conjecture was proven true for prime
Prime knot
In knot theory, a prime knot is a knot that is, in a certain sense, indecomposable. Specifically, it is a non-trivial knot which cannot be written as the knot sum of two non-trivial knots. Knots that are not prime are said to be composite. It can be a nontrivial problem to determine whether a...
, alternating knot
Alternating knot
In knot theory, a link diagram is alternating if the crossings alternate under, over, under, over, as you travel along each component of the link. A link is alternating if it has an alternating diagram....
s.
| Number of crossings | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | OEIS sequence |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Chiral knots | 1 | 0 | 2 | 2 | 7 | 16 | 49 | 152 | 552 | 2118 | 9988 | 46698 | 253292 | 1387166 | N/A |
| Reversible knots | 1 | 0 | 2 | 2 | 7 | 16 | 47 | 125 | 365 | 1015 | 3069 | 8813 | 26712 | 78717 | |
| Fully chiral knots | 0 | 0 | 0 | 0 | 0 | 0 | 2 | 27 | 187 | 1103 | 6919 | 37885 | 226580 | 1308449 | |
| Amphichiral knots | 0 | 1 | 0 | 1 | 0 | 5 | 0 | 13 | 0 | 58 | 0 | 274 | 1 | 1539 | |
| Positive Amphichiral knots | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 6 | 0 | 65 | |
| Negative Amphichiral knots | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 6 | 0 | 40 | 0 | 227 | 1 | 1361 | |
| Fully Amphichiral knots | 0 | 1 | 0 | 1 | 0 | 4 | 0 | 7 | 0 | 17 | 0 | 41 | 0 | 113 |
Chiral knot
The simplest chiral knot is the trefoil knotTrefoil knot
In topology, a branch of mathematics, the trefoil knot is the simplest example of a nontrivial knot. The trefoil can be obtained by joining together the two loose ends of a common overhand knot, resulting in a knotted loop...
, which was shown to be chiral by Max Dehn
Max Dehn
Max Dehn was a German American mathematician and a student of David Hilbert. He is most famous for his work in geometry, topology and geometric group theory...
. All torus knot
Torus knot
In knot theory, a torus knot is a special kind of knot that lies on the surface of an unknotted torus in R3. Similarly, a torus link is a link which lies on the surface of a torus in the same way. Each torus knot is specified by a pair of coprime integers p and q. A torus link arises if p and q...
s are chiral. The Alexander polynomial
Alexander polynomial
In mathematics, the Alexander polynomial is a knot invariant which assigns a polynomial with integer coefficients to each knot type. James Waddell Alexander II discovered this, the first knot polynomial, in 1923...
cannot detect the chirality of a knot, but the Jones polynomial can in some cases; if Vk(q) ≠ Vk(q−1), then the knot is chiral, however the converse is not true. The HOMFLY polynomial
HOMFLY polynomial
In the mathematical field of knot theory, the HOMFLY polynomial, sometimes called the HOMFLY-PT polynomial or the generalized Jones polynomial, is a 2-variable knot polynomial, i.e. a knot invariant in the form of a polynomial of variables m and l....
is even better at detecting chirality, but no knot invariant
Knot invariant
In the mathematical field of knot theory, a knot invariant is a quantity defined for each knot which is the same for equivalent knots. The equivalence is often given by ambient isotopy but can be given by homeomorphism. Some invariants are indeed numbers, but invariants can range from the...
is known which can fully detect chirality.
Reversible knot
A chiral knot that is invertibleInvertible knot
In mathematics, especially in the area of topology known as knot theory, an invertible knot is a knot that can be continuously deformed to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant...
is classified as a reversible knot.
Fully chiral knot
If a knot is not equivalent to its inverseInvertible knot
In mathematics, especially in the area of topology known as knot theory, an invertible knot is a knot that can be continuously deformed to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant...
or its mirror image, it is a fully chiral knot.
Amphichiral knot

Orientability
In mathematics, orientability is a property of surfaces in Euclidean space measuring whether or not it is possible to make a consistent choice of surface normal vector at every point. A choice of surface normal allows one to use the right-hand rule to define a "clockwise" direction of loops in the...
-reversing self-homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
of the 3-sphere
3-sphere
In mathematics, a 3-sphere is a higher-dimensional analogue of a sphere. It consists of the set of points equidistant from a fixed central point in 4-dimensional Euclidean space...
, α, fixing the knot set-wise.
All amphichiral alternating knot
Alternating knot
In knot theory, a link diagram is alternating if the crossings alternate under, over, under, over, as you travel along each component of the link. A link is alternating if it has an alternating diagram....
s have even crossing number
Crossing number (knot theory)
In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....
. The only known amphichiral knot with odd crossing number is a 15-crossing knot discovered by Hoste
Hoste
Hoste can refer to* Hoste, a village in the Trnava Region of Slovakia* Hoste, a commune in the Moselle département of France* Hoste, an island in the Magallanes y la Antártica Chilena Region of Chile...
et al.
Fully amphichiral
If a knot is isotopicIsotopic
The word isotopic has a number of different meanings, including:* In the physical sciences, to do with chemical isotopes;* In mathematics, to do with a relation called isotopy.* In geometry, isotopic refers to facet-transitivity....
to both its reverse and its mirror image, it is fully amphichiral. The simplest knot with this property is the figure eight knot.
Positive amphichiral
If the self-homeomorphism, α, preserves the orientation of the knot, it is said to be positive amphichiral. This is equivalent to the knot being isotopic to its mirror. No knots with crossing number smaller than twelve are positive amphichiral.Negative amphichiral


