Invertible knot
Encyclopedia
In mathematics
, especially in the area of topology
known as knot theory
, an invertible knot is a knot
that can be continuously deformed
to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant
. An invertible link is the link
equivalent of an invertible knot.
It has long been known that most of the simple knots, such as the trefoil knot
and the figure-eight knot are invertible. In 1962 Ralph Fox
conjectured that some knots were non-invertible, but it was not proved that non-invertible knots exist until H. F. Trotter discovered an infinite family of pretzel knots that were non-invertible in 1963. It is now known the majority of knots are non-invertible.
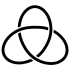All knots with crossing number
of 7 or less are known to be invertible. No general method is known that can distinguish if a given knot is invertible. The problem can be translated into algebraic terms, but unfortunately there is no known algorithm to solve this algebraic problem.
and figure-eight knot
, are strongly invertible.
The simplest example of a non-invertible knot is the knot 817 (Alexander-Briggs notation) or .2.2 (Conway notation
). The pretzel knot 7, 5, 3 is non-invertible, as are all pretzel knots of the form (2p + 1), (2q + 1), (2r + 1), where p, q, and r are distinct integers, which is the infinite family proven to be non-invertible by Trotter.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, especially in the area of topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
known as knot theory
Knot theory
In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...
, an invertible knot is a knot
Knot (mathematics)
In mathematics, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3, considered up to continuous deformations . A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a...
that can be continuously deformed
Ambient isotopy
In the mathematical subject of topology, an ambient isotopy, also called an h-isotopy, is a kind of continuous distortion of an "ambient space", a manifold, taking a submanifold to another submanifold. For example in knot theory, one considers two knots the same if one can distort one knot into the...
to itself, but with its orientation reversed. A non-invertible knot is any knot which does not have this property. The invertibility of a knot is a knot invariant
Knot invariant
In the mathematical field of knot theory, a knot invariant is a quantity defined for each knot which is the same for equivalent knots. The equivalence is often given by ambient isotopy but can be given by homeomorphism. Some invariants are indeed numbers, but invariants can range from the...
. An invertible link is the link
Link (knot theory)
In mathematics, a link is a collection of knots which do not intersect, but which may be linked together. A knot can be described as a link with one component. Links and knots are studied in a branch of mathematics called knot theory...
equivalent of an invertible knot.
Background
| Number of crossings | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | OEIS sequence |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Non-invertible knots | 0 | 0 | 0 | 0 | 0 | 1 | 2 | 33 | 187 | 1144 | 6919 | 38118 | 226581 | 1309875 | |
| Invertible knots | 1 | 1 | 2 | 3 | 7 | 20 | 47 | 132 | 365 | 1032 | 3069 | 8854 | 26712 | 78830 |
It has long been known that most of the simple knots, such as the trefoil knot
Trefoil knot
In topology, a branch of mathematics, the trefoil knot is the simplest example of a nontrivial knot. The trefoil can be obtained by joining together the two loose ends of a common overhand knot, resulting in a knotted loop...
and the figure-eight knot are invertible. In 1962 Ralph Fox
Ralph Fox
Ralph Hartzler Fox was an American mathematician. As a professor at Princeton University, he taught and advised many of the contributors to the Golden Age of differential topology, and he played an important role in the modernization and main-streaming of knot theory.Ralph Fox attended Swarthmore...
conjectured that some knots were non-invertible, but it was not proved that non-invertible knots exist until H. F. Trotter discovered an infinite family of pretzel knots that were non-invertible in 1963. It is now known the majority of knots are non-invertible.
Invertible knots
Crossing number (knot theory)
In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....
of 7 or less are known to be invertible. No general method is known that can distinguish if a given knot is invertible. The problem can be translated into algebraic terms, but unfortunately there is no known algorithm to solve this algebraic problem.
Strongly invertible knots
A more abstract way to define an invertible knot is to say there is an orientation-preserving homeomorphism of the 3-sphere which takes the knot to itself but reverses the orientation along the knot. By imposing the stronger condition that the homeomorphism also be an involution, i.e. have period 2 in the homeomorphism group of the 3-sphere, we arrive at the definition of a strongly invertible knot. All knots with tunnel number one, such as the trefoil knotTrefoil knot
In topology, a branch of mathematics, the trefoil knot is the simplest example of a nontrivial knot. The trefoil can be obtained by joining together the two loose ends of a common overhand knot, resulting in a knotted loop...
and figure-eight knot
Figure-eight knot (mathematics)
In knot theory, a figure-eight knot is the unique knot with a crossing number of four. This is the smallest possible crossing number except for the unknot and trefoil knot...
, are strongly invertible.
Non-invertible knots
Conway notation (knot theory)
In knot theory, Conway notation, invented by John Horton Conway, is a way of describing knots that makes many of their properties clear. It composes a knot using certain operations on tangles to construct it.-Tangles:...
). The pretzel knot 7, 5, 3 is non-invertible, as are all pretzel knots of the form (2p + 1), (2q + 1), (2r + 1), where p, q, and r are distinct integers, which is the infinite family proven to be non-invertible by Trotter.