The Tait conjectures are conjectures made by Peter Guthrie Tait

Peter Guthrie Tait

Peter Guthrie Tait FRSE was a Scottish mathematical physicist, best known for the seminal energy physics textbook Treatise on Natural Philosophy, which he co-wrote with Kelvin, and his early investigations into knot theory, which contributed to the eventual formation of topology as a mathematical...

 in his study of knots

History of knot theory

For thousands of years, knots have been used for basic purposes such as recording information, fastening and tying objects together. Over time people realized that different knots were better at different tasks, such as climbing or sailing. Knots were also regarded as having spiritual and...

. The Tait conjectures involve concepts in knot theory

Knot theory

In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...

 such as alternating knot

Alternating knot

In knot theory, a link diagram is alternating if the crossings alternate under, over, under, over, as you travel along each component of the link. A link is alternating if it has an alternating diagram....

s, chirality, and writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

. All of the Tait conjectures have been solved, the most recent being the Tait flyping conjecture proven in 1991 by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

 and William Menasco

William Menasco

William W. Menasco is a topologist and a professor at the University at Buffalo. He is best known for his work in knot theory.-Biography:Menasco received is B.A. from the University of California, Los Angeles in 1975, and his Ph.D. from the University of California, Berkeley in 1981, where his...

.

Background

Tait came up with his conjectures after his attempt to tabulate

Knot tabulation

Ever since Sir William Thomson's vortex theory, mathematicians have tried to classify and tabulate all possible knots. As of May 2008 all prime knots up to 16 crossings have been tabulated.-Beginnings:...

 all knots in the late 19th century. As a founder of the field of knot theory

Knot theory

In topology, knot theory is the study of mathematical knots. While inspired by knots which appear in daily life in shoelaces and rope, a mathematician's knot differs in that the ends are joined together so that it cannot be undone. In precise mathematical language, a knot is an embedding of a...

, his work lacks a mathematically rigorous framework, and it is unclear whether the conjectures apply to all knots, or just to alternating knot

Alternating knot

In knot theory, a link diagram is alternating if the crossings alternate under, over, under, over, as you travel along each component of the link. A link is alternating if it has an alternating diagram....

s. Most of them are only true for alternating knot

Alternating knot

In knot theory, a link diagram is alternating if the crossings alternate under, over, under, over, as you travel along each component of the link. A link is alternating if it has an alternating diagram....

s. In the Tait conjectures, a knot diagram is reduced if all the isthmi have been removed.

The Tait conjectures

Tait conjectured that in certain circumstances, crossing number

Crossing number (knot theory)

In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....

 was a knot invariant

Knot invariant

In the mathematical field of knot theory, a knot invariant is a quantity defined for each knot which is the same for equivalent knots. The equivalence is often given by ambient isotopy but can be given by homeomorphism. Some invariants are indeed numbers, but invariants can range from the...

, specifically:

Any reduced diagram of an alternating link has the fewest possible crossings

Crossing number (knot theory)

In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....

.

In other words, the crossing number of a reduced, alternating link is an invariant

Knot invariant

In the mathematical field of knot theory, a knot invariant is a quantity defined for each knot which is the same for equivalent knots. The equivalence is often given by ambient isotopy but can be given by homeomorphism. Some invariants are indeed numbers, but invariants can range from the...

 of the knot. This conjecture was proven by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

, Louis Kauffman

Louis Kauffman

Louis H. Kauffman is an American mathematician, topologist, and professor of Mathematics in the Department of Mathematics, Statistics, and Computer science at the University of Illinois at Chicago...

 and K. Murasugi in 1987, using the Jones polynomial.
Another one of his conjectures:

A reduced alternating link with zero writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

 is achiral

Amphichiral knot

In the mathematical field of knot theory, a chiral knot is a knot that is not equivalent to its mirror image. An oriented knot that is equivalent to its mirror image is an amphichiral knot, also called an achiral knot or amphicheiral knot. The chirality of a knot is a knot invariant...

.

This conjecture was also proven by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

.

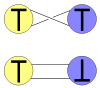
The Tait flyping conjecture

The Tait flyping conjecture can be stated:

Given any two reduced alternating diagrams D₁ and D₂ of an oriented, prime alternating link: D₁ may be transformed to D₂ by means of a sequence of certain simple moves called flype

Flype

In the mathematical theory of knots, a flype is a kind of manipulation of knot and link diagramsused in the Tait flyping conjecture.It consists of twisting a part of a knot, a tangle: T by 180 degrees. Flype comes from an old Scottish word meaning to fold or to turn back. Two reduced alternating...

s. Also known as the Tait flyping conjecture.

The Tait flyping conjecture was proven by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

 and William Menasco

William Menasco

William W. Menasco is a topologist and a professor at the University at Buffalo. He is best known for his work in knot theory.-Biography:Menasco received is B.A. from the University of California, Los Angeles in 1975, and his Ph.D. from the University of California, Berkeley in 1981, where his...

 in 1991.
The Tait flyping conjecture implies some more of Tait's conjectures:

Any two reduced diagrams of the same alternating knot

Knot (mathematics)

In mathematics, a knot is an embedding of a circle in 3-dimensional Euclidean space, R3, considered up to continuous deformations . A crucial difference between the standard mathematical and conventional notions of a knot is that mathematical knots are closed—there are no ends to tie or untie on a...

 have the same writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

.

This follows because flyping

Flype

In the mathematical theory of knots, a flype is a kind of manipulation of knot and link diagramsused in the Tait flyping conjecture.It consists of twisting a part of a knot, a tangle: T by 180 degrees. Flype comes from an old Scottish word meaning to fold or to turn back. Two reduced alternating...

 preserves writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

. This was proven earlier by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

, Louis Kauffman

Louis Kauffman

Louis H. Kauffman is an American mathematician, topologist, and professor of Mathematics in the Department of Mathematics, Statistics, and Computer science at the University of Illinois at Chicago...

 and K. Murasugi in 1987.
For non-alternating knots this conjecture is not true, assuming so lead to the duplication of the Perko pair

Perko pair

In the mathematical theory of knots, the Perko pair, named after Kenneth Perko, is a pair of entries in classical knot tables that actually represent the same knot. In Rolfsen's knot table, this supposed pair of distinct knots is labeled 10161 and 10162...

, because it has two reduced projections with different writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

.
The flyping conjecture also implies this conjecture:

Alternating amphicheiral knots have even crossing number

Crossing number (knot theory)

In the mathematical area of knot theory, the crossing number of a knot is the minimal number of crossings of any diagram of the knot. It is a knot invariant....

.

This follows because a knot's mirror image has opposite writhe

Writhe

In knot theory, the writhe is a property of an oriented link diagram. The writhe is the total number of positive crossings minus the total number of negative crossings....

.
This one is also only true for alternating knots, a non-alternating amphichiral

Chirality (mathematics)

In geometry, a figure is chiral if it is not identical to its mirror image, or, more precisely, if it cannot be mapped to its mirror image by rotations and translations alone. For example, a right shoe is different from a left shoe, and clockwise is different from counterclockwise.A chiral object...

 knot with crossing number 15 was found, by Morwen Thistlethwaite

Morwen Thistlethwaite

Morwen B. Thistlethwaite is a knot theorist and professor of mathematics for the University of Tennessee in Knoxville. He has made important contributions to both knot theory, and Rubik's cube group theory.-Biography:...

.