Spin wave
Encyclopedia
Spin waves are propagating disturbances in the ordering of magnetic materials. These low-lying collective excitations occur in magnetic lattices with continuous symmetry
. From the equivalent quasiparticle point of view, spin waves are known as magnon
s, which are boson modes of the spin lattice that correspond roughly to the phonon
excitations of the nuclear lattice. As temperature is increased, the thermal excitation of spin waves reduces a ferromagnet's
spontaneous magnetization
. The energies of spin waves are typically only μeV in keeping with typical Curie point
s at room temperature and below. The discussion of spin waves in antiferromagnets is presently beyond the scope of this article.
The simplest way of understanding spin waves is to consider the Hamiltonian
for the Heisenberg ferromagnet:
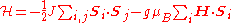
where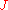
is the exchange energy, the operators 
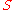represent the spins
at Bravais lattice points,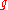
is the Landé g-factor
,
is the Bohr magneton and 
is the internal field which includes the external field plus any "molecular" field.
Note that in the classical continuum case and in 1+1 dimensions Heisenberg ferromagnet equation has the form
In 1+1, 2+1 and 3+1 dimensions this equation admits several integrable and non-integrable extensions like the Landau-Lifshitz equation, the Ishimori equation
and so on.
For a ferromagnet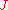
> 0 and the ground state of the Hamiltonian 
is that in which all spins are aligned parallel with the field 
. That 
is an eigenstate of 
can be verified by rewriting it in terms of the spin-raising and -lowering operators given by:
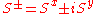
resulting in
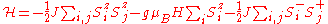
where
has been taken as the direction of the magnetic field. The spin-lowering operator 
annihilates the state with minimum projection of spin along the z-axis, while the spin-raising operator 
annihilates the ground state with maximum spin projection along the 
-axis. Since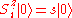 
for the maximally aligned state, we find
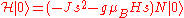
where N is the total number of Bravais lattice sites. The proposition that the ground state is an eigenstate of the Hamiltonian is confirmed.
One might guess that the first excited state of the Hamiltonian has one randomly selected spin at position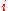
rotated so that 
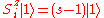, but in fact this arrangement of spins is not an eigenstate. The reason is that such a state is transformed by the spin raising and lowering operators. The operator 
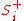will increase the z-projection of the spin at position 
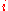back to its low-energy orientation, but the operator 
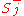will lower the z-projection of the spin at position 
.
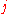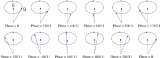
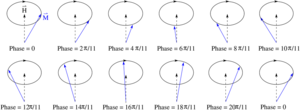
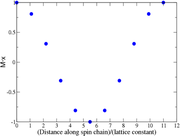
The combined effect of the two operators is therefore to propagate the rotated spin to a new position, which is a hint that the correct eigenstate is a spin wave, namely a superposition of states with one reduced spin. The exchange energy penalty associated with changing the orientation of one spin is reduced by spreading the disturbance over a long wavelength. The degree of misorientation of any two near-neighbor spins is thereby minimized.
From this explanation one can see why the Ising model
magnet with discrete symmetry
has no spin waves: the notion of spreading a disturbance in the spin lattice over a long wavelength makes no sense when spins have only two possible orientations. The existence of low-energy excitations is related to the fact that in the absence of an external field, the spin system has an infinite number of degenerate ground states with infinitesimally different spin orientations. That these ground states exist can be seen from the fact that the state
does not have the full rotational symmetry of the Hamiltonian 
, a phenomenon which is called spontaneous symmetry breaking
.
In this model the magnetization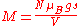
where 
is the volume. The propagation of spin waves is described by the Landau-Lifzhitz equation of motion:
where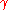
is the gyromagnetic ratio and 
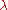is the damping constant. The cross-products in this forbidding-looking equation show that the propagation of spin waves is governed by the torques generated by internal and external fields. (An equivalent form is the Landau-Lifshitz-Gilbert equation, which replaces the final term by a more "simply looking" equivalent one.)
The first term on the r.h.s. describes the precession of the magnetization under the influence of the applied field, while the above-mentioned final term describes how the magnetization vector "spirals in" towards the field direction as time progresses. In metals the damping forces described by the constant
are in many cases dominated by the eddy currents.
One important difference between phonons and magnons lies in their dispersion relation
s. The dispersion relation for phonons is to first order linear in wavevector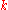
: 
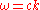, where 
is frequency, and 
is the velocity of sound. Magnons have a parabolic dispersion relation: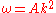 
where the parameter 
represents a "spin stiffness
." The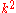
form is the third term of a Taylor expansion of a cosine term in the energy expression originating from the 
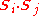dot-product.The underlying reason for the difference in dispersion relation is that ferromagnets violate time-reversal symmetry. Two adjacent spins in a solid with lattice constant 
that participate in a mode with wavevector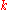 
have an angle between them equal to 
.
, inelastic light
scattering (Brillouin scattering
, Raman scattering
and inelastic X-ray
scattering), inelastic electron scattering (spin-resolved electron energy loss spectroscopy
), and spin-wave resonance (ferromagnetic resonance
). In the first method the energy loss of a beam of neutrons that excite a magnon is measured, typically as a function of scattering vector (or equivalently momentum transfer), temperature and external magnetic field. Inelastic neutron scattering measurements can determine the dispersion curve for magnons just as they can for phonons.
Important inelastic neutron scattering facilities are present at the ISIS neutron source
in Oxfordshire, UK, the Institut Laue-Langevin
in Grenoble
, France, the High Flux Isotope Reactor
at Oak Ridge National Laboratory
in Tennessee, USA, and at the National Institute of Standards and Technology
in Maryland, USA. Brillouin scattering similarly measures the energy loss of photon
s (usually at a convenient visible wavelength) reflected from or transmitted through a magnetic material. Brillouin spectroscopy is similar to the more widely known Raman scattering
but probes a lower energy and has a higher energy resolution in order to be able to detect the meV energy of magnons. Ferromagnetic (or antiferromagnetic) resonance instead measures the absorption of microwave
s, incident on a magnetic material, by spin waves, typically as a function of angle, temperature and applied field. Ferromagnetic resonance is a convenient laboratory method for determining the effect of magnetocrystalline anisotropy
on the dispersion of spin waves. Very recently, one group in Max Planck Institute for Microstructurephysics in Halle Germany proved that by using spin polarized electron energy loss spectroscopy (SPEELS), very high energy surface magnons can be exited. This technique allows people first time to probe the magnons and its dispersion in the ultrathin magnetical system. The first experiment was successful done in 5 ML Fe film by SPEELS, the signature of magnons were revealed. Later, with momentum resolution, magnon dispersion and full peak was explored in 8 ML fcc Co film on Cu(001) and 8 ML hcp Co on W(110), respectively. Those magnons are obtained up to the SBZ at the energy range about few hundreds meV.
components used in microwave
devices. The reciprocal of the lowest frequency of the characteristic spin waves of a magnetic material gives a time scale for the switching of a device based on that material.
Continuous symmetry
In mathematics, continuous symmetry is an intuitive idea corresponding to the concept of viewing some symmetries as motions, as opposed to e.g. reflection symmetry, which is invariance under a kind of flip from one state to another. It has largely and successfully been formalised in the...
. From the equivalent quasiparticle point of view, spin waves are known as magnon
Magnon
A magnon is a collective excitation of the electrons' spin structure in a crystal lattice. In contrast, a phonon is a collective excitation of the crystal lattice atoms or ions. In the equivalent wave picture of quantum mechanics, a magnon can be viewed as a quantized spin wave. As a...
s, which are boson modes of the spin lattice that correspond roughly to the phonon
Phonon
In physics, a phonon is a collective excitation in a periodic, elastic arrangement of atoms or molecules in condensed matter, such as solids and some liquids...
excitations of the nuclear lattice. As temperature is increased, the thermal excitation of spin waves reduces a ferromagnet's
Ferromagnetism
Ferromagnetism is the basic mechanism by which certain materials form permanent magnets, or are attracted to magnets. In physics, several different types of magnetism are distinguished...
spontaneous magnetization
Spontaneous magnetization
Spontaneous magnetization is the term used to describe the appearance of an ordered spin state at zero applied magnetic field in a ferromagnetic or ferrimagnetic material below a critical point called the Curie temperature or .-Overview:...
. The energies of spin waves are typically only μeV in keeping with typical Curie point
Curie point
In physics and materials science, the Curie temperature , or Curie point, is the temperature at which a ferromagnetic or a ferrimagnetic material becomes paramagnetic on heating; the effect is reversible. A magnet will lose its magnetism if heated above the Curie temperature...
s at room temperature and below. The discussion of spin waves in antiferromagnets is presently beyond the scope of this article.
Theory
Hamiltonian (quantum mechanics)
In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...
for the Heisenberg ferromagnet: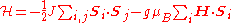where
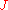is the exchange energy, the operators 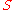represent the spinsSpin (physics)
In quantum mechanics and particle physics, spin is a fundamental characteristic property of elementary particles, composite particles , and atomic nuclei.It is worth noting that the intrinsic property of subatomic particles called spin and discussed in this article, is related in some small ways,...
at Bravais lattice points,
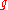is the Landé g-factorLandé g-factor
In physics, the Landé g-factor is a particular example of a g-factor, namely for an electron with both spin and orbital angular momenta. It is named after Alfred Landé, who first described it in 1921....
,
is the Bohr magneton and is the internal field which includes the external field plus any "molecular" field.Note that in the classical continuum case and in 1+1 dimensions Heisenberg ferromagnet equation has the form
In 1+1, 2+1 and 3+1 dimensions this equation admits several integrable and non-integrable extensions like the Landau-Lifshitz equation, the Ishimori equation
Ishimori equation
The Ishimori equation is a partial differential equation proposed by the Japanese mathematician . Its interest is as the first example of a nonlinear spin-one field model in the plane that is integrable .-Equation:The IE has the form...
and so on.
For a ferromagnet
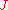> 0 and the ground state of the Hamiltonian is that in which all spins are aligned parallel with the field . That is an eigenstate of can be verified by rewriting it in terms of the spin-raising and -lowering operators given by: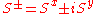resulting in
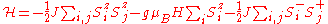
where
has been taken as the direction of the magnetic field. The spin-lowering operator annihilates the state with minimum projection of spin along the z-axis, while the spin-raising operator annihilates the ground state with maximum spin projection along the -axis. Since 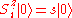for the maximally aligned state, we find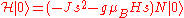where N is the total number of Bravais lattice sites. The proposition that the ground state is an eigenstate of the Hamiltonian is confirmed.
One might guess that the first excited state of the Hamiltonian has one randomly selected spin at position
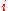rotated so that 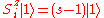, but in fact this arrangement of spins is not an eigenstate. The reason is that such a state is transformed by the spin raising and lowering operators. The operator 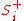will increase the z-projection of the spin at position 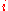back to its low-energy orientation, but the operator 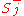will lower the z-projection of the spin at position 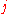.The combined effect of the two operators is therefore to propagate the rotated spin to a new position, which is a hint that the correct eigenstate is a spin wave, namely a superposition of states with one reduced spin. The exchange energy penalty associated with changing the orientation of one spin is reduced by spreading the disturbance over a long wavelength. The degree of misorientation of any two near-neighbor spins is thereby minimized.
From this explanation one can see why the Ising model
Ising model
The Ising model is a mathematical model of ferromagnetism in statistical mechanics. The model consists of discrete variables called spins that can be in one of two states . The spins are arranged in a graph , and each spin interacts with its nearest neighbors...
magnet with discrete symmetry
Discrete symmetry
A discrete symmetry is a symmetry that describes non-continuous changes in a system. For example, a square possesses discrete rotational symmetry, as only rotations by multiples of right angles will preserve the square's original appearance. Discrete symmetries sometimes involve some type of...
has no spin waves: the notion of spreading a disturbance in the spin lattice over a long wavelength makes no sense when spins have only two possible orientations. The existence of low-energy excitations is related to the fact that in the absence of an external field, the spin system has an infinite number of degenerate ground states with infinitesimally different spin orientations. That these ground states exist can be seen from the fact that the state
does not have the full rotational symmetry of the Hamiltonian , a phenomenon which is called spontaneous symmetry breakingSpontaneous symmetry breaking
Spontaneous symmetry breaking is the process by which a system described in a theoretically symmetrical way ends up in an apparently asymmetric state....
.
In this model the magnetization
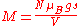where is the volume. The propagation of spin waves is described by the Landau-Lifzhitz equation of motion:where
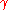is the gyromagnetic ratio and 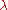is the damping constant. The cross-products in this forbidding-looking equation show that the propagation of spin waves is governed by the torques generated by internal and external fields. (An equivalent form is the Landau-Lifshitz-Gilbert equation, which replaces the final term by a more "simply looking" equivalent one.)The first term on the r.h.s. describes the precession of the magnetization under the influence of the applied field, while the above-mentioned final term describes how the magnetization vector "spirals in" towards the field direction as time progresses. In metals the damping forces described by the constant
are in many cases dominated by the eddy currents.One important difference between phonons and magnons lies in their dispersion relation
Dispersion relation
In physics and electrical engineering, dispersion most often refers to frequency-dependent effects in wave propagation. Note, however, that there are several other uses of the word "dispersion" in the physical sciences....
s. The dispersion relation for phonons is to first order linear in wavevector
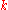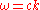: , where is frequency, and is the velocity of sound. Magnons have a parabolic dispersion relation: 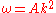where the parameter represents a "spin stiffnessSpin stiffness
The spin-stiffness or spin rigidity or helicity modulus or the "superfluid density" is a constant which represents the change in the ground state energy of a spin system as a result of introducing a slow in plane twist of the spins...
." The
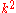form is the third term of a Taylor expansion of a cosine term in the energy expression originating from the 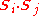dot-product.The underlying reason for the difference in dispersion relation is that ferromagnets violate time-reversal symmetry. Two adjacent spins in a solid with lattice constant that participate in a mode with wavevector 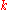have an angle between them equal to .Experimental observation
Spin waves are observed through four experimental methods: inelastic neutron scatteringNeutron scattering
Neutron scattering,the scattering of free neutrons by matter,is a physical processand an experimental technique using this processfor the investigation of materials.Neutron scattering as a physical process is of primordial importance...
, inelastic light
Light
Light or visible light is electromagnetic radiation that is visible to the human eye, and is responsible for the sense of sight. Visible light has wavelength in a range from about 380 nanometres to about 740 nm, with a frequency range of about 405 THz to 790 THz...
scattering (Brillouin scattering
Brillouin scattering
Brillouin scattering, named after Léon Brillouin, occurs when light in a medium interacts with time dependent optical density variations and changes its energy and path. The density variations may be due to acoustic modes, such as phonons, magnetic modes, such as magnons, or temperature gradients...
, Raman scattering
Raman scattering
Raman scattering or the Raman effect is the inelastic scattering of a photon. It was discovered by Sir Chandrasekhara Venkata Raman and Kariamanickam Srinivasa Krishnan in liquids, and by Grigory Landsberg and Leonid Mandelstam in crystals....
and inelastic X-ray
X-ray
X-radiation is a form of electromagnetic radiation. X-rays have a wavelength in the range of 0.01 to 10 nanometers, corresponding to frequencies in the range 30 petahertz to 30 exahertz and energies in the range 120 eV to 120 keV. They are shorter in wavelength than UV rays and longer than gamma...
scattering), inelastic electron scattering (spin-resolved electron energy loss spectroscopy
Electron energy loss spectroscopy
In electron energy loss spectroscopy a material is exposed to a beam of electrons with a known, narrow range of kinetic energies. Some of the electrons will undergo inelastic scattering, which means that they lose energy and have their paths slightly and randomly deflected...
), and spin-wave resonance (ferromagnetic resonance
Ferromagnetic resonance
Ferromagnetic resonance, or FMR, is a spectroscopic technique to probe the magnetization of ferromagnetic materials. It is a standard tool for probing spin waves and spin dynamics...
). In the first method the energy loss of a beam of neutrons that excite a magnon is measured, typically as a function of scattering vector (or equivalently momentum transfer), temperature and external magnetic field. Inelastic neutron scattering measurements can determine the dispersion curve for magnons just as they can for phonons.
Phonon
In physics, a phonon is a collective excitation in a periodic, elastic arrangement of atoms or molecules in condensed matter, such as solids and some liquids...
Important inelastic neutron scattering facilities are present at the ISIS neutron source
ISIS neutron source
ISIS is a pulsed neutron and muon source. It is situated at the Rutherford Appleton Laboratory on the Harwell Science and Innovation Campus in Oxfordshire, United Kingdom and is part of the Science and Technology Facilities Council...
in Oxfordshire, UK, the Institut Laue-Langevin
Institut Laue-Langevin
The Institut Laue–Langevin, or ILL, is an internationally-financed scientific facility, situated in Grenoble, France. It is one of the world centres for research using neutrons...
in Grenoble
Grenoble
Grenoble is a city in southeastern France, at the foot of the French Alps where the river Drac joins the Isère. Located in the Rhône-Alpes region, Grenoble is the capital of the department of Isère...
, France, the High Flux Isotope Reactor
High Flux Isotope Reactor
The High Flux Isotope Reactor is a nuclear research reactor located at Oak Ridge National Laboratory in Oak Ridge, Tennessee, United States...
at Oak Ridge National Laboratory
Oak Ridge National Laboratory
Oak Ridge National Laboratory is a multiprogram science and technology national laboratory managed for the United States Department of Energy by UT-Battelle. ORNL is the DOE's largest science and energy laboratory. ORNL is located in Oak Ridge, Tennessee, near Knoxville...
in Tennessee, USA, and at the National Institute of Standards and Technology
National Institute of Standards and Technology
The National Institute of Standards and Technology , known between 1901 and 1988 as the National Bureau of Standards , is a measurement standards laboratory, otherwise known as a National Metrological Institute , which is a non-regulatory agency of the United States Department of Commerce...
in Maryland, USA. Brillouin scattering similarly measures the energy loss of photon
Photon
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
s (usually at a convenient visible wavelength) reflected from or transmitted through a magnetic material. Brillouin spectroscopy is similar to the more widely known Raman scattering
Raman scattering
Raman scattering or the Raman effect is the inelastic scattering of a photon. It was discovered by Sir Chandrasekhara Venkata Raman and Kariamanickam Srinivasa Krishnan in liquids, and by Grigory Landsberg and Leonid Mandelstam in crystals....
but probes a lower energy and has a higher energy resolution in order to be able to detect the meV energy of magnons. Ferromagnetic (or antiferromagnetic) resonance instead measures the absorption of microwave
Microwave
Microwaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
s, incident on a magnetic material, by spin waves, typically as a function of angle, temperature and applied field. Ferromagnetic resonance is a convenient laboratory method for determining the effect of magnetocrystalline anisotropy
Magnetocrystalline anisotropy
Magnetocrystalline anisotropy is the dependence of the internal energy of a ferromagnet on the direction of its magnetization. As a result, certain crystallographic directions are preferred directions, or easy axes, for the magnetization. It is a special case of magnetic anisotropy...
on the dispersion of spin waves. Very recently, one group in Max Planck Institute for Microstructurephysics in Halle Germany proved that by using spin polarized electron energy loss spectroscopy (SPEELS), very high energy surface magnons can be exited. This technique allows people first time to probe the magnons and its dispersion in the ultrathin magnetical system. The first experiment was successful done in 5 ML Fe film by SPEELS, the signature of magnons were revealed. Later, with momentum resolution, magnon dispersion and full peak was explored in 8 ML fcc Co film on Cu(001) and 8 ML hcp Co on W(110), respectively. Those magnons are obtained up to the SBZ at the energy range about few hundreds meV.
Practical significance
When magnetoelectronic devices are operated at high frequencies, the generation of spin waves can be an important energy loss mechanism. Spin wave generation limits the linewidths and therefore the quality factors Q of ferriteFerrite (magnet)
Ferrites are chemical compounds consisting of ceramic materials with iron oxide as their principal component. Many of them are magnetic materials and they are used to make permanent magnets, ferrite cores for transformers, and in various other applications.Many ferrites are spinels with the...
components used in microwave
Microwave
Microwaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
devices. The reciprocal of the lowest frequency of the characteristic spin waves of a magnetic material gives a time scale for the switching of a device based on that material.