Preferred number
Encyclopedia
In industrial design
, preferred numbers (also called preferred values) are standard guidelines for choosing exact product dimensions within a given set of constraints.
Product developers must choose numerous lengths, distances, diameters, volumes, and other characteristic quantities. While all of these choices are constrained by considerations of functionality, usability, compatibility, safety or cost, there usually remains considerable leeway in the exact choice for many dimensions.
Preferred numbers serve two purposes:
proposed in the 1870s a set of preferred numbers for use with the metric system. His system was adopted in 1952 as international standard
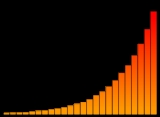
ISO 3. Renard's system of preferred numbers divides the interval from 1 to 10 into 5, 10, 20, or 40 steps. The factor between two consecutive numbers in a Renard series is constant (before rounding), namely the 5th, 10th, 20th, or 40th root of 10 (1.58, 1.26, 1.12, and 1.06, respectively), which leads to a geometric sequence. This way, the maximum relative error is minimized if an arbitrary number is replaced by the nearest Renard number multiplied by the appropriate power of 10.
These numbers may be rounded to any arbitrary precision, as they are irrational. R5, to various precisions:
Ones: 1 2 3 4 6
Tenths: 1.0 1.6 2.5 4.0 6.3
Hundredths: 1.00 1.58 2.51 3.98 6.31
Example: If our design constraints tell us that the two screws in our gadget should be placed between 32 mm and 55 mm apart, we make it 40 mm, because 4 is in the R5 series of preferred numbers.
Example: If you want to produce a set of nails with lengths between roughly 15 and 300 mm, then the application of the R5 series would lead to a product repertoire of 16 mm, 25 mm, 40 mm, 63 mm, 100 mm, 160 mm, and 250 mm long nails.
If a finer resolution is needed, another five numbers are added to the series, one after each of the original R5 numbers, and we end up with the R10 series:
R10: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
Where an even finer grading is needed, the R20, R40, and R80 series can be applied:
R20: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
R40: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.06 1.32 1.70 2.12 2.65 3.35 4.25 5.30 6.70 8.50
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
1.18 1.50 1.90 2.36 3.00 3.75 4.75 6.00 7.50 9.50
R80: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.03 1.28 1.65 2.06 2.58 3.25 4.12 5.15 6.50 8.25
1.06 1.32 1.70 2.12 2.65 3.35 4.25 5.30 6.70 8.50
1.09 1.36 1.75 2.18 2.72 3.45 4.37 5.45 6.90 8.75
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
1.15 1.45 1.85 2.30 2.90 3.65 4.62 5.80 7.30 9.25
1.18 1.50 1.90 2.36 3.00 3.75 4.75 6.00 7.50 9.50
1.22 1.55 1.95 2.43 3.07 3.87 4.87 6.15 7.75 9.75
In some applications more rounded values are desirable, either because the numbers from the normal series would imply an unrealistically high accuracy, or because an integer value is needed (e.g., the number of teeth in a gear). For these needs, more rounded versions of the Renard series have been defined in ISO 3:
R5": 1 1.5 2.5 4 6
R10': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
R10": 1 1.2 1.5 2 2.5 3 4 5 6 8
R20': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
1.1 1.4 1.8 2.2 2.8 3.6 4.5 5.6 7.1 9
R20": 1 1.2 1.5 2 2.5 3 4 5 6 8
1.1 1.4 1.8 2.2 2.8 3.5 4.5 5.5 7 9
R40': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
1.05 1.3 1.7 2.1 2.6 3.4 4.2 5.3 6.7 8.5
1.1 1.4 1.8 2.2 2.8 3.6 4.5 5.6 7.1 9
1.2 1.5 1.9 2.4 3 3.8 4.8 6 7.5 9.5
As the Renard numbers repeat after every 10-fold change of the scale, they are particularly well-suited for use with SI
units. It makes no difference whether the Renard numbers are used with metre
s or kilometre
s. But one would end up with two incompatible sets of nicely spaced dimensions if they were applied, for instance, with both yard
s and mile
s.
Renard numbers are rounded results of the formula
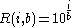 ,
,
where b is the selected series value (for example b = 40 for the R40 series), and i is the i-th element of this series (with i = 0 through i = b).
s are preferred numbers, with two exceptions. These are likely accidental, but remarkable in that they are in the R10 series whether expressed in inches or millimeters.
The more common gauge is the Irish gauge
, 63 inches, which rounds to 1600 millimeters, both numbers in the R10 series. It is also used in Australia and Brazil. The other gauge is just half this, 800 millimeters or 31.5 inches, and is used by the Wengernalpbahn
in Switzerland, between Lauterbrunnen and Grindelwald by way of Kleine Scheidegg.
is sometimes used as a cruder alternative:
This series covers a decade
(1:10 ratio) in three steps. Adjacent values differ by factors 2 or 2.5. Unlike the Renard series, the 1-2-5 series has not been formally adopted as an international standard
. However, the Renard series R10 can be used to extend the 1-2-5 series to a finer graduation.
This series is used to define the scales for graphs and for instruments that display in a two-dimensional form with a graticule, such as oscilloscopes.
The denominations
of most modern currencies follow a 1-2-5 series. An exception are some quarter-value coins, such as the Canadian quarter and the United States quarter (the latter denominated as "quarter dollar" rather than 25 cents). A ¼-½-1 series (... 0.1 0.25 0.5 1 2.5 5 10 ...) is used by currencies derived from the former Dutch gulden (Aruban florin
, Netherlands Antillean gulden
, Surinamese dollar), some Middle Eastern currencies (Iraqi
and Jordanian
dinars, Lebanese pound
, Syrian pound
), and the Seychellois rupee. However, newer notes introduced in Lebanon and Syria due to inflation follow the standard 1-2-5 series instead.
 In electronics
In electronics
, international standard IEC
60063 defines another preferred number series for resistor
s, capacitor
s, inductor
s and Zener diodes. It works similarly to the Renard series, except that it subdivides the interval from 1 to 10 into 6, 12, 24, etc. steps. These subdivisions ensure that when some arbitrary value is replaced with the nearest preferred number, the maximum relative error will be on the order of 20%, 10%, 5%, etc.
Use of the E series is mostly restricted to resistors, capacitors and inductors. Commonly produced dimensions for other types of electrical components are either chosen from the Renard series instead (for example fuses
) or are defined in relevant product standards (for example wires
).
The IEC 60063 numbers are as follows. The E6 series is every other element of the E12 series, which is in turn every other element of the E24 series:
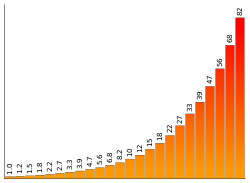
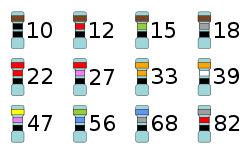
E6 ( 20%): 10 15 22 33 47 68
E12 ( 10%): 10 12 15 18 22 27 33 39 47 56 68 82
E24 ( 5%): 10 12 15 18 22 27 33 39 47 56 68 82
11 13 16 20 24 30 36 43 51 62 75 91
With the E48 series, a third decimal place is added, and the values are slightly adjusted. Again, the E48 series is every other value of the E96 series, which is every other value of the E192 series:
E48 ( 2%): 100 121 147 178 215 261 316 383 464 562 681 825
105 127 154 187 226 274 332 402 487 590 715 866
110 133 162 196 237 287 348 422 511 619 750 909
115 140 169 205 249 301 365 442 536 649 787 953
E96 ( 1%): 100 121 147 178 215 261 316 383 464 562 681 825
102 124 150 182 221 267 324 392 475 576 698 845
105 127 154 187 226 274 332 402 487 590 715 866
107 130 158 191 232 280 340 412 499 604 732 887
110 133 162 196 237 287 348 422 511 619 750 909
113 137 165 200 243 294 357 432 523 634 768 931
115 140 169 205 249 301 365 442 536 649 787 953
118 143 174 210 255 309 374 453 549 665 806 976
E192 (0.5%) 100 121 147 178 215 261 316 383 464 562 681 825
101 123 149 180 218 264 320 388 470 569 690 835
102 124 150 182 221 267 324 392 475 576 698 845
104 126 152 184 223 271 328 397 481 583 706 856
105 127 154 187 226 274 332 402 487 590 715 866
106 129 156 189 229 277 336 407 493 597 723 876
107 130 158 191 232 280 340 412 499 604 732 887
109 132 160 193 234 284 344 417 505 612 741 898
110 133 162 196 237 287 348 422 511 619 750 909
111 135 164 198 240 291 352 427 517 626 759 920
113 137 165 200 243 294 357 432 523 634 768 931
114 138 167 203 246 298 361 437 530 642 777 942
115 140 169 205 249 301 365 442 536 649 787 953
117 142 172 208 252 305 370 448 542 657 796 965
118 143 174 210 255 309 374 453 549 665 806 976
120 145 176 213 258 312 379 459 556 673 816 988
The E192 series is also used for 0.25% and 0.1% tolerance resistors.
1% resistors are available in both the E24 values and the E96 values.
Major dimensions (e.g., grid lines on drawings, distances between wall centers or surfaces, widths of shelves and kitchen components) are multiples of 100 mm, i.e. one decimetre
. This size is called the "basic module" (and represented in the standards by the letter M). Preference is given to the multiples of 300 mm (3 M) and 600 mm (6 M) of the basic module (see also "metric foot
"). For larger dimensions, preference is given to multiples of the modules 12 M (= 1.2 m), 15 M (= 1.5 m), 30 M (= 3 m), and 60 M (= 6 m). For smaller dimensions, the submodular increments 50 mm or 25 mm are used. (ISO
2848, BS
6750)
Dimensions chosen this way can easily be divided by a large number of factors without ending up with millimetre fractions. For example, a multiple of 600 mm (6 M) can always be divided into 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 25, 30, etc. parts, each of which is again an integral number of millimetres.
s use the square root
of two and related numbers (√√√2, √√2, √2, 2, or 2√2) as factors between neighbor dimensions (Lichtenberg
series, ISO 216
). The √2 factor also appears between the standard pen thicknesses for technical drawings (0.13, 0.18, 0.25, 0.35, 0.50, 0.70, 1.00, 1.40, and 2.00 mm). This way, the right pen size is available to continue a drawing that has been magnified to a different standard paper size.
1 2 4 8 16 32 64 128 256 512 1024 ...
Where a finer grading is needed, additional preferred numbers are obtained by multiplying a power of two with a small odd integer:
(×3) 6 12 24 48 96 192 384 768 1536 ...
(×5) 10 20 40 80 160 320 640 1280 2560 ...
(×7) 14 28 56 112 224 448 896 1792 3584 ...
In computer graphics, widths and heights of raster images
are preferred to be multiples of 16, as many compression algorithms (JPEG
, MPEG) divide color images into square blocks of that size. Black-and-white JPEG images are divided into 8x8 blocks. Screen resolutions often follow the same principle.
Preferred aspect ratio
s have also an important influence here, e.g. 2:1, 3:2, 4:3, 5:3, 5:4, 8:5, 16:9.
An example of such a regulation is the European Union directive on the volume of certain prepackaged liquids (75/106/EEC http://europa.eu/eur-lex/en/consleg/pdf/1975/en_1975L0106_do_001.pdf). It restricts the list of allowed wine-bottle sizes to 0.1, 0.25 (1/4), 0.375 (3/8), 0.5 (1/2), 0.75 (3/4), 1, 1.5, 2, 3, and 5 litres. Similar lists exist for several other types of products. They vary and often deviate significantly from any geometric series in order to accommodate traditional sizes when feasible. Adjacent package sizes in these lists differ typically by factors 2/3 or 3/4, in some cases even 1/2, 4/5, or some other ratio of two small integers.
, theremin
, etc.) can play a tone at any arbitrary frequency,
other instruments (such as pianos) can only play a limited set of tones.
The very popular "twelve-tone equal temperament" selects tones from the geometric sequence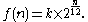
where k is typically 440 Hz
, though other standards have been used. However, other less common tuning systems have also been historically important as preferred audio frequencies.
Since 210≈103, 21/12≈103/120=101/40, and the resultant frequency spacing is very similar to the R40 series.
The aperture size controls how much light enters the camera. It's measured in f-stops
: f/1.4, f/2, f/2.8, f/4, etc. Full f-stops are a square root of 2 apart. Digital cameras often subdivide these into thirds, so each f-stop is a sixth root of 2, rounded to two significant digits: 1.0, 1.1, 1.2, 1.4, 1.6, 1.8, 2.0, 2.2, 2.5, 2.8, 3.2, 3.5, 4.0.
The film speed
(or digital equivalent) controls how quickly light is recorded. It's expressed as ISO values such as ISO 100, ISO 200, ISO 400, ISO 800. These are usually a power of 2 apart from each other, although other film speeds do exist.
The shutter speed
controls how long the camera records light. These are expressed as fractions of a second, roughly but not exactly based on powers of 2: 1 second, 1/2, 1/4, 1/8, 1/15, 1/30, 1/60, 1/125, 1/250, 1/500, 1/1000 of a second.
Industrial design
Industrial design is the use of a combination of applied art and applied science to improve the aesthetics, ergonomics, and usability of a product, but it may also be used to improve the product's marketability and production...
, preferred numbers (also called preferred values) are standard guidelines for choosing exact product dimensions within a given set of constraints.
Product developers must choose numerous lengths, distances, diameters, volumes, and other characteristic quantities. While all of these choices are constrained by considerations of functionality, usability, compatibility, safety or cost, there usually remains considerable leeway in the exact choice for many dimensions.
Preferred numbers serve two purposes:
- Using them increases the probability of compatibility between objects designed at different times by different people. In other words, it is one tactic among many in standardizationStandardizationStandardization is the process of developing and implementing technical standards.The goals of standardization can be to help with independence of single suppliers , compatibility, interoperability, safety, repeatability, or quality....
, whether within a company or within an industry, and it is usually desirable in industrial contexts. (The opposite motive can also apply, if it is in a manufacturer's financial interest: for example, manufacturers of consumer products often have a financial interest in lack of compatibility, in planned obsolescencePlanned obsolescencePlanned obsolescence or built-in obsolescence in industrial design is a policy of deliberately planning or designing a product with a limited useful life, so it will become obsolete or nonfunctional after a certain period of time...
, and in selling name-brand and model-specific replacement parts.) - They are chosen such that when a product is manufactured in many different sizes, these will end up roughly equally spaced on a logarithmic scaleLogarithmic scaleA logarithmic scale is a scale of measurement using the logarithm of a physical quantity instead of the quantity itself.A simple example is a chart whose vertical axis increments are labeled 1, 10, 100, 1000, instead of 1, 2, 3, 4...
. They therefore help to minimize the number of different sizes that need to be manufactured or kept in stock.
Renard numbers
The French army engineer Col. Charles RenardCharles Renard
Charles Renard was a French military engineer. After the Franco-Prussian War of 1870/71 he started work on the design of air ships at the French army aeronautical department. Together with Arthur C...
proposed in the 1870s a set of preferred numbers for use with the metric system. His system was adopted in 1952 as international standard
International standard
International standards are standards developed by international standards organizations. International standards are available for consideration and use, worldwide...
ISO 3. Renard's system of preferred numbers divides the interval from 1 to 10 into 5, 10, 20, or 40 steps. The factor between two consecutive numbers in a Renard series is constant (before rounding), namely the 5th, 10th, 20th, or 40th root of 10 (1.58, 1.26, 1.12, and 1.06, respectively), which leads to a geometric sequence. This way, the maximum relative error is minimized if an arbitrary number is replaced by the nearest Renard number multiplied by the appropriate power of 10.
These numbers may be rounded to any arbitrary precision, as they are irrational. R5, to various precisions:
Ones: 1 2 3 4 6
Tenths: 1.0 1.6 2.5 4.0 6.3
Hundredths: 1.00 1.58 2.51 3.98 6.31
Example: If our design constraints tell us that the two screws in our gadget should be placed between 32 mm and 55 mm apart, we make it 40 mm, because 4 is in the R5 series of preferred numbers.
Example: If you want to produce a set of nails with lengths between roughly 15 and 300 mm, then the application of the R5 series would lead to a product repertoire of 16 mm, 25 mm, 40 mm, 63 mm, 100 mm, 160 mm, and 250 mm long nails.
If a finer resolution is needed, another five numbers are added to the series, one after each of the original R5 numbers, and we end up with the R10 series:
R10: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
Where an even finer grading is needed, the R20, R40, and R80 series can be applied:
R20: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
R40: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.06 1.32 1.70 2.12 2.65 3.35 4.25 5.30 6.70 8.50
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
1.18 1.50 1.90 2.36 3.00 3.75 4.75 6.00 7.50 9.50
R80: 1.00 1.25 1.60 2.00 2.50 3.15 4.00 5.00 6.30 8.00
1.03 1.28 1.65 2.06 2.58 3.25 4.12 5.15 6.50 8.25
1.06 1.32 1.70 2.12 2.65 3.35 4.25 5.30 6.70 8.50
1.09 1.36 1.75 2.18 2.72 3.45 4.37 5.45 6.90 8.75
1.12 1.40 1.80 2.24 2.80 3.55 4.50 5.60 7.10 9.00
1.15 1.45 1.85 2.30 2.90 3.65 4.62 5.80 7.30 9.25
1.18 1.50 1.90 2.36 3.00 3.75 4.75 6.00 7.50 9.50
1.22 1.55 1.95 2.43 3.07 3.87 4.87 6.15 7.75 9.75
In some applications more rounded values are desirable, either because the numbers from the normal series would imply an unrealistically high accuracy, or because an integer value is needed (e.g., the number of teeth in a gear). For these needs, more rounded versions of the Renard series have been defined in ISO 3:
R5": 1 1.5 2.5 4 6
R10': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
R10": 1 1.2 1.5 2 2.5 3 4 5 6 8
R20': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
1.1 1.4 1.8 2.2 2.8 3.6 4.5 5.6 7.1 9
R20": 1 1.2 1.5 2 2.5 3 4 5 6 8
1.1 1.4 1.8 2.2 2.8 3.5 4.5 5.5 7 9
R40': 1 1.25 1.6 2 2.5 3.2 4 5 6.3 8
1.05 1.3 1.7 2.1 2.6 3.4 4.2 5.3 6.7 8.5
1.1 1.4 1.8 2.2 2.8 3.6 4.5 5.6 7.1 9
1.2 1.5 1.9 2.4 3 3.8 4.8 6 7.5 9.5
As the Renard numbers repeat after every 10-fold change of the scale, they are particularly well-suited for use with SI
Si
Si, si, or SI may refer to :- Measurement, mathematics and science :* International System of Units , the modern international standard version of the metric system...
units. It makes no difference whether the Renard numbers are used with metre
Metre
The metre , symbol m, is the base unit of length in the International System of Units . Originally intended to be one ten-millionth of the distance from the Earth's equator to the North Pole , its definition has been periodically refined to reflect growing knowledge of metrology...
s or kilometre
Kilometre
The kilometre is a unit of length in the metric system, equal to one thousand metres and is therefore exactly equal to the distance travelled by light in free space in of a second...
s. But one would end up with two incompatible sets of nicely spaced dimensions if they were applied, for instance, with both yard
Yard
A yard is a unit of length in several different systems including English units, Imperial units and United States customary units. It is equal to 3 feet or 36 inches...
s and mile
Mile
A mile is a unit of length, most commonly 5,280 feet . The mile of 5,280 feet is sometimes called the statute mile or land mile to distinguish it from the nautical mile...
s.
Renard numbers are rounded results of the formula
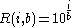 ,
,where b is the selected series value (for example b = 40 for the R40 series), and i is the i-th element of this series (with i = 0 through i = b).
Rail gauges
Virtually no rail gaugeRail gauge
Track gauge or rail gauge is the distance between the inner sides of the heads of the two load bearing rails that make up a single railway line. Sixty percent of the world's railways use a standard gauge of . Wider gauges are called broad gauge; smaller gauges, narrow gauge. Break-of-gauge refers...
s are preferred numbers, with two exceptions. These are likely accidental, but remarkable in that they are in the R10 series whether expressed in inches or millimeters.
The more common gauge is the Irish gauge
Irish gauge
Irish gauge railways use a track gauge of . It is used in* Ireland * Australia where it is also known as Victorian Broad Gauge* Brazil where it is also known as Bitola larga no Brasil....
, 63 inches, which rounds to 1600 millimeters, both numbers in the R10 series. It is also used in Australia and Brazil. The other gauge is just half this, 800 millimeters or 31.5 inches, and is used by the Wengernalpbahn
Wengernalpbahn
Wengernalpbahn is a 19.091 km long, gauge rack railway line in Switzerland, which runs from Lauterbrunnen and Grindelwald to Kleine Scheidegg, making it the world's longest continuous rack and pinion railway....
in Switzerland, between Lauterbrunnen and Grindelwald by way of Kleine Scheidegg.
1-2-5 series
In applications for which the R5 series provides a too fine graduation, the 1-2-5 seriesis sometimes used as a cruder alternative:
- ... 0.1 0.2 0.5 1 2 5 10 20 50 100 200 500 1000 ...
This series covers a decade
Decade (log scale)
One decade is a factor of 10 difference between two numbers measured on a logarithmic scale. It is especially useful when referring to frequencies and when describing frequency response of electronic systems, such as audio amplifiers and filters.-Calculations:The factor-of-ten in a decade can be...
(1:10 ratio) in three steps. Adjacent values differ by factors 2 or 2.5. Unlike the Renard series, the 1-2-5 series has not been formally adopted as an international standard
International standard
International standards are standards developed by international standards organizations. International standards are available for consideration and use, worldwide...
. However, the Renard series R10 can be used to extend the 1-2-5 series to a finer graduation.
This series is used to define the scales for graphs and for instruments that display in a two-dimensional form with a graticule, such as oscilloscopes.
The denominations
Denomination (currency)
Denomination is a proper description of a currency amount, usually for coins or banknotes. Denominations may also be used with other means of payment like gift cards. See also Redenomination.-Subunit and super unit:...
of most modern currencies follow a 1-2-5 series. An exception are some quarter-value coins, such as the Canadian quarter and the United States quarter (the latter denominated as "quarter dollar" rather than 25 cents). A ¼-½-1 series (... 0.1 0.25 0.5 1 2.5 5 10 ...) is used by currencies derived from the former Dutch gulden (Aruban florin
Aruban florin
The florin is the currency of Aruba. It is subdivided into 100 cents. The florin was introduced in 1986, replacing the Netherlands Antillean guilder at par.-History:...
, Netherlands Antillean gulden
Netherlands Antillean gulden
The guilder is presently the currency of two of the five islands which until 2010 formed the Netherlands Antilles. It is subdivided into 100 cents . The guilder was replaced by the US dollar on 1 January 2011 on the other former islands of the Netherlands Antilles: Bonaire, Saba and Sint Eustatius...
, Surinamese dollar), some Middle Eastern currencies (Iraqi
Iraqi dinar
The dinar is the currency of Iraq. It is issued by the Central Bank of Iraq and is subdivided into 1,000 fils , although inflation has rendered the fils obsolete.-History:...
and Jordanian
Jordanian dinar
The dinar is the currency of Jordan. The dinar is divided into 10 dirham, 100 qirsh or 1000 fils....
dinars, Lebanese pound
Lebanese pound
The Lebanese pound is the currency unit of Lebanon. It is divided into 100 piastres but inflation has eliminated the subdivisions....
, Syrian pound
Syrian pound
-Use of 10 Syrian pound coins in Norway:The shape of the 10 Syrian pound coin has been found to so resemble the 20 Norwegian krone coin that it can fool vending machines, coins-to-cash machines, arcade machines, and any other coin-operated, automated service machine in the country...
), and the Seychellois rupee. However, newer notes introduced in Lebanon and Syria due to inflation follow the standard 1-2-5 series instead.
E series


Electronics
Electronics is the branch of science, engineering and technology that deals with electrical circuits involving active electrical components such as vacuum tubes, transistors, diodes and integrated circuits, and associated passive interconnection technologies...
, international standard IEC
International Electrotechnical Commission
The International Electrotechnical Commission is a non-profit, non-governmental international standards organization that prepares and publishes International Standards for all electrical, electronic and related technologies – collectively known as "electrotechnology"...
60063 defines another preferred number series for resistor
Resistor
A linear resistor is a linear, passive two-terminal electrical component that implements electrical resistance as a circuit element.The current through a resistor is in direct proportion to the voltage across the resistor's terminals. Thus, the ratio of the voltage applied across a resistor's...
s, capacitor
Capacitor
A capacitor is a passive two-terminal electrical component used to store energy in an electric field. The forms of practical capacitors vary widely, but all contain at least two electrical conductors separated by a dielectric ; for example, one common construction consists of metal foils separated...
s, inductor
Inductor
An inductor is a passive two-terminal electrical component used to store energy in a magnetic field. An inductor's ability to store magnetic energy is measured by its inductance, in units of henries...
s and Zener diodes. It works similarly to the Renard series, except that it subdivides the interval from 1 to 10 into 6, 12, 24, etc. steps. These subdivisions ensure that when some arbitrary value is replaced with the nearest preferred number, the maximum relative error will be on the order of 20%, 10%, 5%, etc.
Use of the E series is mostly restricted to resistors, capacitors and inductors. Commonly produced dimensions for other types of electrical components are either chosen from the Renard series instead (for example fuses
Fuse (electrical)
In electronics and electrical engineering, a fuse is a type of low resistance resistor that acts as a sacrificial device to provide overcurrent protection, of either the load or source circuit...
) or are defined in relevant product standards (for example wires
IEC 60228
IEC 60228 is the International Electrotechnical Commission's international standard on conductors of insulated cables.Among other things, it defines a set of standard wire cross-sectional areas:...
).
The IEC 60063 numbers are as follows. The E6 series is every other element of the E12 series, which is in turn every other element of the E24 series:
E6 ( 20%): 10 15 22 33 47 68
E12 ( 10%): 10 12 15 18 22 27 33 39 47 56 68 82
E24 ( 5%): 10 12 15 18 22 27 33 39 47 56 68 82
11 13 16 20 24 30 36 43 51 62 75 91
With the E48 series, a third decimal place is added, and the values are slightly adjusted. Again, the E48 series is every other value of the E96 series, which is every other value of the E192 series:
E48 ( 2%): 100 121 147 178 215 261 316 383 464 562 681 825
105 127 154 187 226 274 332 402 487 590 715 866
110 133 162 196 237 287 348 422 511 619 750 909
115 140 169 205 249 301 365 442 536 649 787 953
E96 ( 1%): 100 121 147 178 215 261 316 383 464 562 681 825
102 124 150 182 221 267 324 392 475 576 698 845
105 127 154 187 226 274 332 402 487 590 715 866
107 130 158 191 232 280 340 412 499 604 732 887
110 133 162 196 237 287 348 422 511 619 750 909
113 137 165 200 243 294 357 432 523 634 768 931
115 140 169 205 249 301 365 442 536 649 787 953
118 143 174 210 255 309 374 453 549 665 806 976
E192 (0.5%) 100 121 147 178 215 261 316 383 464 562 681 825
101 123 149 180 218 264 320 388 470 569 690 835
102 124 150 182 221 267 324 392 475 576 698 845
104 126 152 184 223 271 328 397 481 583 706 856
105 127 154 187 226 274 332 402 487 590 715 866
106 129 156 189 229 277 336 407 493 597 723 876
107 130 158 191 232 280 340 412 499 604 732 887
109 132 160 193 234 284 344 417 505 612 741 898
110 133 162 196 237 287 348 422 511 619 750 909
111 135 164 198 240 291 352 427 517 626 759 920
113 137 165 200 243 294 357 432 523 634 768 931
114 138 167 203 246 298 361 437 530 642 777 942
115 140 169 205 249 301 365 442 536 649 787 953
117 142 172 208 252 305 370 448 542 657 796 965
118 143 174 210 255 309 374 453 549 665 806 976
120 145 176 213 258 312 379 459 556 673 816 988
The E192 series is also used for 0.25% and 0.1% tolerance resistors.
1% resistors are available in both the E24 values and the E96 values.
Buildings
In the construction industry, it was felt that typical dimensions must be easy to use in mental arithmetic. Therefore, rather than using elements of a geometric series, a different system of preferred dimensions has evolved in this area, known as "modular coordination".Major dimensions (e.g., grid lines on drawings, distances between wall centers or surfaces, widths of shelves and kitchen components) are multiples of 100 mm, i.e. one decimetre
Decimetre
A decimetre is a unit of length in the metric system, equal to one tenth of a metre, the SI base unit of length. In simple words there are 10 cm in a decimetre....
. This size is called the "basic module" (and represented in the standards by the letter M). Preference is given to the multiples of 300 mm (3 M) and 600 mm (6 M) of the basic module (see also "metric foot
Metric foot
A metric foot is a nickname occasionally used in the United Kingdom for a length of 300 millimetres . A metric foot can be divided into twelve "metric inches" of 25 millimetres each...
"). For larger dimensions, preference is given to multiples of the modules 12 M (= 1.2 m), 15 M (= 1.5 m), 30 M (= 3 m), and 60 M (= 6 m). For smaller dimensions, the submodular increments 50 mm or 25 mm are used. (ISO
International Organization for Standardization
The International Organization for Standardization , widely known as ISO, is an international standard-setting body composed of representatives from various national standards organizations. Founded on February 23, 1947, the organization promulgates worldwide proprietary, industrial and commercial...
2848, BS
British Standards
British Standards are the standards produced by BSI Group which is incorporated under a Royal Charter...
6750)
Dimensions chosen this way can easily be divided by a large number of factors without ending up with millimetre fractions. For example, a multiple of 600 mm (6 M) can always be divided into 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 25, 30, etc. parts, each of which is again an integral number of millimetres.
Paper documents, envelopes, and drawing pens
Standard metric paper sizePaper size
Many paper size standards conventions have existed at different times and in different countries. Today there is one widespread international ISO standard and a localised standard used in North America . The paper sizes affect writing paper, stationery, cards, and some printed documents...
s use the square root
Square root
In mathematics, a square root of a number x is a number r such that r2 = x, or, in other words, a number r whose square is x...
of two and related numbers (√√√2, √√2, √2, 2, or 2√2) as factors between neighbor dimensions (Lichtenberg
Georg Christoph Lichtenberg
Georg Christoph Lichtenberg was a German scientist, satirist and Anglophile. As a scientist, he was the first to hold a professorship explicitly dedicated to experimental physics in Germany...
series, ISO 216
ISO 216
ISO 216 specifies international standard paper sizes used in most countries in the world today. It defines the "A" and "B" series of paper sizes, including A4, the most commonly available size...
). The √2 factor also appears between the standard pen thicknesses for technical drawings (0.13, 0.18, 0.25, 0.35, 0.50, 0.70, 1.00, 1.40, and 2.00 mm). This way, the right pen size is available to continue a drawing that has been magnified to a different standard paper size.
Computer engineering
When dimensioning computer components, the powers of two are frequently used as preferred numbers:1 2 4 8 16 32 64 128 256 512 1024 ...
Where a finer grading is needed, additional preferred numbers are obtained by multiplying a power of two with a small odd integer:
(×3) 6 12 24 48 96 192 384 768 1536 ...
(×5) 10 20 40 80 160 320 640 1280 2560 ...
(×7) 14 28 56 112 224 448 896 1792 3584 ...
| 16: | 15: | 12: | |
|---|---|---|---|
| :8 | 2:1 | 3:2 | |
| :9 | 16:9 | 5:3 | 4:3 |
| :10 | 8:5 | 3:2 | |
| :12 | 4:3 | 5:4 | 1:1 |
In computer graphics, widths and heights of raster images
Raster graphics
In computer graphics, a raster graphics image, or bitmap, is a data structure representing a generally rectangular grid of pixels, or points of color, viewable via a monitor, paper, or other display medium...
are preferred to be multiples of 16, as many compression algorithms (JPEG
JPEG
In computing, JPEG . The degree of compression can be adjusted, allowing a selectable tradeoff between storage size and image quality. JPEG typically achieves 10:1 compression with little perceptible loss in image quality....
, MPEG) divide color images into square blocks of that size. Black-and-white JPEG images are divided into 8x8 blocks. Screen resolutions often follow the same principle.
Preferred aspect ratio
Aspect ratio
The aspect ratio of a shape is the ratio of its longer dimension to its shorter dimension. It may be applied to two characteristic dimensions of a three-dimensional shape, such as the ratio of the longest and shortest axis, or for symmetrical objects that are described by just two measurements,...
s have also an important influence here, e.g. 2:1, 3:2, 4:3, 5:3, 5:4, 8:5, 16:9.
Retail packaging
In some countries, consumer-protection laws restrict the number of different prepackaged sizes in which certain products can be sold, in order to make it easier for consumers to compare prices.An example of such a regulation is the European Union directive on the volume of certain prepackaged liquids (75/106/EEC http://europa.eu/eur-lex/en/consleg/pdf/1975/en_1975L0106_do_001.pdf). It restricts the list of allowed wine-bottle sizes to 0.1, 0.25 (1/4), 0.375 (3/8), 0.5 (1/2), 0.75 (3/4), 1, 1.5, 2, 3, and 5 litres. Similar lists exist for several other types of products. They vary and often deviate significantly from any geometric series in order to accommodate traditional sizes when feasible. Adjacent package sizes in these lists differ typically by factors 2/3 or 3/4, in some cases even 1/2, 4/5, or some other ratio of two small integers.
Music
While some instruments (tromboneTrombone
The trombone is a musical instrument in the brass family. Like all brass instruments, sound is produced when the player’s vibrating lips cause the air column inside the instrument to vibrate...
, theremin
Theremin
The theremin , originally known as the aetherphone/etherophone, thereminophone or termenvox/thereminvox is an early electronic musical instrument controlled without discernible physical contact from the player. It is named after its Russian inventor, Professor Léon Theremin, who patented the device...
, etc.) can play a tone at any arbitrary frequency,
other instruments (such as pianos) can only play a limited set of tones.
The very popular "twelve-tone equal temperament" selects tones from the geometric sequence
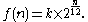
where k is typically 440 Hz
A440 (Concert A)
A440 is the musical note A above middle C. It has a frequency of 440 Hz and serves as a general tuning standard for musical pitch.Prior to the standardization on 440 Hz, many countries and organizations followed the Austrian government's 1885 recommendation of 435 Hz...
, though other standards have been used. However, other less common tuning systems have also been historically important as preferred audio frequencies.
Since 210≈103, 21/12≈103/120=101/40, and the resultant frequency spacing is very similar to the R40 series.
Photography
In photography, aperture, exposure, and film speed generally follow powers of 2:The aperture size controls how much light enters the camera. It's measured in f-stops
F-number
In optics, the f-number of an optical system expresses the diameter of the entrance pupil in terms of the focal length of the lens; in simpler terms, the f-number is the focal length divided by the "effective" aperture diameter...
: f/1.4, f/2, f/2.8, f/4, etc. Full f-stops are a square root of 2 apart. Digital cameras often subdivide these into thirds, so each f-stop is a sixth root of 2, rounded to two significant digits: 1.0, 1.1, 1.2, 1.4, 1.6, 1.8, 2.0, 2.2, 2.5, 2.8, 3.2, 3.5, 4.0.
The film speed
Film speed
Film speed is the measure of a photographic film's sensitivity to light, determined by sensitometry and measured on various numerical scales, the most recent being the ISO system....
(or digital equivalent) controls how quickly light is recorded. It's expressed as ISO values such as ISO 100, ISO 200, ISO 400, ISO 800. These are usually a power of 2 apart from each other, although other film speeds do exist.
The shutter speed
Shutter speed
In photography, shutter speed is a common term used to discuss exposure time, the effective length of time a camera's shutter is open....
controls how long the camera records light. These are expressed as fractions of a second, roughly but not exactly based on powers of 2: 1 second, 1/2, 1/4, 1/8, 1/15, 1/30, 1/60, 1/125, 1/250, 1/500, 1/1000 of a second.

