Truncated cuboctahedron
Encyclopedia
In geometry
, the truncated cuboctahedron is an Archimedean solid
. It has 12 square
faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges. Since each of its faces has point symmetry (equivalently, 180° rotation
al symmetry), the truncated cuboctahedron is a zonohedron
.
The Cartesian coordinates for the vertices of a truncated cuboctahedron having edge length 2 and centered at the origin are all permutations of:
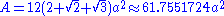
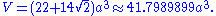
Therefore, there may seem to exist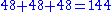
vertices. However, we have over-counted the vertices thrice since a square, hexagon, and octagon meet at each vertex. Consequently, we divide 144 by 3 to correct for our over-counting: 
.
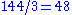has edge lengths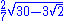
, 
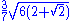and 
.
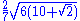of the faces of this polyhedron, one color for each face type.
A 2-uniform coloring also exists with alternately colored hexagons.
The name truncated cuboctahedron, given originally by Johannes Kepler
, is a little misleading. If you truncate
a cuboctahedron
by cutting the corners off, you do not get this uniform figure: some of the faces will be rectangle
s. However, the resulting figure is topologically
equivalent to a truncated cuboctahedron and can always be deformed until the faces are regular.
The alternative name great rhombicuboctahedron refers to the fact that the 12 square faces lie in the same planes as the 12 faces of the rhombic dodecahedron
which is dual to the cuboctahedron. Compare to small rhombicuboctahedron.
One unfortunate point of confusion: There is a nonconvex uniform polyhedron
by the same name. See nonconvex great rhombicuboctahedron.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the truncated cuboctahedron is an Archimedean solid
Archimedean solid
In geometry an Archimedean solid is a highly symmetric, semi-regular convex polyhedron composed of two or more types of regular polygons meeting in identical vertices...
. It has 12 square
Square (geometry)
In geometry, a square is a regular quadrilateral. This means that it has four equal sides and four equal angles...
faces, 8 regular hexagonal faces, 6 regular octagonal faces, 48 vertices and 72 edges. Since each of its faces has point symmetry (equivalently, 180° rotation
Rotation
A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...
al symmetry), the truncated cuboctahedron is a zonohedron
Zonohedron
A zonohedron is a convex polyhedron where every face is a polygon with point symmetry or, equivalently, symmetry under rotations through 180°. Any zonohedron may equivalently be described as the Minkowski sum of a set of line segments in three-dimensional space, or as the three-dimensional...
.
Cartesian coordinates
The Cartesian coordinates for the vertices of a truncated cuboctahedron having edge length 2 and centered at the origin are all permutations of:
- (±1, ±(1+√2), ±(1+2√2))
Area and volume
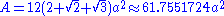
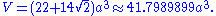
The area A and the volume V of the truncated cuboctahedron of edge length a are:Vertices
To derive the number of vertices, we note that each vertex is the meeting point of a square, hexagon, and octagon.- Each of the 12 squares with their 4 vertices contribute 48 vertices because
. - Each of the 8 hexagons with their 6 vertices contribute 48 vertices because
. - Each of the 6 octagons with their 8 vertices contribute 48 vertices because
.
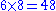Therefore, there may seem to exist
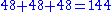vertices. However, we have over-counted the vertices thrice since a square, hexagon, and octagon meet at each vertex. Consequently, we divide 144 by 3 to correct for our over-counting: 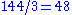.Dual
If the original truncated cuboctahedron has edge length 1, its dual disdyakis dodecahedronDisdyakis dodecahedron
In geometry, a disdyakis dodecahedron, or hexakis octahedron, is a Catalan solid and the dual to the Archimedean truncated cuboctahedron. As such it is face-transitive but with irregular face polygons...
has edge lengths
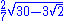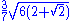, and 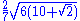.Uniform colorings
There is only one uniform coloringUniform coloring
In geometry, a uniform coloring is a property of a uniform figure that is colored to be vertex-transitive...
of the faces of this polyhedron, one color for each face type.
A 2-uniform coloring also exists with alternately colored hexagons.
Other names
Alternate interchangeable names are:- Truncated cuboctahedron (Johannes KeplerJohannes KeplerJohannes Kepler was a German mathematician, astronomer and astrologer. A key figure in the 17th century scientific revolution, he is best known for his eponymous laws of planetary motion, codified by later astronomers, based on his works Astronomia nova, Harmonices Mundi, and Epitome of Copernican...
) - Rhombitruncated cuboctahedron (Magnus WenningerMagnus WenningerFather Magnus J. Wenninger OSB is a mathematician who works on constructing polyhedron models, and wrote the first book on their construction.-Early life and education:...
) - Great rhombicuboctahedron (Robert Williams)
- Great rhombcuboctahedron (Peter Cromwell)
- Omnitruncated cube or cantitruncated cube (Norman Johnson)
The name truncated cuboctahedron, given originally by Johannes Kepler
Johannes Kepler
Johannes Kepler was a German mathematician, astronomer and astrologer. A key figure in the 17th century scientific revolution, he is best known for his eponymous laws of planetary motion, codified by later astronomers, based on his works Astronomia nova, Harmonices Mundi, and Epitome of Copernican...
, is a little misleading. If you truncate
Truncation (geometry)
In geometry, a truncation is an operation in any dimension that cuts polytope vertices, creating a new facet in place of each vertex.- Uniform truncation :...
a cuboctahedron
Cuboctahedron
In geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron,...
by cutting the corners off, you do not get this uniform figure: some of the faces will be rectangle
Rectangle
In Euclidean plane geometry, a rectangle is any quadrilateral with four right angles. The term "oblong" is occasionally used to refer to a non-square rectangle...
s. However, the resulting figure is topologically
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
equivalent to a truncated cuboctahedron and can always be deformed until the faces are regular.
The alternative name great rhombicuboctahedron refers to the fact that the 12 square faces lie in the same planes as the 12 faces of the rhombic dodecahedron
Rhombic dodecahedron
In geometry, the rhombic dodecahedron is a convex polyhedron with 12 rhombic faces. It is an Archimedean dual solid, or a Catalan solid. Its dual is the cuboctahedron.-Properties:...
which is dual to the cuboctahedron. Compare to small rhombicuboctahedron.
One unfortunate point of confusion: There is a nonconvex uniform polyhedron
Nonconvex uniform polyhedron
In geometry, a uniform star polyhedron is a self-intersecting uniform polyhedron. They are also sometimes called nonconvex polyhedra to imply self-intersecting...
by the same name. See nonconvex great rhombicuboctahedron.
See also
- cube
- cuboctahedronCuboctahedronIn geometry, a cuboctahedron is a polyhedron with eight triangular faces and six square faces. A cuboctahedron has 12 identical vertices, with two triangles and two squares meeting at each, and 24 identical edges, each separating a triangle from a square. As such it is a quasiregular polyhedron,...
- octahedronOctahedronIn geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex....
- truncated icosidodecahedronTruncated icosidodecahedronIn geometry, the truncated icosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed by two or more types of regular polygon faces....
- Truncated octahedronTruncated octahedronIn geometry, the truncated octahedron is an Archimedean solid. It has 14 faces , 36 edges, and 24 vertices. Since each of its faces has point symmetry the truncated octahedron is a zonohedron....
- truncated tetratetrahedron
External links
- http://www.dr-mikes-math-games-for-kids.com/polyhedral-nets.html?net=K1RB4IgXRIYFsRCy4aHC6dnfSy7rq2q4f3v4yl4nRAbEtZul3CvUo1k8TrBjkgTqbZvaXjrwBrfylwUDWC8lfzMM1h1E0NDKFJh7udiMaOsp1DWdM1xR11UHzHVHzVTY4gCc1nRXW879CvTzcUwX9wUb4vJUXWfCEBFZvcR1dJrJyE4FpNhyWBHvw6Qyuph3rT9jgDZRKNn8FaEqBr5dZ1o9SBpnEObkMMx31INNrEf75EZOWGdEXwvDNeJIuKbtorvRJHy4iYcMHnYO1v1axRBQudr31fS0np5Jn7nuzR0Vx&name=Truncated+Cuboctahedron#appletEditable printable net of a truncated cuboctahedron with interactive 3D view]
- The Uniform Polyhedra
- Virtual Reality Polyhedra The Encyclopedia of Polyhedra
- great Rhombicuboctahedron: paper strips for plaiting