
Thermodynamic potentials
Encyclopedia
A thermodynamic potential is a scalar function
used to represent the thermodynamic state
of a system
. The concept of thermodynamic potentials was introduced by Pierre Duhem
in 1886. Josiah Willard Gibbs
in his papers used the term fundamental functions. One main thermodynamic potential that has a physical interpretation is the internal energy
, U. It is the energy of configuration of a given system of conservative force
s (that is why it is a potential) and only has meaning with respect to a defined set of references (or data
). Expressions for all other thermodynamic energy potentials are derivable via Legendre transforms from an expression for U. In thermodynamics
, certain forces, such as gravity, are typically disregarded when formulating expressions for potentials. For example, while all the working fluid
in a steam engine
may have higher energy due to gravity while sitting on top of Mt. Everest than it would at the bottom of the Mariana trench
, the gravitational potential energy term in the formula for the internal energy would usually be ignored because changes in gravitational potential within the engine during operation would be negligible.
where T = temperature
, S = entropy
, p = pressure
, V = volume. The Helmholtz free energy is often denoted by the symbol F, but the use of A is preferred by IUPAC. is the number of particles of type i in the system and
is the number of particles of type i in the system and 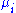 is the chemical potential
is the chemical potential
for an i-type particle. For the sake of completeness, the set of all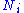 are also included as natural variables, although they are sometimes ignored.
are also included as natural variables, although they are sometimes ignored.
These five common potentials are all energy potentials, but there are also entropy potentials
. The thermodynamic square
can be used as a tool to recall and derive some of the potentials.
Just as in mechanics
, where potential energy
is defined as capacity to do work, similarly different potentials have different meanings. Internal energy
(U) is the capacity to do work plus the capacity to release heat. Gibbs energy is the capacity to do non-mechanical work. Enthalpy
is the capacity to do non-mechanical work plus the capacity to release heat. Helmholtz free energy is the capacity to do mechanical work (useful work). From these definitions we can say that ΔU is the energy added to the system, ΔF is the total work done on it, ΔG is the non-mechanical work done on it, and ΔH is the sum of non-mechanical work done on the system and the heat given to it.
Thermodynamic potentials are very useful when calculating the equilibrium results of a chemical reaction
, or when measuring the properties of materials in a chemical reaction. The chemical reactions usually take place under some simple constraints such as constant pressure and temperature, or constant entropy and volume, and when this is true, there is a corresponding thermodynamic potential that comes into play. Just as in mechanics, the system will tend towards lower values of potential and at equilibrium, under these constraints, the potential will take on an unchanging minimum value. The thermodynamic potentials can also be used to estimate the total amount of energy available from a thermodynamic system under the appropriate constraint.
In particular: (see principle of minimum energy
for a derivation)
Notice that the set of natural variables for the above four potentials are formed from every combination of the T-S and P-V variables, excluding any pairs of conjugate variables
. There is no reason to ignore the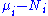 conjugate pairs, and in fact we may define four additional potentials for each species. Using IUPAC notation in which the brackets contain the natural variables (other than the main four), we have:
conjugate pairs, and in fact we may define four additional potentials for each species. Using IUPAC notation in which the brackets contain the natural variables (other than the main four), we have:
If there is only one species, then we are done. But, if there are, say, two species, then there will be additional potentials such as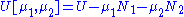 and so on. If there are
and so on. If there are  dimensions to the thermodynamic space, then there are
dimensions to the thermodynamic space, then there are 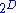 unique thermodynamic potentials. For the most simple case, a single phase ideal gas, there will be three dimensions, yielding eight thermodynamic potentials.
unique thermodynamic potentials. For the most simple case, a single phase ideal gas, there will be three dimensions, yielding eight thermodynamic potentials.
In statistical mechanics
,
the relationship between the Helmholtz free energy
and the
partition function
is fundamental, and is used to calculate the
thermodynamic properties
of matters; see
configuration integral
for more details.
, any differential change in the internal energy U of a system can be written as the sum of heat flowing into the system and work done by the system on the environment, along with any change due to the addition of new particles to the system:
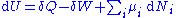
where is the infinitesimal
is the infinitesimal
heat flow into the system, and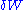 is the infinitesimal work done by the system,
is the infinitesimal work done by the system,  is the chemical potential
is the chemical potential
of particle type i and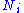 is the number of type i particles. (Note that neither
is the number of type i particles. (Note that neither 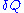 nor
nor 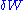 is exact differential
is exact differential
s. Small changes in these variables are, therefore, represented with δ rather than d.)
By the second law of thermodynamics
, we can express the internal energy change in terms of state functions and their differentials. In case of quasistatic changes we have:
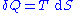
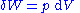
where
and V is volume, and the equality holds for reversible processes.
This leads to the standard differential form of the internal energy in case of a quasistatic reversible change:
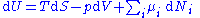
Since U, S and V are thermodynamic functions of state, the above relation holds also for arbitrary non-reversible changes. If the system has more external variables than just the volume that can change, the fundamental thermodynamic relation generalizes to:
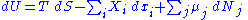
Here the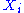 are the generalized forces corresponding to the external variables
are the generalized forces corresponding to the external variables 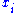 .
.
Applying Legendre transforms
repeatedly, the following differential relations hold for the four potentials:
Note that the infinitesimals on the right-hand side of each of the above equations are of the natural variables of the potential on the left-hand side.
Similar equations can be developed for all of the other thermodynamic potentials of the system. There will be one fundamental equation for each thermodynamic potential, resulting in a total of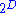 fundamental equations.
fundamental equations.
The differences between the four thermodynamic potentials can be summarized as follows:
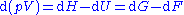
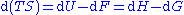
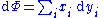
where and
and 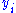 are conjugate pairs, and the
are conjugate pairs, and the  are the natural variables of the potential
are the natural variables of the potential 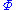 . From the chain rule
. From the chain rule
it follows that:
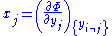
Where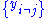 is the set of all natural variables of
is the set of all natural variables of 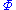 except
except  . This yields expressions for various thermodynamic parameters in terms of the derivatives of the potentials with respect to their natural variables. These equations are known as equations of state since they specify parameters of the thermodynamic state. If we restrict ourselves to the potentials U,F,H and G, then we have:
. This yields expressions for various thermodynamic parameters in terms of the derivatives of the potentials with respect to their natural variables. These equations are known as equations of state since they specify parameters of the thermodynamic state. If we restrict ourselves to the potentials U,F,H and G, then we have:
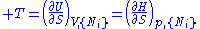
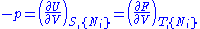
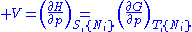
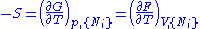
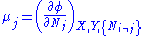
where, in the last equation, is any of the thermodynamic potentials U, F, H, G and
is any of the thermodynamic potentials U, F, H, G and  are the set of natural variables for that potential, excluding
are the set of natural variables for that potential, excluding  . If we use all potentials, then we will have more equations of state such as
. If we use all potentials, then we will have more equations of state such as
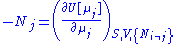
and so on. In all, there will be D equations for each potential, resulting in a total of D 2D equations of state. If the D equations of state for a particular potential are known, then the fundamental equation for that potential can be determined. This means that all thermodynamic information about the system will be known, and that the fundamental equations for any other potential can be found, along with the corresponding equations of state.
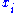 and
and 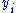 to be conjugate pairs, and the
to be conjugate pairs, and the  to be the natural variables of some potential
to be the natural variables of some potential 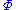 . We may take the "cross differentials" of the state equations, which obey the following relationship:
. We may take the "cross differentials" of the state equations, which obey the following relationship:
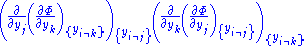
From these we get the Maxwell relations
. There will be (D-1)/2 of them for each potential giving a total of D(D-1)/2 equations in all. If we restrict ourselves the U, F, H, G
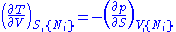
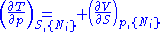
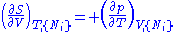

Using the equations of state involving the chemical potential we get equations such as:
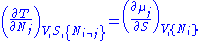
and using the other potentials we can get equations such as:
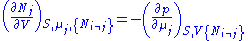
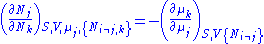
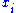 and
and 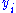 to be conjugate pairs, and the
to be conjugate pairs, and the 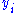 to be the natural variables of the internal energy.
to be the natural variables of the internal energy.
Since all of the natural variables of the internal energy U are extensive quantities
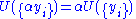
it follows from Euler's homogeneous function theorem
that the internal energy can be written as:
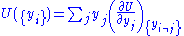
From the equations of state, we then have:
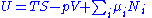
Substituting into the expressions for the other main potentials we have:
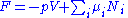

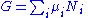
As in the above sections, this process can be carried out on all of the other thermodynamic potentials. Note that the Euler integrals are sometimes also referred to as fundamental equations.
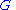 can be expanded locally at equilibrium
can be expanded locally at equilibrium
in terms of the thermodynamic state as:
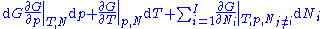
With the substitution of two of the Maxwell relations
and the definition of chemical potential
, this is transformed into:
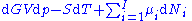
The chemical potential is just another name for the partial molar Gibbs free energy, and as such: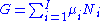
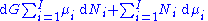
Subtracting yields the Gibbs–Duhem relation:

The Gibbs–Duhem is a relationship among the intensive parameters of the system. It follows that for a simple system with components, there will be
components, there will be  independent parameters, or degrees of freedom. For example, a simple system with a single component will have two degrees of freedom, and may be specified by only two parameters, such as pressure and volume for example. The law is named after Josiah Willard Gibbs
independent parameters, or degrees of freedom. For example, a simple system with a single component will have two degrees of freedom, and may be specified by only two parameters, such as pressure and volume for example. The law is named after Josiah Willard Gibbs
and Pierre Duhem
.
Most commonly one considers reactions at constant p and T, so the Gibbs free energy is the most useful potential in studies of chemical reactions.
Scalar field
In mathematics and physics, a scalar field associates a scalar value to every point in a space. The scalar may either be a mathematical number, or a physical quantity. Scalar fields are required to be coordinate-independent, meaning that any two observers using the same units will agree on the...
used to represent the thermodynamic state
Thermodynamic state
A thermodynamic state is a set of values of properties of a thermodynamic system that must be specified to reproduce the system. The individual parameters are known as state variables, state parameters or thermodynamic variables. Once a sufficient set of thermodynamic variables have been...
of a system
Physical system
In physics, the word system has a technical meaning, namely, it is the portion of the physical universe chosen for analysis. Everything outside the system is known as the environment, which in analysis is ignored except for its effects on the system. The cut between system and the world is a free...
. The concept of thermodynamic potentials was introduced by Pierre Duhem
Pierre Duhem
Pierre Maurice Marie Duhem was a French physicist, mathematician and philosopher of science, best known for his writings on the indeterminacy of experimental criteria and on scientific development in the Middle Ages...
in 1886. Josiah Willard Gibbs
Josiah Willard Gibbs
Josiah Willard Gibbs was an American theoretical physicist, chemist, and mathematician. He devised much of the theoretical foundation for chemical thermodynamics as well as physical chemistry. As a mathematician, he invented vector analysis . Yale University awarded Gibbs the first American Ph.D...
in his papers used the term fundamental functions. One main thermodynamic potential that has a physical interpretation is the internal energy
Internal energy
In thermodynamics, the internal energy is the total energy contained by a thermodynamic system. It is the energy needed to create the system, but excludes the energy to displace the system's surroundings, any energy associated with a move as a whole, or due to external force fields. Internal...
, U. It is the energy of configuration of a given system of conservative force
Conservative force
A conservative force is a force with the property that the work done in moving a particle between two points is independent of the path taken. Equivalently, if a particle travels in a closed loop, the net work done by a conservative force is zero.It is possible to define a numerical value of...
s (that is why it is a potential) and only has meaning with respect to a defined set of references (or data
Datum
A geodetic datum is a reference from which measurements are made. In surveying and geodesy, a datum is a set of reference points on the Earth's surface against which position measurements are made, and an associated model of the shape of the earth to define a geographic coordinate system...
). Expressions for all other thermodynamic energy potentials are derivable via Legendre transforms from an expression for U. In thermodynamics
Thermodynamics
Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...
, certain forces, such as gravity, are typically disregarded when formulating expressions for potentials. For example, while all the working fluid
Working fluid
A working fluid is a pressurized gas or liquid that actuates a machine. Examples include steam in a steam engine, air in a hot air engine and hydraulic fluid in a hydraulic motor or hydraulic cylinder...
in a steam engine
Steam engine
A steam engine is a heat engine that performs mechanical work using steam as its working fluid.Steam engines are external combustion engines, where the working fluid is separate from the combustion products. Non-combustion heat sources such as solar power, nuclear power or geothermal energy may be...
may have higher energy due to gravity while sitting on top of Mt. Everest than it would at the bottom of the Mariana trench
Mariana Trench
The Mariana Trench or Marianas Trench is the deepest part of the world's oceans. It is located in the western Pacific Ocean, to the east of the Mariana Islands. The trench is about long but has a mean width of only...
, the gravitational potential energy term in the formula for the internal energy would usually be ignored because changes in gravitational potential within the engine during operation would be negligible.
Description and interpretation
Five common thermodynamic potentials are:where T = temperature
Temperature
Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
, S = entropy
Entropy
Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...
, p = pressure
Pressure
Pressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
, V = volume. The Helmholtz free energy is often denoted by the symbol F, but the use of A is preferred by IUPAC.
 is the number of particles of type i in the system and
is the number of particles of type i in the system and 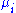 is the chemical potential
is the chemical potentialChemical potential
Chemical potential, symbolized by μ, is a measure first described by the American engineer, chemist and mathematical physicist Josiah Willard Gibbs. It is the potential that a substance has to produce in order to alter a system...
for an i-type particle. For the sake of completeness, the set of all
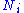 are also included as natural variables, although they are sometimes ignored.
are also included as natural variables, although they are sometimes ignored.These five common potentials are all energy potentials, but there are also entropy potentials
Free entropy
A thermodynamic free entropy is an entropic thermodynamic potential analogous to the free energy. Also known as a Massieu, Planck, or Massieu-Planck potentials , or free information. In statistical mechanics, free entropies frequently appear as the logarithm of a partition function...
. The thermodynamic square
Thermodynamic square
The thermodynamic square is a mnemonic diagram used to help determine thermodynamic relations. The corners represent common conjugate variables while the sides represent thermodynamic potentials...
can be used as a tool to recall and derive some of the potentials.
Just as in mechanics
Mechanics
Mechanics is the branch of physics concerned with the behavior of physical bodies when subjected to forces or displacements, and the subsequent effects of the bodies on their environment....
, where potential energy
Potential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
is defined as capacity to do work, similarly different potentials have different meanings. Internal energy
Internal energy
In thermodynamics, the internal energy is the total energy contained by a thermodynamic system. It is the energy needed to create the system, but excludes the energy to displace the system's surroundings, any energy associated with a move as a whole, or due to external force fields. Internal...
(U) is the capacity to do work plus the capacity to release heat. Gibbs energy is the capacity to do non-mechanical work. Enthalpy
Enthalpy
Enthalpy is a measure of the total energy of a thermodynamic system. It includes the internal energy, which is the energy required to create a system, and the amount of energy required to make room for it by displacing its environment and establishing its volume and pressure.Enthalpy is a...
is the capacity to do non-mechanical work plus the capacity to release heat. Helmholtz free energy is the capacity to do mechanical work (useful work). From these definitions we can say that ΔU is the energy added to the system, ΔF is the total work done on it, ΔG is the non-mechanical work done on it, and ΔH is the sum of non-mechanical work done on the system and the heat given to it.
Thermodynamic potentials are very useful when calculating the equilibrium results of a chemical reaction
Chemical equilibrium
In a chemical reaction, chemical equilibrium is the state in which the concentrations of the reactants and products have not yet changed with time. It occurs only in reversible reactions, and not in irreversible reactions. Usually, this state results when the forward reaction proceeds at the same...
, or when measuring the properties of materials in a chemical reaction. The chemical reactions usually take place under some simple constraints such as constant pressure and temperature, or constant entropy and volume, and when this is true, there is a corresponding thermodynamic potential that comes into play. Just as in mechanics, the system will tend towards lower values of potential and at equilibrium, under these constraints, the potential will take on an unchanging minimum value. The thermodynamic potentials can also be used to estimate the total amount of energy available from a thermodynamic system under the appropriate constraint.
In particular: (see principle of minimum energy
Principle of minimum energy
The principle of minimum energy is essentially a restatement of the second law of thermodynamics. It states that for a closed system, with constant external parameters and entropy, the internal energy will decrease and approach a minimum value at equilibrium...
for a derivation)
- When the entropy (S ) and "external parameters" (e.g. volume) of a closed systemClosed system-In physics:In thermodynamics, a closed system can exchange energy , but not matter, with its surroundings.In contrast, an isolated system cannot exchange any of heat, work, or matter with the surroundings, while an open system can exchange all of heat, work and matter.For a simple system, with...
are held constant, the internal energy (U ) decreases and reaches a minimum value at equilibrium. This follows from the first and second laws of thermodynamics and is called the principle of minimum energy. The following three statements are directly derivable from this principle. - When the temperature (T ) and external parameters of a closed system are held constant, the Helmholtz free energy (A ) decreases and reaches a minimum value at equilibrium.
- When the pressure (p ) and external parameters of a closed system are held constant, the enthalpy (H ) decreases and reaches a minimum value at equilibrium.
- When the temperature (T ), pressure (p ) and external parameters of a closed system are held constant, the Gibbs free energy (G ) decreases and reaches a minimum value at equilibrium.
Natural variables
The variables that are held constant in this process are termed the natural variables of that potential. The natural variables are important not only for the above mentioned reason, but also because if a thermodynamic potential can be determined as a function of its natural variables, all of the thermodynamic properties of the system can be found by taking partial derivatives of that potential with respect to its natural variables and this is true for no other combination of variables. On the converse, if a thermodynamic potential is not given as a function of its natural variables, it will not, in general, yield all of the thermodynamic properties of the system.Notice that the set of natural variables for the above four potentials are formed from every combination of the T-S and P-V variables, excluding any pairs of conjugate variables
Conjugate variables (thermodynamics)
In thermodynamics, the internal energy of a system is expressed in terms of pairs of conjugate variables such as temperature/entropy or pressure/volume. In fact all thermodynamic potentials are expressed in terms of conjugate pairs....
. There is no reason to ignore the
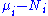 conjugate pairs, and in fact we may define four additional potentials for each species. Using IUPAC notation in which the brackets contain the natural variables (other than the main four), we have:
conjugate pairs, and in fact we may define four additional potentials for each species. Using IUPAC notation in which the brackets contain the natural variables (other than the main four), we have:| Formula | Natural variables |
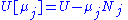 |
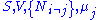 |
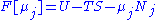 |
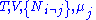 |
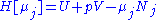 |
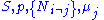 |
 |
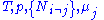 |
If there is only one species, then we are done. But, if there are, say, two species, then there will be additional potentials such as
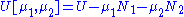 and so on. If there are
and so on. If there are  dimensions to the thermodynamic space, then there are
dimensions to the thermodynamic space, then there are 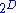 unique thermodynamic potentials. For the most simple case, a single phase ideal gas, there will be three dimensions, yielding eight thermodynamic potentials.
unique thermodynamic potentials. For the most simple case, a single phase ideal gas, there will be three dimensions, yielding eight thermodynamic potentials.In statistical mechanics
Statistical mechanics
Statistical mechanics or statistical thermodynamicsThe terms statistical mechanics and statistical thermodynamics are used interchangeably...
,
the relationship between the Helmholtz free energy
and the
partition function
Partition function (statistical mechanics)
Partition functions describe the statistical properties of a system in thermodynamic equilibrium. It is a function of temperature and other parameters, such as the volume enclosing a gas...
is fundamental, and is used to calculate the
thermodynamic properties
of matters; see
configuration integral
for more details.
The fundamental equations
The definitions of the thermodynamic potentials may be differentiated and, along with the first and second laws of thermodynamics, a set of differential equations known as the fundamental equations follow. By the first law of thermodynamicsFirst law of thermodynamics
The first law of thermodynamics is an expression of the principle of conservation of work.The law states that energy can be transformed, i.e. changed from one form to another, but cannot be created nor destroyed...
, any differential change in the internal energy U of a system can be written as the sum of heat flowing into the system and work done by the system on the environment, along with any change due to the addition of new particles to the system:
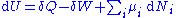
where
 is the infinitesimal
is the infinitesimalInfinitesimal
Infinitesimals have been used to express the idea of objects so small that there is no way to see them or to measure them. The word infinitesimal comes from a 17th century Modern Latin coinage infinitesimus, which originally referred to the "infinite-th" item in a series.In common speech, an...
heat flow into the system, and
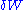 is the infinitesimal work done by the system,
is the infinitesimal work done by the system,  is the chemical potential
is the chemical potentialChemical potential
Chemical potential, symbolized by μ, is a measure first described by the American engineer, chemist and mathematical physicist Josiah Willard Gibbs. It is the potential that a substance has to produce in order to alter a system...
of particle type i and
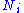 is the number of type i particles. (Note that neither
is the number of type i particles. (Note that neither 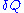 nor
nor 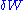 is exact differential
is exact differentialExact differential
A mathematical differential is said to be exact, as contrasted with an inexact differential, if it is of the form dQ, for some differentiable function Q....
s. Small changes in these variables are, therefore, represented with δ rather than d.)
By the second law of thermodynamics
Second law of thermodynamics
The second law of thermodynamics is an expression of the tendency that over time, differences in temperature, pressure, and chemical potential equilibrate in an isolated physical system. From the state of thermodynamic equilibrium, the law deduced the principle of the increase of entropy and...
, we can express the internal energy change in terms of state functions and their differentials. In case of quasistatic changes we have:
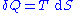
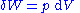
where
- T is temperatureTemperatureTemperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
, - S is entropyEntropyEntropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...
, - p is pressurePressurePressure is the force per unit area applied in a direction perpendicular to the surface of an object. Gauge pressure is the pressure relative to the local atmospheric or ambient pressure.- Definition :...
,
and V is volume, and the equality holds for reversible processes.
This leads to the standard differential form of the internal energy in case of a quasistatic reversible change:
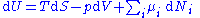
Since U, S and V are thermodynamic functions of state, the above relation holds also for arbitrary non-reversible changes. If the system has more external variables than just the volume that can change, the fundamental thermodynamic relation generalizes to:
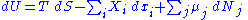
Here the
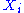 are the generalized forces corresponding to the external variables
are the generalized forces corresponding to the external variables 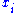 .
.Applying Legendre transforms
Legendre transformation
In mathematics, the Legendre transformation or Legendre transform, named after Adrien-Marie Legendre, is an operation that transforms one real-valued function of a real variable into another...
repeatedly, the following differential relations hold for the four potentials:
 |
 |
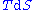 |
 |
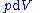 |
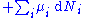 |
|
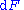 |
 |
 |
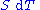 |
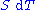 |
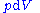 |
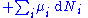 |
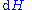 |
 |
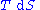 |
 |
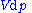 |
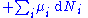 |
|
 |
 |
 |
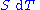 |
 |
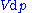 |
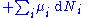 |
Note that the infinitesimals on the right-hand side of each of the above equations are of the natural variables of the potential on the left-hand side.
Similar equations can be developed for all of the other thermodynamic potentials of the system. There will be one fundamental equation for each thermodynamic potential, resulting in a total of
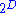 fundamental equations.
fundamental equations.The differences between the four thermodynamic potentials can be summarized as follows:
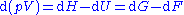
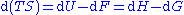
The equations of state
We can use the above equations to derive some differential definitions of some thermodynamic parameters. If we define Φ to stand for any of the thermodynamic potentials, then the above equations are of the form: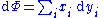
where
 and
and 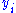 are conjugate pairs, and the
are conjugate pairs, and the  are the natural variables of the potential
are the natural variables of the potential 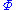 . From the chain rule
. From the chain ruleChain rule
In calculus, the chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f is a function and g is a function, then the chain rule expresses the derivative of the composite function in terms of the derivatives of f and g.In integration, the...
it follows that:
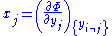
Where
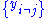 is the set of all natural variables of
is the set of all natural variables of 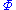 except
except  . This yields expressions for various thermodynamic parameters in terms of the derivatives of the potentials with respect to their natural variables. These equations are known as equations of state since they specify parameters of the thermodynamic state. If we restrict ourselves to the potentials U,F,H and G, then we have:
. This yields expressions for various thermodynamic parameters in terms of the derivatives of the potentials with respect to their natural variables. These equations are known as equations of state since they specify parameters of the thermodynamic state. If we restrict ourselves to the potentials U,F,H and G, then we have: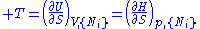
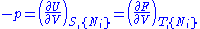
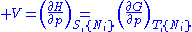
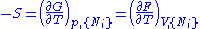
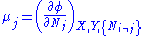
where, in the last equation,
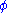 is any of the thermodynamic potentials U, F, H, G and
is any of the thermodynamic potentials U, F, H, G and  are the set of natural variables for that potential, excluding
are the set of natural variables for that potential, excluding 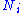 . If we use all potentials, then we will have more equations of state such as
. If we use all potentials, then we will have more equations of state such as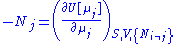
and so on. In all, there will be D equations for each potential, resulting in a total of D 2D equations of state. If the D equations of state for a particular potential are known, then the fundamental equation for that potential can be determined. This means that all thermodynamic information about the system will be known, and that the fundamental equations for any other potential can be found, along with the corresponding equations of state.
The Maxwell relations
Again, define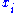 and
and 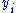 to be conjugate pairs, and the
to be conjugate pairs, and the  to be the natural variables of some potential
to be the natural variables of some potential 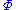 . We may take the "cross differentials" of the state equations, which obey the following relationship:
. We may take the "cross differentials" of the state equations, which obey the following relationship: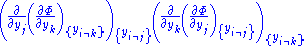
From these we get the Maxwell relations
Maxwell relations
Maxwell's relations are a set of equations in thermodynamics which are derivable from the definitions of the thermodynamic potentials. The Maxwell relations are statements of equality among the second derivatives of the thermodynamic potentials. They follow directly from the fact that the order of...
. There will be (D-1)/2 of them for each potential giving a total of D(D-1)/2 equations in all. If we restrict ourselves the U, F, H, G
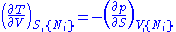
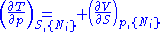
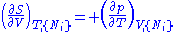

Using the equations of state involving the chemical potential we get equations such as:
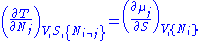
and using the other potentials we can get equations such as:
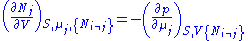
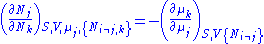
Euler integrals
Again, define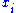 and
and 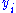 to be conjugate pairs, and the
to be conjugate pairs, and the 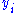 to be the natural variables of the internal energy.
to be the natural variables of the internal energy.Since all of the natural variables of the internal energy U are extensive quantities
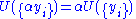
it follows from Euler's homogeneous function theorem
Homogeneous function
In mathematics, a homogeneous function is a function with multiplicative scaling behaviour: if the argument is multiplied by a factor, then the result is multiplied by some power of this factor. More precisely, if is a function between two vector spaces over a field F, and k is an integer, then...
that the internal energy can be written as:
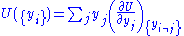
From the equations of state, we then have:
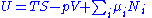
Substituting into the expressions for the other main potentials we have:
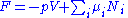

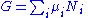
As in the above sections, this process can be carried out on all of the other thermodynamic potentials. Note that the Euler integrals are sometimes also referred to as fundamental equations.
The Gibbs–Duhem relation
Deriving the Gibbs–Duhem equation from basic thermodynamic state equations is straightforward. The Gibbs free energyGibbs free energy
In thermodynamics, the Gibbs free energy is a thermodynamic potential that measures the "useful" or process-initiating work obtainable from a thermodynamic system at a constant temperature and pressure...
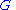 can be expanded locally at equilibrium
can be expanded locally at equilibriumChemical equilibrium
In a chemical reaction, chemical equilibrium is the state in which the concentrations of the reactants and products have not yet changed with time. It occurs only in reversible reactions, and not in irreversible reactions. Usually, this state results when the forward reaction proceeds at the same...
in terms of the thermodynamic state as:
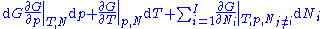
With the substitution of two of the Maxwell relations
Maxwell relations
Maxwell's relations are a set of equations in thermodynamics which are derivable from the definitions of the thermodynamic potentials. The Maxwell relations are statements of equality among the second derivatives of the thermodynamic potentials. They follow directly from the fact that the order of...
and the definition of chemical potential
Chemical potential
Chemical potential, symbolized by μ, is a measure first described by the American engineer, chemist and mathematical physicist Josiah Willard Gibbs. It is the potential that a substance has to produce in order to alter a system...
, this is transformed into:
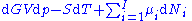
The chemical potential is just another name for the partial molar Gibbs free energy, and as such:
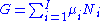
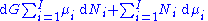
Subtracting yields the Gibbs–Duhem relation:

The Gibbs–Duhem is a relationship among the intensive parameters of the system. It follows that for a simple system with
 components, there will be
components, there will be  independent parameters, or degrees of freedom. For example, a simple system with a single component will have two degrees of freedom, and may be specified by only two parameters, such as pressure and volume for example. The law is named after Josiah Willard Gibbs
independent parameters, or degrees of freedom. For example, a simple system with a single component will have two degrees of freedom, and may be specified by only two parameters, such as pressure and volume for example. The law is named after Josiah Willard GibbsJosiah Willard Gibbs
Josiah Willard Gibbs was an American theoretical physicist, chemist, and mathematician. He devised much of the theoretical foundation for chemical thermodynamics as well as physical chemistry. As a mathematician, he invented vector analysis . Yale University awarded Gibbs the first American Ph.D...
and Pierre Duhem
Pierre Duhem
Pierre Maurice Marie Duhem was a French physicist, mathematician and philosopher of science, best known for his writings on the indeterminacy of experimental criteria and on scientific development in the Middle Ages...
.
Chemical reactions
Changes in these quantities are useful for assessing the degree to which a chemical reaction will proceed. The relevant quantity depends on the reaction conditions, as shown in the following table. Δ denotes the change in the potential and at equilibrium the change will be zero.| Constant V | Constant p | |
|---|---|---|
| Constant S | ΔU | ΔH |
| Constant T | ΔF | ΔG |
Most commonly one considers reactions at constant p and T, so the Gibbs free energy is the most useful potential in studies of chemical reactions.
External links
- Thermodynamic Potentials - Georgia State University
- Chemical Potential Energy: The 'Characteristic' vs the Concentration-Dependent Kind

