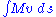Principle of least action
Encyclopedia
In physics
, the principle of least action – or, more accurately, the principle of stationary action – is a variational principle
that, when applied to the action
of a mechanical
system, can be used to obtain the equations of motion for that system. The principle led to the development of the Lagrangian
and Hamiltonian
formulations of classical mechanics
.
The principle remains central in modern physics
and mathematics
, being applied in the theory of relativity
, quantum mechanics
and quantum field theory
, and a focus of modern mathematical investigation in Morse theory
. This article deals primarily with the historical development of the idea; a treatment of the mathematical description and derivation can be found in the article on the action
. The chief examples of the principle of stationary action are Maupertuis' principle
and Hamilton's principle
.
The action principle is preceded by earlier ideas in surveying
and optics
. The rope stretchers of ancient Egypt
stretched corded ropes between two points to measure the path which minimized the distance of separation, and Claudius Ptolemy, in his Geographia
(Bk 1, Ch 2), emphasized that one must correct for "deviations from a straight course"; in ancient Greece
Euclid
states in his Catoptrica that, for the path of light reflecting from a mirror, the angle of incidence
equals the angle of reflection; and Hero of Alexandria
later showed that this path was the shortest length and least time. But the credit for the formulation of the principle as it applies to the action is often given to Pierre-Louis Moreau de Maupertuis
, who wrote about it in 1744 and 1746. However, scholarship indicates that this claim of priority is not so clear; Leonhard Euler
discussed the principle in 1744, and there is evidence that Gottfried Leibniz
preceded both by 39 years.
postulated that "light travels between two given points along the path of shortest time," which is known as the principle of least time or Fermat's principle
.
, who wrote about it in 1744 and 1746. Maupertuis felt that "Nature is thrifty in all its actions", and applied the principle broadly:
This notion of Maupertuis, although somewhat deterministic today, does capture much of the essence of mechanics.
In application to physics, Maupertuis suggested that the quantity to be minimized was the product of the duration (time) of movement within a system by the "vis viva
", twice what we now call the kinetic energy
T of the system.
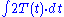
gave a formulation of the action principle in 1744, in very recognizable terms, in the Additamentum 2 to his Methodus Inveniendi Lineas Curvas Maximi Minive Proprietate Gaudentes. He begins the second paragraph :
As Euler states,
is the integral of the momentum over distance traveled, which, in modern notation, equals the reduced action
Thus, Euler made an equivalent and (apparently) independent statement of the variational principle in the same year as Maupertuis, albeit slightly later. Curiously, Euler did not claim any priority, as the following episode shows.
in 1707. Although similar to many of Leibniz's arguments, the principle itself has not been documented in Leibniz's works. König himself showed a copy of a 1707 letter from Leibniz to Jacob Hermann with the principle, but the original letter has been lost. In contentious proceedings, König was accused of forgery, and even the King of Prussia entered the debate, defending Maupertuis, while Voltaire
defended König.
Euler, rather than claiming priority, was a staunch defender of Maupertuis, and Euler himself prosecuted König for forgery before the Berlin Academy on 13 April 1752. The claims of forgery were re-examined 150 years later, and archival work by C.I. Gerhardt in 1898 and W. Kabitz in 1913 uncovered other copies of the letter, and three others cited by König, in the Bernoulli
archives.
, so that his statement of least action in statics is equivalent to the principle that a system of bodies at rest will adopt a configuration that minimizes total potential energy.
in 1760 and he proceeded to apply this to problems in dynamics. In Méchanique Analytique (1788) Lagrange derived the general equations of motion of a mechanical body. William Rowan Hamilton
in 1834 and 1835 applied the variational principle to the classical Lagrangian function
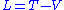to obtain the Euler-Lagrange equations in their present form.
in the 1920s and 1930s, leading to what is now known as Morse theory
. For example, Morse showed that the number of conjugate points
in a trajectory equalled the number of negative eigenvalues in the second variation of the Lagrangian.
have been formulated, such as Gauss' principle of least constraint and its corollary, Hertz's principle of least curvature.
equations of motion and their integral
counterpart has important philosophical implications. The differential equations are statements about quantities localized to a single point in space or single moment of time. For example, Newton's second law
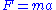states that the instantaneous force 
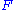applied to a mass 
produces an acceleration 
at the same instant. By contrast, the action principle is not localized to a point; rather, it involves integrals over an interval of time and (for fields) an extended region of space. Moreover, in the usual formulation of classical
action principles, the initial and final states of the system are fixed, e.g.,
In particular, the fixing of the final state appears to give the action principle a teleological character
which has been controversial historically. However, some critics maintain this apparent teleology
occurs because of the way in which the question was asked. By specifying some but not all aspects of both the initial and final conditions (the positions but not the velocities) we are making some inferences about the initial conditions from the final conditions, and it is this "backward" inference that can be seen as a teleological causal influence.
The speculative fiction writer, Ted Chiang
, has a story, Story of Your Life
, that contains visual depictions of Fermat's Principle
along with a discussion of its teleological dimension. Keith Devlin
's The Math Instinct contains a chapter, "Elvis the Welsh Corgi Who Can Do Calculus" that discusses the calculus "embedded" in some animals as they solve the "least time" problem in actual situations.
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, the principle of least action – or, more accurately, the principle of stationary action – is a variational principle
Variational principle
A variational principle is a scientific principle used within the calculus of variations, which develops general methods for finding functions which minimize or maximize the value of quantities that depend upon those functions...
that, when applied to the action
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
of a mechanical
Mechanics
Mechanics is the branch of physics concerned with the behavior of physical bodies when subjected to forces or displacements, and the subsequent effects of the bodies on their environment....
system, can be used to obtain the equations of motion for that system. The principle led to the development of the Lagrangian
Lagrangian mechanics
Lagrangian mechanics is a re-formulation of classical mechanics that combines conservation of momentum with conservation of energy. It was introduced by the Italian-French mathematician Joseph-Louis Lagrange in 1788....
and Hamiltonian
Hamiltonian mechanics
Hamiltonian mechanics is a reformulation of classical mechanics that was introduced in 1833 by Irish mathematician William Rowan Hamilton.It arose from Lagrangian mechanics, a previous reformulation of classical mechanics introduced by Joseph Louis Lagrange in 1788, but can be formulated without...
formulations of classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
.
The principle remains central in modern physics
Modern physics
The term modern physics refers to the post-Newtonian conception of physics. The term implies that classical descriptions of phenomena are lacking, and that an accurate, "modern", description of reality requires theories to incorporate elements of quantum mechanics or Einsteinian relativity, or both...
and mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, being applied in the theory of relativity
Theory of relativity
The theory of relativity, or simply relativity, encompasses two theories of Albert Einstein: special relativity and general relativity. However, the word relativity is sometimes used in reference to Galilean invariance....
, quantum mechanics
Quantum mechanics
Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...
and quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
, and a focus of modern mathematical investigation in Morse theory
Morse theory
In differential topology, the techniques of Morse theory give a very direct way of analyzing the topology of a manifold by studying differentiable functions on that manifold. According to the basic insights of Marston Morse, a differentiable function on a manifold will, in a typical case, reflect...
. This article deals primarily with the historical development of the idea; a treatment of the mathematical description and derivation can be found in the article on the action
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
. The chief examples of the principle of stationary action are Maupertuis' principle
Maupertuis' principle
In classical mechanics, Maupertuis' principle is an integral equation that determines the path followed by a physical system without specifying the time parameterization of that path. It is a special case of the more generally stated principle of least action...
and Hamilton's principle
Hamilton's principle
In physics, Hamilton's principle is William Rowan Hamilton's formulation of the principle of stationary action...
.
The action principle is preceded by earlier ideas in surveying
Surveying
See Also: Public Land Survey SystemSurveying or land surveying is the technique, profession, and science of accurately determining the terrestrial or three-dimensional position of points and the distances and angles between them...
and optics
Optics
Optics is the branch of physics which involves the behavior and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behavior of visible, ultraviolet, and infrared light...
. The rope stretchers of ancient Egypt
Ancient Egypt
Ancient Egypt was an ancient civilization of Northeastern Africa, concentrated along the lower reaches of the Nile River in what is now the modern country of Egypt. Egyptian civilization coalesced around 3150 BC with the political unification of Upper and Lower Egypt under the first pharaoh...
stretched corded ropes between two points to measure the path which minimized the distance of separation, and Claudius Ptolemy, in his Geographia
Geographia (Ptolemy)
The Geography is Ptolemy's main work besides the Almagest...
(Bk 1, Ch 2), emphasized that one must correct for "deviations from a straight course"; in ancient Greece
Ancient Greece
Ancient Greece is a civilization belonging to a period of Greek history that lasted from the Archaic period of the 8th to 6th centuries BC to the end of antiquity. Immediately following this period was the beginning of the Early Middle Ages and the Byzantine era. Included in Ancient Greece is the...
Euclid
Euclid
Euclid , fl. 300 BC, also known as Euclid of Alexandria, was a Greek mathematician, often referred to as the "Father of Geometry". He was active in Alexandria during the reign of Ptolemy I...
states in his Catoptrica that, for the path of light reflecting from a mirror, the angle of incidence
Angle of incidence
Angle of incidence is a measure of deviation of something from "straight on", for example:* in the approach of a ray to a surface, or* the angle at which the wing or horizontal tail of an airplane is installed on the fuselage, measured relative to the axis of the fuselage.-Optics:In geometric...
equals the angle of reflection; and Hero of Alexandria
Hero of Alexandria
Hero of Alexandria was an ancient Greek mathematician and engineerEnc. Britannica 2007, "Heron of Alexandria" who was active in his native city of Alexandria, Roman Egypt...
later showed that this path was the shortest length and least time. But the credit for the formulation of the principle as it applies to the action is often given to Pierre-Louis Moreau de Maupertuis
Pierre Louis Maupertuis
Pierre-Louis Moreau de Maupertuis was a French mathematician, philosopher and man of letters. He became the Director of the Académie des Sciences, and the first President of the Berlin Academy of Science, at the invitation of Frederick the Great....
, who wrote about it in 1744 and 1746. However, scholarship indicates that this claim of priority is not so clear; Leonhard Euler
Leonhard Euler
Leonhard Euler was a pioneering Swiss mathematician and physicist. He made important discoveries in fields as diverse as infinitesimal calculus and graph theory. He also introduced much of the modern mathematical terminology and notation, particularly for mathematical analysis, such as the notion...
discussed the principle in 1744, and there is evidence that Gottfried Leibniz
Gottfried Leibniz
Gottfried Wilhelm Leibniz was a German philosopher and mathematician. He wrote in different languages, primarily in Latin , French and German ....
preceded both by 39 years.
Origins, statement, and the controversy
In the 17th century Pierre de FermatPierre de Fermat
Pierre de Fermat was a French lawyer at the Parlement of Toulouse, France, and an amateur mathematician who is given credit for early developments that led to infinitesimal calculus, including his adequality...
postulated that "light travels between two given points along the path of shortest time," which is known as the principle of least time or Fermat's principle
Fermat's principle
In optics, Fermat's principle or the principle of least time is the principle that the path taken between two points by a ray of light is the path that can be traversed in the least time. This principle is sometimes taken as the definition of a ray of light...
.
Maupertuis' formulation
Credit for the formulation of the principle of least action is commonly given to Pierre Louis MaupertuisPierre Louis Maupertuis
Pierre-Louis Moreau de Maupertuis was a French mathematician, philosopher and man of letters. He became the Director of the Académie des Sciences, and the first President of the Berlin Academy of Science, at the invitation of Frederick the Great....
, who wrote about it in 1744 and 1746. Maupertuis felt that "Nature is thrifty in all its actions", and applied the principle broadly:
This notion of Maupertuis, although somewhat deterministic today, does capture much of the essence of mechanics.
In application to physics, Maupertuis suggested that the quantity to be minimized was the product of the duration (time) of movement within a system by the "vis viva
Vis viva
In the history of science, vis viva is an obsolete scientific theory that served as an elementary and limited early formulation of the principle of conservation of energy...
", twice what we now call the kinetic energy
Kinetic energy
The kinetic energy of an object is the energy which it possesses due to its motion.It is defined as the work needed to accelerate a body of a given mass from rest to its stated velocity. Having gained this energy during its acceleration, the body maintains this kinetic energy unless its speed changes...
T of the system.
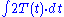
Euler's formulation
Leonhard EulerLeonhard Euler
Leonhard Euler was a pioneering Swiss mathematician and physicist. He made important discoveries in fields as diverse as infinitesimal calculus and graph theory. He also introduced much of the modern mathematical terminology and notation, particularly for mathematical analysis, such as the notion...
gave a formulation of the action principle in 1744, in very recognizable terms, in the Additamentum 2 to his Methodus Inveniendi Lineas Curvas Maximi Minive Proprietate Gaudentes. He begins the second paragraph :
As Euler states,
is the integral of the momentum over distance traveled, which, in modern notation, equals the reduced action
-
.
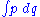Thus, Euler made an equivalent and (apparently) independent statement of the variational principle in the same year as Maupertuis, albeit slightly later. Curiously, Euler did not claim any priority, as the following episode shows.
Disputed priority
Maupertuis' priority was disputed in 1751 by the mathematician Samuel König, who claimed that it had been invented by Gottfried LeibnizGottfried Leibniz
Gottfried Wilhelm Leibniz was a German philosopher and mathematician. He wrote in different languages, primarily in Latin , French and German ....
in 1707. Although similar to many of Leibniz's arguments, the principle itself has not been documented in Leibniz's works. König himself showed a copy of a 1707 letter from Leibniz to Jacob Hermann with the principle, but the original letter has been lost. In contentious proceedings, König was accused of forgery, and even the King of Prussia entered the debate, defending Maupertuis, while Voltaire
Voltaire
François-Marie Arouet , better known by the pen name Voltaire , was a French Enlightenment writer, historian and philosopher famous for his wit and for his advocacy of civil liberties, including freedom of religion, free trade and separation of church and state...
defended König.
Euler, rather than claiming priority, was a staunch defender of Maupertuis, and Euler himself prosecuted König for forgery before the Berlin Academy on 13 April 1752. The claims of forgery were re-examined 150 years later, and archival work by C.I. Gerhardt in 1898 and W. Kabitz in 1913 uncovered other copies of the letter, and three others cited by König, in the Bernoulli
Bernoulli
The Bernoullis were a family of traders and scholars from Basel, Switzerland. The founder of the family, Leon Bernoulli, immigrated to Basel from Antwerp in Flanders in the 16th century, fleeing Spanish oppression....
archives.
Further development
Euler continued to write on the topic; in his Reflexions sur quelques loix generales de la nature (1748), he called the quantity "effort". His expression corresponds to what we would now call potential energyPotential energy
In physics, potential energy is the energy stored in a body or in a system due to its position in a force field or due to its configuration. The SI unit of measure for energy and work is the Joule...
, so that his statement of least action in statics is equivalent to the principle that a system of bodies at rest will adopt a configuration that minimizes total potential energy.
Lagrange and Hamilton
Much of the calculus of variations was stated by Joseph Louis LagrangeJoseph Louis Lagrange
Joseph-Louis Lagrange , born Giuseppe Lodovico Lagrangia, was a mathematician and astronomer, who was born in Turin, Piedmont, lived part of his life in Prussia and part in France, making significant contributions to all fields of analysis, to number theory, and to classical and celestial mechanics...
in 1760 and he proceeded to apply this to problems in dynamics. In Méchanique Analytique (1788) Lagrange derived the general equations of motion of a mechanical body. William Rowan Hamilton
William Rowan Hamilton
Sir William Rowan Hamilton was an Irish physicist, astronomer, and mathematician, who made important contributions to classical mechanics, optics, and algebra. His studies of mechanical and optical systems led him to discover new mathematical concepts and techniques...
in 1834 and 1835 applied the variational principle to the classical Lagrangian function
Lagrangian
The Lagrangian, L, of a dynamical system is a function that summarizes the dynamics of the system. It is named after Joseph Louis Lagrange. The concept of a Lagrangian was originally introduced in a reformulation of classical mechanics by Irish mathematician William Rowan Hamilton known as...
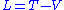to obtain the Euler-Lagrange equations in their present form.Jacobi and Morse
In 1842, Carl Gustav Jacobi tackled the problem of whether the variational principle always found minima as opposed to other stationary points (maxima or stationary saddle points); most of his work focused on geodesics on two-dimensional surfaces. The first clear general statements were given by Marston MorseMarston Morse
Harold Calvin Marston Morse was an American mathematician best known for his work on the calculus of variations in the large, a subject where he introduced the technique of differential topology now known as Morse theory...
in the 1920s and 1930s, leading to what is now known as Morse theory
Morse theory
In differential topology, the techniques of Morse theory give a very direct way of analyzing the topology of a manifold by studying differentiable functions on that manifold. According to the basic insights of Marston Morse, a differentiable function on a manifold will, in a typical case, reflect...
. For example, Morse showed that the number of conjugate points
Conjugate points
In differential geometry, conjugate points are, roughly, points that can almost be joined by a 1-parameter family of geodesics. For example, on a sphere, the north-pole and south-pole are connected by any meridian.-Definition:...
in a trajectory equalled the number of negative eigenvalues in the second variation of the Lagrangian.
Gauss and Hertz
Other extremal principles of classical mechanicsClassical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
have been formulated, such as Gauss' principle of least constraint and its corollary, Hertz's principle of least curvature.
Apparent teleology
The mathematical equivalence of the differentialDifferential equation
A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...
equations of motion and their integral
Integral equation
In mathematics, an integral equation is an equation in which an unknown function appears under an integral sign. There is a close connection between differential and integral equations, and some problems may be formulated either way...
counterpart has important philosophical implications. The differential equations are statements about quantities localized to a single point in space or single moment of time. For example, Newton's second law
Newton's laws of motion
Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...
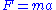states that the instantaneous force 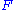applied to a mass produces an acceleration at the same instant. By contrast, the action principle is not localized to a point; rather, it involves integrals over an interval of time and (for fields) an extended region of space. Moreover, in the usual formulation of classicalClassical physics
What "classical physics" refers to depends on the context. When discussing special relativity, it refers to the Newtonian physics which preceded relativity, i.e. the branches of physics based on principles developed before the rise of relativity and quantum mechanics...
action principles, the initial and final states of the system are fixed, e.g.,
- Given that the particle begins at position
at time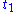 
and ends at position 
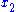at time 
, the physical trajectory that connects these two endpoints is an extremum of the action integral.
In particular, the fixing of the final state appears to give the action principle a teleological character
Teleology
A teleology is any philosophical account which holds that final causes exist in nature, meaning that design and purpose analogous to that found in human actions are inherent also in the rest of nature. The word comes from the Greek τέλος, telos; root: τελε-, "end, purpose...
which has been controversial historically. However, some critics maintain this apparent teleology
Teleology
A teleology is any philosophical account which holds that final causes exist in nature, meaning that design and purpose analogous to that found in human actions are inherent also in the rest of nature. The word comes from the Greek τέλος, telos; root: τελε-, "end, purpose...
occurs because of the way in which the question was asked. By specifying some but not all aspects of both the initial and final conditions (the positions but not the velocities) we are making some inferences about the initial conditions from the final conditions, and it is this "backward" inference that can be seen as a teleological causal influence.
The speculative fiction writer, Ted Chiang
Ted Chiang
Ted Chiang is an American speculative fiction writer. His Chinese name is Chiang Feng-nan.He was born in Port Jefferson, New York and graduated from Brown University with a Computer Science degree. He currently works as a technical writer in the software industry and resides in Bellevue, near...
, has a story, Story of Your Life
Story of Your Life
Story of Your Life is a science fiction short story by Ted Chiang. It was the winner of the 2000 Nebula Award for Best Novella as well as the 1999 Sturgeon award. The major themes explored by this tale are determinism, language, and an interesting take on the Sapir–Whorf hypothesis.-Plot summary:Dr...
, that contains visual depictions of Fermat's Principle
Fermat's principle
In optics, Fermat's principle or the principle of least time is the principle that the path taken between two points by a ray of light is the path that can be traversed in the least time. This principle is sometimes taken as the definition of a ray of light...
along with a discussion of its teleological dimension. Keith Devlin
Keith Devlin
Keith J. Devlin is a British mathematician and popular science writer. He has lived in the USA since 1987 and has dual American-British citizenship.- Biography :...
's The Math Instinct contains a chapter, "Elvis the Welsh Corgi Who Can Do Calculus" that discusses the calculus "embedded" in some animals as they solve the "least time" problem in actual situations.
See also
- Action (physics)Action (physics)In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
- Calculus of variationsCalculus of variationsCalculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
- Hamiltonian mechanicsHamiltonian mechanicsHamiltonian mechanics is a reformulation of classical mechanics that was introduced in 1833 by Irish mathematician William Rowan Hamilton.It arose from Lagrangian mechanics, a previous reformulation of classical mechanics introduced by Joseph Louis Lagrange in 1788, but can be formulated without...
- Hamilton's principleHamilton's principleIn physics, Hamilton's principle is William Rowan Hamilton's formulation of the principle of stationary action...
- Lagrangian mechanicsLagrangian mechanicsLagrangian mechanics is a re-formulation of classical mechanics that combines conservation of momentum with conservation of energy. It was introduced by the Italian-French mathematician Joseph-Louis Lagrange in 1788....
- Maupertuis principle
- Path of least resistancePath of least resistanceThe path of least resistance describes the physical or metaphorical pathway that provides the least resistance to forward motion by a given object or entity, among a set of alternative paths. The concept is often used to describe why an object or entity takes a given path.In physics, the path of...