Petrie polygon
Encyclopedia
In geometry
, a Petrie polygon for a regular polytope
of n dimensions is a skew polygon
such that every (n-1) consecutive sides
(but no n) belong to one of the facets. The Petrie polygon of a regular polygon
is the regular polygon itself; that of a regular polyhedron
is a skew polygon
such that every two consecutive sides
(but no three) belong to one of the face
s.
For every regular polytope there exists an orthogonal projection onto a plane such that one Petrie polygon becomes a regular polygon
with the remainder of the projection interior to it. The plane in question is the Coxeter plane of the symmetry group of the polygon, and the number of sides, h, is Coxeter number
of the Coxeter group
. These polygons and projected graphs are useful in visualizing symmetric structure of the higher dimensional regular polytopes.
He first realized the importance of the regular skew polygons which appear on the surface of regular polyhedra and higher polytopes. He was a lifelong friend of Coxeter, who named these polygons after him.
The idea of Petrie polygons was later extended to semiregular polytopes.
In 1972, a few months after his retirement, Petrie was killed by a car while attempting to cross a motorway near his home in Surrey
.
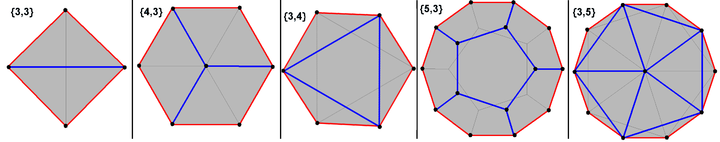
The regular duals
, {p,q} and {q,p}, are contained within the same projected Petrie polygon.
, Hypercube
, Orthoplex), and the Exceptional Lie group En which generate semiregular and uniform polytopes for dimensions 4 to 8.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, a Petrie polygon for a regular polytope
Regular polytope
In mathematics, a regular polytope is a polytope whose symmetry is transitive on its flags, thus giving it the highest degree of symmetry. All its elements or j-faces — cells, faces and so on — are also transitive on the symmetries of the polytope, and are regular polytopes of...
of n dimensions is a skew polygon
Skew polygon
In geometry, a skew polygon is a polygon whose vertices do not lie in a plane. Skew polygons must have at least 4 vertices.A regular skew polygon is a skew polygon with equal edge lengths and which is vertex-transitive....
such that every (n-1) consecutive sides
Edge (geometry)
In geometry, an edge is a one-dimensional line segment joining two adjacent zero-dimensional vertices in a polygon. Thus applied, an edge is a connector for a one-dimensional line segment and two zero-dimensional objects....
(but no n) belong to one of the facets. The Petrie polygon of a regular polygon
Regular polygon
A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...
is the regular polygon itself; that of a regular polyhedron
Regular polyhedron
A regular polyhedron is a polyhedron whose faces are congruent regular polygons which are assembled in the same way around each vertex. A regular polyhedron is highly symmetrical, being all of edge-transitive, vertex-transitive and face-transitive - i.e. it is transitive on its flags...
is a skew polygon
Skew polygon
In geometry, a skew polygon is a polygon whose vertices do not lie in a plane. Skew polygons must have at least 4 vertices.A regular skew polygon is a skew polygon with equal edge lengths and which is vertex-transitive....
such that every two consecutive sides
Edge (geometry)
In geometry, an edge is a one-dimensional line segment joining two adjacent zero-dimensional vertices in a polygon. Thus applied, an edge is a connector for a one-dimensional line segment and two zero-dimensional objects....
(but no three) belong to one of the face
Face (geometry)
In geometry, a face of a polyhedron is any of the polygons that make up its boundaries. For example, any of the squares that bound a cube is a face of the cube...
s.
For every regular polytope there exists an orthogonal projection onto a plane such that one Petrie polygon becomes a regular polygon
Regular polygon
A regular polygon is a polygon that is equiangular and equilateral . Regular polygons may be convex or star.-General properties:...
with the remainder of the projection interior to it. The plane in question is the Coxeter plane of the symmetry group of the polygon, and the number of sides, h, is Coxeter number
Coxeter number
In mathematics, the Coxeter number h is the order of a Coxeter element of an irreducible Coxeter group, hence also of a root system or its Weyl group. It is named after H.S.M. Coxeter.-Definitions:...
of the Coxeter group
Coxeter group
In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...
. These polygons and projected graphs are useful in visualizing symmetric structure of the higher dimensional regular polytopes.
History
John Flinders Petrie (1907-1972) was the only son of Egyptologist Sir W. M. Flinders Petrie. He was born in 1907 and as a schoolboy showed remarkable promise of mathematical ability. In periods of intense concentration he could answer questions about complicated four-dimensional objects by visualizing them.He first realized the importance of the regular skew polygons which appear on the surface of regular polyhedra and higher polytopes. He was a lifelong friend of Coxeter, who named these polygons after him.
The idea of Petrie polygons was later extended to semiregular polytopes.
In 1972, a few months after his retirement, Petrie was killed by a car while attempting to cross a motorway near his home in Surrey
Surrey
Surrey is a county in the South East of England and is one of the Home Counties. The county borders Greater London, Kent, East Sussex, West Sussex, Hampshire and Berkshire. The historic county town is Guildford. Surrey County Council sits at Kingston upon Thames, although this has been part of...
.
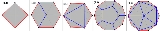
The Petrie polygons of the regular polyhedra
The Petrie polygon of the regular polyhedron {p, q} has h sides, where- cos2(π/h) = cos2(π/p) + cos2(π/q).
The regular duals
Dual polyhedron
In geometry, polyhedra are associated into pairs called duals, where the vertices of one correspond to the faces of the other. The dual of the dual is the original polyhedron. The dual of a polyhedron with equivalent vertices is one with equivalent faces, and of one with equivalent edges is another...
, {p,q} and {q,p}, are contained within the same projected Petrie polygon.
 |
||||
| tetrahedron Tetrahedron In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids... |
cube Cube In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. The cube can also be called a regular hexahedron and is one of the five Platonic solids. It is a special kind of square prism, of rectangular parallelepiped and... |
octahedron Octahedron In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.... |
dodecahedron | icosahedron Icosahedron In geometry, an icosahedron is a regular polyhedron with 20 identical equilateral triangular faces, 30 edges and 12 vertices. It is one of the five Platonic solids.... |
| edge-centered | vertex-centered | face-centered | face-centered | vertex-centered |
| 4 sides | 6 sides | 6 sides | 10 sides | 10 sides |
| V:(4,0) | V:(6,2) | V:(6,0) | V:(10,10,0) | V:(10,2) |
| The Petrie polygons are the exterior of these orthogonal projections. Blue show "front" edges, while black lines show back edges. The concentric rings of vertices are counted starting from the outside working inwards with a notation: V:(a, b, ...), ending in zero if there are no central vertices. |
||||
The Petrie polygon of regular polychora (4-polytopes)
The Petrie polygon for the regular polychora {p, q ,r} can also be determined.{3,3,3} 5-cell 5 sides V:(5,0) |
{3,3,4} 16-cell 16-cell In four dimensional geometry, a 16-cell or hexadecachoron is a regular convex 4-polytope. It is one of the six regular convex 4-polytopes first described by the Swiss mathematician Ludwig Schläfli in the mid-19th century.... 8 sides V:(8,0) |
{4,3,3} tesseract Tesseract In geometry, the tesseract, also called an 8-cell or regular octachoron or cubic prism, is the four-dimensional analog of the cube. The tesseract is to the cube as the cube is to the square. Just as the surface of the cube consists of 6 square faces, the hypersurface of the tesseract consists of 8... 8 sides V:(8,8,0) |
{3,4,3} 24-cell 12 sides V:(12,6,6,0) |
{5,3,3} 120-cell 30 sides V:((30,60)3,603,30,60,0) |
{3,3,5} 600-cell 30 sides V:(30,30,30,30,0) |
The Petrie polygon projections of regular and uniform polytopes
The Petrie polygon projections are most useful for visualization of polytopes of dimension four and higher. This table represents Petrie polygon projections of 3 regular families (SimplexSimplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
, Hypercube
Hypercube
In geometry, a hypercube is an n-dimensional analogue of a square and a cube . It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions, perpendicular to each other and of the same length.An...
, Orthoplex), and the Exceptional Lie group En which generate semiregular and uniform polytopes for dimensions 4 to 8.

