
Jet bundle
Encyclopedia
In differential geometry, the jet bundle is a certain construction which makes a new smooth fiber bundle
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
out of a given smooth fiber bundle. It makes it possible to write differential equation
Differential equation
A differential equation is a mathematical equation for an unknown function of one or several variables that relates the values of the function itself and its derivatives of various orders...
s on sections of a fiber bundle in an invariant form. Jets may also be seen as the coordinate free versions of taylor expansions.
Historically, jet bundles are attributed to Ehresmann, and were an advance on the method (prolongation) of Élie Cartan
Élie Cartan
Élie Joseph Cartan was an influential French mathematician, who did fundamental work in the theory of Lie groups and their geometric applications...
, of dealing geometrically with higher derivatives
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
, by imposing differential form
Differential form
In the mathematical fields of differential geometry and tensor calculus, differential forms are an approach to multivariable calculus that is independent of coordinates. Differential forms provide a better definition for integrands in calculus...
conditions on newly-introduced formal variables. Jet bundles are sometimes called sprays, although sprays
Spray (mathematics)
In differential geometry, a spray is a vector field H on the tangent bundle TM that encodes a quasilinear second order system of ordinary differential equations on the base manifold M. Usually a spray is required to be homogeneous in the sense that its integral curves t→ΦHt∈TM obey the rule...
usually refer more specifically to the associated vector field induced on the corresponding bundle (e.g., the geodesic spray on Finsler manifold
Finsler manifold
In mathematics, particularly differential geometry, a Finsler manifold is a differentiable manifold together with the structure of an intrinsic quasimetric space in which the length of any rectifiable curve is given by the length functional...
s.)
More recently, jet bundles have appeared as a concise way to describe phenomena associated with the derivatives of maps, particularly those associated with the calculus of variations
Calculus of variations
Calculus of variations is a field of mathematics that deals with extremizing functionals, as opposed to ordinary calculus which deals with functions. A functional is usually a mapping from a set of functions to the real numbers. Functionals are often formed as definite integrals involving unknown...
. Consequently, the jet bundle is now recognized as the correct domain for a geometrical covariant field theory
Covariant classical field theory
In recent years, there has been renewed interest in covariant classical field theory. Here, classical fields are represented by sections of fiber bundles and their dynamics is phrased in the context of a finite-dimensional space of fields. Nowadays, it is well known that jet bundles and the...
and much work is done in general relativistic
General relativity
General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1916. It is the current description of gravitation in modern physics...
formulations of fields using this approach.
Jets
Let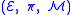 be a fiber bundle
be a fiber bundleFiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
in a category of manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
s and let
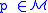 , with
, with 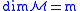 .
.Let
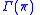 denote the set of all local sections whose domain contains
denote the set of all local sections whose domain contains  . Let
. Let 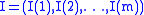 be a multi-index (an ordered
be a multi-index (an ordered  -tuple of integers), then
-tuple of integers), then
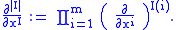
Define the local sections
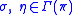 to have the same
to have the same  -jet at
-jet at  if
if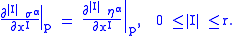
The relation that two maps have the same
 -jet is an equivalence relation
-jet is an equivalence relationEquivalence relation
In mathematics, an equivalence relation is a relation that, loosely speaking, partitions a set so that every element of the set is a member of one and only one cell of the partition. Two elements of the set are considered equivalent if and only if they are elements of the same cell...
. An r-jet is an equivalence class under this relation, and the r-jet with representative
 is denoted
is denoted 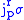 . The integer
. The integer  is also called the order of the jet.
is also called the order of the jet. is the source of
is the source of  .
.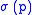 is the target of
is the target of 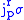 .
.Jet manifolds
The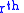 jet manifold of
jet manifold of  is the set
is the set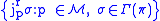
and is denoted
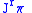 . We may define projections
. We may define projections 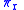 and
and 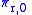 called the source and target projections respectively, by
called the source and target projections respectively, by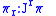 |
 |
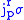 |
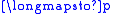 |
 |
 |
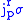 |
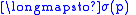 |
If
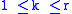 , then the
, then the 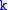 -jet projection is the function
-jet projection is the function 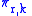 defined by
defined by |
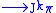 |
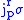 |
 |
From this definition, it is clear that
 and that if
and that if  , then
, then 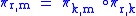 . It is conventional to regard
. It is conventional to regard 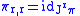 , the identity map
, the identity mapIdentity function
In mathematics, an identity function, also called identity map or identity transformation, is a function that always returns the same value that was used as its argument...
on
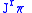 and to identify
and to identify 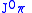 with
with  .
.The functions
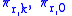 and
and 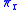 are smooth
are smoothSmooth
Smooth means having a texture that lacks friction. Not rough.Smooth may also refer to:-In mathematics:* Smooth function, a function that is infinitely differentiable; used in calculus and topology...
surjective submersion
Submersion (mathematics)
In mathematics, a submersion is a differentiable map between differentiable manifolds whose differential is everywhere surjective. This is a basic concept in differential topology...
s.
A coordinate system
Coordinate system
In geometry, a coordinate system is a system which uses one or more numbers, or coordinates, to uniquely determine the position of a point or other geometric element. The order of the coordinates is significant and they are sometimes identified by their position in an ordered tuple and sometimes by...
on
 will generate a coordinate system on
will generate a coordinate system on 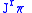 . Let
. Let 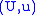 be an adapted coordinate chart on
be an adapted coordinate chart on  , where
, where  . The induced coordinate chart
. The induced coordinate chart 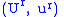 on
on 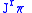 is defined by
is defined by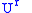 |
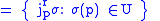 |
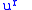 |
 |
where
 |
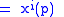 |
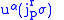 |
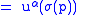 |
and the
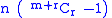 functions
functions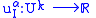
are specified by
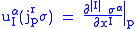
and are known as the derivative coordinates.
Given an atlas of adapted charts
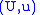 on
on  , the corresponding collection of charts
, the corresponding collection of charts 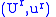 is a finite-dimensional
is a finite-dimensional  atlas on
atlas on 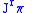 .
.Jet bundles
Since the atlas on each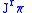 defines a manifold, the triples
defines a manifold, the triples 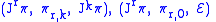 and
and 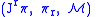 all define fibered manifolds.
all define fibered manifolds.In particular, if
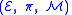 is a fiber bundle, the triple
is a fiber bundle, the triple 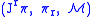 defines the
defines the 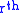 jet bundle of
jet bundle of  .
.If
 is an open submanifold, then
is an open submanifold, then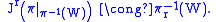
If
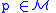 , then the fiber
, then the fiber 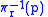 is denoted
is denoted 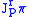 .
.Let
 be a local section of
be a local section of  with domain
with domain  . The
. The 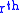 jet prolongation of
jet prolongation of  is the map
is the map  defined by
defined by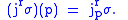
Note that
 , so
, so  really is a section. In local coordinates,
really is a section. In local coordinates, 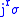 is given by
is given by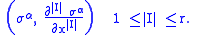
We identify
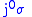 with
with  .
.Example
If is the trivial bundle
is the trivial bundle  , then there is a canonical diffeomorphism
, then there is a canonical diffeomorphismDiffeomorphism
In mathematics, a diffeomorphism is an isomorphism in the category of smooth manifolds. It is an invertible function that maps one differentiable manifold to another, such that both the function and its inverse are smooth.- Definition :...
between the first jet bundle
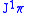 and
and  .
.To construct this diffeomorphism, for each
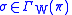 write
write 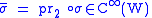 .
.Then, whenever

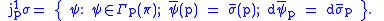
Consequently, the mapping
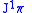 |
 |
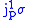 |
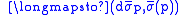 |
is well-defined and is clearly injective. Writing it out in coordinates shows that it is a diffeomorphism, because if
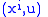 are coordinates on
are coordinates on  , where
, where 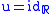 is the identity coordinate, then the derivative coordinates
is the identity coordinate, then the derivative coordinates 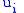 on
on 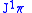 correspond to the coordinates
correspond to the coordinates 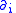 on
on 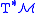 .
.Likewise, if
 is the trivial bundle
is the trivial bundle 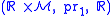 , then there exists a canonical diffeomorphism between
, then there exists a canonical diffeomorphism between 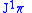 and
and 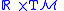
Contact forms
A differential 1-form on the space
on the space 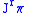 is called a contact form (i.e.
is called a contact form (i.e. 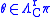 ) if it is pulled back to the zero form on
) if it is pulled back to the zero form on  by all prolongations.
by all prolongations.In other words, if
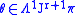 , then
, then 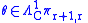 if and only if
if and only ifIf and only if
In logic and related fields such as mathematics and philosophy, if and only if is a biconditional logical connective between statements....
, for every open submanifold
 and every
and every 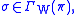

The distribution
Distribution (differential geometry)
In differential geometry, a discipline within mathematics, a distribution is a subset of the tangent bundle of a manifold satisfying certain properties...
on
 generated by the contact forms is called the Cartan distribution. It is the main geometrical structure on jet spaces and plays an important role in the geometric theory of partial differential equation
generated by the contact forms is called the Cartan distribution. It is the main geometrical structure on jet spaces and plays an important role in the geometric theory of partial differential equationPartial differential equation
In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...
s. The Cartan distributions are not involutive
Distribution (differential geometry)
In differential geometry, a discipline within mathematics, a distribution is a subset of the tangent bundle of a manifold satisfying certain properties...
and are of growing dimension when passing to higher order jet spaces. Surprisingly though, when passing to the space of infinite order jets
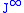 this distribution is involutive and finite dimensional. Its dimension coinciding with the dimension of the base manifold
this distribution is involutive and finite dimensional. Its dimension coinciding with the dimension of the base manifold  .
.Example
Let us consider the case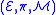 , where
, where  and
and 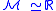 .
.Then,
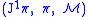 defines the first jet bundle, and may be coordinated by
defines the first jet bundle, and may be coordinated by  , where
, where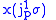 |
 |
 |
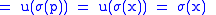 |
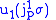 |
 |
for all
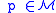 and
and 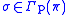 . A general 1-form on
. A general 1-form on 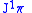 takes the form
takes the form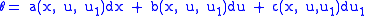
A section
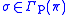 has first prolongation
has first prolongation 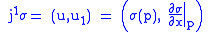 .
.Hence,
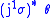 can be calculated as
can be calculated as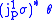 |
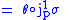 |
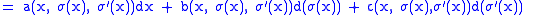 |
|
 |
|
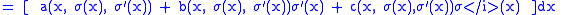 |
|
This will vanish for all sections
 if and only if
if and only if 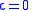 and
and 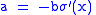 . Hence,
. Hence, 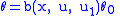 must necessarily be a multiple of the basic contact form
must necessarily be a multiple of the basic contact form 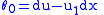 .
.Proceeding to the second jet space
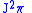 with additional coordinate
with additional coordinate 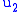 , such that
, such that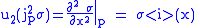
a general 1-form has the construction

This is a contact form if and only if
If and only if
In logic and related fields such as mathematics and philosophy, if and only if is a biconditional logical connective between statements....
 |
 |
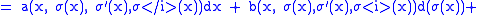 |
|
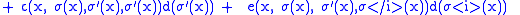 |
|
 |
|
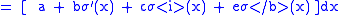 |
|
 |
|
which implies that
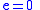 and
and 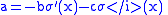 . Therefore,
. Therefore,  is a contact form if and only if
is a contact form if and only if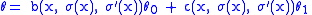
where
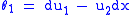 is the next basic contact form
is the next basic contact form(Note that here we are identifying the form
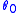 with its pull-back
with its pull-back 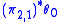 to
to  ).
).In general, providing
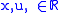 , a contact form on
, a contact form on 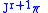 can be written as a linear combination
can be written as a linear combinationLinear combination
In mathematics, a linear combination is an expression constructed from a set of terms by multiplying each term by a constant and adding the results...
of the basic contact forms

where
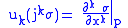 .
.Similar arguments lead to a complete characterization of all contact forms.
In local coordinates, every contact one-form on
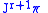 can be written as a linear combination
can be written as a linear combination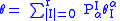
with smooth coefficients
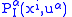 of the basic contact forms
of the basic contact forms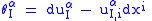
 is known as the order of the contact form
is known as the order of the contact form 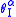 . Note that contact forms on
. Note that contact forms on 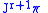 have orders at most
have orders at most  .
.Contact forms provide a characterization of those local sections of
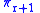 which are prolongations of sections of
which are prolongations of sections of  .
.Let
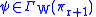 , then
, then 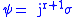 where
where 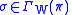 if and only if
if and only if 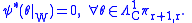
Vector fields
A general vector fieldVector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
on the total space
 , coordinated by
, coordinated by 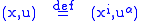 , is
, is
A vector field is called horizontal, meaning all the vertical coefficients vanish, if
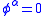 .
.A vector field is called vertical, meaning all the horizontal coefficients vanish, if
 .
.For fixed
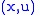 , we identify
, we identify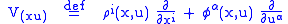
having coordinates
 , with an element in the fiber
, with an element in the fiber 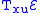 of
of  over
over 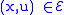 , called a tangent vector
, called a tangent vectorTangent vector
A tangent vector is a vector that is tangent to a curve or surface at a given point.Tangent vectors are described in the differential geometry of curves in the context of curves in Rn. More generally, tangent vectors are elements of a tangent space of a differentiable manifold....
in
 . A section
. A section |
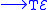 |
 |
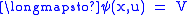 |
is called a vector field on
 with
with 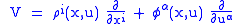 and
and  .
.The jet bundle
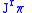 is coordinated by
is coordinated by 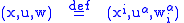 . For fixed
. For fixed 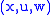 , identify
, identify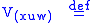 |
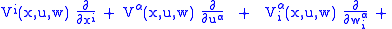 |
 |
|
having coordinates
 , with an element in the fiber
, with an element in the fiber  of
of 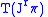 over
over  , called a tangent vector in
, called a tangent vector in 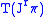 .
.Here,
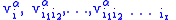 are real-valued functions on
are real-valued functions on  . A section
. A section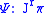 |
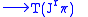 |
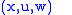 |
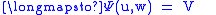 |
is a vector field on
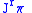 , and we say
, and we say 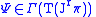 .
.Partial differential equations
Let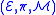 be a fiber bundle. An
be a fiber bundle. An 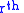 order partial differential equation
order partial differential equationPartial differential equation
In mathematics, partial differential equations are a type of differential equation, i.e., a relation involving an unknown function of several independent variables and their partial derivatives with respect to those variables...
on
 is a closed
is a closedClosed
Closed may refer to:Math* Closure * Closed manifold* Closed orbits* Closed set* Closed differential form* Closed map, a function that is closed.Other* Cloister, a closed walkway* Closed-circuit television...
embedded
Embedding
In mathematics, an embedding is one instance of some mathematical structure contained within another instance, such as a group that is a subgroup....
submanifold
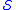 of the jet manifold
of the jet manifold 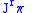 .
.A solution is a local section
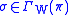 satisfying
satisfying  .
.Let us consider an example of a first order partial differential equation.
Example
Let be the trivial bundle
be the trivial bundle 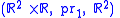 with global coordinates
with global coordinates 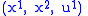 .
.Then the map
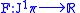 defined by
defined by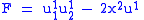
gives rise to the differential equation
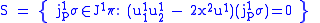
which can be written
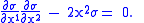
The particular section
 defined by
defined by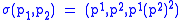
has first prolongation given by
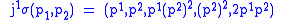
and is a solution of this differential equation, because
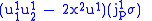 |
 |
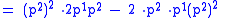 |
|
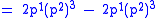 |
|
 |
|
and so
 for every
for every 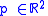 .
.Jet Prolongation
A local diffeomorphism defines a contact transformation of order
defines a contact transformation of order  if it preserves the contact ideal, meaning that if
if it preserves the contact ideal, meaning that if  is any contact form on
is any contact form on 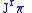 , then
, then 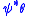 is also a contact form.
is also a contact form.The flow generated by a vector field
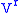 on the jet space
on the jet space 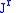 forms a one-parameter group of contact transformations if and only if the Lie derivative
forms a one-parameter group of contact transformations if and only if the Lie derivativeLie derivative
In mathematics, the Lie derivative , named after Sophus Lie by Władysław Ślebodziński, evaluates the change of a vector field or more generally a tensor field, along the flow of another vector field...
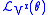 of any contact form
of any contact form  preserves the contact ideal.
preserves the contact ideal.Let us begin with the first order case. Consider a general vector field
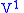 on
on 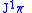 , given by
, given by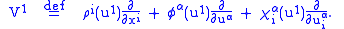
We now apply
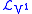 to the basic contact forms
to the basic contact forms 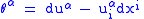 , and obtain
, and obtain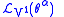 |
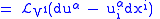 |
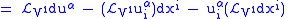 |
|
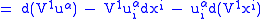 |
|
 |
|
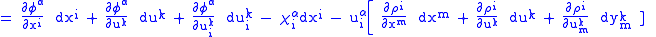 |
|
where we have expanded the exterior derivative
Exterior derivative
In differential geometry, the exterior derivative extends the concept of the differential of a function, which is a 1-form, to differential forms of higher degree. Its current form was invented by Élie Cartan....
of the functions in terms of their coordinates.
Next, we note that

and so we may write
 |
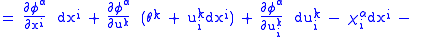 |
 |
|
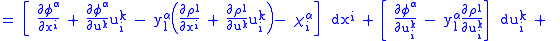 |
|
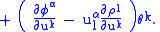 |
|
Therefore,
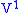 determines a contact transformation if and only if the coefficients of
determines a contact transformation if and only if the coefficients of  and
and 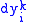 in the formula vanish.
in the formula vanish.The latter requirements imply the contact conditions
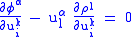
The former requirements provide explicit formulae for the coefficients of the first derivative terms in
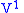 :
: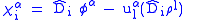 where
where 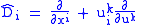
denotes the zeroth order truncation of the total derivative
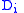 .
.Thus, the contact conditions uniquely prescribe the prolongation of any point or contact vector field. That is, if
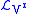 satisfies these equations,
satisfies these equations, 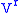 is called the
is called the 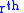 prolongation of
prolongation of 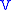 to a vector field on
to a vector field on 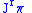 .
.These results are best understood when applied to a particular example. Hence, let us examine the following.
Example
Let us consider the case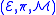 , where
, where  and
and 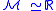 .
.Then,
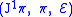 defines the first jet bundle, and may be coordinated by
defines the first jet bundle, and may be coordinated by 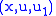 , where
, where |
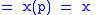 |
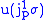 |
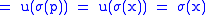 |
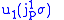 |
|
for all
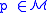 and
and 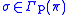 . A contact form on
. A contact form on 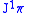 has the form
has the form
Let us consider a vector
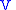 on
on  , having the form
, having the form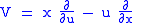
Then, the first prolongation of this vector field to
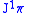 is
is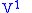 |
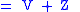 |
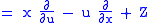 |
|
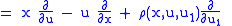 |
|
If we now take the Lie derivative of the contact form with respect to this prolonged vector field,
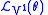 , we obtain
, we obtain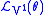 |
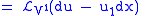 |
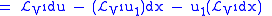 |
|
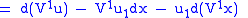 |
|
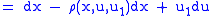 |
|
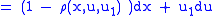 |
|
But, we may identify
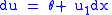 . Thus, we get
. Thus, we get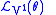 |
 |
 |
|
Hence, for
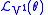 to preserve the contact ideal, we require
to preserve the contact ideal, we require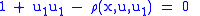 |
|
 |
 |
And so the first prolongation of
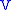 to a vector field on
to a vector field on 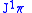 is
is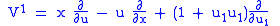
Let us also calculate the second prolongation of
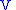 to a vector field on
to a vector field on 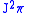 .
.We have
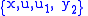 as coordinates on
as coordinates on  . Hence, the prolonged vector has the form
. Hence, the prolonged vector has the form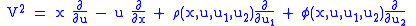
The contacts forms are
 |
 |
 |
 |
To preserve the contact ideal, we require
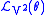 |
 |
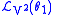 |
 |
Now,
 has no
has no 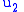 dependency. Hence, from this equation we will pick up the formula for
dependency. Hence, from this equation we will pick up the formula for  , which will necessarily be the same result as we found for
, which will necessarily be the same result as we found for 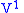 . Therefore, the problem is analogous to prolonging the vector field
. Therefore, the problem is analogous to prolonging the vector field 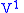 to
to 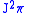 .
.That is to say, we may generate the
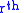 -prolongation of a vector field by recursively applying the Lie derivative of the contact forms with respect to the prolonged vector fields,
-prolongation of a vector field by recursively applying the Lie derivative of the contact forms with respect to the prolonged vector fields,  times.
times.So, we have

and so
 |
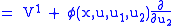 |
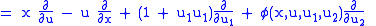 |
|
Therefore, the Lie derivative of the second contact form with respect to
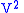 is
is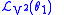 |
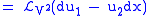 |
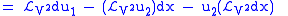 |
|
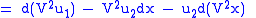 |
|
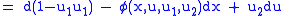 |
|
 |
|
Again, let us identify
 and
and  . Then we have
. Then we have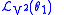 |
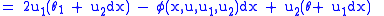 |
 |
|
Hence, for
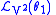 to preserve the contact ideal, we require
to preserve the contact ideal, we require |
|
 |
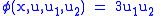 |
And so the second prolongation of
 to a vector field on
to a vector field on  is
is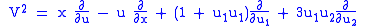
Note that the first prolongation of
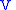 can be recovered by omitting the second derivative terms in
can be recovered by omitting the second derivative terms in  , or by projecting back to
, or by projecting back to  .
.Infinite Jet Spaces
The inverse limitInverse limit
In mathematics, the inverse limit is a construction which allows one to "glue together" several related objects, the precise manner of the gluing process being specified by morphisms between the objects...
of the sequence of projections
 gives rise to the infinite jet space
gives rise to the infinite jet space 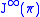 . A point
. A point 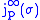 is the equivalence class of sections of
is the equivalence class of sections of  that have the same
that have the same 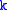 -jet in
-jet in  as
as  for all values of
for all values of  . The natural projection
. The natural projection  maps
maps  into
into  .
.Just by thinking in terms of coordinates,
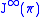 appears to be an infinite-dimensional geometric object. In fact, the simplest way of introducing a differentiable structure on
appears to be an infinite-dimensional geometric object. In fact, the simplest way of introducing a differentiable structure on 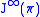 , not relying on differentiable charts, is given by the differential calculus over commutative algebras
, not relying on differentiable charts, is given by the differential calculus over commutative algebrasDifferential calculus over commutative algebras
In mathematics the differential calculus over commutative algebras is a part of commutative algebra based on the observation that most concepts known from classical differential calculus can be formulated in purely algebraic terms...
. Dual to the sequence of projections
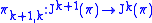 of manifolds is the sequence of injections
of manifolds is the sequence of injections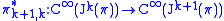
of commutative algebras. Let's denote
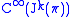 simply by
simply by 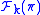 . Take now the direct limit
. Take now the direct limitDirect limit
In mathematics, a direct limit is a colimit of a "directed family of objects". We will first give the definition for algebraic structures like groups and modules, and then the general definition which can be used in any category.- Algebraic objects :In this section objects are understood to be...
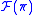 of the
of the  's. It will be a commutative algebra, which can be assumed to be the smooth functions algebra over the geometric object
's. It will be a commutative algebra, which can be assumed to be the smooth functions algebra over the geometric object 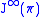 . Observe that
. Observe that 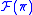 , being born as a direct limit, carries an additional structure: it is a filtered commutative algebra.
, being born as a direct limit, carries an additional structure: it is a filtered commutative algebra.Roughly speaking, a concrete element
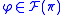 will always belong to some
will always belong to some 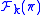 , so it is a smooth function on the finite-dimensional manifold
, so it is a smooth function on the finite-dimensional manifold 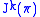 in the usual sense.
in the usual sense.Infinitely prolonged PDE's
Given a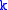 -th order system of PDE's
-th order system of PDE's 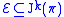 , the collection
, the collection 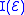 of vanishing on
of vanishing on  smooth functions on
smooth functions on 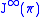 is an ideal
is an idealIdeal
-In philosophy:* Ideal , values that one actively pursues as goals* Platonic ideal, a philosophical idea of trueness of form, associated with Plato-In mathematics:* Ideal , special subsets of a ring considered in abstract algebra...
in the algebra
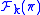 , and hence in the direct limit
, and hence in the direct limit 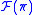 too.
too.Enhance
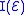 by adding all the possible compositions of total derivative
by adding all the possible compositions of total derivativeTotal derivative
In the mathematical field of differential calculus, the term total derivative has a number of closely related meanings.The total derivative of a function f, of several variables, e.g., t, x, y, etc., with respect to one of its input variables, e.g., t, is different from the partial derivative...
s applied to all its elements. This way we get a new ideal
 of
of 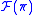 which is now closed under the operation of taking total derivative. The submanifold
which is now closed under the operation of taking total derivative. The submanifold 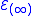 of
of  cut out by
cut out by 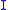 is called the infinite prolongation of
is called the infinite prolongation of  .
.Geometrically,
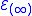 is the manifold of formal solutions of
is the manifold of formal solutions of  . A point
. A point 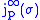 of
of 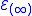 can be easily seen to be represented by a section
can be easily seen to be represented by a section  whose
whose 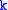 -jet's graph is tangent to
-jet's graph is tangent to  at the point
at the point 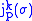 with arbitrarily high order of tangency.
with arbitrarily high order of tangency.Analytically, if
 is given by
is given by  , a formal solution can be understood as the set of Taylor coefficients of a section
, a formal solution can be understood as the set of Taylor coefficients of a section  in a point
in a point  that make vanish the Taylor series
that make vanish the Taylor seriesTaylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
of
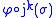 at the point
at the point  .
.Most importantly, the closure properties of
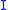 imply that
imply that  is tangent to the infinite-order contact structure
is tangent to the infinite-order contact structure  on
on 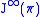 , so that by restricting
, so that by restricting  to
to 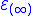 one gets the diffiety
one gets the diffietyDiffiety
In mathematics a diffiety, is a geometrical object introduced by playing the same role in the modern theory of partial differential equations as algebraic varieties play for algebraic equations....
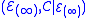 , and can study the associated C-spectral sequence.
, and can study the associated C-spectral sequence.Remark
This article has defined jets of local sections of a bundle, but it is possible to define jets of functions , where
, where  and
and  are manifolds; the jet of
are manifolds; the jet of  then just corresponds to the jet of the section
then just corresponds to the jet of the section |
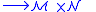 |
 |
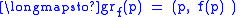 |
(
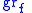 is known as the graph of the function
is known as the graph of the function  ) of the trivial bundle
) of the trivial bundle 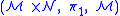 . However, this restriction does not simplify the theory, as the global triviality of
. However, this restriction does not simplify the theory, as the global triviality of  does not imply the global triviality of
does not imply the global triviality of 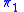 .
.

