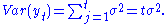Unit root
Encyclopedia
In time series
models in econometrics
(the application of statistical methods
to economics
), a unit root is a feature of processes that evolve through time
that can cause problems in statistical inference
if it is not adequately dealt with.
A linear stochastic process
has a unit root if 1 is a root of the process's characteristic equation
. Such a process is non-stationary
. If the other roots of the characteristic equation lie inside the unit circle — that is, have a modulus (absolute value
) less than one — then the first difference of the process will be stationary.
{
}, and suppose that it can be written as an autoregressive process of order p:
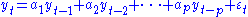
Here, {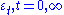
} is a serially uncorrelated, mean zero stochastic process with constant variance 
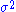. For convenience, assume 
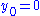. If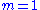 
is a root of the characteristic equation
:
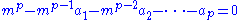
then the stochastic process has a unit root or, alternatively, is integrated of order
one, denoted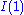
. If m = 1 is a root of multiplicity r, then the stochastic process is integrated of order r, denoted I(r).
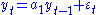, has a unit root when 
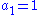. In this example, the characteristic equation is 
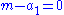. The root of the equation is 
.
If the process has a unit root, then it is a non-stationary time series. That is, the moments of the stochastic process depend on
. To illustrate the effect of a unit root, we can consider the first order case, starting from y0=0:
By repeated substitution, we can write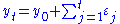
. Then the variance of 
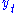is given by:
The variance depends on t since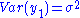
, while 
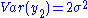. Note that the variance of the series is diverging to infinity with t.
and ARMA
models, other important models arise in regression analysis
where the model errors
may themselves have a time series
structure and thus may need to be modelled by an AR or ARMA process that may have a unit root, as discussed above. The finite sample
properties of regression models with first order ARMA errors, including unit roots, have been analyzed.
(OLS) is used to estimate the slope coefficients of the autoregressive model
. Use of OLS relies on the stochastic process being stationary. When the stochastic process is non-stationary, the use of OLS can produce invalid estimates. Granger and Newbold called such estimates 'spurious regression' results: high R2
values and high t-ratios yielding results with no economic meaning.
To estimate the slope coefficients, one should first conduct a unit root test
, whose null hypothesis
is that a unit root is present. If that hypothesis is rejected, one can use OLS. However, if the presence of a unit root is not rejected, then one should apply the difference operator
to the series. If another unit root test shows the differenced time series to be stationary, OLS can then be applied to this series to estimate the slope coefficients.
For example, in the AR(1) case,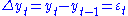
is stationary.
In the AR(2) case,
can be written as 
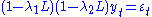where L is a lag operator that decreases the time index of a variable by one period: 
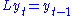. If 
, the model has a unit root and we can define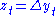 
; then
is stationary if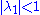
. OLS can be used to estimate the slope coefficient, 
.
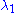If the process has multiple unit roots, the difference operator can be applied multiple times.
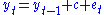
where c is a constant term referred to as the "drift" term, and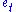
is white noise. Any non-zero value of the noise term, occurring for only one period, will permanently affect the value of 
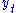as shown in the graph, so deviations from the line 
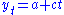are non-stationary; there is no reversion to any trend line. In contrast, a trend stationary process is given by
where k is the slope of the trend and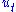
is noise (white noise in the simplest case; more generally, noise following its own stationary autoregressive process). Here any transient noise will not alter the long-run tendency for 
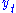to be on the trend line, as also shown in the graph. This process is said to be trend stationary because deviations from the trend line are stationary.
The issue is particularly popular in the literature on business cycles. Research on the subject began with Nelson and Plosser whose paper on GNP
and other output aggregates failed to reject the unit root hypothesis for these series. Since then, a debate—entwined with technical disputes on statistical methods—has ensued. Some economists argue that GDP has a unit root or structural break
, implying that economic downturns result in permanently lower GDP levels in the long run. Other economists argue that GDP is trend-stationary: That is, when GDP dips below trend during a downturn it later returns to the level implied by the trend so that there is no permanent decrease in output. While the literature on the unit root hypothesis may consist of arcane debate on statistical methods, the implications of the hypothesis can have concrete implications for economic forecasts and policies.
Time series
In statistics, signal processing, econometrics and mathematical finance, a time series is a sequence of data points, measured typically at successive times spaced at uniform time intervals. Examples of time series are the daily closing value of the Dow Jones index or the annual flow volume of the...
models in econometrics
Econometrics
Econometrics has been defined as "the application of mathematics and statistical methods to economic data" and described as the branch of economics "that aims to give empirical content to economic relations." More precisely, it is "the quantitative analysis of actual economic phenomena based on...
(the application of statistical methods
Statistics
Statistics is the study of the collection, organization, analysis, and interpretation of data. It deals with all aspects of this, including the planning of data collection in terms of the design of surveys and experiments....
to economics
Economics
Economics is the social science that analyzes the production, distribution, and consumption of goods and services. The term economics comes from the Ancient Greek from + , hence "rules of the house"...
), a unit root is a feature of processes that evolve through time
Dynamical system
A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...
that can cause problems in statistical inference
Statistical inference
In statistics, statistical inference is the process of drawing conclusions from data that are subject to random variation, for example, observational errors or sampling variation...
if it is not adequately dealt with.
A linear stochastic process
Stochastic process
In probability theory, a stochastic process , or sometimes random process, is the counterpart to a deterministic process...
has a unit root if 1 is a root of the process's characteristic equation
Characteristic equation
Characteristic equation may refer to:* Characteristic equation , used to solve linear differential equations* Characteristic equation, a characteristic polynomial equation in linear algebra used to find eigenvalues...
. Such a process is non-stationary
Stationary process
In the mathematical sciences, a stationary process is a stochastic process whose joint probability distribution does not change when shifted in time or space...
. If the other roots of the characteristic equation lie inside the unit circle — that is, have a modulus (absolute value
Absolute value
In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...
) less than one — then the first difference of the process will be stationary.
Definition
Consider a discrete time stochastic processStochastic process
In probability theory, a stochastic process , or sometimes random process, is the counterpart to a deterministic process...
{
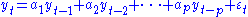
}, and suppose that it can be written as an autoregressive process of order p:Here, {
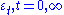} is a serially uncorrelated, mean zero stochastic process with constant variance 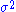. For convenience, assume 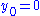. If 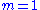is a root of the characteristic equationCharacteristic polynomial
In linear algebra, one associates a polynomial to every square matrix: its characteristic polynomial. This polynomial encodes several important properties of the matrix, most notably its eigenvalues, its determinant and its trace....
:
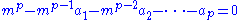
then the stochastic process has a unit root or, alternatively, is integrated of order
Order of integration
Order of integration, denoted I, is a summary statistic for a time series. It reports the minimum number of differences required to obtain a stationary series.- Integration of order zero :...
one, denoted
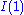. If m = 1 is a root of multiplicity r, then the stochastic process is integrated of order r, denoted I(r).Example
The first order autoregressive model,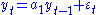, has a unit root when 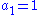. In this example, the characteristic equation is 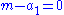. The root of the equation is .If the process has a unit root, then it is a non-stationary time series. That is, the moments of the stochastic process depend on
. To illustrate the effect of a unit root, we can consider the first order case, starting from y0=0:By repeated substitution, we can write
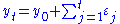. Then the variance of 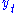is given by:
The variance depends on t since
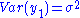, while 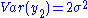. Note that the variance of the series is diverging to infinity with t.Related models
In addition to ARAutoregressive model
In statistics and signal processing, an autoregressive model is a type of random process which is often used to model and predict various types of natural phenomena...
and ARMA
Arma
-Businesses, institutions and organizations:* Agung Rai Museum of Art located in Ubud, Bali - also related to the ARMA resort* American Rock Mechanics Association, a geoscience organization...
models, other important models arise in regression analysis
Regression analysis
In statistics, regression analysis includes many techniques for modeling and analyzing several variables, when the focus is on the relationship between a dependent variable and one or more independent variables...
where the model errors
Errors and residuals in statistics
In statistics and optimization, statistical errors and residuals are two closely related and easily confused measures of the deviation of a sample from its "theoretical value"...
may themselves have a time series
Time series
In statistics, signal processing, econometrics and mathematical finance, a time series is a sequence of data points, measured typically at successive times spaced at uniform time intervals. Examples of time series are the daily closing value of the Dow Jones index or the annual flow volume of the...
structure and thus may need to be modelled by an AR or ARMA process that may have a unit root, as discussed above. The finite sample
Sample size
Sample size determination is the act of choosing the number of observations to include in a statistical sample. The sample size is an important feature of any empirical study in which the goal is to make inferences about a population from a sample...
properties of regression models with first order ARMA errors, including unit roots, have been analyzed.
Estimation when a unit root may be present
Often, ordinary least squaresOrdinary least squares
In statistics, ordinary least squares or linear least squares is a method for estimating the unknown parameters in a linear regression model. This method minimizes the sum of squared vertical distances between the observed responses in the dataset and the responses predicted by the linear...
(OLS) is used to estimate the slope coefficients of the autoregressive model
Autoregressive model
In statistics and signal processing, an autoregressive model is a type of random process which is often used to model and predict various types of natural phenomena...
. Use of OLS relies on the stochastic process being stationary. When the stochastic process is non-stationary, the use of OLS can produce invalid estimates. Granger and Newbold called such estimates 'spurious regression' results: high R2
Coefficient of determination
In statistics, the coefficient of determination R2 is used in the context of statistical models whose main purpose is the prediction of future outcomes on the basis of other related information. It is the proportion of variability in a data set that is accounted for by the statistical model...
values and high t-ratios yielding results with no economic meaning.
To estimate the slope coefficients, one should first conduct a unit root test
Unit root test
In statistics, a unit root test tests whether a time series variable is non-stationary using an autoregressive model. A well-known test that is valid in large samples is the augmented Dickey–Fuller test. The optimal finite sample tests for a unit root in autoregressive models were developed by John...
, whose null hypothesis
Null hypothesis
The practice of science involves formulating and testing hypotheses, assertions that are capable of being proven false using a test of observed data. The null hypothesis typically corresponds to a general or default position...
is that a unit root is present. If that hypothesis is rejected, one can use OLS. However, if the presence of a unit root is not rejected, then one should apply the difference operator
Finite difference
A finite difference is a mathematical expression of the form f − f. If a finite difference is divided by b − a, one gets a difference quotient...
to the series. If another unit root test shows the differenced time series to be stationary, OLS can then be applied to this series to estimate the slope coefficients.
For example, in the AR(1) case,
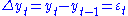is stationary.In the AR(2) case,
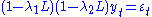can be written as where L is a lag operator that decreases the time index of a variable by one period: 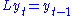. If , the model has a unit root and we can define 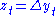; then
is stationary if
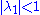. OLS can be used to estimate the slope coefficient, 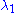.If the process has multiple unit roots, the difference operator can be applied multiple times.
Properties and characteristics of unit-root processes
- Shocks to a unit root process have permanent effects which do not decay as they would if the process were stationary
- As noted above, a unit root process has a variance that depends on t, and diverges to infinity
- If it is known that a series has a unit root, the series can be differenced to render it stationary. For example, if a series
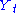is I(1), the series 
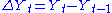is I(0) (stationary). It is hence called a difference stationary series.
Unit root hypothesis
Economists debate whether various economic statistics, especially output, have a unit root or are trend stationary. A unit root process with drift is given in the first-order case by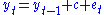where c is a constant term referred to as the "drift" term, and
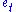is white noise. Any non-zero value of the noise term, occurring for only one period, will permanently affect the value of 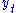as shown in the graph, so deviations from the line 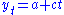are non-stationary; there is no reversion to any trend line. In contrast, a trend stationary process is given bywhere k is the slope of the trend and
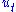is noise (white noise in the simplest case; more generally, noise following its own stationary autoregressive process). Here any transient noise will not alter the long-run tendency for 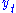to be on the trend line, as also shown in the graph. This process is said to be trend stationary because deviations from the trend line are stationary.The issue is particularly popular in the literature on business cycles. Research on the subject began with Nelson and Plosser whose paper on GNP
GNP
Gross National Product is the market value of all products and services produced in one year by labor and property supplied by the residents of a country...
and other output aggregates failed to reject the unit root hypothesis for these series. Since then, a debate—entwined with technical disputes on statistical methods—has ensued. Some economists argue that GDP has a unit root or structural break
Structural break
A structural break is a concept in econometrics. A structural break appears when we see an unexpected shift in a time series. This can lead to huge forecasting errors and unreliability of the model in general...
, implying that economic downturns result in permanently lower GDP levels in the long run. Other economists argue that GDP is trend-stationary: That is, when GDP dips below trend during a downturn it later returns to the level implied by the trend so that there is no permanent decrease in output. While the literature on the unit root hypothesis may consist of arcane debate on statistical methods, the implications of the hypothesis can have concrete implications for economic forecasts and policies.
See also
- Dickey–Fuller test
- Augmented Dickey–Fuller test
- Unit root testUnit root testIn statistics, a unit root test tests whether a time series variable is non-stationary using an autoregressive model. A well-known test that is valid in large samples is the augmented Dickey–Fuller test. The optimal finite sample tests for a unit root in autoregressive models were developed by John...
- Phillips–Perron test
- CointegrationCointegrationCointegration is a statistical property of time series variables. Two or more time series are cointegrated if they share a common stochastic drift.-Introduction:...
, determining the relationship between two variables having unit roots - Weighted symmetric unit root test (WS)
- Kwiatkowski, Phillips, Schmidt, Shin test, known as KPSS tests