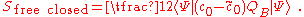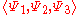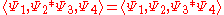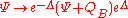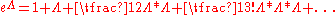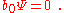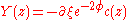String field theory
Encyclopedia
String field theory is a formalism in string theory
in which the dynamics of relativistic
strings is reformulated in the language of quantum field theory
. This is accomplished at the level of perturbation theory
by finding a collection of vertices for joining and splitting strings, as well as string propagator
s, that give a Feynman diagram
-like expansion for string scattering amplitudes. In most string field theories, this expansion is encoded by a classical action
found by second-quantizing the free string and adding interaction terms. As is usually the case in second quantization, a classical field configuration of the second-quantized theory is given by a wave function in the original theory. In the case of string field theory, this implies that a classical configuration, usually called the string field, is given by an element of the free string Fock space
.
The principal advantages of the formalism are that it allows the computation of off-shell amplitudes
and, when a classical action is available, gives non-perturbative information that cannot be seen directly from the standard genus expansion of string scattering. In particular, following the work of Ashoke Sen
, it has been useful in the study of tachyon condensation
on unstable D-branes. It has also had applications to topological string theory
, non-commutative geometry, and strings in low dimensions.
String field theories come in a number of varieties depending on which type of string is second quantized: Open string field theories describe the scattering of open strings, closed string field theories describe closed strings, while open-closed string field theories include both open and closed strings.
In addition, depending on the method used to fix the worldsheet diffeomorphisms and conformal transformations in the original free string theory, the resulting string field theories can be very different. Using light cone gauge
, yields light-cone string field theories whereas using BRST quantization
, one finds covariant string field theories. There are also hybrid string field theories, known as covariantized light-cone string field theories which use elements of both light-cone and BRST gauge-fixed string field theories.
A final form of string field theory, known as background independent open string field theory, takes a very different form; instead of second quantizing the worldsheet string theory, it second quantizes the space of two-dimensional quantum field theories.
and developed by Mandelstam, Michael Green
, John Schwarz and Lars Brink. An explicit description of the second-quantization of the light-cone string was given by Michio Kaku
and Keiji Kikkawa.
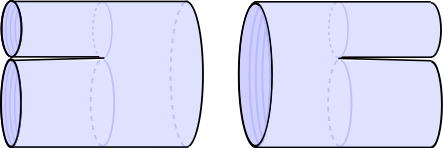
Light-cone string field theories were the first string field theories to be constructed and are based on the simplicity of string scattering in light-cone gauge. For example, in the bosonic closed string
case, the worldsheet scattering diagrams naturally take a Feynman diagram-like form, being built from two ingredients, a propagator
,
and two vertices for splitting and joining strings, which can be used to glue three propagators together,
These vertices and propagators produce a single cover of the moduli space of -point closed string scattering amplitudes so no higher order vertices are required. Similar vertices exist for the open string.
-point closed string scattering amplitudes so no higher order vertices are required. Similar vertices exist for the open string.
When one considers light-cone quantized superstrings, the discussion is more subtle as divergences can arise when the light-cone vertices collide. To produce a consistent theory, it is necessary to introduce higher order vertices, called contact terms, to cancel the divergences.
Light-cone string field theories have the disadvantage that they break manifest Lorentz invariance. However, in backgrounds with light-like killing vectors
, they can considerably simplify the quantization of the string action. Moreover, until the advent of the Berkovits string it was the only known method for quantizing strings in the presence of Ramond-Ramond field
s. In recent research, light-cone string field theory played an important role in understanding strings in pp-wave backgrounds.
String theory
String theory is an active research framework in particle physics that attempts to reconcile quantum mechanics and general relativity. It is a contender for a theory of everything , a manner of describing the known fundamental forces and matter in a mathematically complete system...
in which the dynamics of relativistic
Special relativity
Special relativity is the physical theory of measurement in an inertial frame of reference proposed in 1905 by Albert Einstein in the paper "On the Electrodynamics of Moving Bodies".It generalizes Galileo's...
strings is reformulated in the language of quantum field theory
Quantum field theory
Quantum field theory provides a theoretical framework for constructing quantum mechanical models of systems classically parametrized by an infinite number of dynamical degrees of freedom, that is, fields and many-body systems. It is the natural and quantitative language of particle physics and...
. This is accomplished at the level of perturbation theory
Perturbation theory (quantum mechanics)
In quantum mechanics, perturbation theory is a set of approximation schemes directly related to mathematical perturbation for describing a complicated quantum system in terms of a simpler one. The idea is to start with a simple system for which a mathematical solution is known, and add an...
by finding a collection of vertices for joining and splitting strings, as well as string propagator
Propagator
In quantum mechanics and quantum field theory, the propagator gives the probability amplitude for a particle to travel from one place to another in a given time, or to travel with a certain energy and momentum. Propagators are used to represent the contribution of virtual particles on the internal...
s, that give a Feynman diagram
Feynman diagram
Feynman diagrams are a pictorial representation scheme for the mathematical expressions governing the behavior of subatomic particles, first developed by the Nobel Prize-winning American physicist Richard Feynman, and first introduced in 1948...
-like expansion for string scattering amplitudes. In most string field theories, this expansion is encoded by a classical action
Action (physics)
In physics, action is an attribute of the dynamics of a physical system. It is a mathematical functional which takes the trajectory, also called path or history, of the system as its argument and has a real number as its result. Action has the dimension of energy × time, and its unit is...
found by second-quantizing the free string and adding interaction terms. As is usually the case in second quantization, a classical field configuration of the second-quantized theory is given by a wave function in the original theory. In the case of string field theory, this implies that a classical configuration, usually called the string field, is given by an element of the free string Fock space
Fock space
The Fock space is an algebraic system used in quantum mechanics to describe quantum states with a variable or unknown number of particles. It is named after V. A...
.
The principal advantages of the formalism are that it allows the computation of off-shell amplitudes
Probability amplitude
In quantum mechanics, a probability amplitude is a complex number whose modulus squared represents a probability or probability density.For example, if the probability amplitude of a quantum state is \alpha, the probability of measuring that state is |\alpha|^2...
and, when a classical action is available, gives non-perturbative information that cannot be seen directly from the standard genus expansion of string scattering. In particular, following the work of Ashoke Sen
Ashoke Sen
Ashoke Sen , FRS, is an Indian theoretical physicist. He has made a number of major original contributions to the subject of string theory, including his landmark paper on strong-weak coupling duality or S-duality, which was influential in changing the course of research in the field...
, it has been useful in the study of tachyon condensation
Tachyon condensation
In particle physics, theoretical processes that eliminate or resolve particles or fields into better understood phenomena are called, by extension and metaphor with the macroscopic process, "condensation"...
on unstable D-branes. It has also had applications to topological string theory
Topological string theory
In theoretical physics, topological string theory is a simplified version of string theory. The operators in topological string theory represent the algebra of operators in the full string theory that preserve a certain amount of supersymmetry...
, non-commutative geometry, and strings in low dimensions.
String field theories come in a number of varieties depending on which type of string is second quantized: Open string field theories describe the scattering of open strings, closed string field theories describe closed strings, while open-closed string field theories include both open and closed strings.
In addition, depending on the method used to fix the worldsheet diffeomorphisms and conformal transformations in the original free string theory, the resulting string field theories can be very different. Using light cone gauge
Light cone gauge
In theoretical physics, light cone gauge is an approach to remove the ambiguities arising from a gauge symmetry. While the term refers to several situations, a null component of a field A is set to zero in all cases.-Gauge theory:In gauge theory, light-cone gauge refers to the condition A^+=0...
, yields light-cone string field theories whereas using BRST quantization
BRST quantization
In theoretical physics, BRST quantization is a relatively rigorous mathematical approach to quantizing a field theory with a gauge symmetry...
, one finds covariant string field theories. There are also hybrid string field theories, known as covariantized light-cone string field theories which use elements of both light-cone and BRST gauge-fixed string field theories.
A final form of string field theory, known as background independent open string field theory, takes a very different form; instead of second quantizing the worldsheet string theory, it second quantizes the space of two-dimensional quantum field theories.
Light-cone string field theory
Light-cone string field theories were introduced by Stanley MandelstamStanley Mandelstam
Stanley Mandelstam is a South African-born theoretical physicist. He introduced the relativistically invariant Mandelstam variables into particle physics in 1958 as a convenient coordinate system for formulating his double dispersion relations...
and developed by Mandelstam, Michael Green
Michael Green (physicist)
Michael Boris Green FRS is a British physicist and one of the pioneers of string theory. Currently a professor in the Department of Applied Mathematics and Theoretical Physics and a Fellow in Clare Hall at the University of Cambridge in England, he succeeded Stephen Hawking on 1 November 2009...
, John Schwarz and Lars Brink. An explicit description of the second-quantization of the light-cone string was given by Michio Kaku
Michio Kaku
is an American theoretical physicist, the Henry Semat Professor of Theoretical Physics in the City College of New York of City University of New York, the co-founder of string field theory, and a "communicator" and "popularizer" of science...
and Keiji Kikkawa.
Light-cone string field theories were the first string field theories to be constructed and are based on the simplicity of string scattering in light-cone gauge. For example, in the bosonic closed string
Bosonic string theory
Bosonic string theory is the original version of string theory, developed in the late 1960s.In the early 1970s, supersymmetry was discovered in the context of string theory, and a new version of string theory called superstring theory became the real focus...
case, the worldsheet scattering diagrams naturally take a Feynman diagram-like form, being built from two ingredients, a propagator
Propagator
In quantum mechanics and quantum field theory, the propagator gives the probability amplitude for a particle to travel from one place to another in a given time, or to travel with a certain energy and momentum. Propagators are used to represent the contribution of virtual particles on the internal...
,
and two vertices for splitting and joining strings, which can be used to glue three propagators together,
These vertices and propagators produce a single cover of the moduli space of
 -point closed string scattering amplitudes so no higher order vertices are required. Similar vertices exist for the open string.
-point closed string scattering amplitudes so no higher order vertices are required. Similar vertices exist for the open string.When one considers light-cone quantized superstrings, the discussion is more subtle as divergences can arise when the light-cone vertices collide. To produce a consistent theory, it is necessary to introduce higher order vertices, called contact terms, to cancel the divergences.
Light-cone string field theories have the disadvantage that they break manifest Lorentz invariance. However, in backgrounds with light-like killing vectors
Killing vector field
In mathematics, a Killing vector field , named after Wilhelm Killing, is a vector field on a Riemannian manifold that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flows generated by Killing fields are continuous isometries of the manifold...
, they can considerably simplify the quantization of the string action. Moreover, until the advent of the Berkovits string it was the only known method for quantizing strings in the presence of Ramond-Ramond field
Ramond-Ramond field
In theoretical physics, Ramond–Ramond fields are differential form fields in the 10-dimensional spacetime of type II supergravity theories, which are the classical limits of type II string theory. The ranks of the fields depend on which type II theory is considered...
s. In recent research, light-cone string field theory played an important role in understanding strings in pp-wave backgrounds.
Free covariant string field theory
An important step in the construction of covariant string field theories (preserving manifest Lorentz invariance) was the construction of a covariant kinetic term. This kinetic term can be considered a string field theory in its own right: the string field theory of free strings. Since the work of Warren Siegel, it has been standard to first BRST-quantize the free string theory and then second quantize so that the classical fields of the string field theory include ghosts as well as matter fields. For example, in the case of the bosonic open string theory in 26-dimensional flat spacetime, a general element of the Fock-space of the BRST quantized string takes the form (in radial quantization in the upper half plane),-
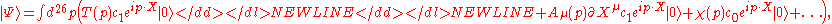
where is the free string vacuum and the dots represent more massive fields. In the language of worldsheet string theory,
is the free string vacuum and the dots represent more massive fields. In the language of worldsheet string theory,  ,
,  , and
, and 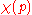 represent the amplitudes for the string to be found in the various basis states. After second quantization, they are interpreted instead as classical fields representing the tachyon
represent the amplitudes for the string to be found in the various basis states. After second quantization, they are interpreted instead as classical fields representing the tachyon  , gauge field
, gauge field  and a ghost field
and a ghost field  .
.
In the worldsheet string theory, the unphysical elements of the Fock space are removed by imposing the condition as well as the equivalence relation
as well as the equivalence relation  . After second quantization, the equivalence relation is interpreted as a gauge invariance, whereas the condition that
. After second quantization, the equivalence relation is interpreted as a gauge invariance, whereas the condition that  is physical is interpreted as an equation of motionEquation of motionEquations of motion are equations that describe the behavior of a system in terms of its motion as a function of time...
is physical is interpreted as an equation of motionEquation of motionEquations of motion are equations that describe the behavior of a system in terms of its motion as a function of time...
. Because the physical fields live at ghostnumber one, it is also assumed that the string field is a ghostnumber one element of the Fock space.
is a ghostnumber one element of the Fock space.
In the case of the open bosonic string a gauge-unfixed action with the appropriate symmetries and equations of motion was originally obtained by André NeveuAndré NeveuAndré Neveu is a French physicist working on string theory and quantum field theory who coinvented the Neveu-Schwarz algebra and the Gross-Neveu model.Neveu studied in Paris at the École normale supérieure...
, Hermann Nicolai and Peter C. West. It is given by-
-
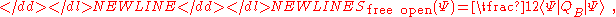
where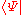 is the BPZ-dual of
is the BPZ-dual of 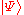 .
.
For the bosonic closed string, construction of a BRST-invariant kinetic term requires additionally that one impose and
and  . The kinetic term is then
. The kinetic term is then
Additional considerations are required for the superstrings to deal with the superghost zero-modes.
Witten's cubic open string field theory
The best studied and simplest of covariant interacting string field theories was constructed by Edward WittenEdward WittenEdward Witten is an American theoretical physicist with a focus on mathematical physics who is currently a professor of Mathematical Physics at the Institute for Advanced Study....
. It describes the dynamics of bosonic open strings and is given by adding to the free open string action a cubic vertex:-
-
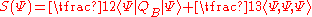 ,
,
-
where, as in the free case, is a ghostnumber one element of the BRST-quantized free bosonic open-string Fock-space.
is a ghostnumber one element of the BRST-quantized free bosonic open-string Fock-space.
The cubic vertex,
is a triliniar map which takes three string fields of total ghostnumber three and yields a number.
Following Witten, who was motivated by ideas from noncommutative geometry, it is conventional to introduce the -product defined implicitly through
-product defined implicitly through
The -product and cubic vertex satisfy a number of important properties (allowing the
-product and cubic vertex satisfy a number of important properties (allowing the 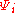 to be general ghost number fields):
to be general ghost number fields):
- Cyclicity :
-
BRST invariance :
For the -product, this implies that
-product, this implies that  acts as a graded derivation
acts as a graded derivation
-
- Associativity
In terms of the cubic vertex,
-
In these equations,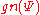 denotes the ghost number of
denotes the ghost number of 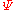 .
.
Gauge invariance
These properties of the cubic vertex are sufficient to show that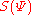 is invariant under
is invariant under
the Yang-Mills-like gauge transformation,
where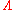 is an infinitesimal gauge parameter. Finite gauge transformations take the form
is an infinitesimal gauge parameter. Finite gauge transformations take the form
where the exponential is defined by,
Equations of motion
The equations of motion are given by
Because the string field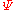 is an infinite collection of ordinary classical fields, these equations represent an infinite collection of non-linear coupled differential equations. There have been two approaches to finding solutions: First, numerically, one can truncate the string field to include only fields with mass less than a fixed bound, a procedure known as "level truncation". This reduces the equations of motion to a finite number of coupled differential equations and has led to the discovery of many solutions. Second, following the work of Martin Schnabl one can seek analytic solutions by carefully picking an ansatz which has simple behavior under star multiplication and action by the BRST operator. This has led to solutions representing marginal deformations as well as the tachyon vacuum solution
is an infinite collection of ordinary classical fields, these equations represent an infinite collection of non-linear coupled differential equations. There have been two approaches to finding solutions: First, numerically, one can truncate the string field to include only fields with mass less than a fixed bound, a procedure known as "level truncation". This reduces the equations of motion to a finite number of coupled differential equations and has led to the discovery of many solutions. Second, following the work of Martin Schnabl one can seek analytic solutions by carefully picking an ansatz which has simple behavior under star multiplication and action by the BRST operator. This has led to solutions representing marginal deformations as well as the tachyon vacuum solution
Quantization
To consistently quantize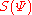 one has to fix a gauge. The traditional choice has been Feynman-Siegel gauge,
one has to fix a gauge. The traditional choice has been Feynman-Siegel gauge,
Because the gauge transformations are themselves redundant (there are gauge transformations of the gauge transformations), the gauge fixing procedure requires introducing an infinite number of ghosts via the BV formalismBatalin-Vilkovisky formalismIn theoretical physics, the Batalin–Vilkovisky formalism was developed as a method for determining the ghost structure for Lagrangian gauge theories, such as gravity and supergravity, whose corresponding Hamiltonian formulation has constraints not related to a Lie algebra In theoretical...
. The complete gauge fixed action is given by
where the field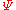 is now allowed to be of arbitrary ghostnumber. In this gauge, the Feynman diagrams are constructed from a single propagator and vertex. The propagator takes the form of a strip of worldsheet of width
is now allowed to be of arbitrary ghostnumber. In this gauge, the Feynman diagrams are constructed from a single propagator and vertex. The propagator takes the form of a strip of worldsheet of width  and length
and length 
There is also an insertion of an integral of the -ghost along the red line. The modulus,
-ghost along the red line. The modulus,  is integrated from 0 to
is integrated from 0 to  .
.
The three vertex can be described as a way of gluing three propagators together as shown in the following picture:
In order to represent the vertex embedded in three dimensions, the propagators have been folded in half along their midpoints. The resulting geometry is completely flat except for a single curvature singularity where the midpoints of the three propagators meet.
These Feynman diagrams generate a complete cover of the moduli space of open string scattering diagrams. It follows that, for on-shell amplitudes, the n-point open string amplitudes computed using Witten's open string field theory are identical to those computed using standard worldsheet methods.
Supersymmetric covariant open string field theories
There are two main constructions of supersymmetric extensions of Witten's cubic open string field theory. The first is very similar in form to its bosonic cousin and is known as modified cubic superstring field theory. The second, due to Nathan Berkovits is very different and is based on a WZWWess-Zumino-Witten modelIn theoretical physics and mathematics, the Wess–Zumino–Witten model, also called the Wess–Zumino–Novikov–Witten model, is a simple model of conformal field theory whose solutions are realized by affine Kac–Moody algebras...
-type action.
Modified cubic superstring field theory
The first consistent extension of Witten's bosonic open string field theory to the RNS string was constructed by
Christian Preitschopf, Charles Thorn and Scott Yost and independently by Irina Aref'eva, P. B. Medvedev and A. P. Zubarev. The NS string field is taken to be a ghostnumber one picture zero string field in the small Hilbert space (i.e. ).
).
The action takes a very similar form to bosonic action,-
-

where
is the inverse picture changing operator. The suggested picture number extension of this theory to the Ramond sector might be problematic.
picture number extension of this theory to the Ramond sector might be problematic.
This action has been shown to reproduce tree-level amplitudes and has a tachyon vacuum solution with the correct energy.
The one subtlety in the action is the insertion of picture changing operators at the midpoint, which imply that the linearized equations of motion take the form
Because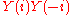 has a non-trivial kernel, there are potentially extra solutions that are not in the cohomology of
has a non-trivial kernel, there are potentially extra solutions that are not in the cohomology of  . However, such solutions would have operator insertions near the midpoint and would be potentially singular, and importance of this problem remains unclear.
. However, such solutions would have operator insertions near the midpoint and would be potentially singular, and importance of this problem remains unclear.
Berkovits superstring field theory
A very different supersymmetric action for the open string was constructed by Nathan Berkovits. It takes the form-
-

where all of the products are performed using the -product including the anticommutator
-product including the anticommutator  , and
, and 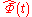 is any string field such that
is any string field such that  and
and 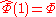 . The string field
. The string field 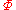 is taken to be in the NS sector of the large Hilbert space, i.e. including the zero mode of
is taken to be in the NS sector of the large Hilbert space, i.e. including the zero mode of 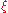 . It is not known how to incorporate the R sector, although some preliminary ideas exist.
. It is not known how to incorporate the R sector, although some preliminary ideas exist.
The equations of motion take the form
The action is invariant under the gauge invariance
The principal advantage of this action is that it free from any insertions of picture-changing operators. It has been shown to reproduce correctly tree level amplitudes and has been found, numerically, to have a tachyon vacuum with appropriate energy. The only known analytic solutions to the classical equations of motion are marginal deformations.
Other formulations of covariant open superstring field theory
A formulation of superstring field theory using the non-minimal pure-spinor variables was introduced by Berkovits. The action is cubic and includes a mid-point insertion whose kernel is trivial. As always within the pure-spinor formulation, the Ramond sector can be easily treated. However, it is not known how to incorporate the GSO- sectors into the formalism.
In an attempt to resolve the allegedly problematic mid-point insertion of the modified cubic theory, Berkovits and Siegel proposed a superstring field theory based on a non-minimal extension of the RNS string, which uses a mid-point insertion with no kernel. It is not clear if such insertions are in any way better than mid-point insertions with non-trivial kernels.
Covariant closed string field theory
Covariant closed string field theories are considerably more complicated than their open string cousins. Even if one wants to construct a string field theory which only reproduces tree-level interactions between closed strings, the classical action must contain an infinite number of vertices consisting of string polyhedra.
If one demands that on-shell scattering diagrams be reproduced to all orders in the string coupling, one must also include additional vertices arising from higher genus (and hence higher order in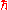 ) as well. In general, a manifestly BV invariant, quantizable action takes the form
) as well. In general, a manifestly BV invariant, quantizable action takes the form
where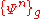 denotes an
denotes an  th order vertex arising from a genus
th order vertex arising from a genus 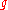 surface and
surface and 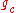 is the closed string coupling. The structure of the vertices is in principle determined by a minimal area prescription, although, even for the polyhedral vertices, explicit computations have only been performed to quintic order.
is the closed string coupling. The structure of the vertices is in principle determined by a minimal area prescription, although, even for the polyhedral vertices, explicit computations have only been performed to quintic order.
Covariant heterotic string field theory
A formulation of the NS sector of the heterotic string was given by Berkovits, Okawa and Zwiebach.
The formulation amalgams bosonic closed string field theory with Berkovits' superstring field theory.
See also
- Conformal field theoryConformal field theoryA conformal field theory is a quantum field theory that is invariant under conformal transformations...
- F-theoryF-theoryF-theory is a branch of string theory developed by Cumrun Vafa. The new vacua described as F-theory were discovered by Vafa, and it also allowed string theorists to construct new realistic vacua — in the form of F-theory compactified on elliptically fibered Calabi-Yau four-folds...
- FuzzballsFuzzballsFuzzballs are theorized by some superstring theory scientists to be the true quantum description of black holes. The theory resolves two intractable problems that classic black holes pose for modern physics:...
- List of string theory topics
- Little string theoryLittle string theoryIn theoretical physics, little string theory is a non-gravitational non-local theory in six spacetime dimensions that can be obtained as an effective theory of NS5-branes in the limit in which gravity decouples. Little string theories exhibit T-duality, much like the full string theory....
- Loop quantum gravityLoop quantum gravityLoop quantum gravity , also known as loop gravity and quantum geometry, is a proposed quantum theory of spacetime which attempts to reconcile the theories of quantum mechanics and general relativity...
- Relationship between string theory and quantum field theoryRelationship between string theory and quantum field theoryMany first principles in quantum field theory are explained, or get further insight, in string theory:* Emission and absorption: one of the most basic building blocks of quantum field theory, is the notion that particles can emit and absorb other particles...
- String cosmologyString cosmologyString cosmology is a relatively new field that tries to apply equations of string theory to solve the questions of early cosmology. A related area of study is brane cosmology ....
- SupergravitySupergravityIn theoretical physics, supergravity is a field theory that combines the principles of supersymmetry and general relativity. Together, these imply that, in supergravity, the supersymmetry is a local symmetry...
- The Elegant UniverseThe Elegant UniverseThe Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory is a book by Brian Greene published in 1999, which introduces string and superstring theory, and provides a comprehensive though non-technical assessment of the theory and some of its shortcomings...
- Zeta function regularizationZeta function regularizationIn mathematics and theoretical physics, zeta function regularization is a type of regularization or summability method that assigns finite values to divergent sums or products, and in particular can be used to define determinants and traces of some self-adjoint operators...
-
-
-
-
-
-
-