
Sea ice emissivity modelling
Encyclopedia
With increased interest in sea ice and its effects on the global climate
, efficient methods are required to monitor both its extent and exchange processes. Satellite-mounted, microwave
radiometers, such SSMI, AMSR and AMSU
, are an ideal tool for the task because they can see through cloud cover, and they have frequent, global coverage. A passive microwave instrument detects objects through emitted radiation since different substance have different emission spectra. To help us detect sea ice more efficiently, we need to model these emission processes. The interaction of sea ice with electromagnetic radiation in the microwave range is still not well understood.
. The diagram to the right shows a ray passing through an ice sheet with several layers. These layers represent the air above the ice, the snow layer (if applicable), ice with different electro-magnetic properties and the water below the ice. Interfaces between the layers may be continuous (in the case of ice with varying salt content along the vertical axis, but formed in the same way and in the same time period), in which case the reflection coefficients, Ri will be zero, or discontinuous (in the case of the ice-snow interface), in which case reflection coefficients must be calculated—see below. Each layer is characterized by its physical properties: temperature, Ti, complex permittivity,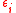 and thickness,
and thickness,  , and will have an upwelling component of the radiation,
, and will have an upwelling component of the radiation,  , and a downwelling component,
, and a downwelling component,  , passing through it. Since we assume plane-parallel geometry, all reflected rays will be at the same angle and we need only account for radiation along a single line-of-sight.
, passing through it. Since we assume plane-parallel geometry, all reflected rays will be at the same angle and we need only account for radiation along a single line-of-sight.
Summing the contributions from each layer generates the following sparse
system of linear equations:


where Ri is the ith reflection coefficient
, calculated via the
Fresnel equations
and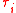 is the ith transmission coefficient
is the ith transmission coefficient
:
Climate
Climate encompasses the statistics of temperature, humidity, atmospheric pressure, wind, rainfall, atmospheric particle count and other meteorological elemental measurements in a given region over long periods...
, efficient methods are required to monitor both its extent and exchange processes. Satellite-mounted, microwave
Microwave
Microwaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
radiometers, such SSMI, AMSR and AMSU
Advanced Microwave Sounding Unit
The Advanced microwave sounding unit is a multi-channel microwave radiometer installed on meteorological satellites. The instrument examines several bands of microwave radiation from the atmosphere to perform atmospheric sounding of temperature and moisture levels.-Products:Level-1 radiance data...
, are an ideal tool for the task because they can see through cloud cover, and they have frequent, global coverage. A passive microwave instrument detects objects through emitted radiation since different substance have different emission spectra. To help us detect sea ice more efficiently, we need to model these emission processes. The interaction of sea ice with electromagnetic radiation in the microwave range is still not well understood.
Radiative transfer modelling
When scattering is neglected, sea ice emissivity can be modelled through radiative transferRadiative transfer
Radiative transfer is the physical phenomenon of energy transfer in the form of electromagnetic radiation. The propagation of radiation through a medium is affected by absorption, emission and scattering processes. The equation of radiative transfer describes these interactions mathematically...
. The diagram to the right shows a ray passing through an ice sheet with several layers. These layers represent the air above the ice, the snow layer (if applicable), ice with different electro-magnetic properties and the water below the ice. Interfaces between the layers may be continuous (in the case of ice with varying salt content along the vertical axis, but formed in the same way and in the same time period), in which case the reflection coefficients, Ri will be zero, or discontinuous (in the case of the ice-snow interface), in which case reflection coefficients must be calculated—see below. Each layer is characterized by its physical properties: temperature, Ti, complex permittivity,
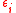 and thickness,
and thickness,  , and will have an upwelling component of the radiation,
, and will have an upwelling component of the radiation,  , and a downwelling component,
, and a downwelling component,  , passing through it. Since we assume plane-parallel geometry, all reflected rays will be at the same angle and we need only account for radiation along a single line-of-sight.
, passing through it. Since we assume plane-parallel geometry, all reflected rays will be at the same angle and we need only account for radiation along a single line-of-sight.Summing the contributions from each layer generates the following sparse
Sparse matrix
In the subfield of numerical analysis, a sparse matrix is a matrix populated primarily with zeros . The term itself was coined by Harry M. Markowitz....
system of linear equations:


where Ri is the ith reflection coefficient
Reflection coefficient
The reflection coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered. A reflection coefficient describes either the amplitude or the intensity of a reflected wave relative to an incident wave...
, calculated via the
Fresnel equations
Fresnel equations
The Fresnel equations , deduced by Augustin-Jean Fresnel , describe the behaviour of light when moving between media of differing refractive indices...
and
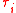 is the ith transmission coefficient
is the ith transmission coefficientTransmission coefficient
The transmission coefficient is used in physics and electrical engineering when wave propagation in a medium containing discontinuities is considered...
:
-

where is the transmission angle in the ith layer, from Snell's lawSnell's lawIn optics and physics, Snell's law is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through a boundary between two different isotropic media, such as water and glass...
is the transmission angle in the ith layer, from Snell's lawSnell's lawIn optics and physics, Snell's law is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through a boundary between two different isotropic media, such as water and glass...
,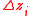 is the layer thickness and
is the layer thickness and 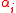 is the attenuation coefficientAttenuation coefficientThe attenuation coefficient is a quantity that characterizes how easily a material or medium can be penetrated by a beam of light, sound, particles, or other energy or matter. A large attenuation coefficient means that the beam is quickly "attenuated" as it passes through the medium, and a small...
is the attenuation coefficientAttenuation coefficientThe attenuation coefficient is a quantity that characterizes how easily a material or medium can be penetrated by a beam of light, sound, particles, or other energy or matter. A large attenuation coefficient means that the beam is quickly "attenuated" as it passes through the medium, and a small...
:
-
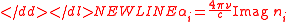
where is the frequency and c is the speed of light—see Beer's law.
is the frequency and c is the speed of light—see Beer's law.
The most important quantity in this calculation, and also the most difficult to establish with any certainty, is the complex refractive indexRefractive indexIn optics the refractive index or index of refraction of a substance or medium is a measure of the speed of light in that medium. It is expressed as a ratio of the speed of light in vacuum relative to that in the considered medium....
,
ni. Since sea ice is non-magnetic, it can be calculated from relative permittivityPermittivityIn electromagnetism, absolute permittivity is the measure of the resistance that is encountered when forming an electric field in a medium. In other words, permittivity is a measure of how an electric field affects, and is affected by, a dielectric medium. The permittivity of a medium describes how...
alone:
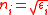
Effective permittivity
As established in the previous section, the most important quantity in radiative transfer calculations of sea ice is the relative permittivity. Sea ice is a complex composite composed of pure ice and included pockets of air and highly saline brineBrineBrine is water, saturated or nearly saturated with salt .Brine is used to preserve vegetables, fruit, fish, and meat, in a process known as brining . Brine is also commonly used to age Halloumi and Feta cheeses, or for pickling foodstuffs, as a means of preserving them...
. The electro-magnetic properties of such a mixture will be different from, and normally somewhere in between (though not always—see, for instance, metamaterialMetamaterialMetamaterials are artificial materials engineered to have properties that may not be found in nature. Metamaterials usually gain their properties from structure rather than composition, using small inhomogeneities to create effective macroscopic behavior....
), those of its constituents. Since it is not just the relative composition that is important, but also the geometry, the calculation of effective permittivities introduces a high level of uncertainty.
Vant et al.
have performed actual measurements of sea relative permittivities at frequencies between 0.1 and 4.0 GHz which they have encapsulated in the following formula:
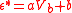
where is the real or imaginary effective relative permittivity, Vb is the relative brine volume—see sea ice growth processesSea ice growth processesSea ice is a complex composite composed primarily of pure ice in various states of crystallization along with air bubbles and included pockets of brine...
is the real or imaginary effective relative permittivity, Vb is the relative brine volume—see sea ice growth processesSea ice growth processesSea ice is a complex composite composed primarily of pure ice in various states of crystallization along with air bubbles and included pockets of brine...
-- and a and b are constants. This empirical model shows some agreement with dielectric mixture models based on Maxwell's equationsMaxwell's equationsMaxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
in the low frequency limit, such as this formula from Sihvola and Kong
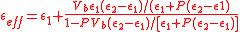
where is the relative permittivity of the background material (pure ice),
is the relative permittivity of the background material (pure ice),  is the relative permittivity of the inclusion material (brine) and P is a depolarization factor based on the geometry of the brine inclusions. Brine inclusions are frequently modelled as vertically-oriented needles for which the depolarization factor is P=0.5 in the vertical direction and P=0. in the horizontal.
is the relative permittivity of the inclusion material (brine) and P is a depolarization factor based on the geometry of the brine inclusions. Brine inclusions are frequently modelled as vertically-oriented needles for which the depolarization factor is P=0.5 in the vertical direction and P=0. in the horizontal.
The two formulas, while they correlate strongly, disagree in both relative and absolute magnitudes.
Pure ice is an almost perfect dielectricDielectricA dielectric is an electrical insulator that can be polarized by an applied electric field. When a dielectric is placed in an electric field, electric charges do not flow through the material, as in a conductor, but only slightly shift from their average equilibrium positions causing dielectric...
with a real permittivity of roughly 3.15 in the microwaveMicrowaveMicrowaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
range which is fairly independent of frequency while the imaginary component is negligible, especially in comparison with the brine which is extremely lossy.
Meanwhile, the permittivity of the brineBrineBrine is water, saturated or nearly saturated with salt .Brine is used to preserve vegetables, fruit, fish, and meat, in a process known as brining . Brine is also commonly used to age Halloumi and Feta cheeses, or for pickling foodstuffs, as a means of preserving them...
, which has both a large real part and a large imaginary part, is normally calculated with a complex formula based on Debye relaxation curves.
Scattering
Emissivity calculations based strictly on radiative transfer tend to underestimate the brightness temperatures of sea ice, especially in the higher frequencies, because both included brine and air pockets within the ice will tend to scatterScatteringScattering is a general physical process where some forms of radiation, such as light, sound, or moving particles, are forced to deviate from a straight trajectory by one or more localized non-uniformities in the medium through which they pass. In conventional use, this also includes deviation of...
the radiation.
Indeed, as ice becomes more opaque with higher frequency, radiative transfer becomes less important while scattering processes begin to dominate.
Scattering in sea ice is frequently modelled with a Born approximationBorn approximationIn scattering theory and, in particular in quantum mechanics, the Born approximation consists of taking the incident field in place of the total field as the driving field at each point in the scatterer. Born approximation is named after Max Born, winner of the 1954 Nobel Prize for physics.It is...
such as in strong fluctuation theory.
Scattering coefficients calculated at each layer must also be vertically integrated. The Microwave Emission Model of Layered Snowpack (MEMLS)
uses a six-flux radiative transfer model to integrate both the scattering coefficients and the effective permittivities with scattering coefficients calculated either empirically or with a distorted Born approximation.
Scattering processes in sea ice are relatively poorly understood and scattering models poorly validated empirically.
Other factors
There are many other factors not accounted for in the models described above. Mills and Heygster, for instance, show that sea ice ridging may have a significant effect on the signal. In such case, the ice can no longer be modelled using plane-parallel geometry. In addition to ridging, surface scattering from smaller-scale roughness must also be considered.
Since the microstructural properties of sea ice tend to be anisotropic, permittivity is ideally modelled as a tensorTensorTensors are geometric objects that describe linear relations between vectors, scalars, and other tensors. Elementary examples include the dot product, the cross product, and linear maps. Vectors and scalars themselves are also tensors. A tensor can be represented as a multi-dimensional array of...
. This anisotropy will also affect the signal in the higher Stokes componentsStokes parametersThe Stokes parameters are a set of values that describe the polarization state of electromagnetic radiation. They were defined by George Gabriel Stokes in 1852, as a mathematically convenient alternative to the more common description of incoherent or partially polarized radiation in terms of its...
, relevant for polarimetric radiometers such as WINDSATWINDSATWINDSAT is a joint NOAA Integrated Program Office/Department of Defense/NASA demonstration project, intended to measure ocean surface wind speed and wind direction from space using a polarimetric radiometer...
.
Both a sloping ice surface, as in the case of ridging—see polarization mixing,
as well as scattering, especially from non-symmetric scatterers,
will cause a transfer of intensity between the different Stokes components—see vector radiative transfer.
See also
- sea ice growth processesSea ice growth processesSea ice is a complex composite composed primarily of pure ice in various states of crystallization along with air bubbles and included pockets of brine...
- metamaterialMetamaterialMetamaterials are artificial materials engineered to have properties that may not be found in nature. Metamaterials usually gain their properties from structure rather than composition, using small inhomogeneities to create effective macroscopic behavior....
- sea ice concentrationSea ice concentrationSea ice concentration is a useful variable for climatescientists and nautical navigators. It is defined as the area ofsea ice relative to the total at a given point in the ocean....
- sea ice thicknessSea ice thicknessSea ice thickness is an important climate-related variable whose determination from satellite measurements is still an unsolved problem. While ice concentration is often used as a marker for climate change, the more important variable is sea ice volume which can be determined by multiplying...
- sea ice growth processes
-

