
Mapping torus
Encyclopedia
In mathematics
, the mapping torus in topology
of a homeomorphism
f of some topological space
X to itself is a particular geometric construction with f. Take the cartesian product
of X with a closed interval I, and glue the boundary components together by the static homeomorphism:
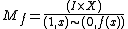
The result is a fiber bundle
whose base is a circle and whose fiber is the original space X.
If X is a manifold
, Mf will be a manifold of dimension one higher, and it is said to "fiber over the circle"
.
Mapping tori of surface homeomorphisms play a key role in the theory of 3-manifold
s and have been intensely studied. If S is a closed surface of genus g ≥ 2 and if f is a self-homeomorphism of S, the mapping torus Mf is a closed
3-manifold
that fibers
over the circle
with fiber S. A deep result of Thurston
states that in this case the 3-manifold
Mf is a hyperbolic
if and only if f is a pseudo-Anosov homeomorphism
of S.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the mapping torus in topology
Topology
Topology is a major area of mathematics concerned with properties that are preserved under continuous deformations of objects, such as deformations that involve stretching, but no tearing or gluing...
of a homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
f of some topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
X to itself is a particular geometric construction with f. Take the cartesian product
Cartesian product
In mathematics, a Cartesian product is a construction to build a new set out of a number of given sets. Each member of the Cartesian product corresponds to the selection of one element each in every one of those sets...
of X with a closed interval I, and glue the boundary components together by the static homeomorphism:
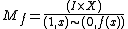
The result is a fiber bundle
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
whose base is a circle and whose fiber is the original space X.
If X is a manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
, Mf will be a manifold of dimension one higher, and it is said to "fiber over the circle"
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
.
Mapping tori of surface homeomorphisms play a key role in the theory of 3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
s and have been intensely studied. If S is a closed surface of genus g ≥ 2 and if f is a self-homeomorphism of S, the mapping torus Mf is a closed
Closed manifold
In mathematics, a closed manifold is a type of topological space, namely a compact manifold without boundary. In contexts where no boundary is possible, any compact manifold is a closed manifold....
3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
that fibers
Fiber bundle
In mathematics, and particularly topology, a fiber bundle is intuitively a space which locally "looks" like a certain product space, but globally may have a different topological structure...
over the circle
Circle
A circle is a simple shape of Euclidean geometry consisting of those points in a plane that are a given distance from a given point, the centre. The distance between any of the points and the centre is called the radius....
with fiber S. A deep result of Thurston
William Thurston
William Paul Thurston is an American mathematician. He is a pioneer in the field of low-dimensional topology. In 1982, he was awarded the Fields Medal for his contributions to the study of 3-manifolds...
states that in this case the 3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
Mf is a hyperbolic
Hyperbolic manifold
In mathematics, a hyperbolic n-manifold is a complete Riemannian n-manifold of constant sectional curvature -1.Every complete, connected, simply-connected manifold of constant negative curvature −1 is isometric to the real hyperbolic space Hn. As a result, the universal cover of any closed manifold...
if and only if f is a pseudo-Anosov homeomorphism
Pseudo-Anosov map
In mathematics, specifically in topology, a pseudo-Anosov map is a type of a diffeomorphism or homeomorphism of a surface. It is a generalization of a linear Anosov diffeomorphism of the torus...
of S.

