
Divisor function
Encyclopedia
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, and specifically in number theory
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
, a divisor function is an arithmetical function related to the divisor
Divisor
In mathematics, a divisor of an integer n, also called a factor of n, is an integer which divides n without leaving a remainder.-Explanation:...
s of an integer
Integer
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
. When referred to as the divisor function, it counts the number of divisors of an integer. It appears in a number of remarkable identities, including relationships on the Riemann zeta function and the Eisenstein series
Eisenstein series
Eisenstein series, named after German mathematician Gotthold Eisenstein, are particular modular forms with infinite series expansions that may be written down directly...
of modular form
Modular form
In mathematics, a modular form is a analytic function on the upper half-plane satisfying a certain kind of functional equation and growth condition. The theory of modular forms therefore belongs to complex analysis but the main importance of the theory has traditionally been in its connections...
s. Divisor functions were studied by Ramanujan, who gave a number of important congruences
Modular arithmetic
In mathematics, modular arithmetic is a system of arithmetic for integers, where numbers "wrap around" after they reach a certain value—the modulus....
and identities
Identity (mathematics)
In mathematics, the term identity has several different important meanings:*An identity is a relation which is tautologically true. This means that whatever the number or value may be, the answer stays the same. For example, algebraically, this occurs if an equation is satisfied for all values of...
.
A related function is the divisor summatory function, which, as the name implies, is a sum over the divisor function.
Definition
The sum of positive divisors function σx(n), for a real or complex number x, is defined as the sumSUM
SUM can refer to:* The State University of Management* Soccer United Marketing* Society for the Establishment of Useful Manufactures* StartUp-Manager* Software User’s Manual,as from DOD-STD-2 167A, and MIL-STD-498...
of the xth powers
Exponentiation
Exponentiation is a mathematical operation, written as an, involving two numbers, the base a and the exponent n...
of the positive divisor
Divisor
In mathematics, a divisor of an integer n, also called a factor of n, is an integer which divides n without leaving a remainder.-Explanation:...
s of n, or

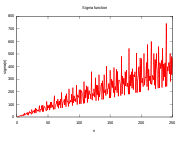
The notations d(n), ν(n) and τ(n) (for the German Teiler = divisors) are also used to denote σ0(n), or the number-of-divisors function . When x is 1, the function is called the sigma function or sum-of-divisors function, and the subscript is often omitted, so σ(n) is equivalent to σ1(n) .
The aliquot sum s(n) of n is the sum of the proper divisors (that is, the divisors excluding n itself), and equals σ1(n) − n; the aliquot sequence
Aliquot sequence
In mathematics, an aliquot sequence is a recursive sequence in which each term is the sum of the proper divisors of the previous term. The aliquot sequence starting with a positive integer k can be defined formally in terms of the sum-of-divisors function σ1 in the following way:For example, the...
of n is formed by repeatedly applying the aliquot sum function.
Example
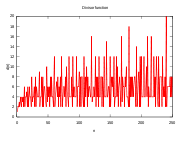
For example, σ0(12) is the number of the divisors of 12:-

while σ1(12) is the sum of all the divisors:
-

and the aliquot sum s(12) of proper divisors is:
-

Table of values
n Divisors σ0(n) σ1(n) s(n) = σ1(n) − n Comment 1 1 1 1 0 square number: σ0(n) is odd; power of 2: s(n) = n − 1 (almost-perfect) 2 1,2 2 3 1 3 1,3 2 4 1 4 1,2,4 3 7 3 square number: σ0(n) is odd; power of 2: s(n) = n − 1 (almost-perfect) 5 1,5 2 6 1 6 1,2,3,6 4 12 6 first perfect number Perfect numberIn number theory, a perfect number is a positive integer that is equal to the sum of its proper positive divisors, that is, the sum of its positive divisors excluding the number itself . Equivalently, a perfect number is a number that is half the sum of all of its positive divisors i.e...
: s(n) = n7 1,7 2 8 1 8 1,2,4,8 4 15 7 power of 2: s(n) = n − 1 (almost-perfect) 9 1,3,9 3 13 4 square number: σ0(n) is odd 10 1,2,5,10 4 18 8 11 1,11 2 12 1 12 1,2,3,4,6,12 6 28 16 first abundant number: s(n) > n 13 1,13 2 14 1 14 1,2,7,14 4 24 10 15 1,3,5,15 4 24 9 16 1,2,4,8,16 5 31 15 square number: σ0(n) is odd; power of 2: s(n) = n − 1 (almost-perfect)
The cases , and so on are tabulated
in , , ,
, , ...
Properties
For a non-square integer every divisor d of n is paired with divisor n/d of n and is then even; for a square integer one divisor (namely
is then even; for a square integer one divisor (namely  ) is not paired with a distinct divisor and
) is not paired with a distinct divisor and  is then odd.
is then odd.
For a prime numberPrime numberA prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
p,

because by definition, the factors of a prime number are 1 and itself. Also,where pn# denotes the primorialPrimorialIn mathematics, and more particularly in number theory, primorial is a function from natural numbers to natural numbers similar to the factorial function, but rather than multiplying successive positive integers, only successive prime numbers are multiplied...
,

since n prime factors allow a sequence of binary selection ( or 1) from n terms for each proper divisor formed.
or 1) from n terms for each proper divisor formed.
Clearly, 1 < d(n) < n and σ(n) > n for all n > 2.
The divisor function is multiplicativeMultiplicative functionIn number theory, a multiplicative function is an arithmetic function f of the positive integer n with the property that f = 1 and whenevera and b are coprime, then...
, but not completely multiplicativeCompletely multiplicative functionIn number theory, functions of positive integers which respect products are important and are called completely multiplicative functions or totally multiplicative functions. Especially in number theory, a weaker condition is also important, respecting only products of coprime numbers, and such...
. The consequence of this is that, if we write

where r = ω(n) is the number of distinct prime factorPrime factorIn number theory, the prime factors of a positive integer are the prime numbers that divide that integer exactly, without leaving a remainder. The process of finding these numbers is called integer factorization, or prime factorization. A prime factor can be visualized by understanding Euclid's...
s of n, pi is the ith prime factor, and ai is the maximum power of pi by which n is divisible, then we have
which is equivalent to the useful formula:
It follows (by setting x = 0) that d(n) is:
For example, if n is 24, there are two prime factors (p1 is 2; p2 is 3); noting that 24 is the product of 23×31, a1 is 3 and a2 is 1. Thus we can calculate d(24) as so:
-

The eight divisors counted by this formula are 1, 2, 4, 8, 3, 6, 12, and 24.
We also note s(n) = σ(n) − n. Here s(n) denotes the sum of the proper divisors of n, i.e. the divisors of n excluding n itself.
This function is the one used to recognize perfect numberPerfect numberIn number theory, a perfect number is a positive integer that is equal to the sum of its proper positive divisors, that is, the sum of its positive divisors excluding the number itself . Equivalently, a perfect number is a number that is half the sum of all of its positive divisors i.e...
s which are the n for which s(n) = n. If s(n) > n then n is an abundant number and if s(n) < n then n is a deficient numberDeficient numberIn number theory, a deficient number or defective number is a number n for which the sum of divisors σIn number theory, a deficient number or defective number is a number n for which the sum of divisors σIn number theory, a deficient number or defective number is a number n for which...
.
If n is a power of 2, e.g. , then
, then  and s(n) = n - 1, which makes n almost-perfectAlmost perfect numberIn mathematics, an almost perfect number is a natural number n such that the sum of all divisors of n is equal to 2n - 1, the sum of all proper divisors of n, s = σ - n, then being equal to n - 1...
and s(n) = n - 1, which makes n almost-perfectAlmost perfect numberIn mathematics, an almost perfect number is a natural number n such that the sum of all divisors of n is equal to 2n - 1, the sum of all proper divisors of n, s = σ - n, then being equal to n - 1...
.
As an example, for two distinct primes p and q with p < q, let

Then

and

where φ(n) is Euler's totient function.
Then, the roots of:
allows us to express p and q in terms of σ(n) and φ(n) only, without even knowing n or p+q, as:

Also, knowing n and either σ(n) or φ(n) (or knowing p+q and either σ(n) or φ(n)) allows us to easily find p and q.
In 1984, Roger Heath-BrownRoger Heath-BrownDavid Rodney "Roger" Heath-Brown F.R.S. , is a British mathematician working in the field of analytic number theory. He was an undergraduate and graduate student of Trinity College, Cambridge; his research supervisor was Alan Baker...
proved that
- d(n) = d(n + 1)
will occur infinitely often.
Series relations
Two Dirichlet series involving the divisor function are:

which for d(n) = σ0(n) gives
and

A Lambert series involving the divisor function is:

for arbitrary complexComplex numberA complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
|q| ≤ 1 and a. This summation also appears as the Fourier series of the Eisenstein seriesEisenstein seriesEisenstein series, named after German mathematician Gotthold Eisenstein, are particular modular forms with infinite series expansions that may be written down directly...
and the invariants of the Weierstrass elliptic functions.
Approximate growth rate
In little-o notation, the divisor function satisfies the inequality (see page 296 of Apostol’s book)
More precisely, Severin Wigert showed that
On the other hand, since there are infinitely many prime numbers,
In Big-O notation, Dirichlet showed that the average orderAverage order of an arithmetic functionIn number theory, the average order of an arithmetic function is some simpler or better-understood function which takes the same values "on average".Let f be an arithmetic function...
of the divisor function satisfies the following inequality (see Theorem 3.3 of Apostol’s book)
where is Euler's constantEuler–Mascheroni constantThe Euler–Mascheroni constant is a mathematical constant recurring in analysis and number theory, usually denoted by the lowercase Greek letter ....
is Euler's constantEuler–Mascheroni constantThe Euler–Mascheroni constant is a mathematical constant recurring in analysis and number theory, usually denoted by the lowercase Greek letter ....
. Improving the bound in this formula is known as Dirichlet's divisor problem
in this formula is known as Dirichlet's divisor problem
The behaviour of the sigma function is irregular. The asymptotic growth rate of the sigma function can be expressed by:
where lim sup is the limit superior. This result is GrönwallThomas Hakon GrönwallThomas Hakon Grönwall or Thomas Hakon Gronwall was a Swedish mathematician. He studied at the University College of Stockholm and Uppsala University and completed his Ph.D. at Uppsala in 1898. Grönwall worked for about a year as a civil engineer in Germany before he immigrated to the United...
's theorem, published in 1913 . His proof uses Mertens' 3rd theoremMertens' theoremsIn number theory, Mertens' theorems are three 1874 results related to the density of prime numbers proved by Franz Mertens...
, which says that

where p denotes a prime.
In 1915, Ramanujan proved that under the assumption of the Riemann hypothesisRiemann hypothesisIn mathematics, the Riemann hypothesis, proposed by , is a conjecture about the location of the zeros of the Riemann zeta function which states that all non-trivial zeros have real part 1/2...
, the inequality: (Robin's inequality)
(Robin's inequality)
holds for all sufficiently large n. In 1984 Guy Robin proved that the inequality is true for all n ≥ 5,041 if and only if the Riemann hypothesis is true . This is Robin's theorem and the inequality became known after him. The largest known value that violates the inequality is n=5,040. If the Riemann hypothesis is true, there are no greater exceptions. If the hypothesis is false, then Robin showed there are an infinite number of values of n that violate the inequality, and it is known that the smallest such n ≥ 5,041 must be superabundant . It has been shown that the inequality holds for large odd and square-free integers, and that the Riemann hypothesis is equivalent to the inequality just for n divisible by the fifth power of a prime .
A related bound was given by Jeffrey LagariasJeffrey LagariasJeffrey Clark Lagarias is a mathematics professor at the University of Michigan.- Education :While in high school in 1966, Lagarias studied astronomy at the Summer Science Program....
in 2002, who proved that the Riemann hypothesis is equivalent to the statement that
for every natural numberNatural numberIn mathematics, the natural numbers are the ordinary whole numbers used for counting and ordering . These purposes are related to the linguistic notions of cardinal and ordinal numbers, respectively...
n, where is the nth harmonic number, .
is the nth harmonic number, .
Robin also proved, unconditionally, that the inequality
holds for all n ≥ 3.
See also
- Euler's totient functionEuler's totient functionIn number theory, the totient \varphi of a positive integer n is defined to be the number of positive integers less than or equal to n that are coprime to n In number theory, the totient \varphi(n) of a positive integer n is defined to be the number of positive integers less than or equal to n that...
(Euler's phi function) - Table of divisorsTable of divisorsThe tables below list all of the divisors of the numbers 1 to 1000.A divisor of an integer n is an integer m, say, for which n/m is again an integer . For example, 3 is a divisor of 21, since 21/3 = 7 .If m is a divisor of n then so is −m...
- Divisor sum convolutions Lists a few identities involving the divisor functions
-
-
-


