For a mathematically rigorous point of view of "discrete spectrum", see decomposition of spectrum (functional analysis)

Decomposition of spectrum (functional analysis)

In mathematics, especially functional analysis, the spectrum of an operator generalizes the notion of eigenvalues. Given an operator, it is sometimes useful to break up the spectrum into various parts...

.

In physics, an elementary (and accurate) explanation of a discrete spectrum is that it is an emission spectrum

Emission spectrum

The emission spectrum of a chemical element or chemical compound is the spectrum of frequencies of electromagnetic radiation emitted by the element's atoms or the compound's molecules when they are returned to a lower energy state....

 or absorption spectrum for which there is only an integer number (or countable number) of intensities. Atomic electronic absorption and emission spectrum are discrete, as contrasted with, for example, the emission spectrum of the sun, which is continuous. Discrete emission spectra of atoms are also referred to as emission line spectra, which show vertical lines at the frequencies (or wavelengths) of emission. To describe the difference between continuous and discrete spectra, we might refer to probability theory—measuring weight versus throwing dice.

Conceivably, our body-weights can be represented by any number, 50.00000 kilograms, 59.99999 kg, 39.999591 kg, the number of decimals determined by our equipment, not by any fundamental restriction on the value of a weight. By contrast, when we throw dice, the throw of a single six-sided die can yield only 1, 2, 3, 4, 5, or 6, and nothing in between; there is a fundamental restriction on the numbers we can get throwing a single die; we cannot get a 3.2, 4.5, or 1.7; we can only get whole numbers between 1 and 6 inclusive. The set of all possible weights is continuous; the set of all possible single-die tosses is discrete.

One segment of the emission spectrum of atomic hydrogen is shown below. Note that we see only four lines.
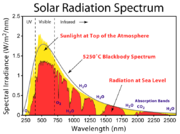
By contrast, the solar spectrum, the spectrum of electromagnetic frequencies emitted by the sun, is continuous; likewise, the Gaussian density function or the Bell Curve is continuous, showing not discrete lines but continuous curves.
When we can visualize discrete spectra and continuous spectra from experimental physics, we can better grasp, by analogy, the mathematical uses of these terms, for example, as used below.

In physics

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

, discrete spectrum is a finite set or a countable set

Countable set

In mathematics, a countable set is a set with the same cardinality as some subset of the set of natural numbers. A set that is not countable is called uncountable. The term was originated by Georg Cantor...

 of eigenvalues of an operator

Operator (physics)

In physics, an operator is a function acting on the space of physical states. As a resultof its application on a physical state, another physical state is obtained, very often along withsome extra relevant information....

. An operator acting on a Hilbert space

Hilbert space

The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...

 is said to have a discrete spectrum if its spectrum consists of isolated point

Isolated point

In topology, a branch of mathematics, a point x of a set S is called an isolated point of S, if there exists a neighborhood of x not containing other points of S.In particular, in a Euclidean space ,...

s. If the spectrum of an operator is not discrete, we say that it is a continuous spectrum

Continuous spectrum

The spectrum of a linear operator is commonly divided into three parts: point spectrum, continuous spectrum, and residual spectrum.If H is a topological vector space and A:H \to H is a linear map, the spectrum of A is the set of complex numbers \lambda such that A - \lambda I : H \to H is not...

.

The position

Position operator

In quantum mechanics, the position operator is the operator that corresponds to the position observable of a particle. Consider, for example, the case of a spinless particle moving on a line. The state space for such a particle is L2, the Hilbert space of complex-valued and square-integrable ...

 and the momentum operator

Momentum operator

In quantum mechanics, momentum is defined as an operator on the wave function. The Heisenberg uncertainty principle defines limits on how accurately the momentum and position of a single observable system can be known at once...

s (in an infinite space) have continuous spectra. But the momentum in a compact space

Compact space

In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...

, the angular momentum

Angular momentum

In physics, angular momentum, moment of momentum, or rotational momentum is a conserved vector quantity that can be used to describe the overall state of a physical system...

, and the Hamiltonian

Hamiltonian (quantum mechanics)

In quantum mechanics, the Hamiltonian H, also Ȟ or Ĥ, is the operator corresponding to the total energy of the system. Its spectrum is the set of possible outcomes when one measures the total energy of a system...

 of various physical systems (the corresponding eigenstates are called bound state

Bound state

In physics, a bound state describes a system where a particle is subject to a potential such that the particle has a tendency to remain localised in one or more regions of space...

) have a discrete (quantized) spectrum. This is a major difference with the corresponding operators in classical mechanics

Classical mechanics

In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...

. Quantum mechanics

Quantum mechanics

Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...

 was therefore named in this way.

The quantum harmonic oscillator

Quantum harmonic oscillator

The quantum harmonic oscillator is the quantum-mechanical analog of the classical harmonic oscillator. Because an arbitrary potential can be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics...

 or the Hydrogen atom

Hydrogen atom

A hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively-charged proton and a single negatively-charged electron bound to the nucleus by the Coulomb force...

 are examples of physical systems in which the Hamiltonian has a discrete spectrum. In the case of the Hydrogen atom, it has both continuous as well as discrete part of the spectrum; the continuous part represents the ion

Ion

An ion is an atom or molecule in which the total number of electrons is not equal to the total number of protons, giving it a net positive or negative electrical charge. The name was given by physicist Michael Faraday for the substances that allow a current to pass between electrodes in a...

ized atom.