
Atwood machine
Encyclopedia
George Atwood
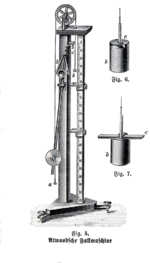
George Atwood was an English mathematician who invented a machine for illustrating the effects of Newton's first law of motion...
as a laboratory experiment to verify the mechanical laws of motion
Newton's laws of motion
Newton's laws of motion are three physical laws that form the basis for classical mechanics. They describe the relationship between the forces acting on a body and its motion due to those forces...
with constant acceleration
Acceleration
In physics, acceleration is the rate of change of velocity with time. In one dimension, acceleration is the rate at which something speeds up or slows down. However, since velocity is a vector, acceleration describes the rate of change of both the magnitude and the direction of velocity. ...
. Atwood's machine is a common classroom demonstration used to illustrate principles of classical mechanics
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
.
The ideal Atwood Machine consists of two objects of mass m1 and m2, connected by an inextensible massless string over an ideal massless pulley
Pulley
A pulley, also called a sheave or a drum, is a mechanism composed of a wheel on an axle or shaft that may have a groove between two flanges around its circumference. A rope, cable, belt, or chain usually runs over the wheel and inside the groove, if present...
.
When m1 = m2, the machine is in neutral equilibrium regardless of the position of the weights.
When m1 ≠ m2 both masses experience uniform acceleration.
Equation for constant acceleration
We are able to derive an equation for the acceleration by using force analysis.If we consider a massless, inextensible string and an ideal massless pulley, the only forces we have to consider are: tension force (T), and the weight of the two masses (W1 and W2). To find an acceleration we need to consider the forces affecting each individual mass.
Using Newton's second law (with a sign convention
Sign convention
In physics, a sign convention is a choice of the physical significance of signs for a set of quantities, in a case where the choice of sign is arbitrary. "Arbitrary" here means that the same physical system can be correctly described using different choices for the signs, as long as one set of...
of
 ) we can derive a system of equations
) we can derive a system of equationsSimultaneous equations
In mathematics, simultaneous equations are a set of equations containing multiple variables. This set is often referred to as a system of equations. A solution to a system of equations is a particular specification of the values of all variables that simultaneously satisfies all of the equations...
for the acceleration (a).
As a sign convention, we assume that a is positive when downward for
 , and that a is positive when upward for
, and that a is positive when upward for  . Weight of
. Weight of  and
and  is simply
is simply  and
and  respectively.
respectively.Forces affecting m1:

Forces affecting m2:

and adding the two previous equations we obtain
 ,
,and our concluding formula for acceleration

Conversely, the acceleration due to gravity, g, can be found by timing the movement of the weights, and calculating a value for the uniform acceleration a:
 .
.The Atwood machine is sometimes used to illustrate the
Lagrangian method
Lagrangian mechanics
Lagrangian mechanics is a re-formulation of classical mechanics that combines conservation of momentum with conservation of energy. It was introduced by the Italian-French mathematician Joseph-Louis Lagrange in 1788....
of deriving equations of motion.
Equation for tension
It can be useful to know an equation for the tension in the string. To evaluate tension we substitute the equation for acceleration in either of the 2 force equations.
For example substituting into
 , we get
, we get
The tension can be found in using this method.
Equations for a pulley with inertia and friction
For very small mass differences between m1 and m2, the rotational inertiaMoment of inertia
In classical mechanics, moment of inertia, also called mass moment of inertia, rotational inertia, polar moment of inertia of mass, or the angular mass, is a measure of an object's resistance to changes to its rotation. It is the inertia of a rotating body with respect to its rotation...
I of the pulley of radius r cannot be neglected. The angular acceleration of the pulley is given by the no-slip condition:

where
 is the angular acceleration. The net torque
is the angular acceleration. The net torqueTorque
Torque, moment or moment of force , is the tendency of a force to rotate an object about an axis, fulcrum, or pivot. Just as a force is a push or a pull, a torque can be thought of as a twist....
is then:

Combining with Newton's second law for the hanging masses, and solving for T1, T2, and a, we get:
Acceleration:

Tension in string segment nearest m1:

Tension in string segment nearest m2:

Should bearing friction be negligible (but not the inertia of the pulley and not the traction of the string on the pulley rim), these equations simplify as the following results:
Acceleration:

Tension in string segment nearest m1:

Tension in string segment nearest m2:

Practical implementations
Atwood's original illustrations show the main pulley's axle resting on the rims of another four wheels, to minimise friction forces from the bearingsRolling-element bearing
A rolling-element bearing, also known as a rolling bearing, is a bearing which carries a load by placing round elements between the two pieces...
. Many historical implementations of the machine follow this design.
An elevator with a counterbalance approximates an ideal Atwood machine and thereby relieves the driving motor from the load of holding the elevator cab — it has to overcome only weight difference and inertia of the two masses. The same principle is used for funicular
Funicular
A funicular, also known as an inclined plane or cliff railway, is a cable railway in which a cable attached to a pair of tram-like vehicles on rails moves them up and down a steep slope; the ascending and descending vehicles counterbalance each other.-Operation:The basic principle of funicular...
railways with two connected railway cars on inclined tracks.
See also
- Kater's pendulumKater's pendulumA Kater's pendulum is a reversible freeswinging pendulum invented by British physicist and army captain Henry Kater in 1817 for use as a gravimeter instrument to measure the local acceleration of gravity. Its advantage is that, unlike previous pendulum gravimetry methods, the pendulum's centre of...
- Swinging Atwood's machineSwinging Atwood's machineThe swinging Atwood's machine is a mechanism that resembles a simple Atwood's machine except that one of the masses is allowed to swing in a two-dimensional plane, producing a dynamical system that is chaotic for some system parameters and initial conditions.Specifically, it comprises two masses ...
- Professor Greenslade's account on the Atwood Machine
- Simplistic Atwood Machine Demonstration

