
Alexander horned sphere
Encyclopedia
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
in 3-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
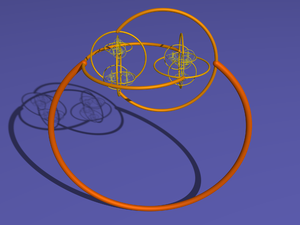
obtained by the following construction, starting with a standard torus:
- Remove a radial slice of the torus.
- Connect a standard punctured torus to each side of the cut, interlinked with the torus on the other side.
- Repeat steps 1–3 on the two tori just added ad infinitum.
By considering only the points of the tori that are not removed at some stage, an embedding results of the sphere with a Cantor set
Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of remarkable and deep properties. It was discovered in 1875 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883....
removed. This embedding extends to the whole sphere, since points approaching two different points of the Cantor set will be at least a fixed distance apart in the construction.
The horned sphere, together with its inside, is a topological 3-ball
Ball (mathematics)
In mathematics, a ball is the space inside a sphere. It may be a closed ball or an open ball ....
, the Alexander horned ball, and so is simply connected; i.e., every loop can be shrunk to a point while staying inside. The exterior is not simply connected, unlike the exterior of the usual round sphere; a loop linking a torus in the above construction cannot be shrunk to a point without touching the horned sphere. This shows that the Jordan–Schönflies theorem does not hold in three dimensions, as Alexander had originally thought. Alexander also proved that the theorem does hold in three dimensions for piecewise linear
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
/smooth
Differentiable manifold
A differentiable manifold is a type of manifold that is locally similar enough to a linear space to allow one to do calculus. Any manifold can be described by a collection of charts, also known as an atlas. One may then apply ideas from calculus while working within the individual charts, since...
embeddings. This is one of the earliest examples where the need for distinction between the topological category
Category (mathematics)
In mathematics, a category is an algebraic structure that comprises "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose...
of manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
s, and the categories of differentiable manifold
Differentiable manifold
A differentiable manifold is a type of manifold that is locally similar enough to a linear space to allow one to do calculus. Any manifold can be described by a collection of charts, also known as an atlas. One may then apply ideas from calculus while working within the individual charts, since...
s, and piecewise linear manifold
Piecewise linear manifold
In mathematics, a piecewise linear manifold is a topological manifold together with a piecewise linear structure on it. Such a structure can be defined by means of an atlas, such that one can pass from chart to chart in it by piecewise linear functions.An isomorphism of PL manifolds is called a PL...
s was noticed.
Now consider Alexander's horned sphere as an embedding
Embedding
In mathematics, an embedding is one instance of some mathematical structure contained within another instance, such as a group that is a subgroup....
into the 3-sphere
3-sphere
In mathematics, a 3-sphere is a higher-dimensional analogue of a sphere. It consists of the set of points equidistant from a fixed central point in 4-dimensional Euclidean space...
, considered as the one-point compactification of the 3-dimensional Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
R3. The closure
Closure (topology)
In mathematics, the closure of a subset S in a topological space consists of all points in S plus the limit points of S. Intuitively, these are all the points that are "near" S. A point which is in the closure of S is a point of closure of S...
of the non-simply connected domain is called the solid Alexander horned sphere. Although the solid horned sphere is not a manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
, R. H. Bing showed that its double
Double (manifold)
In the subject of manifold theory in mathematics, if M is a manifold with boundary, its double is obtained by gluing two copies of M together along their common boundary...
(which is the 3-manifold obtained by gluing two copies of the horned sphere together along the corresponding points of their boundaries) is in fact the 3-sphere. One can consider other gluings of the solid horned sphere to a copy of itself, arising from different homeomorphisms of the boundary sphere to itself. This has also been shown to be the 3-sphere. The solid Alexander horned sphere is an example of a crumpled cube
Crumpled cube
In geometric topology, a branch of mathematics, a crumpled cube is a 2-sphere in R3 together with its interior. showed that the union of a crumpled cube and a 3-ball glued along their boundaries is a 3-sphere....
; i.e., a closed complementary domain of the embedding of a 2-sphere into the 3-sphere.
One can generalize Alexander's construction to generate other horned spheres by increasing the number of horns at each stage of Alexander's construction or considering the analogous construction in higher dimensions.
Other substantially different constructions exist for constructing such "wild" spheres. Another example, also found by Alexander, is Antoine's horned sphere, which is based on Antoine's necklace
Antoine's necklace
In mathematics, Antoine's necklace, discovered by , is an embedding of the Cantor set in 3-dimensional Euclidean space whose complement is not simply connected....
, a pathological embedding of the Cantor set
Cantor set
In mathematics, the Cantor set is a set of points lying on a single line segment that has a number of remarkable and deep properties. It was discovered in 1875 by Henry John Stephen Smith and introduced by German mathematician Georg Cantor in 1883....
into the 3-sphere.
External links
- Zbigniew Fiedorowicz. Math 655 – Introduction to Topology. http://www.math.ohio-state.edu/~fiedorow/math655/ – Lecture notes
- Construction of the Alexander sphere
- rotating animation
- PC OpenGL demo rendering and expanding the cusp

