Stability of the Solar System
Encyclopedia
The stability of the Solar System is a subject of much inquiry in astronomy
. Though the planets have been stable historically, and will be in the short term, their weak gravitational effects on one another can add up in unpredictable ways.
For this reason (among others) the Solar System
is chaotic
, and even the most precise long-term models for the orbital motion of the Solar System are not valid over more than a few tens of millions of years.
The solar system is stable in human terms, in that none of the planets will collide with each other or be ejected from the system in the next few billion years, and the Earth's orbit
will be relatively stable.
Since Newton's law of gravitation
(1687), mathematicians and astronomers (such as Laplace
, Lagrange
, Gauss
, Poincaré
, Kolmogorov
, Vladimir Arnold
and Jürgen Moser
) have searched for evidence for the stability of the planetary motions, and this quest led to many mathematical developments, and several successive 'proofs' of stability for the solar system.
.
 Resonance happens when any two periods have a simple numerical ratio. The most fundamental period for an object in the Solar System is its orbital period
Resonance happens when any two periods have a simple numerical ratio. The most fundamental period for an object in the Solar System is its orbital period
, and orbital resonances pervade the solar system. In 1867, the American astronomer Daniel Kirkwood
noticed that asteroids in the Asteroid belt
are not randomly distributed. There were distinct gaps in the belt, and at locations that corresponded to resonances with Jupiter. For example, there were no asteroids at the 3:1 resonance – a distance of 2.5 astronomical units – or at the 2:1 resonance at 3.3 astronomical units.
Another common form of resonance in the Solar System is spin-orbit resonance, where the period of spin (the time it takes the orbit to rotate once about its axis) has a simple numerical relationship with its orbital period. An example is our own Moon
, which is in a 1:1 spin-orbit resonance that keeps the far side of the moon
away from the Earth
.
in the range of 2–230 million years. In all cases this means that the position of a planet along its orbit ultimately becomes impossible to predict with any certainty (so, for example, the timing of winter and summer become uncertain), but in some cases the orbits themselves may change dramatically. Such chaos manifests most strongly as changes in eccentricity
, with some planets' orbits becoming significantly more—or less—elliptical
.
In calculation, the unknowns include asteroid
s, the solar quadrupole moment, mass loss from the Sun
through radiation and solar wind
, and drag of solar wind on planetary magnetosphere
s, galactic tidal forces, the fractional effect, and effects from passing star
s.
Furthermore, the equations of motion describe a process that is inherently serial
, so there is little gain from massively parallel computers.
–Pluto
system lies in a 3:2 orbital resonance
. C. J. Cohen and E. C. Hubbard at the Naval Surface Warfare Center Dahlgren Division
discovered this in 1965. Although the resonance itself will remain stable in the short term, it becomes impossible to predict the position of Pluto with any degree of accuracy more than 10–20 million years (the Lyapunov time
) into the future.
has an orbital period of 1.769 days, nearly half that of the next satellite Europa
(3.551 days). They are said to be in a 2:1 orbit-orbit resonance. This particular resonance has important consequences because Europa's gravity perturbs
the orbit of Io. As Io moves closer to Jupiter and then further away in the course of a orbit, it experiences significant tidal stresses resulting in active volcanoes, which Voyager
observed.
Europa is also in a 2:1 resonance with the next satellite Ganymede
.
is especially susceptible to Jupiter
's influence because of a small celestial coincidence: Mercury's perihelion, the point where it gets closest to the sun, moves around at a rate of about 1.5 degrees every 1000 years, and Jupiter's perihelion moves around only a little slower. One day, the two may fall into sync, at which time Jupiter's constant gravitational tugs could accumulate and pull Mercury off course.
This could eject it from the Solar System altogether or send it on a collision course with Venus
or Earth.
which, thanks to friction raised within Earth's mantle
by tidal interactions with the Moon
(see below), will be rendered chaotic at some point between 1.5 and 4.5 billion years from now.
. It involved creation of a model on a supercomputer, integrating the orbits of (only) the outer planets. Its results revealed several curious exchanges of energy between the outer planets, but no signs of gross instability.
with Neptune
.
If Pluto's orbit is chaotic, then technically the whole Solar System is chaotic, because each planet, even one as small as Pluto, affects the others to some extent through gravitational interactions.
in Paris published the results of his numerical integration of the Solar System over 200 million years. These were not the full equations of motion, but rather averaged equations along the lines of those used by Laplace
. Laskar's work showed that the Earth's orbit (as well as the orbits of all the inner planets) is chaotic and that an error as small as 15 metres in measuring the position of the Earth today would make it impossible to predict where the Earth would be in its orbit in just over 100 million years' time.
Astronomy
Astronomy is a natural science that deals with the study of celestial objects and phenomena that originate outside the atmosphere of Earth...
. Though the planets have been stable historically, and will be in the short term, their weak gravitational effects on one another can add up in unpredictable ways.
For this reason (among others) the Solar System
Solar System
The Solar System consists of the Sun and the astronomical objects gravitationally bound in orbit around it, all of which formed from the collapse of a giant molecular cloud approximately 4.6 billion years ago. The vast majority of the system's mass is in the Sun...
is chaotic
Chaos theory
Chaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
, and even the most precise long-term models for the orbital motion of the Solar System are not valid over more than a few tens of millions of years.
The solar system is stable in human terms, in that none of the planets will collide with each other or be ejected from the system in the next few billion years, and the Earth's orbit
Earth's orbit
In astronomy, the Earth's orbit is the motion of the Earth around the Sun, at an average distance of about 150 million kilometers, every 365.256363 mean solar days .A solar day is on average 24 hours; it takes 365.256363 of these to orbit the sun once in the sense of returning...
will be relatively stable.
Since Newton's law of gravitation
Newton's law of universal gravitation
Newton's law of universal gravitation states that every point mass in the universe attracts every other point mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them...
(1687), mathematicians and astronomers (such as Laplace
Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace was a French mathematician and astronomer whose work was pivotal to the development of mathematical astronomy and statistics. He summarized and extended the work of his predecessors in his five volume Mécanique Céleste...
, Lagrange
Joseph Louis Lagrange
Joseph-Louis Lagrange , born Giuseppe Lodovico Lagrangia, was a mathematician and astronomer, who was born in Turin, Piedmont, lived part of his life in Prussia and part in France, making significant contributions to all fields of analysis, to number theory, and to classical and celestial mechanics...
, Gauss
Carl Friedrich Gauss
Johann Carl Friedrich Gauss was a German mathematician and scientist who contributed significantly to many fields, including number theory, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy and optics.Sometimes referred to as the Princeps mathematicorum...
, Poincaré
Henri Poincaré
Jules Henri Poincaré was a French mathematician, theoretical physicist, engineer, and a philosopher of science...
, Kolmogorov
Andrey Kolmogorov
Andrey Nikolaevich Kolmogorov was a Soviet mathematician, preeminent in the 20th century, who advanced various scientific fields, among them probability theory, topology, intuitionistic logic, turbulence, classical mechanics and computational complexity.-Early life:Kolmogorov was born at Tambov...
, Vladimir Arnold
Vladimir Arnold
Vladimir Igorevich Arnold was a Soviet and Russian mathematician. While he is best known for the Kolmogorov–Arnold–Moser theorem regarding the stability of integrable Hamiltonian systems, he made important contributions in several areas including dynamical systems theory, catastrophe theory,...
and Jürgen Moser
Jürgen Moser
Jürgen Kurt Moser or Juergen Kurt Moser was a German-American mathematician.-Professional biography:...
) have searched for evidence for the stability of the planetary motions, and this quest led to many mathematical developments, and several successive 'proofs' of stability for the solar system.
Overview and challenges
The orbits of the planets are open to long-term variations, and modeling the solar system is subject to the n-body problemN-body problem
The n-body problem is the problem of predicting the motion of a group of celestial objects that interact with each other gravitationally. Solving this problem has been motivated by the need to understand the motion of the Sun, planets and the visible stars...
.
Resonance

Orbital period
The orbital period is the time taken for a given object to make one complete orbit about another object.When mentioned without further qualification in astronomy this refers to the sidereal period of an astronomical object, which is calculated with respect to the stars.There are several kinds of...
, and orbital resonances pervade the solar system. In 1867, the American astronomer Daniel Kirkwood
Daniel Kirkwood
Daniel Kirkwood was an American astronomer.Born in Harford County, Maryland, he was graduated in mathematics from the York County Academy in York, Pennsylvania in 1838...
noticed that asteroids in the Asteroid belt
Asteroid belt
The asteroid belt is the region of the Solar System located roughly between the orbits of the planets Mars and Jupiter. It is occupied by numerous irregularly shaped bodies called asteroids or minor planets...
are not randomly distributed. There were distinct gaps in the belt, and at locations that corresponded to resonances with Jupiter. For example, there were no asteroids at the 3:1 resonance – a distance of 2.5 astronomical units – or at the 2:1 resonance at 3.3 astronomical units.
Another common form of resonance in the Solar System is spin-orbit resonance, where the period of spin (the time it takes the orbit to rotate once about its axis) has a simple numerical relationship with its orbital period. An example is our own Moon
Moon
The Moon is Earth's only known natural satellite,There are a number of near-Earth asteroids including 3753 Cruithne that are co-orbital with Earth: their orbits bring them close to Earth for periods of time but then alter in the long term . These are quasi-satellites and not true moons. For more...
, which is in a 1:1 spin-orbit resonance that keeps the far side of the moon
Far side of the Moon
The far side of the Moon is the lunar hemisphere that is permanently turned away, and is not visible from the surface of the Earth. The far hemisphere was first photographed by the Soviet Luna 3 probe in 1959, and was first directly observed by human eyes when the Apollo 8 mission orbited the Moon...
away from the Earth
Earth
Earth is the third planet from the Sun, and the densest and fifth-largest of the eight planets in the Solar System. It is also the largest of the Solar System's four terrestrial planets...
.
Predictability
The planets' orbits are chaotic over longer timescales, such that the whole Solar System possesses a Lyapunov timeLyapunov time
In mathematics, the Lyapunov time is the length of time for a dynamical system to become chaotic. The Lyapunov time reflects the limits of the predictability of the system. By convention, it is defined as the time for the distance between nearby trajectories of the system to increase by a factor...
in the range of 2–230 million years. In all cases this means that the position of a planet along its orbit ultimately becomes impossible to predict with any certainty (so, for example, the timing of winter and summer become uncertain), but in some cases the orbits themselves may change dramatically. Such chaos manifests most strongly as changes in eccentricity
Orbital eccentricity
The orbital eccentricity of an astronomical body is the amount by which its orbit deviates from a perfect circle, where 0 is perfectly circular, and 1.0 is a parabola, and no longer a closed orbit...
, with some planets' orbits becoming significantly more—or less—elliptical
Ellipse
In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...
.
In calculation, the unknowns include asteroid
Asteroid
Asteroids are a class of small Solar System bodies in orbit around the Sun. They have also been called planetoids, especially the larger ones...
s, the solar quadrupole moment, mass loss from the Sun
Sun
The Sun is the star at the center of the Solar System. It is almost perfectly spherical and consists of hot plasma interwoven with magnetic fields...
through radiation and solar wind
Solar wind
The solar wind is a stream of charged particles ejected from the upper atmosphere of the Sun. It mostly consists of electrons and protons with energies usually between 1.5 and 10 keV. The stream of particles varies in temperature and speed over time...
, and drag of solar wind on planetary magnetosphere
Magnetosphere
A magnetosphere is formed when a stream of charged particles, such as the solar wind, interacts with and is deflected by the intrinsic magnetic field of a planet or similar body. Earth is surrounded by a magnetosphere, as are the other planets with intrinsic magnetic fields: Mercury, Jupiter,...
s, galactic tidal forces, the fractional effect, and effects from passing star
Star
A star is a massive, luminous sphere of plasma held together by gravity. At the end of its lifetime, a star can also contain a proportion of degenerate matter. The nearest star to Earth is the Sun, which is the source of most of the energy on Earth...
s.
Furthermore, the equations of motion describe a process that is inherently serial
Single threading
In computer programming, single threading is the processing of one command at a time. The opposite of single threading is multithreading. While it has been suggested that the term single threading is misleading, the term has been widely accepted within the functional programming community....
, so there is little gain from massively parallel computers.
Neptune–Pluto resonance
The NeptuneNeptune
Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times...
–Pluto
Pluto
Pluto, formal designation 134340 Pluto, is the second-most-massive known dwarf planet in the Solar System and the tenth-most-massive body observed directly orbiting the Sun...
system lies in a 3:2 orbital resonance
Orbital resonance
In celestial mechanics, an orbital resonance occurs when two orbiting bodies exert a regular, periodic gravitational influence on each other, usually due to their orbital periods being related by a ratio of two small integers. Orbital resonances greatly enhance the mutual gravitational influence of...
. C. J. Cohen and E. C. Hubbard at the Naval Surface Warfare Center Dahlgren Division
Naval Surface Warfare Center Dahlgren Division
The United States Naval Surface Warfare Center, Dahlgren Division , named for Rear Admiral John A. Dahlgren, is located in Dahlgren, Virginia and is part of the Naval Surface Warfare Center. The NSWCDD was founded as the U.S...
discovered this in 1965. Although the resonance itself will remain stable in the short term, it becomes impossible to predict the position of Pluto with any degree of accuracy more than 10–20 million years (the Lyapunov time
Lyapunov time
In mathematics, the Lyapunov time is the length of time for a dynamical system to become chaotic. The Lyapunov time reflects the limits of the predictability of the system. By convention, it is defined as the time for the distance between nearby trajectories of the system to increase by a factor...
) into the future.
Jovian moon resonance
Jupiter's moon IoIo (moon)
Io ) is the innermost of the four Galilean moons of the planet Jupiter and, with a diameter of , the fourth-largest moon in the Solar System. It was named after the mythological character of Io, a priestess of Hera who became one of the lovers of Zeus....
has an orbital period of 1.769 days, nearly half that of the next satellite Europa
Europa (moon)
Europa Slightly smaller than Earth's Moon, Europa is primarily made of silicate rock and probably has an iron core. It has a tenuous atmosphere composed primarily of oxygen. Its surface is composed of ice and is one of the smoothest in the Solar System. This surface is striated by cracks and...
(3.551 days). They are said to be in a 2:1 orbit-orbit resonance. This particular resonance has important consequences because Europa's gravity perturbs
Perturbation
Perturbation or perturb may refer to any of numerous concepts in several fields:* Perturbation theory, mathematical methods that give approximate solutions to problems that cannot be solved exactly...
the orbit of Io. As Io moves closer to Jupiter and then further away in the course of a orbit, it experiences significant tidal stresses resulting in active volcanoes, which Voyager
Voyager
-Technology:*LG Voyager, a mobile phone model manufactured by LG Electronics*NCR Voyager, a computer platform produced by NCR Corporation*Voyager , a computer worm affecting Oracle databases...
observed.
Europa is also in a 2:1 resonance with the next satellite Ganymede
Ganymede (moon)
Ganymede is a satellite of Jupiter and the largest moon in the Solar System. It is the seventh moon and third Galilean satellite outward from Jupiter. Completing an orbit in roughly seven days, Ganymede participates in a 1:2:4 orbital resonance with the moons Europa and Io, respectively...
.
Mercury–Jupiter 1:1 resonance
The planet MercuryMercury (planet)
Mercury is the innermost and smallest planet in the Solar System, orbiting the Sun once every 87.969 Earth days. The orbit of Mercury has the highest eccentricity of all the Solar System planets, and it has the smallest axial tilt. It completes three rotations about its axis for every two orbits...
is especially susceptible to Jupiter
Jupiter
Jupiter is the fifth planet from the Sun and the largest planet within the Solar System. It is a gas giant with mass one-thousandth that of the Sun but is two and a half times the mass of all the other planets in our Solar System combined. Jupiter is classified as a gas giant along with Saturn,...
's influence because of a small celestial coincidence: Mercury's perihelion, the point where it gets closest to the sun, moves around at a rate of about 1.5 degrees every 1000 years, and Jupiter's perihelion moves around only a little slower. One day, the two may fall into sync, at which time Jupiter's constant gravitational tugs could accumulate and pull Mercury off course.
This could eject it from the Solar System altogether or send it on a collision course with Venus
Venus
Venus is the second planet from the Sun, orbiting it every 224.7 Earth days. The planet is named after Venus, the Roman goddess of love and beauty. After the Moon, it is the brightest natural object in the night sky, reaching an apparent magnitude of −4.6, bright enough to cast shadows...
or Earth.
Chaos from geological processes
Another example is Earth's axial tiltAxial tilt
In astronomy, axial tilt is the angle between an object's rotational axis, and a line perpendicular to its orbital plane...
which, thanks to friction raised within Earth's mantle
Mantle (geology)
The mantle is a part of a terrestrial planet or other rocky body large enough to have differentiation by density. The interior of the Earth, similar to the other terrestrial planets, is chemically divided into layers. The mantle is a highly viscous layer between the crust and the outer core....
by tidal interactions with the Moon
Moon
The Moon is Earth's only known natural satellite,There are a number of near-Earth asteroids including 3753 Cruithne that are co-orbital with Earth: their orbits bring them close to Earth for periods of time but then alter in the long term . These are quasi-satellites and not true moons. For more...
(see below), will be rendered chaotic at some point between 1.5 and 4.5 billion years from now.
LONGSTOP
Project LONGSTOP (Long-term Gravitational Study of the Outer Planets) was a 1982 international consortium of Solar System dynamicists led by Archie RoyArchie Roy
- Career :Professor Archie Edminston Roy , was educated at Hillhead High School and the University of Glasgow. He is married to Frances with three sons; Dr. Archie W N Roy, Ian Roy and David Roy....
. It involved creation of a model on a supercomputer, integrating the orbits of (only) the outer planets. Its results revealed several curious exchanges of energy between the outer planets, but no signs of gross instability.
Digital Orrery
Another project involved constructing the Digital Orrery by Gerry Sussman and his MIT group in 1988. The group used a supercomputer to integrate the orbits of the outer planets over 845 million years (some 20 per cent of the age of the Solar System). In 1988, Sussman and Wisdom found data using the Orrery which revealed that Pluto's orbit shows signs of chaos, due in part to its peculiar resonanceResonance
In physics, resonance is the tendency of a system to oscillate at a greater amplitude at some frequencies than at others. These are known as the system's resonant frequencies...
with Neptune
Neptune
Neptune is the eighth and farthest planet from the Sun in the Solar System. Named for the Roman god of the sea, it is the fourth-largest planet by diameter and the third largest by mass. Neptune is 17 times the mass of Earth and is slightly more massive than its near-twin Uranus, which is 15 times...
.
If Pluto's orbit is chaotic, then technically the whole Solar System is chaotic, because each planet, even one as small as Pluto, affects the others to some extent through gravitational interactions.
Laskar #1
In 1989, Jacques Laskar of the Bureau des LongitudesBureau des Longitudes
The Bureau des Longitudes is a French scientific institution, founded by decree of 25 June 1795 and charged with the improvement of nautical navigation, standardisation of time-keeping, geodesy and astronomical observation. During the 19th century, it was responsible for synchronizing clocks...
in Paris published the results of his numerical integration of the Solar System over 200 million years. These were not the full equations of motion, but rather averaged equations along the lines of those used by Laplace
Pierre-Simon Laplace
Pierre-Simon, marquis de Laplace was a French mathematician and astronomer whose work was pivotal to the development of mathematical astronomy and statistics. He summarized and extended the work of his predecessors in his five volume Mécanique Céleste...
. Laskar's work showed that the Earth's orbit (as well as the orbits of all the inner planets) is chaotic and that an error as small as 15 metres in measuring the position of the Earth today would make it impossible to predict where the Earth would be in its orbit in just over 100 million years' time.
Laskar & Gastineau
Jacques Laskar and his colleague Mickaël Gastineau in 2009 took a more thorough approach by directly simulating 2500 possible futures. Each of the 2500 cases has slightly different initial conditions: Mercury's position varies by about 1 metre between one simulation and the next. In 20 cases, Mercury goes into a dangerous orbit and often ends up colliding with Venus or plunging into the sun. Moving in such a warped orbit, Mercury's gravity is more likely to shake other planets out of their settled paths: in one simulated case its perturbations send Mars heading towards Earth.See also
- Clearing the neighbourhoodClearing the neighbourhood"Clearing the neighbourhood of its orbit" is a criterion for a celestial body to be considered a planet in the Solar System. This was one of the three criteria adopted by the International Astronomical Union in its 2006 definition of planet....
- Future of the EarthFuture of the EarthThe biological and geological future of the Earth can be extrapolated based upon the estimated effects of several long-term influences. These include the chemistry at the Earth's surface, the rate of cooling of the planet's interior, the gravitational interactions with other objects in the Solar...
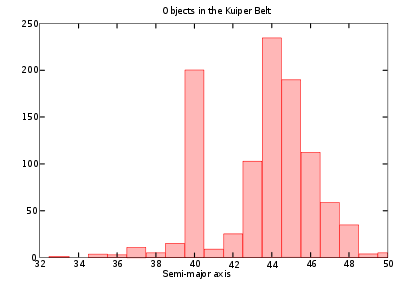
- Resonant trans-Neptunian objectResonant trans-Neptunian objectIn astronomy, a resonant trans-Neptunian object is a trans-Neptunian object in mean motion orbital resonance with Neptune. The orbital periods of the resonant objects are in a simple integer relations with the period of Neptune e.g. 1:2, 2:3 etc...
- Risks to civilization, humans and planet EarthRisks to civilization, humans and planet EarthVarious existential risks could threaten humankind as a whole, have adverse consequences for the course of human civilization, or even cause the end of planet Earth.-Types of risks:...

