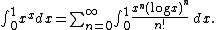Sophomore's dream
Encyclopedia
In mathematics, sophomore's dream is a name occasionally used for the identities
(especially the first)

discovered in 1697 by Johann Bernoulli
.
The name "sophomore's dream", which appears in , is in contrast to the name "freshman's dream
" which is given to the incorrectIncorrect unless one is working over a field
or unital commutative ring
of prime characteristic
n. The correct result is given by the binomial theorem
. equation (x + y)n = xn + yn. The sophomore
's dream has a similar too-good-to-be-true feel, but is in fact true.
 We prove the second identity; the first is completely analogous.
We prove the second identity; the first is completely analogous.
The key ingredients of the proof are:
Expand xx as
Thus by termwise integration,
To evaluate the above integrals we perform the change of variable in the integral
 , with
, with  , so the integral
, so the integral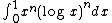
writes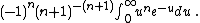
By the well-known Euler's integral identity for the Gamma function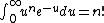
so that: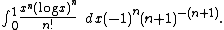
Summing these (and changing indexing so it starts at n = 1
instead of n = 0) yields the formula.
Identity (mathematics)
In mathematics, the term identity has several different important meanings:*An identity is a relation which is tautologically true. This means that whatever the number or value may be, the answer stays the same. For example, algebraically, this occurs if an equation is satisfied for all values of...
(especially the first)

discovered in 1697 by Johann Bernoulli
Johann Bernoulli
Johann Bernoulli was a Swiss mathematician and was one of the many prominent mathematicians in the Bernoulli family...
.
The name "sophomore's dream", which appears in , is in contrast to the name "freshman's dream
Freshman's dream
The freshman's dream is a name sometimes given to the error n = xn + yn, where n is a real number . Beginning students commonly make this error in computing the exponential of a sum of real numbers...
" which is given to the incorrectIncorrect unless one is working over a field
Field (mathematics)
In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
or unital commutative ring
Commutative ring
In ring theory, a branch of abstract algebra, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra....
of prime characteristic
Characteristic (algebra)
In mathematics, the characteristic of a ring R, often denoted char, is defined to be the smallest number of times one must use the ring's multiplicative identity element in a sum to get the additive identity element ; the ring is said to have characteristic zero if this repeated sum never reaches...
n. The correct result is given by the binomial theorem
Binomial theorem
In elementary algebra, the binomial theorem describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the power n into a sum involving terms of the form axbyc, where the exponents b and c are nonnegative integers with , and the coefficient a of...
. equation (x + y)n = xn + yn. The sophomore
Sophomore
Sophomore is a term used in the United States to describe a student in the second year of study at high school or university.The word is also used as a synonym for "second", for the second album or EP released by a musician or group, the second movie of a director, or the second season of a...
's dream has a similar too-good-to-be-true feel, but is in fact true.
Proof

The key ingredients of the proof are:
- Write xx = exp(x log x).
- Expand exp(x log x) using the power series for exp.
- Integrate termwise.
- Integrate by substitutionIntegration by substitutionIn calculus, integration by substitution is a method for finding antiderivatives and integrals. Using the fundamental theorem of calculus often requires finding an antiderivative. For this and other reasons, integration by substitution is an important tool for mathematicians...
.
Expand xx as
Thus by termwise integration,
To evaluate the above integrals we perform the change of variable in the integral
Integration by substitution
In calculus, integration by substitution is a method for finding antiderivatives and integrals. Using the fundamental theorem of calculus often requires finding an antiderivative. For this and other reasons, integration by substitution is an important tool for mathematicians...
 , with
, with  , so the integral
, so the integral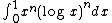
writes
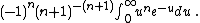
By the well-known Euler's integral identity for the Gamma function
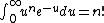
so that:
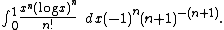
Summing these (and changing indexing so it starts at n = 1
instead of n = 0) yields the formula.
Formula
- Jonathan BorweinJonathan BorweinJonathan Michael Borwein is a Scottish mathematician who holds an appointment as Laureate Professor of mathematics at the University of Newcastle, Australia. Noted for his prolific and creative work throughout the international mathematical community, he is a close associate of David H...
, David H. BaileyDavid H. BaileyDavid Harold Bailey is a mathematician and computer scientist. He received his B.S. in mathematics from Brigham Young University in 1972 and his Ph.D. in mathematics from Stanford University in 1976...
, Roland Girgensohn Experimentation in Mathematics: Computational Paths to Discovery 2004, Page 44. - William Dunham, The Calculus Gallery, Masterpieces from Newton to Lebesgue, Princeton University Press, Princeton, NJ 2005, p. 46-51.
- OEIS, and
- Pólya and Gábor SzegőGábor SzegoGábor Szegő was a Hungarian mathematician. He was one of the foremost analysts of his generation and made fundamental contributions to the theory of Toeplitz matrices and orthogonal polynomials.-Life:...
, Problems and Theorems in Analysis (part I, problem 160; 1998, p. 36) - Weisstein, Eric W.Eric W. WeissteinEric W. Weisstein is an encyclopedist who created and maintains MathWorld and Eric Weisstein's World of Science . He currently works for Wolfram Research, Inc.-Education:...
Sophomore's Dream. From MathWorld—A Wolfram Web Resource.
Function
- Literature for x^x and Sophomore's Dream, Tetration Forum, 03/02/2010
- The Coupled Exponential, Jay A. Fantini, Gilbert C. Kloepfer, 1998
- Sophomore's Dream Function, Jean Jacquelin, 1998, 13 pp.