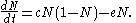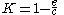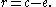Metapopulation
Encyclopedia
A metapopulation consists of a group of spatially separated population
s of the same species
which interact at some level. The term metapopulation was coined by Richard Levins
in 1970 to describe a model of population dynamics of insect pests in agricultural fields, but the idea has been most broadly applied to species in naturally or artificially fragmented habitats
. In Levins' own words, it consists of "a population of populations".
A metapopulation is generally considered to consist of several distinct populations together with areas of suitable habitat which are currently unoccupied. In classical metapopulation theory, each population cycles in relative independence of the other populations and eventually goes extinct as a consequence of demographic stochastic
ity (fluctuations in population size due to random demographic events); the smaller the population, the more prone it is to extinction.
Although individual populations have finite life-spans, the metapopulation as a whole is often stable because immigrants from one population (which may, for example, be experiencing a population boom) are likely to re-colonize habitat which has been left open by the extinction of another population. They may also emigrate to a small population and rescue that population from extinction (called the rescue effect).
The development of metapopulation theory, in conjunction with the development of source-sink dynamics
, emphasised the importance of connectivity between seemingly isolated populations. Although no single population may be able to guarantee the long-term survival of a given species, the combined effect of many populations may be able to do this.
Metapopulation theory was first developed for terrestrial ecosystems, and subsequently applied to the marine realm. In fisheries science, the term "sub-population" is equivalent to the metapopulation science term "local population". Most marine examples are provided by relatively sedentary species occupying discrete patches of habitat, with both local recruitment and recruitment from other local populations in the larger metapopulation. Kritzer & Sale have argued against strict application of the metapopulation definitional criteria that extinction risks to local populations must be non-negligible.
An important contributor to metapopulation theory is the Finnish
biologist, Ilkka Hanski
http://www.helsinki.fi/~ihanski/, of the University of Helsinki
.
and spatial heterogeneity
were conducted by G.F. Gause in the 1930s, based on the Lotka-Volterra equation
, which was formulated in the mid-1920s, but no further application had been conducted. The Lotka-Volterra equation suggested that the relationship between predators and their prey would result in population oscillations over time based on the initial densities of predator and prey. Gause's early experiments to prove the predicted oscillations of this theory failed because the predator-prey interactions were not influenced by immigration. However, once immigration was introduced, the population cycles accurately depicted the oscillations predicted by the Lotka-Volterra equation, with the peaks in prey abundance shifted slightly to the left of the peaks of the predator densities. Huffaker's experiments expanded on those of Gause by examining how both the factors of migration and spatial heterogeneity lead to predator-prey oscillations.
and habitat patchiness would later describe the conditions of a metapopulation relating to how groups of spatially separated populations of species interact with one another. Huffaker's experiment is significant because it showed how metapopulations can directly affect the predator-prey interactions and in turn influence population dynamics.
In spatially structured landscapes, species populations exist as metapopulations,
which consist of subpopulations each of which inhabits a habitat patch
Agglomeration payment
If individual members of species can move between patches, this is beneficial
for survival of metapopulation because it allows recolonization of patches
Let N be the fraction of patches occupied at a given time. During a time dt, each occupied patch can become unoccupied with an extinction probability edt. Additionally, 1 − N of the patches are unoccupied. Assuming a constant rate c of propagule
generation from each of the N occupied patches, during a time dt, each unoccupied patch can become occupied with a colonization probability cNdt . Accordingly, the time rate of change of occupied patches, dN/dt, is
This equation is mathematically equivalent to the logistic model, with a carrying capacity K given by
and growth rate r
At equilibrium, therefore, some fraction of the species's habitat will always be unoccupied.
. Metapopulations are particularly useful when discussing species in disturbed habitats, and the viability of their populations
, i.e., how likely they are to become extinct in a given time interval. The Levins model cannot address this issue. A simple way to extend the Levins' model to incorporate space and stochastic considerations is by using the contact process (mathematics). Simple modifications to this model can also incorporate for patch dynamics
. At a given percolation threshold
, habitat fragmentation
effects take place in these configuration predicting more drastic extinction thresholds.
For conservation biology
purposes, metapopulation models must include (a) the finite nature of metapopulations (how many patches are suitable for habitat), and (b) the probabilistic
nature of extinction and colonisation. Also, note that in order to apply these models, the extinctions and colonisations of the patches must be asynchronous.
with landscape ecology
, a habitat landscape can be nanofabricated on-chip by building a collection of nanofabricated bacterial habitats, and connecting them by corridors in different topological arrangements and with nano-scale channels providing them with the local ecosystem service of habitat renewal. These landscapes of MHPs can be used as physical implementations of an adaptive landscape : by generating a spatial mosaic of patches of opportunity distributed in space and time. The patchy nature of these fluidic landscapes allows for the study of adapting bacterial cells in a metapopulation system operating on-chip within a synthetic ecosystem. The metapopulation biology and evolutionary ecology
of these bacterial systems, in these synthetic ecosystems, can be addressed using experimental biophysics
.
of amphibian metamorphosis in small vernal ponds. Alternative ecological strategies have evolved. For example, some salamanders forgo metamorphosis and sexually mature as aquatic neotenes
. The seasonal duration of wetlands and the migratory range of the species determines which ponds are connected and if they form a metapopulation. The duration of the life history stages of amphibians relative to the duration of the vernal pool before it dries up regulates the ecological development of metapopulations connecting aquatic patches to terrestrial patches.
Population
A population is all the organisms that both belong to the same group or species and live in the same geographical area. The area that is used to define a sexual population is such that inter-breeding is possible between any pair within the area and more probable than cross-breeding with individuals...
s of the same species
Species
In biology, a species is one of the basic units of biological classification and a taxonomic rank. A species is often defined as a group of organisms capable of interbreeding and producing fertile offspring. While in many cases this definition is adequate, more precise or differing measures are...
which interact at some level. The term metapopulation was coined by Richard Levins
Richard Levins
Richard "Dick" Levins is a mathematical ecologist, and political activist. He is best known for his work on evolution in changing environments....
in 1970 to describe a model of population dynamics of insect pests in agricultural fields, but the idea has been most broadly applied to species in naturally or artificially fragmented habitats
Habitat fragmentation
Habitat fragmentation as the name implies, describes the emergence of discontinuities in an organism's preferred environment , causing population fragmentation...
. In Levins' own words, it consists of "a population of populations".
A metapopulation is generally considered to consist of several distinct populations together with areas of suitable habitat which are currently unoccupied. In classical metapopulation theory, each population cycles in relative independence of the other populations and eventually goes extinct as a consequence of demographic stochastic
Stochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
ity (fluctuations in population size due to random demographic events); the smaller the population, the more prone it is to extinction.
Although individual populations have finite life-spans, the metapopulation as a whole is often stable because immigrants from one population (which may, for example, be experiencing a population boom) are likely to re-colonize habitat which has been left open by the extinction of another population. They may also emigrate to a small population and rescue that population from extinction (called the rescue effect).
The development of metapopulation theory, in conjunction with the development of source-sink dynamics
Source-sink dynamics
Source-sink dynamics is a theoretical model used by ecologists to describe how variation in habitat quality may affect the population growth or decline of organisms....
, emphasised the importance of connectivity between seemingly isolated populations. Although no single population may be able to guarantee the long-term survival of a given species, the combined effect of many populations may be able to do this.
Metapopulation theory was first developed for terrestrial ecosystems, and subsequently applied to the marine realm. In fisheries science, the term "sub-population" is equivalent to the metapopulation science term "local population". Most marine examples are provided by relatively sedentary species occupying discrete patches of habitat, with both local recruitment and recruitment from other local populations in the larger metapopulation. Kritzer & Sale have argued against strict application of the metapopulation definitional criteria that extinction risks to local populations must be non-negligible.
An important contributor to metapopulation theory is the Finnish
Finland
Finland , officially the Republic of Finland, is a Nordic country situated in the Fennoscandian region of Northern Europe. It is bordered by Sweden in the west, Norway in the north and Russia in the east, while Estonia lies to its south across the Gulf of Finland.Around 5.4 million people reside...
biologist, Ilkka Hanski
Ilkka Hanski
Ilkka A. Hanski FRS is a Finnish scientist, working in the field of ecology, at Helsinki University, Finland. The Metapopulation Research Group led by Hanski has been nominated as a Center of Excellence by the Academy of Finland...
http://www.helsinki.fi/~ihanski/, of the University of Helsinki
University of Helsinki
The University of Helsinki is a university located in Helsinki, Finland since 1829, but was founded in the city of Turku in 1640 as The Royal Academy of Turku, at that time part of the Swedish Empire. It is the oldest and largest university in Finland with the widest range of disciplines available...
.
Predation and Oscillations
The first experiments with predationPredation
In ecology, predation describes a biological interaction where a predator feeds on its prey . Predators may or may not kill their prey prior to feeding on them, but the act of predation always results in the death of its prey and the eventual absorption of the prey's tissue through consumption...
and spatial heterogeneity
Spatial heterogeneity
Spatial heterogeneity is a property generally ascribed to a landscape or to a population. It refers to the uneven distribution of various concentrations of each species within an area. A landscape with spatial heterogeneity has a mix of concentrations of multiple species of plants or animals , or...
were conducted by G.F. Gause in the 1930s, based on the Lotka-Volterra equation
Lotka-Volterra equation
The Lotka–Volterra equations, also known as the predator–prey equations, are a pair of first-order, non-linear, differential equations frequently used to describe the dynamics of biological systems in which two species interact, one a predator and one its prey...
, which was formulated in the mid-1920s, but no further application had been conducted. The Lotka-Volterra equation suggested that the relationship between predators and their prey would result in population oscillations over time based on the initial densities of predator and prey. Gause's early experiments to prove the predicted oscillations of this theory failed because the predator-prey interactions were not influenced by immigration. However, once immigration was introduced, the population cycles accurately depicted the oscillations predicted by the Lotka-Volterra equation, with the peaks in prey abundance shifted slightly to the left of the peaks of the predator densities. Huffaker's experiments expanded on those of Gause by examining how both the factors of migration and spatial heterogeneity lead to predator-prey oscillations.
Huffaker's experiments on predator-prey interactions (1958)
In order to study predation and population oscillations, Huffaker used mite species, one being the predator and the other being the prey. He set up a controlled experiment using oranges, which the prey fed on, as the spatially structured habitat in which the predator and prey would interact. At first, Huffaker experienced difficulties similar to those of Gause in creating a stable predator-prey interaction. By using oranges only, the prey species quickly went extinct followed consequently with predator extinction. However, he discovered that by modifying the spatial structure of the habitat, he could manipulate the population dynamics and allow the overall survival rate for both species to increase. He did this by altering the distance between the prey and oranges (their food), establishing barriers to predator movement, and creating corridors for the prey to disperse. These changes resulted in increased habitat patches and in turn provided more areas for the prey to seek temporary protection. When the prey would go extinct locally at one habitat patch, they were able to reestablish by migrating to new patches before being attacked by predators. This habitat spatial structure of patches allowed for coexistence between the predator and prey species and promoted a stable population oscillation model. Although the term metapopulation had not yet been coined, the environmental factors of spatial heterogeneitySpatial heterogeneity
Spatial heterogeneity is a property generally ascribed to a landscape or to a population. It refers to the uneven distribution of various concentrations of each species within an area. A landscape with spatial heterogeneity has a mix of concentrations of multiple species of plants or animals , or...
and habitat patchiness would later describe the conditions of a metapopulation relating to how groups of spatially separated populations of species interact with one another. Huffaker's experiment is significant because it showed how metapopulations can directly affect the predator-prey interactions and in turn influence population dynamics.
In spatially structured landscapes, species populations exist as metapopulations,
which consist of subpopulations each of which inhabits a habitat patch
Agglomeration payment
If individual members of species can move between patches, this is beneficial
for survival of metapopulation because it allows recolonization of patches
The Levins model
Levins' original model applied to a metapopulation distributed over many patches of suitable habitat with significantly less interaction between patches than within a patch. Population dynamics within a patch were simplified to the point where only presence and absence were considered. Each patch in his model is either populated or not.Let N be the fraction of patches occupied at a given time. During a time dt, each occupied patch can become unoccupied with an extinction probability edt. Additionally, 1 − N of the patches are unoccupied. Assuming a constant rate c of propagule
Propagule
In horticulture, a propagule is any plant material used for the purpose of plant propagation. In asexual reproduction, a propagule may be a woody, semi-hardwood, or softwood cutting, leaf section, or any number of other plant parts. In sexual reproduction, a propagule is a seed or spore...
generation from each of the N occupied patches, during a time dt, each unoccupied patch can become occupied with a colonization probability cNdt . Accordingly, the time rate of change of occupied patches, dN/dt, is
This equation is mathematically equivalent to the logistic model, with a carrying capacity K given by
and growth rate r
At equilibrium, therefore, some fraction of the species's habitat will always be unoccupied.
Stochasticity and Metapopulations
Huffaker's studies of spatial structure and species interactions are an example of early experimentation in metapopulation dynamics. Since the experiments of Huffaker and Levins, models have been created which integrate stochastic factors. These models have proven that the combination of environmental variability (stochascity) and relatively small migration rates cause indefinite or unpredictable persistence. However, Huffaker's experiment almost guaranteed infinite persistence because of the controlled immigration variable.Stochastic patch occupancy models (SPOMs)
One major drawback of the Levins model is that it is deterministic, whereas the fundamental metapopulation processes are stochasticStochastic
Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...
. Metapopulations are particularly useful when discussing species in disturbed habitats, and the viability of their populations
Minimum Viable Population
Minimum viable population is a lower bound on the population of a species, such that it can survive in the wild. This term is used in the fields of biology, ecology, and conservation biology...
, i.e., how likely they are to become extinct in a given time interval. The Levins model cannot address this issue. A simple way to extend the Levins' model to incorporate space and stochastic considerations is by using the contact process (mathematics). Simple modifications to this model can also incorporate for patch dynamics
Patch dynamics
Patch dynamics is a conceptual approach to ecosystem and habitat analysis that emphasizes dynamics of heterogeneity within a system ....
. At a given percolation threshold
Percolation threshold
Percolation threshold is a mathematical term related to percolation theory, which is the formation of long-range connectivity in random systems. Below the threshold a giant connected component does not exist while above it, there exists a giant component of the order of system size...
, habitat fragmentation
Habitat fragmentation
Habitat fragmentation as the name implies, describes the emergence of discontinuities in an organism's preferred environment , causing population fragmentation...
effects take place in these configuration predicting more drastic extinction thresholds.
For conservation biology
Conservation biology
Conservation biology is the scientific study of the nature and status of Earth's biodiversity with the aim of protecting species, their habitats, and ecosystems from excessive rates of extinction...
purposes, metapopulation models must include (a) the finite nature of metapopulations (how many patches are suitable for habitat), and (b) the probabilistic
Probability theory
Probability theory is the branch of mathematics concerned with analysis of random phenomena. The central objects of probability theory are random variables, stochastic processes, and events: mathematical abstractions of non-deterministic events or measured quantities that may either be single...
nature of extinction and colonisation. Also, note that in order to apply these models, the extinctions and colonisations of the patches must be asynchronous.
Micro Habitat Patches (MHPs) and bacterial metapopulations
By combining nanotechnologyNanotechnology
Nanotechnology is the study of manipulating matter on an atomic and molecular scale. Generally, nanotechnology deals with developing materials, devices, or other structures possessing at least one dimension sized from 1 to 100 nanometres...
with landscape ecology
Landscape ecology
Landscape ecology is the science of studying and improving relationships between urban development and ecological processes in the environment and particular ecosystems...
, a habitat landscape can be nanofabricated on-chip by building a collection of nanofabricated bacterial habitats, and connecting them by corridors in different topological arrangements and with nano-scale channels providing them with the local ecosystem service of habitat renewal. These landscapes of MHPs can be used as physical implementations of an adaptive landscape : by generating a spatial mosaic of patches of opportunity distributed in space and time. The patchy nature of these fluidic landscapes allows for the study of adapting bacterial cells in a metapopulation system operating on-chip within a synthetic ecosystem. The metapopulation biology and evolutionary ecology
Evolutionary ecology
Evolutionary ecology lies at the intersection of ecology and evolutionary biology. It approaches the study of ecology in a way that explicitly considers the evolutionary histories of species and the interactions between them. Conversely, it can be seen as an approach to the study of evolution that...
of these bacterial systems, in these synthetic ecosystems, can be addressed using experimental biophysics
Biophysics
Biophysics is an interdisciplinary science that uses the methods of physical science to study biological systems. Studies included under the branches of biophysics span all levels of biological organization, from the molecular scale to whole organisms and ecosystems...
.
Life history evolution
Metapopulation models have been used to explain life-history evolution, such as the ecological stabilityEcological stability
Ecological stability can refer to types of stability in a continuum ranging from resilience to constancy to persistence. The precise definition depends on the ecosystem in question, the variable or variables of interest, and the overall context...
of amphibian metamorphosis in small vernal ponds. Alternative ecological strategies have evolved. For example, some salamanders forgo metamorphosis and sexually mature as aquatic neotenes
Neoteny
Neoteny , also called juvenilization , is one of the two ways by which paedomorphism can arise. Paedomorphism is the retention by adults of traits previously seen only in juveniles, and is a subject studied in the field of developmental biology. In neoteny, the physiological development of an...
. The seasonal duration of wetlands and the migratory range of the species determines which ponds are connected and if they form a metapopulation. The duration of the life history stages of amphibians relative to the duration of the vernal pool before it dries up regulates the ecological development of metapopulations connecting aquatic patches to terrestrial patches.
See also
- Competition (biology)Competition (biology)Competition is an interaction between organisms or species, in which the fitness of one is lowered by the presence of another. Limited supply of at least one resource used by both is required. Competition both within and between species is an important topic in ecology, especially community ecology...
- Conservation biologyConservation biologyConservation biology is the scientific study of the nature and status of Earth's biodiversity with the aim of protecting species, their habitats, and ecosystems from excessive rates of extinction...
- Landscape ecologyLandscape ecologyLandscape ecology is the science of studying and improving relationships between urban development and ecological processes in the environment and particular ecosystems...
- Lotka-Volterra equationLotka-Volterra equationThe Lotka–Volterra equations, also known as the predator–prey equations, are a pair of first-order, non-linear, differential equations frequently used to describe the dynamics of biological systems in which two species interact, one a predator and one its prey...
- OscillationOscillationOscillation is the repetitive variation, typically in time, of some measure about a central value or between two or more different states. Familiar examples include a swinging pendulum and AC power. The term vibration is sometimes used more narrowly to mean a mechanical oscillation but sometimes...
- Population viability analysisPopulation viability analysisPopulation viability analysis is a species-specific method of risk assessment frequently used in conservation biology.It is traditionally defined as the process that determines the probability that a population will go extinct within a given number of years.More recently, PVA has been described...
- PredationPredationIn ecology, predation describes a biological interaction where a predator feeds on its prey . Predators may or may not kill their prey prior to feeding on them, but the act of predation always results in the death of its prey and the eventual absorption of the prey's tissue through consumption...
- Spatial heterogeneitySpatial heterogeneitySpatial heterogeneity is a property generally ascribed to a landscape or to a population. It refers to the uneven distribution of various concentrations of each species within an area. A landscape with spatial heterogeneity has a mix of concentrations of multiple species of plants or animals , or...