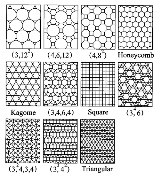
, which is the formation of long-range connectivity in random systems. Below the threshold a giant connected component does not exist while above it, there exists a giant component of the order of system size. In engineering and coffee making, percolation represents the flow of fluids through porous media, but in the mathematics and physics worlds it generally refers to simplified lattice models
of random systems or networks (graphs), and the nature of the connectivity in them.
The percolation threshold is the critical value of the occupation probability
p, or more generally a critical surface for a group of parameters p1, p2, ...,
such that infinite connectivity (percolation
) first occurs.
The most common percolation model is to take a regular lattice, like a square lattice, and
make it into a random network by randomly "occupying" sites (vertices) or bonds (edges) with a
statistically independent probability p.
"An oasis in the middle of the ocean: Office buildings, hotels, theaters and shopping centers, sitting slightly above the surface of the sea in neat rows surrounded by greenery and flowers, with canals of clear blue water, water taxis and gondolas providing transportation for the inhabitants."
![]()
"A new country that has never existed before, which will be built in a moderately tropical sea, a perfect climate, a paradise: Utopia!"
![]()
"The International University of Advanced Studies, has been founded and is dedicated to teaching the disciplines and imparting the knowledge that will be needed in the next century for survival on Earth, the colonization of the oceans, and the exploration and colonization of space. Access to classes taught by the University may be obtained over the Internet."
![]()
"New Utopia will be managed by a Board of Governors; managing what we believe to be the most perfect city/state ever conceived, blending the philosophies of both Ayn Rand and Robert Heinlein."
![]()

