
Lévy flight
Encyclopedia
A Lévy flight is a random walk
in which the step-lengths have a probability distribution
that is heavy-tailed
. When defined as a walk in a space of dimension greater than one, the steps made are in isotropic random directions. The name "Lévy flight" refers to the French mathematician Paul Pierre Lévy
.
The term "Lévy flight" was coined by Benoît Mandelbrot
, who used this for one specific definition of the distribution of step sizes. He used the term Cauchy flight for the case where the distribution of step sizes is a Cauchy distribution
, and Rayleigh flight for when the distribution is a normal distribution (which is not an example of a heavy-tailed probability distribution).
Later researchers have extended the use of the term "Lévy flight" to include cases where the random walk takes place on a discrete grid rather than on a continuous space.
in which the steps are defined in terms of the step-lengths, which have a certain probability distribution
, with the directions of the steps being isotropic and random.
The particular case for which Mandelbrot used the term "Lévy flight" is defined by the survivor function (commonly known as the survival function) of the distribution of step-sizes, U, being
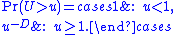
Here D is a parameter related to the fractal dimension
and the distribution is a particular case of the Pareto distribution. Later researchers allow the distribution of step sizes to be any distribution for which the survival function
has a power-like tail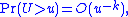
for some k satisfying 1 < k < 3. (Here the notation O is the Big O notation
.) Such distributions have an infinite variance
. Typical examples are the symmetric stable distributions.
.. For general distributions of the step-size, satisfying the power-like condition, the distance from the origin of the random walk tends, after a large number of steps, to a stable distribution.
The exponential scaling of the step lengths gives Lévy flights a scale invariant
property, and they are used to model data that exhibits clustering.
and is useful in stochastic measurement and simulations for random or pseudo-random natural phenomena. Examples include earthquake
data analysis, financial mathematics, cryptography
, signals analysis as well as many applications in astronomy
, biology
, and physics
.
When sharks and other ocean predators can’t find food, they abandon Brownian motion
, the random motion seen in swirling gas molecules, for Lévy flight — a mix of long trajectories and short, random movements found in turbulent fluids. Researchers analyzed over 12 million movements recorded over 5,700 days in 55 radio-tagged animals from 14 ocean predator species in the Atlantic and Pacific Oceans, including silky shark
s, yellowfin tuna
, blue marlin and swordfish. The data showed that Lévy flights interspersed with Brownian motion can describe the animals' hunting patterns. Birds and other animals also seem to follow Lévy flights when searching for food.
Efficient routing in a network can be performed by links having a Levy flight length distribution with specific values of alpha.
Random walk
A random walk, sometimes denoted RW, is a mathematical formalisation of a trajectory that consists of taking successive random steps. For example, the path traced by a molecule as it travels in a liquid or a gas, the search path of a foraging animal, the price of a fluctuating stock and the...
in which the step-lengths have a probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
that is heavy-tailed
Heavy-tailed distribution
In probability theory, heavy-tailed distributions are probability distributions whose tails are not exponentially bounded: that is, they have heavier tails than the exponential distribution...
. When defined as a walk in a space of dimension greater than one, the steps made are in isotropic random directions. The name "Lévy flight" refers to the French mathematician Paul Pierre Lévy
Paul Pierre Lévy
Paul Pierre Lévy was a Jewish French mathematician who was active especially in probability theory, introducing martingales and Lévy flights...
.
The term "Lévy flight" was coined by Benoît Mandelbrot
Benoît Mandelbrot
Benoît B. Mandelbrot was a French American mathematician. Born in Poland, he moved to France with his family when he was a child...
, who used this for one specific definition of the distribution of step sizes. He used the term Cauchy flight for the case where the distribution of step sizes is a Cauchy distribution
Cauchy distribution
The Cauchy–Lorentz distribution, named after Augustin Cauchy and Hendrik Lorentz, is a continuous probability distribution. As a probability distribution, it is known as the Cauchy distribution, while among physicists, it is known as the Lorentz distribution, Lorentz function, or Breit–Wigner...
, and Rayleigh flight for when the distribution is a normal distribution (which is not an example of a heavy-tailed probability distribution).
Later researchers have extended the use of the term "Lévy flight" to include cases where the random walk takes place on a discrete grid rather than on a continuous space.
Definition
A Lévy flight is a random walkRandom walk
A random walk, sometimes denoted RW, is a mathematical formalisation of a trajectory that consists of taking successive random steps. For example, the path traced by a molecule as it travels in a liquid or a gas, the search path of a foraging animal, the price of a fluctuating stock and the...
in which the steps are defined in terms of the step-lengths, which have a certain probability distribution
Probability distribution
In probability theory, a probability mass, probability density, or probability distribution is a function that describes the probability of a random variable taking certain values....
, with the directions of the steps being isotropic and random.
The particular case for which Mandelbrot used the term "Lévy flight" is defined by the survivor function (commonly known as the survival function) of the distribution of step-sizes, U, being
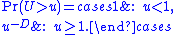
Here D is a parameter related to the fractal dimension
Fractal dimension
In fractal geometry, the fractal dimension, D, is a statistical quantity that gives an indication of how completely a fractal appears to fill space, as one zooms down to finer and finer scales. There are many specific definitions of fractal dimension. The most important theoretical fractal...
and the distribution is a particular case of the Pareto distribution. Later researchers allow the distribution of step sizes to be any distribution for which the survival function
Survival function
The survival function, also known as a survivor function or reliability function, is a property of any random variable that maps a set of events, usually associated with mortality or failure of some system, onto time. It captures the probability that the system will survive beyond a specified time...
has a power-like tail
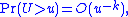
for some k satisfying 1 < k < 3. (Here the notation O is the Big O notation
Big O notation
In mathematics, big O notation is used to describe the limiting behavior of a function when the argument tends towards a particular value or infinity, usually in terms of simpler functions. It is a member of a larger family of notations that is called Landau notation, Bachmann-Landau notation, or...
.) Such distributions have an infinite variance
Variance
In probability theory and statistics, the variance is a measure of how far a set of numbers is spread out. It is one of several descriptors of a probability distribution, describing how far the numbers lie from the mean . In particular, the variance is one of the moments of a distribution...
. Typical examples are the symmetric stable distributions.
Properties
Lévy flights are, by construction, Markov processesMarkov property
In probability theory and statistics, the term Markov property refers to the memoryless property of a stochastic process. It was named after the Russian mathematician Andrey Markov....
.. For general distributions of the step-size, satisfying the power-like condition, the distance from the origin of the random walk tends, after a large number of steps, to a stable distribution.
The exponential scaling of the step lengths gives Lévy flights a scale invariant
Scale invariance
In physics and mathematics, scale invariance is a feature of objects or laws that do not change if scales of length, energy, or other variables, are multiplied by a common factor...
property, and they are used to model data that exhibits clustering.
Applications
The definition of a Lévy flight stems from the mathematics related to chaos theoryChaos theory
Chaos theory is a field of study in mathematics, with applications in several disciplines including physics, economics, biology, and philosophy. Chaos theory studies the behavior of dynamical systems that are highly sensitive to initial conditions, an effect which is popularly referred to as the...
and is useful in stochastic measurement and simulations for random or pseudo-random natural phenomena. Examples include earthquake
Earthquake
An earthquake is the result of a sudden release of energy in the Earth's crust that creates seismic waves. The seismicity, seismism or seismic activity of an area refers to the frequency, type and size of earthquakes experienced over a period of time...
data analysis, financial mathematics, cryptography
Cryptography
Cryptography is the practice and study of techniques for secure communication in the presence of third parties...
, signals analysis as well as many applications in astronomy
Astronomy
Astronomy is a natural science that deals with the study of celestial objects and phenomena that originate outside the atmosphere of Earth...
, biology
Biology
Biology is a natural science concerned with the study of life and living organisms, including their structure, function, growth, origin, evolution, distribution, and taxonomy. Biology is a vast subject containing many subdivisions, topics, and disciplines...
, and physics
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
.
When sharks and other ocean predators can’t find food, they abandon Brownian motion
Brownian motion
Brownian motion or pedesis is the presumably random drifting of particles suspended in a fluid or the mathematical model used to describe such random movements, which is often called a particle theory.The mathematical model of Brownian motion has several real-world applications...
, the random motion seen in swirling gas molecules, for Lévy flight — a mix of long trajectories and short, random movements found in turbulent fluids. Researchers analyzed over 12 million movements recorded over 5,700 days in 55 radio-tagged animals from 14 ocean predator species in the Atlantic and Pacific Oceans, including silky shark
Silky shark
The silky shark is a species of requiem shark, family Carcharhinidae, named for the smooth texture of its skin. It is one of the most abundant sharks in the pelagic zone, and can be found around the world in tropical waters. Highly mobile and migratory, this shark is most often found over the edge...
s, yellowfin tuna
Yellowfin tuna
The yellowfin tuna is a species of tuna found in pelagic waters of tropical and subtropical oceans worldwide.Yellowfin is often marketed as ahi, from its Hawaiian name ahi although the name ahi in Hawaiian also refers to the closely related bigeye tuna. The species name, albacares can lead to...
, blue marlin and swordfish. The data showed that Lévy flights interspersed with Brownian motion can describe the animals' hunting patterns. Birds and other animals also seem to follow Lévy flights when searching for food.
Efficient routing in a network can be performed by links having a Levy flight length distribution with specific values of alpha.
See also
- Fat-tailed distribution
- Heavy-tailed distributionHeavy-tailed distributionIn probability theory, heavy-tailed distributions are probability distributions whose tails are not exponentially bounded: that is, they have heavier tails than the exponential distribution...
- Lévy processLévy processIn probability theory, a Lévy process, named after the French mathematician Paul Lévy, is any continuous-time stochastic process that starts at 0, admits càdlàg modification and has "stationary independent increments" — this phrase will be explained below...
- Lévy alpha-stable distribution
- Lévy flight foraging hypothesisLévy flight foraging hypothesisThe Lévy flight foraging hypothesis is a hypothesis in the field of biology that may be stated as follows:Since Lévy flights and walks can optimize search efficiencies, therefore natural selection should have led to adaptations for Lévy flight foraging....

