Irrotational vector field
Encyclopedia
In vector calculus a conservative vector field is a vector field
which is the gradient
of a function
, known in this context as a scalar potential
. Conservative vector fields have the property that the line integral
from one point to another is independent of the choice of path connecting the two points: it is path independent. Conversely, path independence is equivalent to the vector field being conservative. Conservative vector fields are also irrotational, meaning that (in three-dimensions) they have vanishing curl. In fact, an irrotational vector field is necessarily conservative provided that a certain condition on the geometry of the domain holds: it must be simply connected.
An irrotational vector field which is also solenoidal is called a Laplacian vector field because it is the gradient of a solution of Laplace's equation
.
is said to be conservative if there exists a scalar field 
such that
Here
denotes the gradient
of
. When the above equation holds, 
is called a scalar potential
for
.
The fundamental theorem of vector calculus
states that any vector field can be expressed as the sum of a conservative vector field and a solenoidal field.
is a region of three-dimensional space, and that 
is a rectifiable path in 
with start point 
and end point 
. If 
is a conservative vector field then the gradient theorem
states that
This holds as a consequence of the Chain Rule
and the Fundamental Theorem of Calculus
.
An equivalent formulation of this is to say that
for every closed loop in S. The converse is also true: if the circulation of v around every closed loop in an open set
S is zero, then v is a conservative vector field.
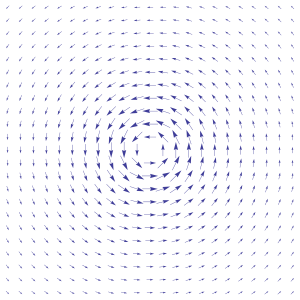A vector field 
is said to be irrotational if its curl is zero. That is, if
For this reason, such vector fields are sometimes referred to as curl-free vector fields.
It is an identity of vector calculus that for any scalar field
:
Therefore every conservative vector field is also an irrotational vector field.
Provided that
is a simply-connected region, the converse of this is true: every
irrotational vector field is also a conservative vector field.
The above statement is not true if
is not simply-connected. Let 
be the usual 3-dimensional space, except with the 
-axis removed; that is 
. Now define a vector field by
Then
exists and has zero curl at every point in 
; that is
is irrotational. However the circulation of 
around the unit circle in the 
-plane is equal to 
. Therefore 
does not have the path independence property discussed above, and is not conservative.
In a simply-connected region an irrotational vector field has the path independence property. This can be seen by noting that in such a region an irrotational vector field is conservative, and conservative vector fields have the path independence property. The result can also be proved directly by using Stokes' theorem
. In a connected region any vector field which has the path independence property must also be irrotational.
More abstractly, a conservative vector field is an exact 1-form. That is, it is a 1-form equal to the exterior derivative
of some 0-form (scalar field)
. An irrotational vector field is a closed 1-form
. Since d2 = 0, any exact form is closed, so any conservative vector field is irrotational. The domain is simply connected if and only if its first homology group is 0, which is equivalent to its first cohomology group being 0. The first de Rham cohomology
group
is 0 if and only if all closed 1-forms are exact.
of a fluid is a vector field, and the vorticity 
of the flow can be defined by
A common alternative notation for vorticity is
.
If
is irrotational, with 
, then the flow is said to be an irrotational flow. The vorticity of an irrotational flow is zero.
Kelvin's circulation theorem
states that a fluid that is irrotational in an inviscid flow
will remain irrotational. This result can be derived from the vorticity transport equation, obtained by taking the curl of the Navier-stokes equations.
For a two-dimensional flow the vorticity acts as a measure of the local rotation of fluid elements. Note that the vorticity does not imply anything about the global behaviour of a fluid. It is possible for a fluid traveling in a straight line to have vorticity, and it is possible for a fluid which moves in a circle to be irrotational.
is conservative then the force is said to be a conservative force
.
The most prominent examples of conservative forces are the force of gravity and the electric field associated to a static charge. According to Newton's law of gravitation
, the gravitational force,
, acting on a mass 
, due to a mass 
which is a distance 
away, obeys the equation
where
is the Gravitational Constant
and
is a unit vector pointing from 
towards 
. The force of gravity is conservative because 
, where
is the Gravitational potential energy.
For conservative force
s, path independence can be interpreted to mean that the work done in going from a point
to a point 
is independent of the path chosen, and that the work W done in going around a closed loop is zero:
The total energy
of a particle moving under the influence of conservative forces is conserved, in the sense that a loss of potential energy is converted to an equal quantity of kinetic energy or vice versa.
Vector field
In vector calculus, a vector field is an assignmentof a vector to each point in a subset of Euclidean space. A vector field in the plane for instance can be visualized as an arrow, with a given magnitude and direction, attached to each point in the plane...
which is the gradient
Gradient
In vector calculus, the gradient of a scalar field is a vector field that points in the direction of the greatest rate of increase of the scalar field, and whose magnitude is the greatest rate of change....
of a function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
, known in this context as a scalar potential
Scalar potential
A scalar potential is a fundamental concept in vector analysis and physics . The scalar potential is an example of a scalar field...
. Conservative vector fields have the property that the line integral
Line integral
In mathematics, a line integral is an integral where the function to be integrated is evaluated along a curve.The function to be integrated may be a scalar field or a vector field...
from one point to another is independent of the choice of path connecting the two points: it is path independent. Conversely, path independence is equivalent to the vector field being conservative. Conservative vector fields are also irrotational, meaning that (in three-dimensions) they have vanishing curl. In fact, an irrotational vector field is necessarily conservative provided that a certain condition on the geometry of the domain holds: it must be simply connected.
An irrotational vector field which is also solenoidal is called a Laplacian vector field because it is the gradient of a solution of Laplace's equation
Laplace's equation
In mathematics, Laplace's equation is a second-order partial differential equation named after Pierre-Simon Laplace who first studied its properties. This is often written as:where ∆ = ∇² is the Laplace operator and \varphi is a scalar function...
.
Definition
A vector field is said to be conservative if there exists a scalar field such thatHere
denotes the gradientGradient
In vector calculus, the gradient of a scalar field is a vector field that points in the direction of the greatest rate of increase of the scalar field, and whose magnitude is the greatest rate of change....
of
. When the above equation holds, is called a scalar potentialScalar potential
A scalar potential is a fundamental concept in vector analysis and physics . The scalar potential is an example of a scalar field...
for
.The fundamental theorem of vector calculus
Helmholtz decomposition
In physics and mathematics, in the area of vector calculus, Helmholtz's theorem, also known as the fundamental theorem of vector calculus, states that any sufficiently smooth, rapidly decaying vector field in three dimensions can be resolved into the sum of an irrotational vector field and a...
states that any vector field can be expressed as the sum of a conservative vector field and a solenoidal field.
Path independence
A key property of a conservative vector field is that its integral along a path depends only on the endpoints of that path, not the particular route taken. Suppose that is a region of three-dimensional space, and that is a rectifiable path in with start point and end point . If is a conservative vector field then the gradient theoremGradient theorem
The gradient theorem, also known as the fundamental theorem of calculus for line integrals, says that a line integral through a gradient field can be evaluated by evaluating the original scalar field at the endpoints of the curve: \phi\left-\phi\left = \int_L...
states that
This holds as a consequence of the Chain Rule
Chain rule
In calculus, the chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f is a function and g is a function, then the chain rule expresses the derivative of the composite function in terms of the derivatives of f and g.In integration, the...
and the Fundamental Theorem of Calculus
Fundamental theorem of calculus
The first part of the theorem, sometimes called the first fundamental theorem of calculus, shows that an indefinite integration can be reversed by a differentiation...
.
An equivalent formulation of this is to say that
for every closed loop in S. The converse is also true: if the circulation of v around every closed loop in an open set
Open set
The concept of an open set is fundamental to many areas of mathematics, especially point-set topology and metric topology. Intuitively speaking, a set U is open if any point x in U can be "moved" a small amount in any direction and still be in the set U...
S is zero, then v is a conservative vector field.
Irrotational vector fields
is said to be irrotational if its curl is zero. That is, ifFor this reason, such vector fields are sometimes referred to as curl-free vector fields.
It is an identity of vector calculus that for any scalar field
:Therefore every conservative vector field is also an irrotational vector field.
Provided that
is a simply-connected region, the converse of this is true: everyirrotational vector field is also a conservative vector field.
The above statement is not true if
is not simply-connected. Let be the usual 3-dimensional space, except with the -axis removed; that is . Now define a vector field byThen
exists and has zero curl at every point in ; that is is irrotational. However the circulation of around the unit circle in the -plane is equal to . Therefore does not have the path independence property discussed above, and is not conservative.In a simply-connected region an irrotational vector field has the path independence property. This can be seen by noting that in such a region an irrotational vector field is conservative, and conservative vector fields have the path independence property. The result can also be proved directly by using Stokes' theorem
Stokes' theorem
In differential geometry, Stokes' theorem is a statement about the integration of differential forms on manifolds, which both simplifies and generalizes several theorems from vector calculus. Lord Kelvin first discovered the result and communicated it to George Stokes in July 1850...
. In a connected region any vector field which has the path independence property must also be irrotational.
More abstractly, a conservative vector field is an exact 1-form. That is, it is a 1-form equal to the exterior derivative
Exterior derivative
In differential geometry, the exterior derivative extends the concept of the differential of a function, which is a 1-form, to differential forms of higher degree. Its current form was invented by Élie Cartan....
of some 0-form (scalar field)
. An irrotational vector field is a closed 1-formClosed form
-Maths:* Closed-form expression, a finitary expression* Closed differential form, a differential form \alpha with the property that d\alpha = 0-Poetry:* In poetry analysis, a type of poetry that exhibits regular structure, such as meter or a rhyming pattern;...
. Since d2 = 0, any exact form is closed, so any conservative vector field is irrotational. The domain is simply connected if and only if its first homology group is 0, which is equivalent to its first cohomology group being 0. The first de Rham cohomology
De Rham cohomology
In mathematics, de Rham cohomology is a tool belonging both to algebraic topology and to differential topology, capable of expressing basic topological information about smooth manifolds in a form particularly adapted to computation and the concrete representation of cohomology classes...
group
is 0 if and only if all closed 1-forms are exact.Irrotational flows
The flow velocityFlow velocity
In fluid dynamics the flow velocity, or velocity field, of a fluid is a vector field which is used to mathematically describe the motion of a fluid...
of a fluid is a vector field, and the vorticity of the flow can be defined byA common alternative notation for vorticity is
.If
is irrotational, with , then the flow is said to be an irrotational flow. The vorticity of an irrotational flow is zero.Kelvin's circulation theorem
Kelvin's circulation theorem
In fluid mechanics, Kelvin's circulation theorem states In an inviscid, barotropic flow with conservative body forces, the circulation around a closed curve moving with the fluid remains constant with time. The theorem was developed by William Thomson, 1st Baron Kelvin...
states that a fluid that is irrotational in an inviscid flow
Inviscid flow
In fluid dynamics there are problems that are easily solved by using the simplifying assumption of an ideal fluid that has no viscosity. The flow of a fluid that is assumed to have no viscosity is called inviscid flow....
will remain irrotational. This result can be derived from the vorticity transport equation, obtained by taking the curl of the Navier-stokes equations.
For a two-dimensional flow the vorticity acts as a measure of the local rotation of fluid elements. Note that the vorticity does not imply anything about the global behaviour of a fluid. It is possible for a fluid traveling in a straight line to have vorticity, and it is possible for a fluid which moves in a circle to be irrotational.
Conservative forces
If the vector field associated to a force is conservative then the force is said to be a conservative forceConservative force
A conservative force is a force with the property that the work done in moving a particle between two points is independent of the path taken. Equivalently, if a particle travels in a closed loop, the net work done by a conservative force is zero.It is possible to define a numerical value of...
.
The most prominent examples of conservative forces are the force of gravity and the electric field associated to a static charge. According to Newton's law of gravitation
Newton's law of universal gravitation
Newton's law of universal gravitation states that every point mass in the universe attracts every other point mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them...
, the gravitational force,
, acting on a mass , due to a mass which is a distance away, obeys the equationwhere
is the Gravitational ConstantGravitational constant
The gravitational constant, denoted G, is an empirical physical constant involved in the calculation of the gravitational attraction between objects with mass. It appears in Newton's law of universal gravitation and in Einstein's theory of general relativity. It is also known as the universal...
and
is a unit vector pointing from towards . The force of gravity is conservative because , whereis the Gravitational potential energy.
For conservative force
Conservative force
A conservative force is a force with the property that the work done in moving a particle between two points is independent of the path taken. Equivalently, if a particle travels in a closed loop, the net work done by a conservative force is zero.It is possible to define a numerical value of...
s, path independence can be interpreted to mean that the work done in going from a point
to a point is independent of the path chosen, and that the work W done in going around a closed loop is zero:
The total energy
Conservation of energy
The nineteenth century law of conservation of energy is a law of physics. It states that the total amount of energy in an isolated system remains constant over time. The total energy is said to be conserved over time...
of a particle moving under the influence of conservative forces is conserved, in the sense that a loss of potential energy is converted to an equal quantity of kinetic energy or vice versa.
See also
- Beltrami vector field
- Complex lamellar vector field
- Helmholtz decompositionHelmholtz decompositionIn physics and mathematics, in the area of vector calculus, Helmholtz's theorem, also known as the fundamental theorem of vector calculus, states that any sufficiently smooth, rapidly decaying vector field in three dimensions can be resolved into the sum of an irrotational vector field and a...
- Solenoidal vector field
- Longitudinal and transverse vector fields