
Hilbert-Schmidt operator
Encyclopedia
In mathematics
, a Hilbert–Schmidt operator, named for David Hilbert
and Erhard Schmidt
, is a bounded operator
A on a Hilbert space
H with finite Hilbert–Schmidt norm
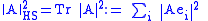
where is the norm of H and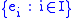 an orthonormal basis
an orthonormal basis
of H for an index set . Note that the index set need not be countable. This definition is independent of the choice of the basis, and therefore
. Note that the index set need not be countable. This definition is independent of the choice of the basis, and therefore
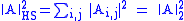
for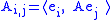 and
and  the Schatten norm
the Schatten norm
of . In Euclidean space
. In Euclidean space
 is also called Frobenius norm.
is also called Frobenius norm.
The product of two Hilbert–Schmidt operators has finite trace class norm
; therefore, if A and B are two Hilbert–Schmidt operators, the Hilbert–Schmidt inner product can be defined as
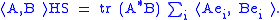
The Hilbert–Schmidt operators form a two-sided *-ideal
in the Banach algebra
of bounded operators on H. They also form a Hilbert space, which can be shown to be natural
ly isometrically isomorphic to the tensor product of Hilbert spaces
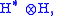
where H* is the dual space
of H.
The Hilbert–Schmidt operators are closed in the norm topology if, and only if, H is finite dimensional.
An important class of examples is provided by Hilbert–Schmidt integral operators.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a Hilbert–Schmidt operator, named for David Hilbert
David Hilbert
David Hilbert was a German mathematician. He is recognized as one of the most influential and universal mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many areas, including invariant theory and the axiomatization of...
and Erhard Schmidt
Erhard Schmidt
Erhard Schmidt was a German mathematician whose work significantly influenced the direction of mathematics in the twentieth century. He was born in Tartu, Governorate of Livonia . His advisor was David Hilbert and he was awarded his doctorate from Georg-August University of Göttingen in 1905...
, is a bounded operator
Bounded operator
In functional analysis, a branch of mathematics, a bounded linear operator is a linear transformation L between normed vector spaces X and Y for which the ratio of the norm of L to that of v is bounded by the same number, over all non-zero vectors v in X...
A on a Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
H with finite Hilbert–Schmidt norm
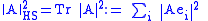
where is the norm of H and
 an orthonormal basis
an orthonormal basisOrthonormal basis
In mathematics, particularly linear algebra, an orthonormal basis for inner product space V with finite dimension is a basis for V whose vectors are orthonormal. For example, the standard basis for a Euclidean space Rn is an orthonormal basis, where the relevant inner product is the dot product of...
of H for an index set
 . Note that the index set need not be countable. This definition is independent of the choice of the basis, and therefore
. Note that the index set need not be countable. This definition is independent of the choice of the basis, and therefore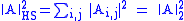
for
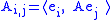 and
and  the Schatten norm
the Schatten normSchatten norm
In mathematics, specifically functional analysis, the Schatten norm arises as a generalization of p-integrability similar to the trace class norm and the Hilbert–Schmidt norm.-Definition:...
of
 . In Euclidean space
. In Euclidean spaceEuclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
 is also called Frobenius norm.
is also called Frobenius norm.The product of two Hilbert–Schmidt operators has finite trace class norm
Trace class
In mathematics, a trace class operator is a compact operator for which a trace may be defined, such that the trace is finite and independent of the choice of basis....
; therefore, if A and B are two Hilbert–Schmidt operators, the Hilbert–Schmidt inner product can be defined as
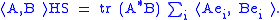
The Hilbert–Schmidt operators form a two-sided *-ideal
Ideal (ring theory)
In ring theory, a branch of abstract algebra, an ideal is a special subset of a ring. The ideal concept allows the generalization in an appropriate way of some important properties of integers like "even number" or "multiple of 3"....
in the Banach algebra
Banach algebra
In mathematics, especially functional analysis, a Banach algebra, named after Stefan Banach, is an associative algebra A over the real or complex numbers which at the same time is also a Banach space...
of bounded operators on H. They also form a Hilbert space, which can be shown to be natural
Natural transformation
In category theory, a branch of mathematics, a natural transformation provides a way of transforming one functor into another while respecting the internal structure of the categories involved. Hence, a natural transformation can be considered to be a "morphism of functors". Indeed this intuition...
ly isometrically isomorphic to the tensor product of Hilbert spaces
Tensor product of Hilbert spaces
In mathematics, and in particular functional analysis, the tensor product of Hilbert spaces is a way to extend the tensor product construction so that the result of taking a tensor product of two Hilbert space is another Hilbert space. Roughly speaking, the tensor product is the completion of the...
where H* is the dual space
Dual space
In mathematics, any vector space, V, has a corresponding dual vector space consisting of all linear functionals on V. Dual vector spaces defined on finite-dimensional vector spaces can be used for defining tensors which are studied in tensor algebra...
of H.
The Hilbert–Schmidt operators are closed in the norm topology if, and only if, H is finite dimensional.
An important class of examples is provided by Hilbert–Schmidt integral operators.

