Fréchet manifold
Encyclopedia
In mathematics
, in particular in nonlinear analysis, a Fréchet manifold is a topological space
modeled on a Fréchet space
in much the same way as a manifold is modeled on a Euclidean space
.
More precisely, a Fréchet manifold consists of a Hausdorff space
X with an atlas of coordinate charts over Fréchet spaces whose transitions are smooth mappings
. Thus X has an open cover {Uα}α ε I, and a collection of homeomorphism
s φα : Uα → Fα onto their images, where Fα are Fréchet spaces, such that
” Fréchet manifolds up to homeomorphism quite nicely. A 1969 theorem of David Henderson states that every infinite-dimensional, separable, metric
Fréchet manifold X can be embedded
as an open subset of the infinite-dimensional, separable Hilbert space
, H (up to linear isomorphism, there is only one such space).
The embedding homeomorphism can be used as a global chart for X. Thus, in the infinite-dimensional, separable, metric case, the “only” Fréchet manifolds are the open subsets of Hilbert space.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, in particular in nonlinear analysis, a Fréchet manifold is a topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
modeled on a Fréchet space
Fréchet space
In functional analysis and related areas of mathematics, Fréchet spaces, named after Maurice Fréchet, are special topological vector spaces. They are generalizations of Banach spaces...
in much the same way as a manifold is modeled on a Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
.
More precisely, a Fréchet manifold consists of a Hausdorff space
Hausdorff space
In topology and related branches of mathematics, a Hausdorff space, separated space or T2 space is a topological space in which distinct points have disjoint neighbourhoods. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" is the most frequently...
X with an atlas of coordinate charts over Fréchet spaces whose transitions are smooth mappings
Differentiation in Fréchet spaces
In mathematics, in particular in functional analysis and nonlinear analysis, it is possible to define the derivative of a function between two Fréchet spaces. This notion of differentiation is significantly weaker than the derivative in a Banach space. Nevertheless, it is the weakest notion of...
. Thus X has an open cover {Uα}α ε I, and a collection of homeomorphism
Homeomorphism
In the mathematical field of topology, a homeomorphism or topological isomorphism or bicontinuous function is a continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are...
s φα : Uα → Fα onto their images, where Fα are Fréchet spaces, such that
-
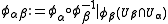is smooth for all pairs of indices α, β.
Classification up to homeomorphism
It is by no means true that a finite-dimensional manifold of dimension n is globally homeomorphic to Rn, or even an open subset of Rn. However, in an infinite-dimensional setting, it is possible to classify “well-behavedWell-behaved
Mathematicians very frequently speak of whether a mathematical object — a function, a set, a space of one sort or another — is "well-behaved" or not. The term has no fixed formal definition, and is dependent on mathematical interests, fashion, and taste...
” Fréchet manifolds up to homeomorphism quite nicely. A 1969 theorem of David Henderson states that every infinite-dimensional, separable, metric
Metric space
In mathematics, a metric space is a set where a notion of distance between elements of the set is defined.The metric space which most closely corresponds to our intuitive understanding of space is the 3-dimensional Euclidean space...
Fréchet manifold X can be embedded
Embedding
In mathematics, an embedding is one instance of some mathematical structure contained within another instance, such as a group that is a subgroup....
as an open subset of the infinite-dimensional, separable Hilbert space
Hilbert space
The mathematical concept of a Hilbert space, named after David Hilbert, generalizes the notion of Euclidean space. It extends the methods of vector algebra and calculus from the two-dimensional Euclidean plane and three-dimensional space to spaces with any finite or infinite number of dimensions...
, H (up to linear isomorphism, there is only one such space).
The embedding homeomorphism can be used as a global chart for X. Thus, in the infinite-dimensional, separable, metric case, the “only” Fréchet manifolds are the open subsets of Hilbert space.