Etendue
Encyclopedia
Etendue or étendue is a property of pencils of rays in an optical system
, which characterizes how "spread out" light
is in area and angle. It may also be seen as a volume in phase space.
From the source point of view, it is the area of the source times the solid angle
the system's entrance pupil
subtends as seen from the source. From the system point of view, the etendue is the area of the entrance pupil times the solid angle the source subtends as seen from the pupil. These definitions must be applied for infinitesimally small "elements" of area and solid angle, which must then be summed over both the source and the diaphragm as shown below.
Etendue is important because it never decreases in any optical system. A perfect optical system produces an image with the same etendue as the source. The etendue is related to the Lagrange invariant and the optical invariant, which share the property of being constant in an ideal optical system. The radiance
of an optical system is equal to the derivative of the radiant flux
with respect to the etendue.
The term étendue comes from the French word for extent or spread. The French word for the optical property is étendue géométrique, meaning "geometrical extent".
Other names for this property are acceptance, throughput, light-grasp, collecting power, and the AΩ product. Throughput and AΩ product are especially used in radiometry
and radiative transfer where it is related to the view factor (or shape factor). It is a central concept in nonimaging optics
.
n. The surface is crossed by (or emits) light confined to a solid angle, dΩ, at an angle θ with the normal nS . The area of dS projected in the direction of the light propagation is
. The etendue of this light crossing dS is defined in 2D
as
and in 3D
as
.
Because angles, solid angles, and refractive indices are dimensionless quantities
, etendue has units of area (given by dS).
Conservation of etendue can be derived in different contexts, such as from from optical first principles, from Hamiltonian optics
or from the second law of thermodynamics
.
of refractive index n that is perfectly transparent (shown). To compute the etendue of the system, one must consider the contribution of each point on the surface of the light source as they cast rays to each point on the receiver.
According to the definition above, the etendue of the light crossing dΣ towards dS is given by:
where
is the solid angle defined by area dS at area dΣ. Accordingly, the etendue of the light crossing dS coming from dΣ is given by:
where
is the solid angle defined by area dΣ. These expressions result in 
showing that etendue is conserved as light propagates in free space.
The etendue of the whole system is then:
If both surfaces dΣ and dS are immersed in air (or in vacuum), n=1 and the expression above for the etendue may be written as
where
is the view factor between differential areas dΣ and dS. Integration on dΣ and dS results in 
which allows the etendue between two surfaces to be obtained from the view factors between those surfaces, as provided in a list of view factors for specific geometry cases or in several heat transfer
textbooks.
The conservation of etendue in free space is related to the reciprocity theorem for view factors.
is constant. However, etendue is also conserved in refractions and reflections. Figure "etendue in refraction" shows an infinitesimal surface dS on the xy plane separating two media of refractive indices nΣ and nS.
The normal to dS points in the direction of the z axis. Incoming light is confined to a solid angle dΩΣ and reaches dS at an angle θΣ to its normal. Refracted light is confined to a solid angle dΩS and leaves dS at an angle θS to its normal. The directions of the incoming and refracted light are contained in a plane making an angle φ to the z axis, defining these directions in a spherical coordinate system
. With these definitions, Snell's law
of refraction can be written as
and its derivative relative to θ
multiplied by each other result in
where both sides of the equation were also multiplied by dφ which does not change on refraction. This expression can now be written as
and multiplying both sides by dS we get
⇔ 
showing that the etendue of the light refracted at dS is conserved. The same result is also valid for the case of a reflection at a surface dS, in which case nΣ=nS and θΣ=θS.
is defined by
where n is the refractive index in which dS is immersed and d2Φ is the is the radiant flux
emitted by or crossing surface dS inside solid angle dΩ. As light travels through an ideal optical system, both the etendue and the energy flux are conserved. Therefore, the basic radiance defined as
is also conserved. In real systems etendue may increase (for example due to diffusion) or the light flux may decrease (for example due to absorption) and, therefore, basic radiance may decrease. However, etendue may not decrease and energy flux may not increase and, therefore, basic radiance may not increase.
, at a point in space, a light ray may be completely defined by a point P=(x,y,z), a unit Euclidean vector
indicating its direction and the refractive index n at point P. The optical momentum of the ray at that point is defined by
with
. The geometry of the optical momentum vector is illustrated in figure "optical momentum".
In a spherical coordinate system
p may be written as
from which
and therefore, for an infinitesimal area dS=dxdy on the xy plane immersed in a meduim of refractive index n, the etendue is given by
which is an infinitesimal volume in phase space x,y,p,q. Conservation or etendue in phase space is the equivalent in optics to Liouville's theorem
in classical mechanics. Etendue as volume in phase space is commonly used in nonimaging optics
.
Noting that
is the numerical aperture
, NA, of the beam of light, this can also be expressed as
.
Note that dΩ is expressed in a spherical coordinate system
. Now, if a large surface S is crossed by (or emits) light also confined to a cone of angle α, the etendue of the light crossing S is
The limit on maximum concentration (shown) is an optic with an entrance aperture, S, in air (nI=1) collecting light within a solid angle of angle 2α and sending it to a smaller area receiver Σ immersed in a medium of refractive index n, whose points are illuminated within a solid angle of angle 2β. From the above expression, the etendue of the incoming light is
and the etendue of the light reaching the receiver is
Conservation of etendue GI=GR then gives
where C is the concentration of the optic. For a given angular aperture, α, of the incoming light, this concentration will be maximum for the maximum value of β, that is β=π/2. The maximum possible concentration is then
In the case that the incident index is not unity, we have
and so
and in the best-case limit of
, this becomes
.
If the optic were a collimator
instead of a concentrator, the light direction is reversed and conservation of etendue gives us the minimum aperture, S, for a given output full angle 2α.
Optics
Optics is the branch of physics which involves the behavior and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behavior of visible, ultraviolet, and infrared light...
, which characterizes how "spread out" light
Light
Light or visible light is electromagnetic radiation that is visible to the human eye, and is responsible for the sense of sight. Visible light has wavelength in a range from about 380 nanometres to about 740 nm, with a frequency range of about 405 THz to 790 THz...
is in area and angle. It may also be seen as a volume in phase space.
From the source point of view, it is the area of the source times the solid angle
Solid angle
The solid angle, Ω, is the two-dimensional angle in three-dimensional space that an object subtends at a point. It is a measure of how large that object appears to an observer looking from that point...
the system's entrance pupil
Entrance pupil
In an optical system, the entrance pupil is the optical image of the physical aperture stop, as 'seen' through the front of the lens system. The corresponding image of the aperture as seen through the back of the lens system is called the exit pupil...
subtends as seen from the source. From the system point of view, the etendue is the area of the entrance pupil times the solid angle the source subtends as seen from the pupil. These definitions must be applied for infinitesimally small "elements" of area and solid angle, which must then be summed over both the source and the diaphragm as shown below.
Etendue is important because it never decreases in any optical system. A perfect optical system produces an image with the same etendue as the source. The etendue is related to the Lagrange invariant and the optical invariant, which share the property of being constant in an ideal optical system. The radiance
Radiance
Radiance and spectral radiance are radiometric measures that describe the amount of radiation such as light or radiant heat that passes through or is emitted from a particular area, and falls within a given solid angle in a specified direction. They are used to characterize both emission from...
of an optical system is equal to the derivative of the radiant flux
Radiant flux
In radiometry, radiant flux or radiant power is the measure of the total power of electromagnetic radiation...
with respect to the etendue.
The term étendue comes from the French word for extent or spread. The French word for the optical property is étendue géométrique, meaning "geometrical extent".
Other names for this property are acceptance, throughput, light-grasp, collecting power, and the AΩ product. Throughput and AΩ product are especially used in radiometry
Radiometry
In optics, radiometry is a set of techniques for measuring electromagnetic radiation, including visible light. Radiometric techniques characterize the distribution of the radiation's power in space, as opposed to photometric techniques, which characterize the light's interaction with the human eye...
and radiative transfer where it is related to the view factor (or shape factor). It is a central concept in nonimaging optics
Nonimaging optics
Nonimaging optics is the branch of optics concerned with the optimal transfer of light radiation between a source and a target...
.
Definition
An infinitesimal surface element, dS, with normal nS is immersed in a medium of refractive indexRefractive index
In optics the refractive index or index of refraction of a substance or medium is a measure of the speed of light in that medium. It is expressed as a ratio of the speed of light in vacuum relative to that in the considered medium....
n. The surface is crossed by (or emits) light confined to a solid angle, dΩ, at an angle θ with the normal nS . The area of dS projected in the direction of the light propagation is
. The etendue of this light crossing dS is defined in 2DTwo-dimensional space
- Details :Bi-dimensional space is a geometric model of the planar projection of the physical universe in which we live.The two dimensions are commonly called length and width .Both directions lies in the same plane....
as
and in 3D
Three-dimensional space
Three-dimensional space is a geometric 3-parameters model of the physical universe in which we live. These three dimensions are commonly called length, width, and depth , although any three directions can be chosen, provided that they do not lie in the same plane.In physics and mathematics, a...
as
.Because angles, solid angles, and refractive indices are dimensionless quantities
Dimensionless quantity
In dimensional analysis, a dimensionless quantity or quantity of dimension one is a quantity without an associated physical dimension. It is thus a "pure" number, and as such always has a dimension of 1. Dimensionless quantities are widely used in mathematics, physics, engineering, economics, and...
, etendue has units of area (given by dS).
Conservation of etendue
As shown below, etendue is conserved as light travels through free space and at refractions or reflections. It is then also conserved as light travels through optical systems where it suffers perfect reflections or refractions. However, if light was to hit, say, a diffuser, its solid angle would increase, increasing the etendue. Etendue can then remain constant or it can increase as light propagates through an optic, but it cannot decrease.Conservation of etendue can be derived in different contexts, such as from from optical first principles, from Hamiltonian optics
Hamiltonian optics
Lagrangian optics and Hamiltonian optics are two formulations of geometrical optics which share much of the mathematical formalism with Lagrangian mechanics and Hamiltonian mechanics.-Hamilton's principle:...
or from the second law of thermodynamics
Second law of thermodynamics
The second law of thermodynamics is an expression of the tendency that over time, differences in temperature, pressure, and chemical potential equilibrate in an isolated physical system. From the state of thermodynamic equilibrium, the law deduced the principle of the increase of entropy and...
.
Conservation of etendue in free space
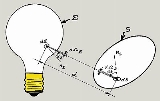
Consider a light source, Σ, and a light "receiver", S, both of which are extended surfaces (rather than differential elements), and which are separated by a mediumMedium (optics)
An optical medium is material through which electromagnetic waves propagate. It is a form of transmission medium. The permittivity and permeability of the medium define how electromagnetic waves propagate in it...
of refractive index n that is perfectly transparent (shown). To compute the etendue of the system, one must consider the contribution of each point on the surface of the light source as they cast rays to each point on the receiver.
According to the definition above, the etendue of the light crossing dΣ towards dS is given by:
where
is the solid angle defined by area dS at area dΣ. Accordingly, the etendue of the light crossing dS coming from dΣ is given by:where
is the solid angle defined by area dΣ. These expressions result in showing that etendue is conserved as light propagates in free space.The etendue of the whole system is then:
If both surfaces dΣ and dS are immersed in air (or in vacuum), n=1 and the expression above for the etendue may be written as
where
is the view factor between differential areas dΣ and dS. Integration on dΣ and dS results in which allows the etendue between two surfaces to be obtained from the view factors between those surfaces, as provided in a list of view factors for specific geometry cases or in several heat transferHeat transfer
Heat transfer is a discipline of thermal engineering that concerns the exchange of thermal energy from one physical system to another. Heat transfer is classified into various mechanisms, such as heat conduction, convection, thermal radiation, and phase-change transfer...
textbooks.
The conservation of etendue in free space is related to the reciprocity theorem for view factors.
Conservation of etendue in refractions and reflections
The conservation of etendue discussed above applies to the case of light propagation in free space, or more generally, in a medium in which the refractive indexRefractive index
In optics the refractive index or index of refraction of a substance or medium is a measure of the speed of light in that medium. It is expressed as a ratio of the speed of light in vacuum relative to that in the considered medium....
is constant. However, etendue is also conserved in refractions and reflections. Figure "etendue in refraction" shows an infinitesimal surface dS on the xy plane separating two media of refractive indices nΣ and nS.
The normal to dS points in the direction of the z axis. Incoming light is confined to a solid angle dΩΣ and reaches dS at an angle θΣ to its normal. Refracted light is confined to a solid angle dΩS and leaves dS at an angle θS to its normal. The directions of the incoming and refracted light are contained in a plane making an angle φ to the z axis, defining these directions in a spherical coordinate system
Spherical coordinate system
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its inclination angle measured from a fixed zenith direction, and the azimuth angle of...
. With these definitions, Snell's law
Snell's law
In optics and physics, Snell's law is a formula used to describe the relationship between the angles of incidence and refraction, when referring to light or other waves passing through a boundary between two different isotropic media, such as water and glass...
of refraction can be written as
and its derivative relative to θ
multiplied by each other result in
where both sides of the equation were also multiplied by dφ which does not change on refraction. This expression can now be written as
and multiplying both sides by dS we get
⇔ showing that the etendue of the light refracted at dS is conserved. The same result is also valid for the case of a reflection at a surface dS, in which case nΣ=nS and θΣ=θS.
Conservation of basic radiance
RadianceRadiance
Radiance and spectral radiance are radiometric measures that describe the amount of radiation such as light or radiant heat that passes through or is emitted from a particular area, and falls within a given solid angle in a specified direction. They are used to characterize both emission from...
is defined by
where n is the refractive index in which dS is immersed and d2Φ is the is the radiant flux
Radiant flux
In radiometry, radiant flux or radiant power is the measure of the total power of electromagnetic radiation...
emitted by or crossing surface dS inside solid angle dΩ. As light travels through an ideal optical system, both the etendue and the energy flux are conserved. Therefore, the basic radiance defined as
is also conserved. In real systems etendue may increase (for example due to diffusion) or the light flux may decrease (for example due to absorption) and, therefore, basic radiance may decrease. However, etendue may not decrease and energy flux may not increase and, therefore, basic radiance may not increase.
Etendue as a volume in phase space
In the context of Hamiltonian opticsHamiltonian optics
Lagrangian optics and Hamiltonian optics are two formulations of geometrical optics which share much of the mathematical formalism with Lagrangian mechanics and Hamiltonian mechanics.-Hamilton's principle:...
, at a point in space, a light ray may be completely defined by a point P=(x,y,z), a unit Euclidean vector
indicating its direction and the refractive index n at point P. The optical momentum of the ray at that point is defined bywith
. The geometry of the optical momentum vector is illustrated in figure "optical momentum".In a spherical coordinate system
Spherical coordinate system
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its inclination angle measured from a fixed zenith direction, and the azimuth angle of...
p may be written as
from which
and therefore, for an infinitesimal area dS=dxdy on the xy plane immersed in a meduim of refractive index n, the etendue is given by
which is an infinitesimal volume in phase space x,y,p,q. Conservation or etendue in phase space is the equivalent in optics to Liouville's theorem
Liouville's theorem (Hamiltonian)
In physics, Liouville's theorem, named after the French mathematician Joseph Liouville, is a key theorem in classical statistical and Hamiltonian mechanics...
in classical mechanics. Etendue as volume in phase space is commonly used in nonimaging optics
Nonimaging optics
Nonimaging optics is the branch of optics concerned with the optimal transfer of light radiation between a source and a target...
.
Maximum concentration
Consider an infinitesimal area, dS, immersed in a medium of refractive index n crossed by (or emitting) light inside a cone of angle α. The etendue of this light is given by Noting that
is the numerical apertureNumerical aperture
In optics, the numerical aperture of an optical system is a dimensionless number that characterizes the range of angles over which the system can accept or emit light. By incorporating index of refraction in its definition, NA has the property that it is constant for a beam as it goes from one...
, NA, of the beam of light, this can also be expressed as
.Note that dΩ is expressed in a spherical coordinate system
Spherical coordinate system
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the radial distance of that point from a fixed origin, its inclination angle measured from a fixed zenith direction, and the azimuth angle of...
. Now, if a large surface S is crossed by (or emits) light also confined to a cone of angle α, the etendue of the light crossing S is
The limit on maximum concentration (shown) is an optic with an entrance aperture, S, in air (nI=1) collecting light within a solid angle of angle 2α and sending it to a smaller area receiver Σ immersed in a medium of refractive index n, whose points are illuminated within a solid angle of angle 2β. From the above expression, the etendue of the incoming light is
and the etendue of the light reaching the receiver is
Conservation of etendue GI=GR then gives
where C is the concentration of the optic. For a given angular aperture, α, of the incoming light, this concentration will be maximum for the maximum value of β, that is β=π/2. The maximum possible concentration is then
In the case that the incident index is not unity, we have
and so
and in the best-case limit of
, this becomes.If the optic were a collimator
Collimator
A collimator is a device that narrows a beam of particles or waves. To "narrow" can mean either to cause the directions of motion to become more aligned in a specific direction or to cause the spatial cross section of the beam to become smaller.- Optical collimators :In optics, a collimator may...
instead of a concentrator, the light direction is reversed and conservation of etendue gives us the minimum aperture, S, for a given output full angle 2α.
Further reading
- Xutao Sun et al., 2006, "Etendue analysis and measurement of light source with elliptical reflector", Displays (27), 56–61.