In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, the Dandelin spheres are one or two sphere

Sphere

A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...

s that are tangent

Tangent

In geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...

 both to a plane

Plane

-Physical objects:* Aeroplane or airplane, a fixed-wing aircraft* Plane , a woodworking tool to smooth surfaces* Platanus, a genus of trees with the common name "plane"* Acer pseudoplatanus, a tree species sometimes called "plane"...

 and to a cone

Cone

A cone is a basic geometrical shape; see cone .Cone may also refer to:-Mathematics:*Cone , a family of morphisms resembling a geometric cone...

 that intersects the plane. The intersection of the cone and the plane is a conic section

Conic section

In mathematics, a conic section is a curve obtained by intersecting a cone with a plane. In analytic geometry, a conic may be defined as a plane algebraic curve of degree 2...

, and the point at which either sphere touches the plane is a focus

Focus (geometry)

In geometry, the foci are a pair of special points with reference to which any of a variety of curves is constructed. For example, foci can be used in defining conic sections, the four types of which are the circle, ellipse, parabola, and hyperbola...

 of the conic section, so the Dandelin spheres are also sometimes called focal spheres.

The Dandelin spheres were discovered in 1822. They are named in honor of the Belgian

Belgium

Belgium , officially the Kingdom of Belgium, is a federal state in Western Europe. It is a founding member of the European Union and hosts the EU's headquarters, and those of several other major international organisations such as NATO.Belgium is also a member of, or affiliated to, many...

 mathematician Germinal Pierre Dandelin

Germinal Pierre Dandelin

Germinal Pierre Dandelin was a mathematician, soldier, and professor of engineering. He was born near Paris to a French father and Belgian mother, studying first at Ghent then returning to Paris to study at the École Polytechnique. He was wounded fighting under Napoleon. He worked for the...

, though Adolphe Quetelet

Adolphe Quetelet

Lambert Adolphe Jacques Quetelet was a Belgian astronomer, mathematician, statistician and sociologist. He founded and directed the Brussels Observatory and was influential in introducing statistical methods to the social sciences...

 is sometimes given partial credit as well. The Dandelin spheres can be used to prove at least two important theorems. Both of those theorems were known for centuries before Dandelin, but he made it easier to prove them.

The first theorem is that a closed conic section (i.e. an ellipse

Ellipse

In geometry, an ellipse is a plane curve that results from the intersection of a cone by a plane in a way that produces a closed curve. Circles are special cases of ellipses, obtained when the cutting plane is orthogonal to the cone's axis...

) is the locus

Locus (mathematics)

In geometry, a locus is a collection of points which share a property. For example a circle may be defined as the locus of points in a plane at a fixed distance from a given point....

 of points such that the sum of the distances to two fixed points (the foci) is constant. This was known to Ancient Greek

Ancient Greece

Ancient Greece is a civilization belonging to a period of Greek history that lasted from the Archaic period of the 8th to 6th centuries BC to the end of antiquity. Immediately following this period was the beginning of the Early Middle Ages and the Byzantine era. Included in Ancient Greece is the...

 mathematicians such as Apollonius of Perga

Apollonius of Perga

Apollonius of Perga [Pergaeus] was a Greek geometer and astronomer noted for his writings on conic sections. His innovative methodology and terminology, especially in the field of conics, influenced many later scholars including Ptolemy, Francesco Maurolico, Isaac Newton, and René Descartes...

, but the Dandelin spheres facilitate the proof.

The second theorem is that for any conic section, the distance from a fixed point (the focus) is proportional to the distance from a fixed line (the directrix), the constant of proportionality being called the eccentricity

Eccentricity (mathematics)

In mathematics, the eccentricity, denoted e or \varepsilon, is a parameter associated with every conic section. It can be thought of as a measure of how much the conic section deviates from being circular.In particular,...

. Again, this theorem was known to the Ancient Greeks, such as Pappus of Alexandria

Pappus of Alexandria

Pappus of Alexandria was one of the last great Greek mathematicians of Antiquity, known for his Synagoge or Collection , and for Pappus's Theorem in projective geometry...

, but the Dandelin spheres facilitate the proof.

A conic section has one Dandelin sphere for each focus. In particular, an ellipse has two Dandelin spheres, both touching the same nappe

Nappe (disambiguation)

In geology a nappe is a complex recumbent fold system.In geometry, a nappe is half of a double cone.In engineering a nappe can also refer to a sheet of water flowing over a dam or similar structure....

 of the cone. A hyperbola

Hyperbola

In mathematics a hyperbola is a curve, specifically a smooth curve that lies in a plane, which can be defined either by its geometric properties or by the kinds of equations for which it is the solution set. A hyperbola has two pieces, called connected components or branches, which are mirror...

 has two Dandelin spheres, touching opposite nappes of the cone. A parabola

Parabola

In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...

 has just one Dandelin sphere.

Proof that the curve has constant sum of distances to foci

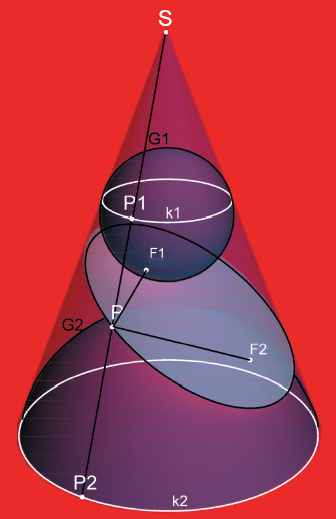
Consider the illustration, depicting a plane intersecting a cone to form an ellipse (the interior of the ellipse is colored light blue). The two Dandelin spheres are shown, one (G1) above the ellipse, and one (G2) below. The intersection of each sphere with the cone is a circle (colored white).

• Each sphere touches the plane at a point, and let us call those two points F₁ and F₂.
• Let P be a typical point on the ellipse.
• Prove: The sum of distances d(F₁, P) + d(F₂, P) remain constant as the point P moves along the curve.
  • A line passing through P and the vertex
    Apex (geometry)
    In geometry, an apex is the vertex which is in some sense the highest of the figure to which it belongs.*In an isosceles triangle, the apex is the vertex where the two sides of equal length meet, opposite the unequal third side....
    
     S of the cone intersects the two circles at points P₁ and P₂.
  • As P moves along the ellipse, P₁ and P₂ move along the two circles.
  • The distance from F₁ to P is the same as the distance from P₁ to P, because lines PF₁ and PP₁ are both tangent
    Tangent
    In geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...
    
     to the same sphere (G1).
  • Likewise, the distance from F₂ to P is the same as the distance from P₂ to P, because lines PF₂ and PP₂ are both tangent
    Tangent
    In geometry, the tangent line to a plane curve at a given point is the straight line that "just touches" the curve at that point. More precisely, a straight line is said to be a tangent of a curve at a point on the curve if the line passes through the point on the curve and has slope where f...
    
     to the same sphere (G2).
  • Consequently, the sum of distances d(F₁, P) + d(F₂, P) must be constant as P moves along the curve because the sum of distances d(P₁, P) + d(P₂, P) also remains constant.
    • This follows from the fact that P lies on the straight line from P₁ to P₂, and the distance from P₁ to P₂ remains constant.

This proves a result that had been proved in a different manner by Apollonius of Perga

Apollonius of Perga

Apollonius of Perga [Pergaeus] was a Greek geometer and astronomer noted for his writings on conic sections. His innovative methodology and terminology, especially in the field of conics, influenced many later scholars including Ptolemy, Francesco Maurolico, Isaac Newton, and René Descartes...

.

If (as is often done) one takes the definition of the ellipse to be the locus of points P such that d(F₁, P) + d(F₂, P) = a constant, then the argument above proves that the intersection of a plane with a cone is indeed an ellipse. That the intersection of the plane with the cone is symmetric about the perpendicular bisector of the line through F₁ and F₂ may be counterintuitive, but this argument makes it clear.

Adaptations of this argument work for hyperbolas and parabolas as intersections of a plane with a cone. Another adaptation works for an ellipse realized as the intersection of a plane with a right circular cylinder

Cylinder (geometry)

A cylinder is one of the most basic curvilinear geometric shapes, the surface formed by the points at a fixed distance from a given line segment, the axis of the cylinder. The solid enclosed by this surface and by two planes perpendicular to the axis is also called a cylinder...

.

Proof of the focus-directrix property

The directrix of a conic section can be found using Dandelin's construction. Each Dandelin sphere intersects the cone at a circle; let both of these circles define their own planes. The intersections of these two planes with the conic section's plane will be two parallel lines; these lines are the directrices of the conic section. However, a parabola has only one Dandelin sphere, and thus has only one directrix.

Using the Dandelin spheres, it can be proved that any conic section is the locus of points for which the distance from a point (focus) is proportional to the distance from the directrix. Ancient Greek mathematicians such as Pappus of Alexandria

Pappus of Alexandria

Pappus of Alexandria was one of the last great Greek mathematicians of Antiquity, known for his Synagoge or Collection , and for Pappus's Theorem in projective geometry...

 were aware of this property, but the Dandelin spheres facilitate the proof.

Neither Dandelin nor Quetelet used the Dandelin spheres to prove the focus-directrix property. The first to do so was apparently Pierce Morton in 1829. The focus-directrix property is essential to proving that astronomical objects move along conic sections around the Sun.

External links

• Dandelin Spheres page by Hop David
• Math Academy page on Dandelin's spheres
• Java applet JDandelin